Submit Manuscript
Peer Review
Editor Work
| Citation: | YANG Chen, WU Lei, YANG Wei, et al. Cross-ambiguity function shaping through fractional quadratic programming[J]. Journal of Radars, 2024, 13(1): 174–186. doi: 10.12000/JR23126
|
Cross-ambiguity Function Shaping Through Fractional Quadratic Programming
doi: 10.12000/JR23126
More Information-
Abstract
Due to the mismatch between transmit waveforms and receive filters, Cross-Ambiguity Function (CAF) shaping plays an important role in the design of cognitive radar waveforms and allows more freedom for waveform optimization problem than conventional ambiguity function shaping. A CAF shaping method is proposed for designing phase-shift keying transmit waveforms and receive filters jointly to maximize the output Signal-to-Interference-plus-Noise Ratio (SINR), thereby solving the problem of weaking-moving target detection under strong clutter conditions. The optimization problem is first modeled as a quadratic fractional programming problem under the Constant Modulus (CM) constraint of the transmit waveform. The conjugated gradient method is utilized to solve the minimization problem of the Stiefel manifold space through the introduction of auxiliary variables; furthermore the nonconvex optimization problem is converted into a unimodular quadratic programming problem. An algorithm based on alternately iterative maximization and power method-like iteration is proposed to solve the quadratic optimization problem. Since transmit waveforms are limited by hardware and achieving CM is difficult, the nearest vector method is employed under the constraint of a low peak-to-average power ratio. Finally, the experiments with simulated and real measured data under different parameters reveal that the transmit waveforms and receive filters designed using the proposed method exhibit better SINR performance and faster convergence speed compared with other existing algorithms. -
-
References
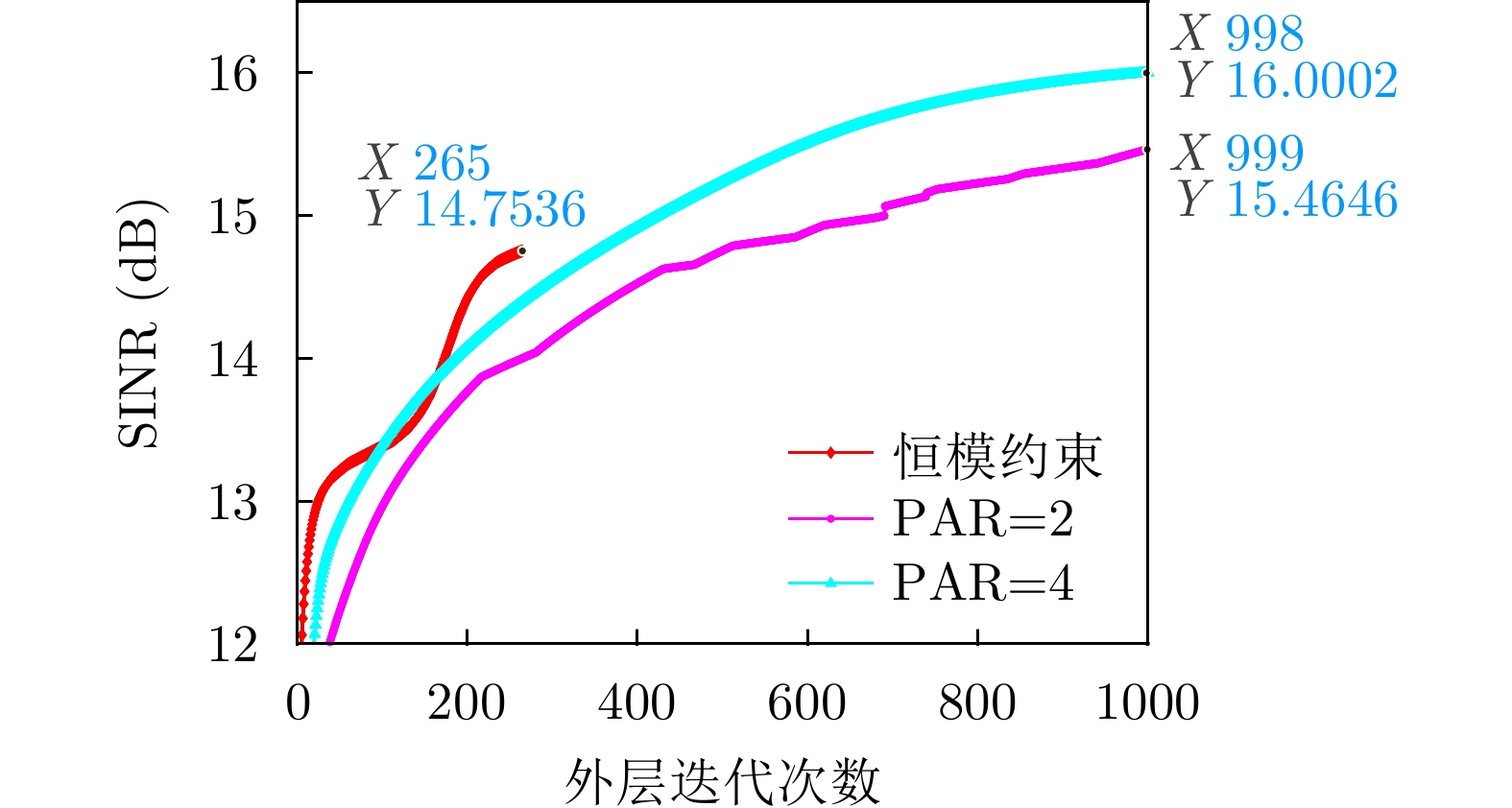
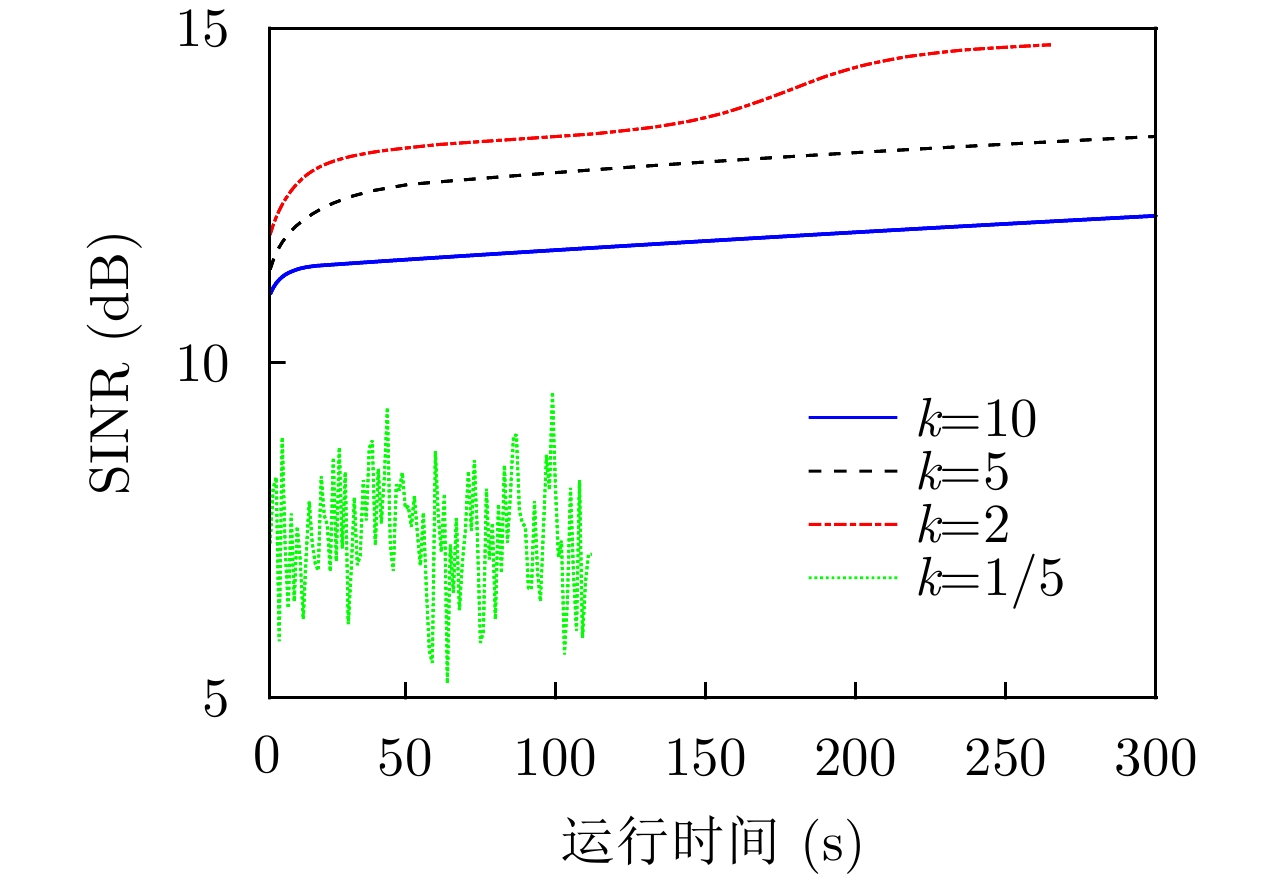
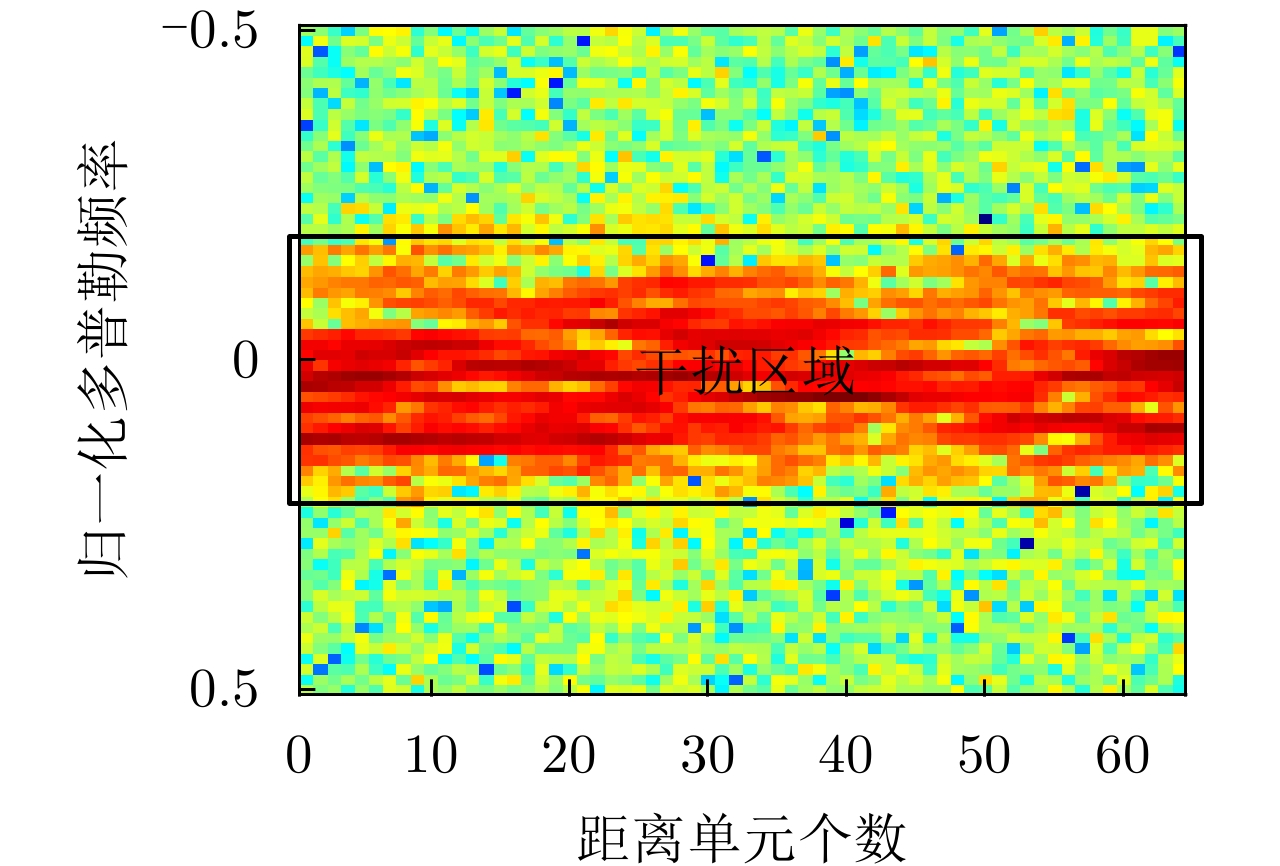
[1] STOICA P, HE Hao, and LI Jian. Optimization of the receive filter and transmit sequence for active sensing[J]. IEEE Transactions on Signal Processing, 2012, 60(4): 1730–1740. doi: 10.1109/TSP.2011.2179652[2] STOICA P, LI Jian, and XUE Ming. Transmit codes and receive filters for radar[J]. IEEE Signal Processing Magazine, 2008, 25(6): 94–109. doi: 10.1109/MSP.2008.929231[3] 余若峰, 杨威, 付耀文, 等. 面向不同雷达任务的认知波形优化综述[J]. 电子学报, 2022, 50(3): 726–752. doi: 10.12263/DZXB.20211068YU Ruofeng, YANG Wei, FU Yaowen, et al. A review on cognitive waveform optimization for different radar missions[J]. Acta Electronica Sinica, 2022, 50(3): 726–752. doi: 10.12263/DZXB.20211068[4] LEVANON N and MOZESON E. Radar Signals[M]. New York: John Wiley & Sons, 2004: 7–9.[5] CUI Guolong, FU Yue, YU Xianxiang, et al. Local ambiguity function shaping via uni-modular sequence design[J]. IEEE Signal Processing Letters, 2017, 24(7): 977–981. doi: 10.1109/lsp.2017.2700396[6] HAYKIN S. Cognitive radar: A way of the future[J]. IEEE Signal Processing Magazine, 2006, 23(1): 30–40. doi: 10.1109/msp.2006.1593335[7] ARLERY F, KASSAB R, TAN U, et al. Efficient gradient method for locally optimizing the periodic/aperiodic ambiguity function[C]. IEEE Radar Conference (RadarConf), Philadelphia, USA, 2016: 1–6[8] ARLERY F, KASSAB R, TAN U, et al. Efficient optimization of the ambiguity functions of multi-static radar waveforms[C]. 17th International Radar Symposium (IRS), Krakow, Poland, 2016: 1–6.[9] WANG Fulai, FENG Sijia, YIN Jiapeng, et al. Unimodular sequence and receiving filter design for local ambiguity function shaping[J]. IEEE Transactions on Geoscience and Remote Sensing, 2022, 60: 5113012. doi: 10.1109/tgrs.2022.3171253[10] AUBRY A, DE MAIO A, JIANG Bo, et al. Ambiguity function shaping for cognitive radar via complex quartic optimization[J]. IEEE Transactions on Signal Processing, 2013, 61(22): 5603–5619. doi: 10.1109/TSP.2013.2273885[11] QIU Xiangfeng, JIANG Weidong, ZHANG Xinyu, et al. Quartic riemannian trust region algorithm for cognitive radar ambiguity function shaping[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4022005. doi: 10.1109/LGRS.2022.3151679[12] WU Linlong, BABU P, and PALOMAR D P. Cognitive radar-based sequence design via sinr maximization[J]. IEEE Transactions on Signal Processing, 2017, 65(3): 779–793. doi: 10.1109/tsp.2016.2621723[13] HE Hao, STOICA P, and LI Jian. On synthesizing cross ambiguity functions[C]. IEEE Internationl Conference on Acoustics, Speech and Signal Processing (ICASSP), Prague, Czech Republic, 2011: 3536–3539[14] CHEN Zihao, LIANG Junli, WANG Tao, et al. Generalized MBI algorithm for designing sequence set and mismatched filter bank with ambiguity function constraints[J]. IEEE Transactions on Signal Processing, 2022, 70: 2918–2933. doi: 10.1109/TSP.2022.3181346[15] HE Hao, LI Jian, and STOICA P. Waveform Design for Active Sensing Systems: A Computational Approach[M]. Cambridge: Cambridge University Press, 2012: 106–121.[16] SOLTANALIAN M, TANG Bo, LI Jian, et al. Joint design of the receive filter and transmit sequence for active sensing[J]. IEEE Signal Processing Letters, 2013, 20(5): 423–426. doi: 10.1109/lsp.2013.2250279[17] ESMAEILI-NAJAFABADI H, LEUNG H, and MOO P W. Unimodular waveform design with desired ambiguity function for cognitive radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2020, 56(3): 2489–2496. doi: 10.1109/taes.2019.2942411[18] 付月, 崔国龙, 余显祥. 信号相关杂波背景下稳健的恒模序列与接收滤波器设计方法[J]. 雷达学报, 2017, 6(3): 292–299. doi: 10.12000/JR16158FU Yue, CUI Guolong, and YU Xianxiang. Robust design of constant modulus sequence and receiver filter in the presence of signal-dependent clutter[J]. Journal of Radars, 2017, 6(3): 292–299. doi: 10.12000/JR16158[19] LIU Tianjun, FAN Pingzhi, ZHOU Zhengchun, et al. Unimodular sequence design with good local auto- and cross-ambiguity function for MSPSR system[C], IEEE 89th Vehicular Technology Conference (VTC2019-Spring), Kuala Lumpur, Malaysia, 2019: 1–5.[20] WU Linlong, BABU P, and PALOMAR D P. Transmit waveform/receive filter design for MIMO radar with multiple waveform constraints[J]. IEEE Transactions on Signal Processing, 2018, 66(6): 1526–1540. doi: 10.1109/tsp.2017.2787115[21] 邱祥风, 姜卫东, 张新禹, 等. 认知MIMO雷达发射波形与接收滤波器联合优化设计方法[J]. 系统工程与电子技术, 2023, 45(2): 386–393. doi: 10.12305/j.issn.1001-506X.2023.02.08QIU Xiangfeng, JIANG Weidong, ZHANG Xinyu, et al. Joint optimization design method for cognitive MIMO radar transmit waveform and receive filter[J]. Systems Engineering and Electronics, 2023, 45(2): 386–393. doi: 10.12305/j.issn.1001-506X.2023.02.08[22] AUBRY A, DEMAIO A, FARINA A, et al. Knowledge-aided (potentially cognitive) transmit signal and receive filter design in signal-dependent clutter[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(1): 93–117. doi: 10.1109/TAES.2013.6404093[23] SOLTANALIAN M and STOICA P. Designing unimodular codes via quadratic optimization[J]. IEEE Transactions on Signal Processing, 2014, 62(5): 1221–1234. doi: 10.1109/tsp.2013.2296883[24] GHARANJIK A, SOLTANALIAN M, SHANKAR M R B, et al. Grab-n-Pull: A max-min fractional quadratic programming framework with applications in signal and information processing[J]. Signal Processing, 2019, 160: 1–12. doi: 10.1016/j.sigpro.2019.02.006[25] TROPP J A, DHILLON I S, HEATH R W, et al. Designing structured tight frames via an alternating projection method[J]. IEEE Transactions on Information Theory, 2005, 51(1): 188–209. doi: 10.1109/tit.2004.839492[26] 张贤达. 矩阵分析与应用[M]. 2版. 北京: 清华大学出版社, 2013: 447–450.ZHANG Xianda. Matrix Analysis and Applications[M]. 2nd ed, Beijing: Tsinghua University Press, 2013: 447–450. -
Proportional views
- Publishing Ethics
- Journal Insights
- Abstracting & Indexing
- Peer Review Policies
- Guide for Authors
- ISSN 2095-283X (Print)ISSN 2097-339X (Online)
- CN 10-1030/TN
- CODEN LXEUAO
About Journal
- Sponsor: China Radio Detection and Ranging Industry Association (CRIA)
- Phone: 010-58887062
- Email：radars@aircas.ac.cn
- Publisher: Leida Xuebao Bianjibu (Editorial office of the Journal of Radars)
Contacts Us
京ICP备20021838号-8
Supported by: Beijing Renhe Information Technology Co. Ltd
Export File
Citation
Format
Content
DownLoad:
- Figure 1. Interference energy distribution
- Figure 2. The convergence curve of objective function response value in the proposed algorithm
- Figure 3. SINR versus the iteration times of different algorithms
- Figure 4. CAF generated by five different algorithms
-
Figure 5. Distance cut (
$r = 1,2,3$ $N{\text{ = 50}}$ - Figure 6. SINR of five different algorithms versus code length
- Figure 7. The real and imaginary parts of transmitting waveform under different PAR constraints
- Figure 8. SINR versus the iteration time under different PAR constraints
- Figure 9. SINR with respect to running time under different values of k
- Figure 10. Range-Doppler diagram of real measured data from an airport in Hainan
- Figure 11. Results of applying Alg. 1 to the real measured data