Volume 7 Issue 4
Aug.  2018
Turn off MathJax
Article Contents
Abstract
References
Article Navigation >  Journal of Radars  > 2018  >  7(4): 523-530
WANG Xuesong and CHEN Siwei. Polarimetric synthetic aperture radar interpretation and recognition: Advances and perspectives[J]. Journal of Radars, 2020, 9(2): 259–276. doi: 10.12000/JR19109
Citation: Chen Fangxiang, Yi Wei, Zhou Tao, Kong Lingjiang. Passive Direct Location Determination for Multiple Sources Based on FRFT[J]. Journal of Radars, 2018, 7(4): 523-530. doi: 10.12000/JR18027
shu

Passive Direct Location Determination for Multiple Sources Based on FRFT

DOI: 10.12000/JR18027

  • School of Information and Communication Engineering, University of Electronic Science and Technology of China, Chengdu 611731, China

Funds:  The National Natural Science Foundation of China (61771110), The Chang Jiang Scholars Program, The 111 Project (B17008), The Fundamental Research Funds of Central Universities (ZYGX2016J031), The Chinese Postdoctoral Science Foundation (2014M550465), Special Grant (2016T90845)
  • Received Date: 2018-03-27
  • Rev Recd Date: 2018-06-22
  • Publish Date: 2018-08-28
    Abstract
  • The Direct Position Determination (DPD) method can fully use the information of an observed signal, and it is known to outperform the traditional two-step methods at a low Signal-to-Noise Ratio (SNR). To solve the problem of localizing multiple transmitters with unknown Linear Frequency Modulation (LFM) signals in multi-static passive radar systems, a multi-target positioning algorithm based on DPD algorithm and FRactional Fourier Transform (FRFT) is proposed. First, according to the established signal model, the theoretically optimal high-dimensional maximum likelihood estimator is deduced; Then, to address the high computational complexity of the estimator in jointly estimating high-dimensional signal parameters and positions of sources, we propose a dimensionality reduction strategy based on the FRFT and some basic classification algorithms to transform the multi-target localizing problem to a multiple single-target localizing problem. Through this method, the position and the corresponding signal parameters of each source can be efficiently estimated by a four-dimensional grid search. Simulation results show that the proposed method outperforms the existing DPD algorithm, and it can determine the positions of sources without directly utilizing the transmitted signal information.

     

    • FullText(HTML)
    • References(17)
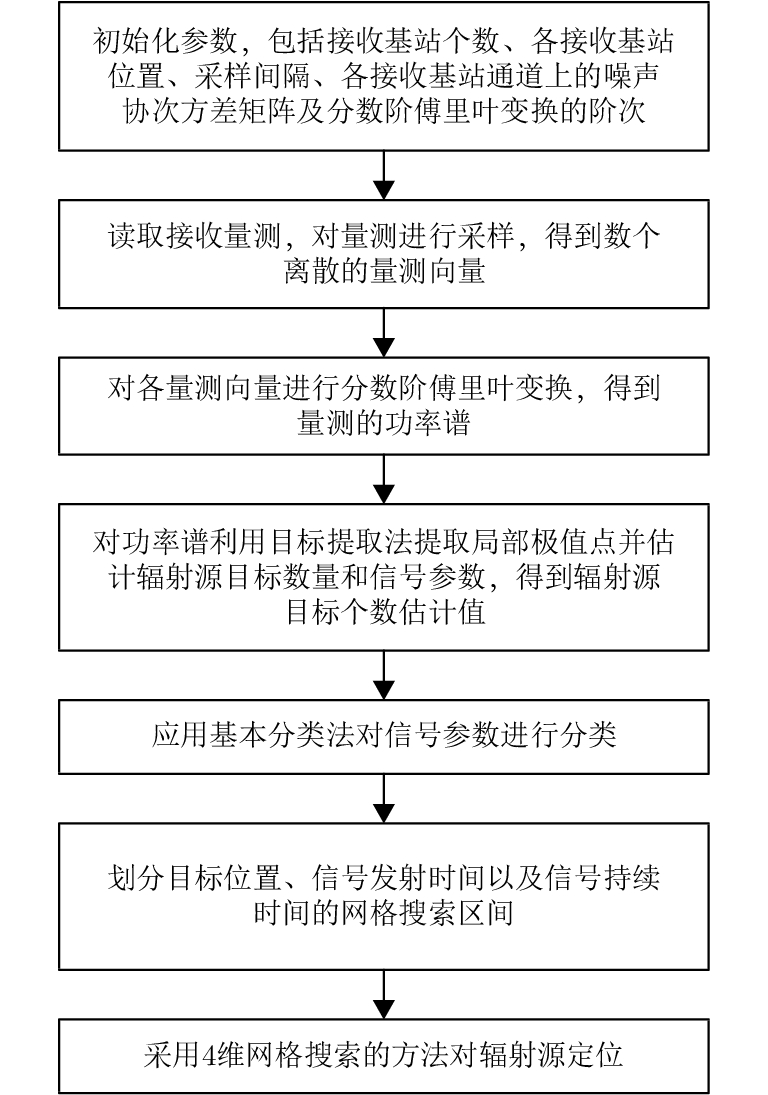
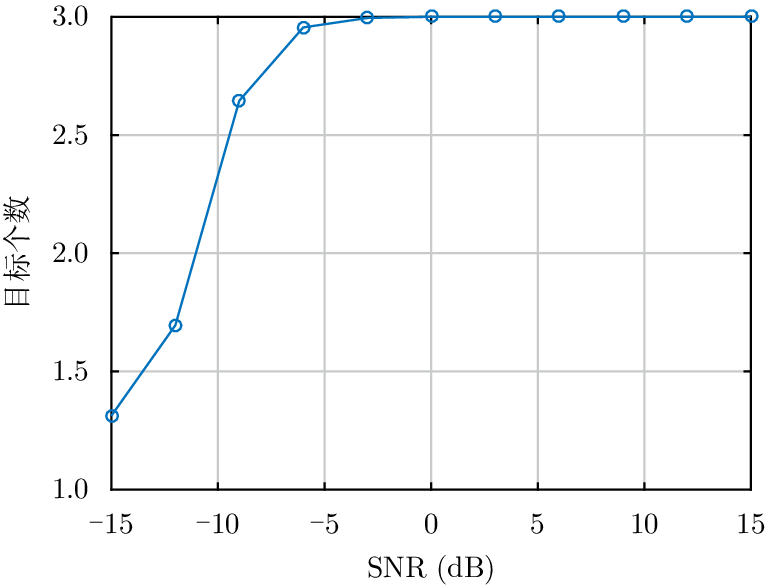
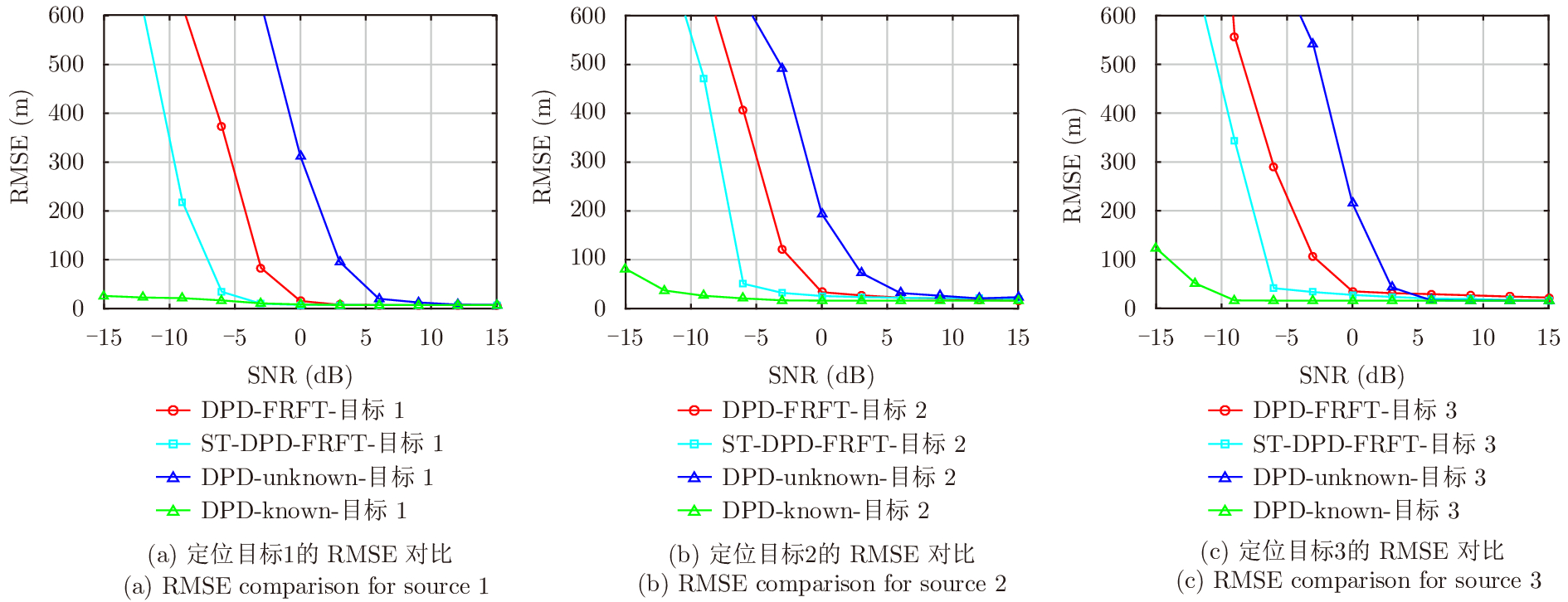
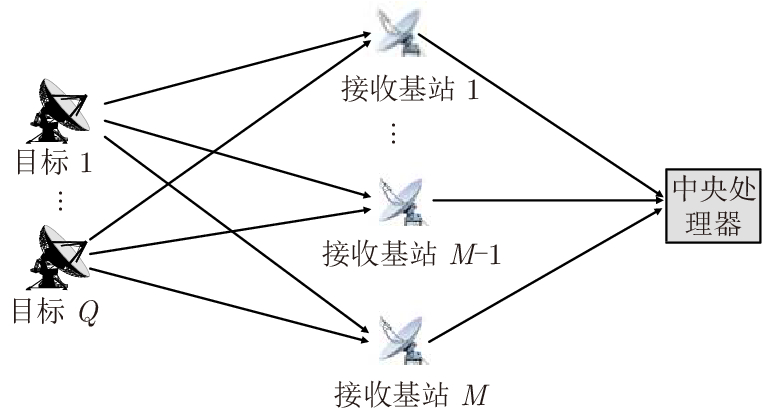
  • [1]
    Amar A and Weiss A J. Localization of narrowband radio emitters based on Doppler frequency shifts[J]. IEEE Transactions on Signal Processing, 2008, 56(11): 5500–5508. DOI: 10.1109/TSP.2008.929655
    [2]
    贾兴江. 运动多站无源定位关键技术研究[D]. [博士论文], 国防科学技术大学, 2011: 3–42

    Jia Xing-jiang. Research on passive location technologies of multiple moving observers[D]. [Ph.D. dissertation], National University of Defense Technology, 2011: 3–42
    [3]
    Tirer T and Weiss A J. High resolution localization of narrowband radio emitters based on Doppler frequency shifts[J]. Signal Processing, 2017, 141: 288–298. DOI: 10.1016/j.sigpro.2017.06.019
    [4]
    赵勇胜, 赵拥军, 赵闯. 联合角度和时差的单站无源相干定位加权最小二乘算法[J]. 雷达学报, 2016, 5(3): 302–311. DOI: 10.12000/JR15133

    Zhao Yong-sheng, Zhao Yong-jun, and Zhao Chuang. Weighted least squares algorithm for single-observer passive coherent location using DOA and TDOA measurements[J]. Journal of Radars, 2016, 5(3): 302–311. DOI: 10.12000/JR15133
    [5]
    Weiss A J. Direct position determination of narrowband radio frequency transmitters[J]. IEEE Signal Processing Letters, 2004, 11(5): 513–516. DOI: 10.1109/LSP.2004.826501
    [6]
    Yi W, Chen Z H, Hoseinnezhad R, et al. Joint estimation of location and signal parameters for an LFM emitter[J]. Signal Processing, 2017, 134: 100–112. DOI: 10.1016/j.sigpro.2016.11.014
    [7]
    Oispuu M and Nickel U. Direct detection and position determination of multiple sources with intermittent emission[J]. Signal Processing, 2010, 90(12): 3056–3064. DOI: 10.1016/j.sigpro.2010.05.010
    [8]
    Ozaktas H M, Arikan O, Kutay M A, et al. Digital computation of the fractional Fourier transform[J]. IEEE Transactions on Signal Processing, 1996, 44(9): 2141–2150. DOI: 10.1109/78.536672
    [9]
    Saxena R and Singh K. Fractional Fourier transform: A novel tool for signal processing[J]. Journal of the Indian Institute of Science, 2005, 85(1): 11–26.
    [10]
    Namias V. The fractional order Fourier transform and its application to quantum mechanics[J]. IMA Journal of Applied Mathematics, 1980, 25(3): 241–265. DOI: 10.1093/imamat/25.3.241
    [11]
    田瑞琦, 鲍庆龙, 王丁禾, 等. 基于FRFT与Keystone变换的运动目标参数估计算法[J]. 雷达学报, 2014, 3(5): 511–517. DOI: 10.3724/SP.J.1300.2014.14058

    Tian Rui-qi, Bao Qing-long, Wang Ding-he, et al. An algorithm for target parameter estimation based on fractional Fourier and Keystone transforms[J]. Journal of Radars, 2014, 3(5): 511–517. DOI: 10.3724/SP.J.1300.2014.14058
    [12]
    Almeida L B. The fractional Fourier transform and time-frequency representations[J]. IEEE Transactions on Signal Processing, 1994, 42(11): 3084–3091. DOI: 10.1109/78.330368
    [13]
    吴超楠. 基于分数阶傅里叶变换的高精度线性调频信号参数估计方法[D]. [硕士论文], 华南理工大学, 2014: 15–63

    Wu Chaonan. High-precision parameter estimation for LFM signal based on fractional Fourier transform[D]. [Master dissertation], South China University of Technology, 2014: 15–63
    [14]
    Schonhoff T A and Giordano A A. Detection and Estimation Theory and its Applications[M]. Upper Saddle River, NJ: Prentice Hall, 2006
    [15]
    艾越. 分置MIMO雷达多目标信号级定位算法研究[D]. [硕士论文], 电子科技大学, 2015: 19–23

    Ai Yue. Research of MIMO radar with widely separated antennas signal level multi-target localization[D]. [Master dissertation], University of Electronic Science and Technology of China, 2015: 19–23
    [16]
    Schultz R R and Stevenson R L. A Bayesian approach to image expansion for improved definition[J]. IEEE Transactions on Image Processing, 1994, 3(3): 233–242. DOI: 10.1109/83.287017
    [17]
    Sneath P H A and Sokal R R. Numerical taxonomy: The principles and practice of numerical classification[J]. Taxon, 1963, 12(5): 190–199.
    • Relative Articles

  • Relative Articles

    [1]YIN Junjun, LUO Jiahao, LI Xiang, DAI Xiaokang, YANG Jian. Ship Detection Based on Polarimetric SAR Gradient and Complex Wishart Classifier[J]. Journal of Radars, 2024, 13(2): 396-410. doi: 10.12000/JR23198
    [2]DING Jinshan, ZHONG Chao, WEN Liwu, XU Zhong. Joint Detection of Moving Target in Video Synthetic Aperture Radar[J]. Journal of Radars, 2022, 11(3): 313-323. doi: 10.12000/JR22036
    [3]WANG Jingjing, LIU Zheng, XIE Rong, RAN Lei. HRRP Target Recognition Method for Full Polarimetric Radars by Combining Cameron Decomposition and Fusing RKELM[J]. Journal of Radars, 2021, 10(6): 944-955. doi: 10.12000/JR21099
    [4]LIU Tao, YANG Ziyuan, JIANG Yanni, GAO Gui. Review of Ship Detection in Polarimetric Synthetic Aperture Imagery （in English）[J]. Journal of Radars, 2021, 10(1): 1-19. doi: 10.12000/JR20155
    [5]QUAN Sinong, FAN Hui, DAI Dahai, WANG Wei, XIAO Shunping, WANG Xuesong. Recognition of Ships and Chaff Clouds Based on Sophisticated Polarimetric Target Decomposition[J]. Journal of Radars, 2021, 10(1): 61-73. doi: 10.12000/JR20123
    [6]CUI Xingchao, SU Yi, CHEN Siwei. Polarimetric SAR Ship Detection Based on Polarimetric Rotation Domain Features and Superpixel Technique[J]. Journal of Radars, 2021, 10(1): 35-48. doi: 10.12000/JR20147
    [7]PANG Lei, ZHANG Fengli, WANG Guojun, LIU Na, SHAO Yun, ZHANG Jiameng, ZHAO Yuchuan, PANG Lei. Imaging Simulation and Damage Assessment Feature Analysis of Ku Band Polarized SAR of Buildings[J]. Journal of Radars, 2020, 9(3): 578-587. doi: 10.12000/JR20061
    [8]WEI Yangkai, ZENG Tao, CHEN Xinliang, DING Zegang, FAN Yujie, WEN Yuhan. Parametric SAR Imaging for Typical Lines and Surfaces[J]. Journal of Radars, 2020, 9(1): 143-153. doi: 10.12000/JR19077
    [9]Zhou Yejian, Zhang Lei, Wang Hongxian, Xing Mengdao. Performance Analysis on ISAR Imaging of Space Targets[J]. Journal of Radars, 2017, 6(1): 17-24. doi: 10.12000/JR16136
    [10]Yang Wen, Zhong Neng, Yan Tianheng, Yang Xiangli. Classification of Polarimetric SAR Images Based on the Riemannian Manifold[J]. Journal of Radars, 2017, 6(5): 433-441. doi: 10.12000/JR17031
    [11]Tao Chensong, Chen Siwei, Li Yongzhen, Xiao Shunping. Polarimetric SAR Terrain Classification Using Polarimetric Features Derived from Rotation Domain[J]. Journal of Radars, 2017, 6(5): 524-532. doi: 10.12000/JR16131
    [12]Chen Siwei, Li Yongzhen, Wang Xuesong, Xiao Shunping. Polarimetric SAR Target Scattering Interpretation in Rotation Domain: Theory and Application[J]. Journal of Radars, 2017, 6(5): 442-455. doi: 10.12000/JR17033
    [13]Zhang Jingjing, Hong Wen, Yin Qiang. Robust Distributed-target-based Calibration Method for Polarimetric SAR Using Spherically Truncated Covariance Matrix[J]. Journal of Radars, 2016, 5(6): 701-710. doi: 10.12000/JR16138
    [14]Ji Kefeng, Wang Haibo, Leng Xiangguang, Xing Xiangwei, Kang Lihong. Spaceborne Compact Polarimetric Synthetic Aperture Radar for Ship Detection[J]. Journal of Radars, 2016, 5(6): 607-619. doi: 10.12000/JR16083
    [15]Yang Ruliang, Dai Bowei, Li Haiying. Polarization Hierarchy and System Operating Architecture for Polarimetric Synthetic Aperture Radar[J]. Journal of Radars, 2016, 5(2): 132-142. doi: 10.12000/JR16013
    [16]Wu Jiani, Chen Yongguang, Dai Dahai, Pang Bo, Wang Xuesong. Scattering Mechanism Identification Based on Polarimetric HRRP of Manmade Target[J]. Journal of Radars, 2016, 5(2): 174-181. doi: 10.12000/JR16026
    [17]Zhou Wei, Sun Yan-li, Xu Cheng-bin, Guan Jian. A Method for Discrimination of Ship Target and Azimuth Ambiguity in Multi-polarimetric SAR Imagery[J]. Journal of Radars, 2015, 4(1): 84-92. doi: 10.12000/JR14147
    [18]Xu Cheng-bin, Zhou Wei, Cong Yu, Guan Jian. Ship Analysis and Detection in High-resolution Pol-SAR Imagery Based on Peak Zone[J]. Journal of Radars, 2015, 4(3): 367-373. doi: 10.12000/JR14093
    [19]Yan Jian, Li Yang, Yin Qiang, Hong Wen. Freeman-Durden Decomposition with Oriented Dihedral Scattering[J]. Journal of Radars, 2014, 3(5): 574-582. doi: 10.3724/SP.J.1300.2014.14057
    [20]Wu Yi-rong. Concept on Multidimensional Space Joint-observation SAR[J]. Journal of Radars, 2013, 2(2): 135-142. doi: 10.3724/SP.J.1300.2013.13047
    • Supplements(0)
    • Cited By

  • Cited by

    Periodical cited type(0)

    Other cited types(1)

  • Created with Highcharts 5.0.7Amount of accessChart context menuAbstract Views, HTML Views, PDF Downloads StatisticsAbstract ViewsHTML ViewsPDF Downloads2024-052024-062024-072024-082024-092024-102024-112024-122025-012025-022025-032025-040255075100125150
    Created with Highcharts 5.0.7Chart context menuAccess Class DistributionFULLTEXT: 32.8 %FULLTEXT: 32.8 %META: 47.4 %META: 47.4 %PDF: 19.8 %PDF: 19.8 %FULLTEXTMETAPDF
    Created with Highcharts 5.0.7Chart context menuAccess Area Distribution其他: 8.5 %其他: 8.5 %其他: 0.9 %其他: 0.9 %China: 1.2 %China: 1.2 %San Mateo: 0.1 %San Mateo: 0.1 %上海: 4.8 %上海: 4.8 %东京: 0.4 %东京: 0.4 %东营: 0.1 %东营: 0.1 %临汾: 0.2 %临汾: 0.2 %临沂: 0.2 %临沂: 0.2 %京都: 0.1 %京都: 0.1 %伦敦: 0.2 %伦敦: 0.2 %佛山: 0.2 %佛山: 0.2 %保定: 0.1 %保定: 0.1 %信阳: 0.1 %信阳: 0.1 %兰州: 0.3 %兰州: 0.3 %内江: 0.2 %内江: 0.2 %加利福尼亚: 0.1 %加利福尼亚: 0.1 %包头: 0.8 %包头: 0.8 %北京: 12.2 %北京: 12.2 %十堰: 0.4 %十堰: 0.4 %南京: 3.3 %南京: 3.3 %南宁: 0.1 %南宁: 0.1 %南昌: 0.5 %南昌: 0.5 %南通: 0.1 %南通: 0.1 %台州: 0.3 %台州: 0.3 %合肥: 0.8 %合肥: 0.8 %呼和浩特: 0.2 %呼和浩特: 0.2 %哈尔滨: 0.5 %哈尔滨: 0.5 %哥伦布: 0.1 %哥伦布: 0.1 %嘉兴: 0.2 %嘉兴: 0.2 %天津: 0.5 %天津: 0.5 %太原: 0.3 %太原: 0.3 %威海: 0.5 %威海: 0.5 %安康: 0.2 %安康: 0.2 %定西: 0.1 %定西: 0.1 %宣城: 0.3 %宣城: 0.3 %崇左: 0.1 %崇左: 0.1 %巴中: 0.1 %巴中: 0.1 %常州: 0.5 %常州: 0.5 %常德: 0.2 %常德: 0.2 %广元: 0.1 %广元: 0.1 %广州: 0.6 %广州: 0.6 %庆阳: 0.1 %庆阳: 0.1 %廊坊: 0.3 %廊坊: 0.3 %延安: 0.1 %延安: 0.1 %开封: 0.9 %开封: 0.9 %张家口: 3.3 %张家口: 3.3 %张家界: 0.1 %张家界: 0.1 %徐州: 0.2 %徐州: 0.2 %怀化: 0.1 %怀化: 0.1 %成都: 3.5 %成都: 3.5 %扬州: 0.6 %扬州: 0.6 %斯特灵: 0.1 %斯特灵: 0.1 %新乡: 0.1 %新乡: 0.1 %新德里: 0.2 %新德里: 0.2 %无锡: 0.4 %无锡: 0.4 %昆明: 1.7 %昆明: 1.7 %杭州: 1.5 %杭州: 1.5 %松原: 0.1 %松原: 0.1 %枣庄: 0.1 %枣庄: 0.1 %桂林: 0.6 %桂林: 0.6 %榆林: 0.3 %榆林: 0.3 %武汉: 0.9 %武汉: 0.9 %汉中: 0.1 %汉中: 0.1 %汕尾: 0.1 %汕尾: 0.1 %池州: 0.1 %池州: 0.1 %洛阳: 0.9 %洛阳: 0.9 %济南: 0.4 %济南: 0.4 %淮南: 0.2 %淮南: 0.2 %深圳: 0.4 %深圳: 0.4 %温州: 0.5 %温州: 0.5 %湖州: 0.4 %湖州: 0.4 %湘西: 0.1 %湘西: 0.1 %滨州: 0.2 %滨州: 0.2 %漯河: 2.5 %漯河: 2.5 %珠海: 0.3 %珠海: 0.3 %石家庄: 0.4 %石家庄: 0.4 %秦皇岛: 0.2 %秦皇岛: 0.2 %纽约: 1.0 %纽约: 1.0 %绵阳: 0.2 %绵阳: 0.2 %芒廷维尤: 7.0 %芒廷维尤: 7.0 %芝加哥: 1.6 %芝加哥: 1.6 %苏州: 0.3 %苏州: 0.3 %荆门: 0.1 %荆门: 0.1 %葵涌: 0.1 %葵涌: 0.1 %蚌埠: 0.1 %蚌埠: 0.1 %衡水: 0.2 %衡水: 0.2 %衡阳: 0.4 %衡阳: 0.4 %西宁: 6.1 %西宁: 6.1 %西安: 8.0 %西安: 8.0 %西雅图: 0.1 %西雅图: 0.1 %诺沃克: 8.4 %诺沃克: 8.4 %贵阳: 0.5 %贵阳: 0.5 %赤峰: 0.2 %赤峰: 0.2 %运城: 0.3 %运城: 0.3 %遵义: 0.1 %遵义: 0.1 %邯郸: 0.5 %邯郸: 0.5 %郑州: 0.7 %郑州: 0.7 %重庆: 0.3 %重庆: 0.3 %银川: 0.1 %银川: 0.1 %长春: 0.2 %长春: 0.2 %长沙: 0.9 %长沙: 0.9 %长治: 0.1 %长治: 0.1 %防城港: 0.1 %防城港: 0.1 %陇南: 0.1 %陇南: 0.1 %香港: 0.2 %香港: 0.2 %黔东南: 0.1 %黔东南: 0.1 %齐齐哈尔: 0.2 %齐齐哈尔: 0.2 %其他其他ChinaSan Mateo上海东京东营临汾临沂京都伦敦佛山保定信阳兰州内江加利福尼亚包头北京十堰南京南宁南昌南通台州合肥呼和浩特哈尔滨哥伦布嘉兴天津太原威海安康定西宣城崇左巴中常州常德广元广州庆阳廊坊延安开封张家口张家界徐州怀化成都扬州斯特灵新乡新德里无锡昆明杭州松原枣庄桂林榆林武汉汉中汕尾池州洛阳济南淮南深圳温州湖州湘西滨州漯河珠海石家庄秦皇岛纽约绵阳芒廷维尤芝加哥苏州荆门葵涌蚌埠衡水衡阳西宁西安西雅图诺沃克贵阳赤峰运城遵义邯郸郑州重庆银川长春长沙长治防城港陇南香港黔东南齐齐哈尔

Catalog

    通讯作者: 陈斌, bchen63@163.com
    • 1. 

      沈阳化工大学材料科学与工程学院 沈阳 110142

    1. 本站搜索
    2. 百度学术搜索
    3. 万方数据库搜索
    4. CNKI搜索
    Get Citation
    PDF
    XML
    Article views(3153) PDF downloads(341) Cited by(1)
    Proportional views
    Related

    京ICP备20021838号-8   Supported by: Beijing Renhe Information Technology Co. Ltd     百度统计

    /

    DownLoad:  Full-Size Img  PowerPoint
    Return