单脉冲雷达多点源参数估计与抗干扰技术进展

马佳智; 施龙飞; 徐振海; 王雪松

doi:10.12000/JR18093

单脉冲雷达多点源参数估计与抗干扰技术进展

DOI: 10.12000/JR18093

国防科技大学电子科学学院 CEMEE 国家重点实验室长沙 410073

基金项目: 国家自然科学基金(60490692, 61871385)

详细信息

作者简介:
马佳智(1987–)，男，山西大同人，博士，讲师。2017年在国防科技大学电子科学学院获得博士学位，现担任国防科技大学电子科学学院讲师，主要研究方向为极化信息处理、精确制导技术。E-mail: jzmanudt@163.com

施龙飞(1978–)，男，安徽芜湖人，博士，副研究员。2007年在国防科技大学电子科学学院获得博士学位，现担任国防科技大学电子科学学院副研究员，主要研究方向为雷达信号处理、雷达对抗技术。E-mail: longfei_shi@sina.com

徐振海(1977–)，男，河南卫辉人，博士，教授。2004年在国防科学技术大学电子科学与工程学院获得博士学位，现担任国防科技大学电子科学学院教授，主要研究方向为阵列信号处理、子阵划分技术、目标分辨技术。E-mail: xzh930@aliyun.com

王雪松(1972–)，男，内蒙古包头人，博士，教授。1999年在国防科学技术大学电子科学与工程学院获得博士学位，现担任国防科技大学电子科学学院教授，院长，主要研究方向为极化信息处理、雷达目标识别、新体制雷达技术。E-mail: wangxuesong@nudt.edu.cn

通讯作者:
马佳智 jzmanudt@163.com

中图分类号: TN95
计量
- 文章访问数: 4752
- HTML全文浏览量: 1873
- PDF下载量: 386
- 被引次数: 0
出版历程
- 收稿日期: 2018-11-07
- 修回日期: 2019-02-20
- 网络出版日期: 2019-02-28

Overview of Multi-source Parameter Estimation and Jamming Mitigation for Monopulse Radars

State Key Laboratory of Complex Electromagnetic Environmental Effects on Electronics and Information System, National University of Defense Technology, Changsha 410073, China

Funds: The National Nature Science Foundation of China (60490692, 61871385)

More Information

Corresponding author: MA Jiazhi, jzmanudt@163.com

摘要

摘要: 单脉冲测角是当前主动雷达广泛采用的主流测角技术，大量应用于警戒跟踪、精确制导等雷达/雷达导引头探测领域。该文简要回顾了主瓣多点源条件下的单脉冲信号处理理论与技术发展历程，围绕单脉冲雷达多点源参数估计与抗干扰技术最新成果进行综述，最后对单脉冲雷达多点源参数估计与抗干扰技术的未来发展做了展望。
- 单脉冲雷达 /
- 多点源干扰抑制 /
- 多源分辨 /
- 扩展目标估计 /
- 抗干扰
Abstract: Monopulse is a mainstream technique used to acquire the angle information about active radar systems that are widely used in air defense warning, target tracking, and precision guidance. This study briefly reviews the development history of the monopulse theory and technology for the main-lobe multi-source condition. The importance of several key technologies within multi-source parameters estimation and multi-source jamming mitigation is also summarized. Finally, the future development of monopulse technology to resolve the problem of multi-source jamming is considered.
- Monopulse radar /
- Multi-source jamming /
- Unresolved targets resolution /
- Extended targets estimation /
- Anti-interference

HTML全文

图 1 文献[29]中对两类混合散射中心目标的角度中心与角度扩展的估计结果

Figure 1. The estimation results of the angle and the extension of two extended targets in Ref. [29]

下载: 全尺寸图片幻灯片

图 2 文献[38]中对5个不可分辨目标的角度估计结果

Figure 2. Angle estimation results of five unresolved targets in Ref. [38]

下载: 全尺寸图片幻灯片

图 3 几种新型的多频双极化单脉冲天线

Figure 3. Several types of multi-band dual-polarization monopulse antenna

下载: 全尺寸图片幻灯片

图 4 文献[100]中校正后雷达单脉冲比

Figure 4. The corrected monopulse ratios after adaptive digital beam forming in Ref. [100]

下载: 全尺寸图片幻灯片

参考文献(124)

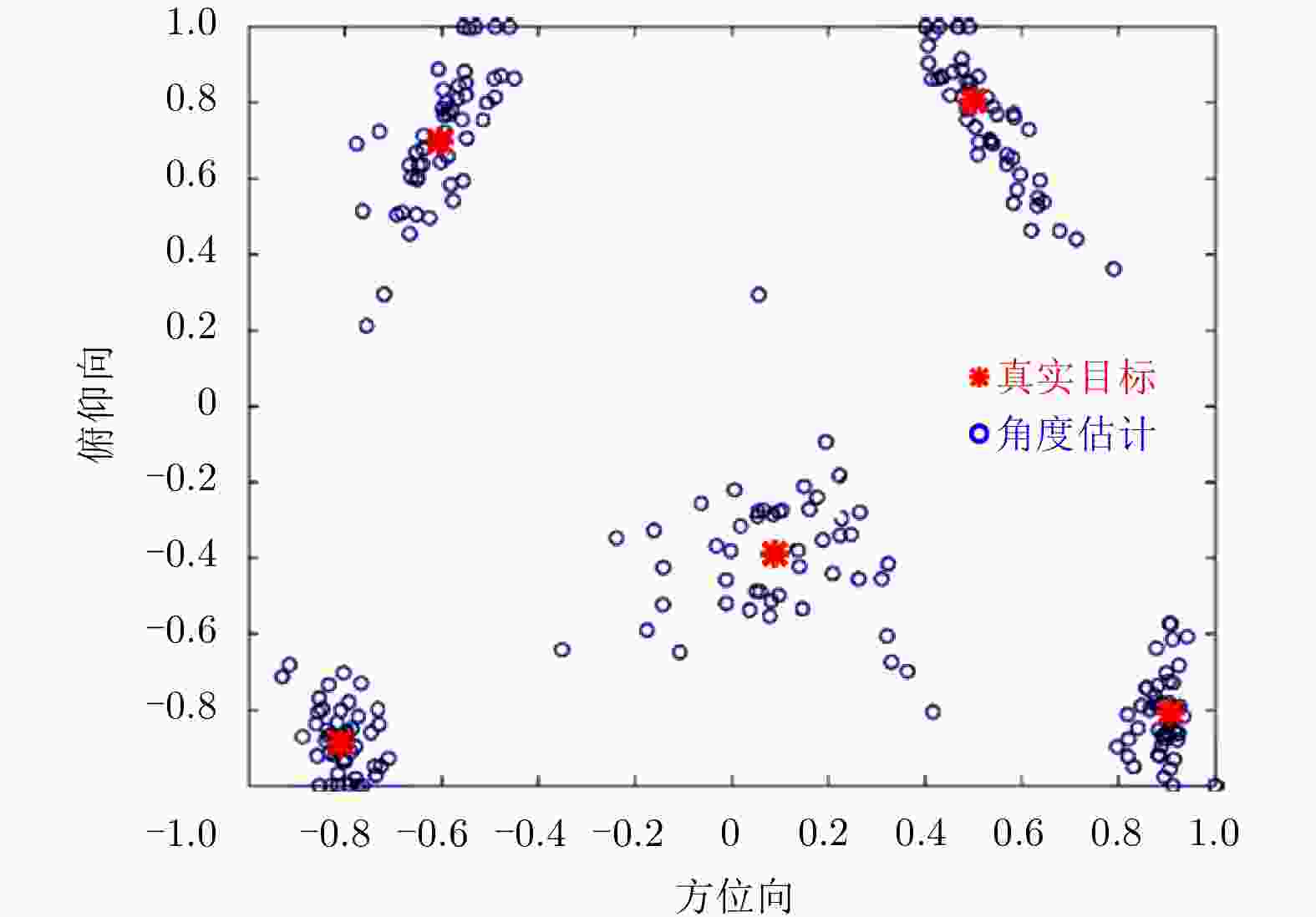
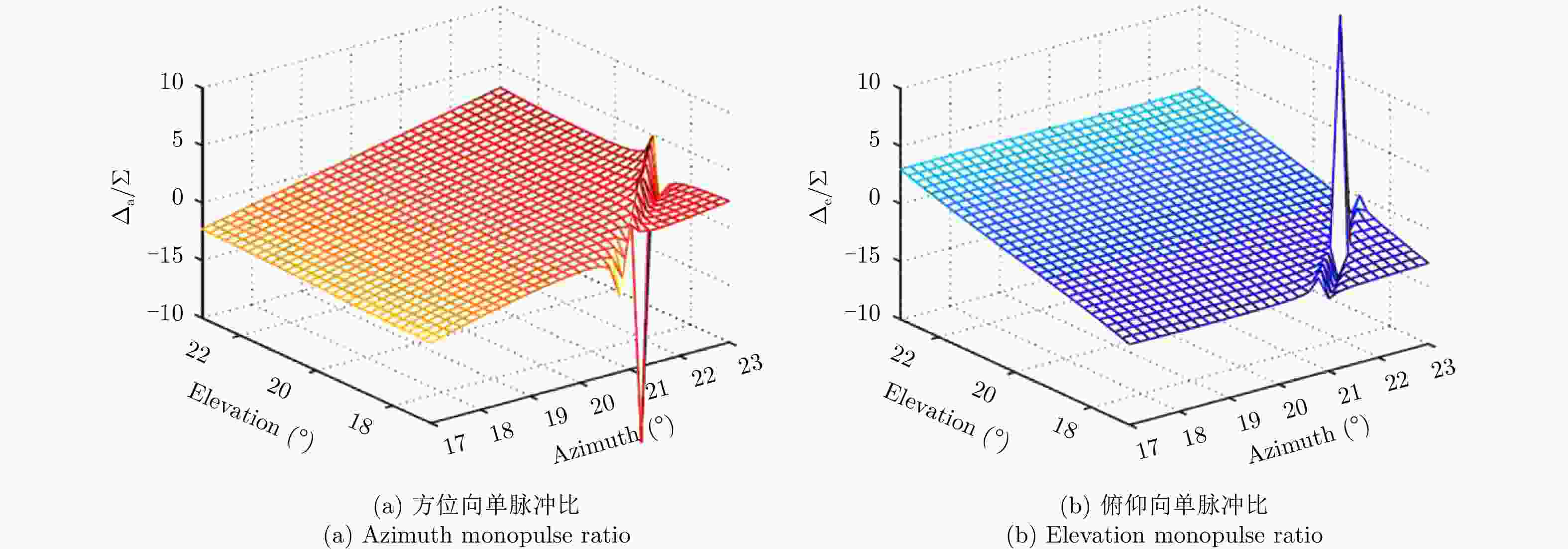
[1]	SHERMAN S M and BARTON D K. Monopulse Principles and Techniques[M]. Norwood, MA: Artech House, 2011: 1–18.
[2]	HOWARD D D. Radar target glint in tracking and guidance system based on echo signal phase distortion[J]. Proceedings of NEC, 1959, 15: 840–849.
[3]	LINDSAY J E. Angular glint and the moving, rotating, complex radar target[J]. IEEE Transactions on Aerospace and Electronic Systems, 1968, AES-4(2): 164–173. doi: 10.1109/TAES.1968.5408954
[4]	DUNN H H and HOWARD D D. Radar target amplitude, angle, and Doppler scintillation from analysis of the echo signal propagating in space[J]. IEEE Transactions on Microwave Theory and Techniques, 1968, 16(9): 715–728. doi: 10.1109/TMTT.1968.1126776
[5]	KAJENSKI P J. Comparison of two theories of angle glint: Polarization considerations[J]. IEEE Transactions on Aerospace and Electronic Systems, 2006, 42(1): 206–210. doi: 10.1109/TAES.2006.1603415
[6]	YIN Hongcheng and HUANG Peikang. Unification and comparison between two concepts of radar target angular glint[J]. IEEE Transactions on Aerospace and Electronic Systems, 1995, 31(2): 778–783. doi: 10.1109/7.381924
[7]	YIN Hongcheng and HUANG Peikang. Further comparison between two concepts of radar target angular glint[J]. IEEE Transactions on Aerospace and Electronic Systems, 2008, 44(1): 372–380. doi: 10.1109/TAES.2008.4517012
[8]	SHERMAN S M. Complex indicated angles applied to unresolved radar targets and multipath[J]. IEEE Transactions on Aerospace and Electronic Systems, 1971, AES-7(1): 160–170. doi: 10.1109/TAES.1971.310264
[9]	DU PLESSIS W P, ODENDAAL J W, and JOUBERT J. Extended analysis of retrodirective cross-eye jamming[J]. IEEE Transactions on Antennas and Propagation, 2009, 57(9): 2803–2806. doi: 10.1109/TAP.2009.2027353
[10]	HARWOOD N M, DAWBER W N, KING D J, et al. Multiple-element crosseye[J]. IET Radar, Sonar & Navigation, 2007, 1(1): 67–73. doi: 10.1049/iet-rsn:20060042
[11]	殷红成, 王超, 黄培康. 雷达目标角闪烁三种表示的内在联系(英文)[J]. 雷达学报, 2014, 3(2): 119–128. doi: 10.3724/SP.J.1300.2014.14025 YIN Hongcheng, WANG Chao, and HUANG Peikang. Inherent relations among the three representations of radar target angular glint[J]. Journal of Radars, 2014, 3(2): 119–128. doi: 10.3724/SP.J.1300.2014.14025
[12]	MONAKOV A. Physical and statistical properties of the complex monopulse ratio[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(2): 960–968. doi: 10.1109/TAES.2013.6494392
[13]	SHARENSON S. Angle estimation accuracy with a monopulse radar in the search mode[J]. IRE Transactions on Aerospace and Navigational Electronics, 1962, ANE-9(3): 175–179. doi: 10.1109/TANE3.1962.4201876
[14]	KANTER I. Multiple Gaussian targets: The track-on-jam problem[J]. IEEE Transactions on Aerospace and Electronic Systems, 1977, AES-13(6): 620–623. doi: 10.1109/TAES.1977.308502
[15]	KANTER I. Corrections to " multiple Gaussian targets: The track-on-jam problem”[J]. IEEE Transactions on Aerospace and Electronic Systems, 1978, AES-14(3): 544. doi: 10.1109/TAES.1978.308619
[16]	KANTER I. Varieties of average monopulse responses to multiple targets[J]. IEEE Transactions on Aerospace and Electronic Systems, 1981, AES-17(1): 25–28. doi: 10.1109/TAES.1981.309032
[17]	KANTER I. The probability density function of the monopulse ratio for n looks at a combination of constant and Rayleigh targets[J]. IEEE Transactions on Information Theory, 1977, 23(5): 643–648. doi: 10.1109/TIT.1977.1055778
[18]	ASSEO S J. Effect of monopulse signal thresholding on tracking multiple targets[J]. IEEE Transactions on Aerospace and Electronic Systems, 1974, AES-10(4): 504–509. doi: 10.1109/TAES.1974.307805
[19]	ASSEO S J. Corrections to " detection of target multiplicity using monopulse quadrature angle”[J]. IEEE Transactions on Aerospace and Electronic Systems, 1981, AES-17(6): 466–468. doi: 10.1109/TAES.1981.309077
[20]	TULLSSON B E. Monopulse tracking of Rayleigh targets: A simple approach[J]. IEEE Transactions on Aerospace and Electronic Systems, 1991, 27(3): 520–531. doi: 10.1109/7.81434
[21]	GROVES G W, BLAIR W D, and CHOW W C. Probability distribution of complex monopulse ratio with arbitrary correlation between channels[J]. IEEE Transactions on Aerospace and Electronic Systems, 1997, 33(4): 1345–1350. doi: 10.1109/7.625136
[22]	SEIFER A D. Monopulse-radar angle tracking in noise or noise jamming[J]. IEEE Transactions on Aerospace and Electronic Systems, 1992, 28(3): 622–638. doi: 10.1109/7.256285
[23]	BLAIR W D and BRANDT-PEARCE M. Unresolved Rayleigh target detection using monopulse measurements[J]. IEEE Transactions on Aerospace and Electronic Systems, 1998, 34(2): 543–552. doi: 10.1109/7.670335
[24]	SINHA A, KIRUBARAJAN T, and BAR-SHALOM Y. Maximum likelihood angle extractor for two closely spaced targets[J]. IEEE Transactions on Aerospace and Electronic Systems, 2002, 38(1): 183–203. doi: 10.1109/7.993239
[25]	NICKEL U. Performance of corrected adaptive monopulse estimation[J]. IEE Proceedings - Radar, Sonar and Navigation, 1999, 146(1): 17–24. doi: 10.1049/ip-rsn:19990257
[26]	NICKEL U. Overview of generalized monopulse estimation[J]. IEEE Aerospace and Electronic Systems Magazine, 2006, 21(6): 27–56. doi: 10.1109/MAES.2006.1662039
[27]	NICKEL U R O, CHAUMETTE E, and LARZABAL P. Statistical performance prediction of generalized monopulse estimation[J]. IEEE Transactions on Aerospace and Electronic Systems, 2011, 47(1): 381–404. doi: 10.1109/TAES.2011.5705682
[28]	CHAUMETTE E, NICKEL U, and LARZABAL P. Detection and parameter estimation of extended targets using the generalized monopulse estimator[J]. IEEE Transactions on Aerospace and Electronic Systems, 2012, 48(4): 3389–3417. doi: 10.1109/TAES.2012.6324719
[29]	NICKEL U, CHAUMETTE E, and LARZABAL P. Estimation of extended targets using the generalized monopulse estimator: Extension to a mixed target model[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(3): 2084–2096. doi: 10.1109/TAES.2013.6558043
[30]	DU PLESSIS W P. Platform skin return and retrodirective cross-eye jamming[J]. IEEE Transactions on Aerospace and Electronic Systems, 2012, 48(1): 490–501. doi: 10.1109/TAES.2012.6129650
[31]	DU PLESSIS W P. Cross-eye gain in multiloop retrodirective cross-eye jamming[J]. IEEE Transactions on Aerospace and Electronic Systems, 2016, 52(2): 875–882. doi: 10.1109/TAES.2016.140112
[32]	LIU Tianpeng, LIAO Dongping, and WEI Xizhang. Performance analysis of multiple-element retrodirective cross-eye jamming based on linear array[J]. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(3): 1867–1876. doi: 10.1109/TAES.2015.140035
[33]	LIU Tianpeng, LIU Zhen, LIAO Dongping, et al. Platform skin return and multiple-element linear retrodirective cross-eye jamming[J]. IEEE Transactions on Aerospace and Electronic Systems, 2016, 52(2): 821–835. doi: 10.1109/TAES.2016.140949
[34]	MA Jiazhi, SHI Longfei, CUI Gang, et al. Further analysis of retrodirective cross-eye jamming: Polarization considerations[C]. Proceedings of the 12th European Conference on Antennas and Propagation, London, UK, 2018: 1–5. doi: 10.1049/cp.2018.1252.
[35]	GLASS J D and BLAIR W D. Detection of Rayleigh targets using adjacent matched filter samples[J]. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(3): 1927–1941. doi: 10.1109/TAES.2015.140683
[36]	GLASS J D, BLAIR W D, and LANTERMAN A D. Joint-bin monopulse processing of Rayleigh targets[J]. IEEE Transactions on Signal Processing, 2015, 63(24): 6673–6683. doi: 10.1109/TSP.2015.2478749
[37]	BLAIR W D and BRANDT-PEARCE M. Monopulse DOA estimation of two unresolved Rayleigh targets[J]. IEEE Transactions on Aerospace and Electronic Systems, 2001, 37(2): 452–469. doi: 10.1109/7.937461
[38]	ZHANG Xin, WILLETT P K, and BAR-SHALOM Y. Monopulse radar detection and localization of multiple unresolved targets via joint bin processing[J]. IEEE Transactions on Signal Processing, 2005, 53(4): 1225–1236. doi: 10.1109/TSP.2005.843732
[39]	ZHANG Xin, WILLETT P, and BAR-SHALOM Y. Detection and localization of multiple unresolved extended targets via monopulse radar signal processing[J]. IEEE Transactions on Aerospace and Electronic Systems, 2009, 45(2): 455–472. doi: 10.1109/TAES.2009.5089534
[40]	WILLETT P, BLAIR W D, and ZHANG Xin. The multitarget monopulse CRLB for matched filter samples[J]. IEEE Transactions on Signal Processing, 2007, 55(8): 4183–4197. doi: 10.1109/TSP.2007.894405
[41]	ISAAC A, WILLETT P, and BAR-SHALOM Y. MCMC methods for tracking two closely spaced targets using monopulse radar channel signals[J]. IET Radar, Sonar & Navigation, 2007, 1(3): 221–229. doi: 10.1049/iet-rsn:20060117
[42]	NANDAKUMARAN N, SINHA A, and KIRUBARAJAN T. Joint detection and tracking of unresolved targets with monopulse radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2008, 44(4): 1326–1341. doi: 10.1109/TAES.2008.4667712
[43]	LEE S P, CHO B L, LEE S M, et al. Unambiguous angle estimation of unresolved targets in monopulse radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(2): 1170–1177. doi: 10.1109/TAES.2014.140178
[44]	ZHENG Yibin, TSENG S M, and YU K B. Closed-form four-channel monopulse two-target resolution[J]. IEEE Transactions on Aerospace and Electronic Systems, 2003, 39(3): 1083–1089. doi: 10.1109/TAES.2003.1238760
[45]	CROUSE D F, NICKEL U, and WILLETT P. Comments on " closed-form four-channel monopulse two-target resolution”[J]. IEEE Transactions on Aerospace and Electronic Systems, 2012, 48(1): 913–916. doi: 10.1109/TAES.2012.6129678
[46]	JARDAK S, AHMED S, and ALOUINI M S. Generalised two target localisation using passive monopulse radar[J]. IET Radar, Sonar & Navigation, 2017, 11(6): 932–936. doi: 10.1049/iet-rsn.2016.0495
[47]	MA Jiazhi, SHI Longfei, and LIU Jian. Improved two-targets resolution using dual-polarization radar with interlaced subarray partition[C]. Proceedings of the 13th IEEE International Conference on Electronic Measurement & Instruments, Yangzhou, China, 2017: 397–400. doi: 10.1109/ICEMI.2017.8265831.
[48]	戴幻尧, 王建路, 韩慧, 等. 基于对角差信号的单脉冲雷达二维角估计新方法[J]. 现代雷达, 2017, 39(1): 22–25, 31. doi: 10.16592/j.cnki.1004-7859.2017.01.005 DAI Huanyao, WANG Jianlu, HAN Hui, et al. Two dimension DOA estimation method of monopulse radar based on diagonal difference channel signal[J]. Modern Radar, 2017, 39(1): 22–25, 31. doi: 10.16592/j.cnki.1004-7859.2017.01.005
[49]	徐振海, 肖顺平, 熊子源. 阵列雷达低角跟踪技术[M]. 北京: 科学出版社, 2014. XU Zhenhai, XIAO Shunping, and XIONG Ziyuan. Low Angle Tracking Techniques for Array Radars[M]. Beijing: Science Press, 2014.
[50]	WHITE W D. Low-angle radar tracking in the presence of multipath[J]. IEEE Transactions on Aerospace and Electronic Systems, 1974, AES-10(6): 835–852. doi: 10.1109/TAES.1974.307892
[51]	WHITE W D. Double null technique for low angle tracking[J]. Microwave Journal, 1976, 19(12): 35–38, 60.
[52]	徐振海, 熊子源, 宋聃, 等. 阵列雷达双零点单脉冲低角跟踪算法[J]. 国防科技大学学报, 2015, 37(1): 130–135. doi: 10.11887/j.cn.201501022 XU Zhenhai, XIONG Ziyuan, SONG Dan, et al. Double-null monopulse low-angle tracking algorithm with array radars[J]. Journal of National University of Defense Technology, 2015, 37(1): 130–135. doi: 10.11887/j.cn.201501022
[53]	SEBT M A, SHEIKHI A, and NAYEBI M M. Robust low-angle estimation by an array radar[J]. IET Radar, Sonar & Navigation, 2010, 4(6): 780–790. doi: 10.1049/iet-rsn.2009.0067
[54]	XU Zhenhai, XIONG Ziyuan, WU Jiani, et al. Symmetrical difference pattern monopulse for low-angle tracking with array radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2016, 52(6): 2676–2684. doi: 10.1109/TAES.2016.140436
[55]	赵英俊, 李荣锋, 王永良, 等. 基于主瓣多径干扰抑制的米波雷达测角方法[J]. 华中科技大学学报(自然科学版), 2013, 41(4): 51–55. doi: 10.13245/j.husst.2013.04.014 ZHAO Yingjun, LI Rongfeng, WANG Yongliang, et al. Angles measurement of meter-wave radars by mainlobe multipath jamming suppression[J]. Journal of Huazhong University of Science and Technology (Natural Science Edition), 2013, 41(4): 51–55. doi: 10.13245/j.husst.2013.04.014
[56]	PARK D, YANG E, AHN S, et al. Adaptive beamforming for low-angle target tracking under multipath interference[J]. IEEE Transactions on Aerospace and Electronic Systems, 2014, 50(4): 2564–2577. doi: 10.1109/TAES.2014.130185
[57]	朱伟, 陈伯孝. 强相干干扰下微弱信号波达方向估计[J]. 电波科学学报, 2013, 28(2): 212–219. doi: 10.13443/j.cjors.2013.02.019 ZHU Wei and CHEN Baixiao. Weak signal DOA estimation under coherent intensive interferences[J]. Chinese Journal of Radio Science, 2013, 28(2): 212–219. doi: 10.13443/j.cjors.2013.02.019
[58]	DARVISHI H and SEBT M A. Adaptive hybrid method for low-angle target tracking in multipath[J]. IET Radar, Sonar & Navigation, 2018, 12(9): 931–937. doi: 10.1049/iet-rsn.2018.5114
[59]	SHANG She, ZHANG Shouhong, and ZHANG Xiushe. Investigation on low-angle tracking technique for HRR radar[C]. Proceedings of 2001 CIE International Conference on Radar Proceedings, Beijing, China, 2001: 839–842. doi: 10.1109/ICR.2001.984842.
[60]	HU Jingyuan, ZHAO Guoqiang, and SUN Houjun. A dual polarization multi-layer array antenna used by digital system[C]. Proceedings of 2015 Asia-Pacific Microwave Conference, Nanjing, China, 2015, 3: 1–3. doi: 10.1109/APMC.2015.7413305.
[61]	LI Teng, MENG Hongfu, and DOU Wenbin. Design and implementation of dual-frequency dual-polarization slotted waveguide antenna array for Ka-band application[J]. IEEE Antennas and Wireless Propagation Letters, 2014, 13: 1317–1320. doi: 10.1109/LAWP.2014.2337355
[62]	HASANI H, PEIXEIRO C, SKRIVERVIK A K, et al. Single-layer quad-band printed reflectarray antenna with dual linear polarization[J]. IEEE Transactions on Antennas and Propagation, 2015, 63(12): 5522–5528. doi: 10.1109/TAP.2015.2481918
[63]	NERI F. Introduction to Electronic Defense Systems[M]. Boston, MA: Artech House, 2001.
[64]	MANGULIS V. Frequency diversity in low-angle radar tracking[J]. IEEE Transactions on Aerospace and Electronic Systems, 1981, AES-17(1): 149–153. doi: 10.1109/TAES.1981.309050
[65]	BOSSE E, TURNER R M, and RISEBOROUGH E S. Model-based multifrequency array signal processing for low-angle tracking[J]. IEEE Transactions on Aerospace and Electronic Systems, 1995, 31(1): 194–210. doi: 10.1109/7.366303
[66]	ZHOU Jie, WANG Jianming, and XING Wenge. Study on low-angle tracking technique for shipboard phased array radar[C]. Proceedings of 2006 CIE International Conference on Radar, Shanghai, China, 2006: 1–4. doi: 10.1109/ICR.2006.343591.
[67]	ZHANG Y D, LI Xin, and AMIN G M. Target localization in multipath environment through the exploitation of multi-frequency array[C]. Proceedings of 2010 International Waveform Diversity and Design Conference, Niagara Falls, Canada, 2010: 206–210. doi: 10.1109/WDD.2010.5592512.
[68]	ZHU Yutang, ZHAO Yongbo, and SHUI Penglang. Low-angle target tracking using frequency-agile refined maximum likelihood algorithm[J]. IET Radar, Sonar & Navigation, 2017, 11(3): 491–497. doi: 10.1049/iet-rsn.2016.0301
[69]	BLAIR W D, GROVES G W, BAR-SHALOM Y, et al. Frequency agility and fusion for tracking targets in the presence of multipath propagation[C]. Proceedings of 1994 IEEE National Radar Conference, Atlanta, GA, USA, 1994: 166–170. doi: 10.1109/NRC.1994.328118.
[70]	施龙飞. 雷达极化抗干扰技术研究[D]. [博士论文], 国防科学技术大学, 2007. SHI Longfei. Study on the suppression of interference with radar polarization information[D]. [Ph.D. dissertation], National University of Defense Technology, 2007.
[71]	KWAK H, YANG E, and CHUN H. Vector sensor arrays in DOA estimation for the low angle tracking[C]. Proceedings of 2007 International Waveform Diversity and Design Conference, Pisa, Italy, 2007: 183–187. doi: 10.1109/WDDC.2007.4339406.
[72]	施龙飞, 王雪松, 肖顺平. 低空镜像角闪烁的极化抑制[J]. 电波科学学报, 2008, 23(6): 1038–1044. doi: 10.3969/j.issn.1005-0388.2008.06.005 SHI Longfei, WANG Xuesong, and XIAO Shunping. Depressing of angle glint of low-altitude enantiomorphous target by polarization diversity[J]. Chinese Journal of Radio Science, 2008, 23(6): 1038–1044. doi: 10.3969/j.issn.1005-0388.2008.06.005
[73]	宋志勇, 肖怀铁, 祝依龙, 等. 基于扩展单脉冲比的拖曳式诱饵存在性检测[J]. 航空学报, 2011, 32(9): 1656–1668. SONG Zhiyong, XIAO Huaitie, ZHU Yilong, et al. Detection of presence of towed radar active decoy based on extended monopulse ratio[J]. Acta Aeronautica et Astronautica Sinica, 2011, 32(9): 1656–1668.
[74]	王建路, 戴幻尧, 韩慧, 等. 雷达导引头对拖曳式诱饵干扰的检测和识别新方法[J]. 航天电子对抗, 2016, 32(6): 5–8, 28. doi: 10.16328/j.htdz8511.2016.06.002 WANG Jianlu, DAI Huanyao, HAN Hui, et al. A new method for detection and recognition of towed decoy jamming by radar seeker[J]. Aerospace Electronic Warfare, 2016, 32(6): 5–8, 28. doi: 10.16328/j.htdz8511.2016.06.002
[75]	李永祯, 胡万秋, 孙豆, 等. 一种基于极化信息的机载拖曳式诱饵存在性检测与抑制方法研究[J]. 雷达学报, 2016, 5(6): 666–672. doi: 10.12000/JR16115 LI Yongzhen, HU Wanqiu, SUN Dou, et al. Scheme for polarization detection and suppression of TRAD[J]. Journal of Radars, 2016, 5(6): 666–672. doi: 10.12000/JR16115
[76]	李永祯, 胡万秋, 程旭, 等. 相干两点源角欺骗干扰的极化鉴别方法研究[J]. 兵工学报, 2013, 34(9): 1078–1083. doi: 10.3969/j.issn.1000-1093.2013.09.004 LI Yongzhen, HU Wanqiu, CHENG Xu, et al. Research on polarization discrimination algorithm for coherent dual-source angle deception interference[J]. Acta Armamentarii, 2013, 34(9): 1078–1083. doi: 10.3969/j.issn.1000-1093.2013.09.004
[77]	宗志伟, 李永祯, 施龙飞, 等. 全极化雷达相干两点源角度欺骗干扰识别方法[J]. 电波科学学报, 2014, 29(4): 621–626. doi: 10.13443/j.cjors.2013071201 ZONG Zhiwei, LI Yongzhen, SHI Longfei, et al. Discrimination method for coherent dual point sources angular deception using fully polarimetric radar[J]. Chinese Journal of Radio Science, 2014, 29(4): 621–626. doi: 10.13443/j.cjors.2013071201
[78]	马佳智. 极化雷达导引头多点源参数估计与抗干扰技术研究[D]. [博士论文], 国防科学技术大学, 2017. MA Jiazhi. Study on multi-sources parameter estimation and jamming mitigation for polarimetric radar seeker[D]. [Ph.D. dissertation], National University of Defense Technology, 2017.
[79]	施龙飞, 毛楚乔, 张建明, 等. 基于极化-空间谱特征的雷达目标检测方法[J]. 雷达科学与技术, 2018, 16(2): 174–180. doi: 10.3969/j.issn.1672-2337.2018.02.010 SHI Longfei, MAO Chuqiao, ZHANG Jianming, et al. A target detection method based on polarization-space joint spectrum characteristic[J]. Radar Science and Technology, 2018, 16(2): 174–180. doi: 10.3969/j.issn.1672-2337.2018.02.010
[80]	胥文泉, 施龙飞, 肖顺平. 基于相位分集的目标极化特征检测方法[J]. 雷达科学与技术, 2018, 16(3): 275–280, 285. doi: 10.3969/j.issn.1672-2337.2018.03.007 XU Wenquan, SHI Longfei, and XIAO Shunping. A target detection method with polarization characteristic based on phase diversity[J]. Radar Science and Technology, 2018, 16(3): 275–280, 285. doi: 10.3969/j.issn.1672-2337.2018.03.007
[81]	GRECO M, GINI F, and FARINA A. Radar detection and classification of jamming signals belonging to a cone class[J]. IEEE Transactions on Signal Processing, 2008, 56(5): 1984–1993. doi: 10.1109/TSP.2007.909326
[82]	卢龙云, 李明, 陈洪猛, 等. 基于奇异谱分析的抗数字射频存储距离波门拖引干扰[J]. 电子与信息学报, 2016, 38(3): 600–606. doi: 10.11999/JEIT150550 LU Longyun, LI Ming, CHEN Hongmeng, et al. Countering DRFM range gate pull-off jamming based on singular spectrum analysis[J]. Journal of Electronics &Information Technology, 2016, 38(3): 600–606. doi: 10.11999/JEIT150550
[83]	ZHANG Jindong, ZHU Daiyin, and ZHANG Gong. New antivelocity deception jamming technique using pulses with adaptive initial phases[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(2): 1290–1300. doi: 10.1109/TAES.2013.6494414
[84]	李永祯. 瞬态极化统计特性及处理的研究[D]. [博士论文], 国防科学技术大学, 2004. LI Yongzhen. Study on statistical characteristics and processing of instantaneous polarization[D]. [Ph.D. dissertation], National University of Defense Technology, 2004.
[85]	王涛. 弹道中段目标极化域特征提取与识别[D]. [博士论文], 国防科学技术大学, 2006. WANG Tao. Feature extraction and recognition of targets in ballistic midcourse in polarization-domain[D]. [Ph.D. dissertation], National University of Defense Technology, 2006.
[86]	SHI Longfei, WANG Xuesong, and XIAO Shunping. Polarization discrimination between repeater false-target and radar target[J]. Science in China Series F: Information Sciences, 2009, 52(1): 149–158. doi: 10.1007/s11432-009-0009-9
[87]	ZONG Zhiwei, SHI Longfei, and WANG Xuesong. Commonality used to discriminate active repetition false targets based on polarisation characteristics of antenna[J]. IET Radar, Sonar & Navigation, 2016, 10(7): 1178–1185. doi: 10.1049/iet-rsn.2015.0421
[88]	宗志伟. 弹道中段目标极化雷达识别方法[D]. [博士论文], 国防科学技术大学, 2016. ZONG Zhiwei. Discrimination methods for ballistic targets base on polarization radar[D]. [Ph.D. dissertation], National University of Defense Technology, 2016.
[89]	沈允春, 谢俊好, 刘庆普. 识别箔条云新方案[J]. 系统工程与电子技术, 1995(4): 60–63. doi: 10.3321/j.issn:1001-506X.1995.04.011 SHEN Yunchun, XIE Junhao, and LIU Qingpu. A novel scheme for identifying chaff interference[J]. Systems Engineering and Electronics, 1995(4): 60–63. doi: 10.3321/j.issn:1001-506X.1995.04.011
[90]	刘庆普, 沈允春. 箔条云极化识别方案性能分析[J]. 系统工程与电子技术, 1996(11): 1–7. doi: 10.3321/j.issn:1001-506X.1996.11.001 LIU Qingpu and SHEN Yunchun. Performance analysis of identifying chaff interference using polarization characteristics[J]. Systems Engineering and Electronics, 1996(11): 1–7. doi: 10.3321/j.issn:1001-506X.1996.11.001
[91]	李金梁. 箔条干扰的特性与雷达抗箔条技术研究[D]. [博士论文], 国防科学技术大学, 2010. LI Jinliang. Study on characteristics of chaff jamming and anti-chaff technology for radar[D]. [Ph.D. dissertation], National University of Defense Technology, 2010.
[92]	朱珍珍, 汤广富, 程翥, 等. 基于极化分解的舰船和角反射器鉴别方法[J]. 舰船电子对抗, 2010, 33(6): 15–21. doi: 10.3969/j.issn.1673-9167.2010.06.003 ZHU Zhenzhen, TANG Guangfu, CHENG Zhu, et al. Discrimination method of ship and corner reflector based on polarization decomposition[J]. Shipboard Electronic Countermeasure, 2010, 33(6): 15–21. doi: 10.3969/j.issn.1673-9167.2010.06.003
[93]	YU K B and MURROW D J. Adaptive digital beamforming for angle estimation in jamming[J]. IEEE Transactions on Aerospace and Electronic Systems, 2001, 37(2): 508–523. doi: 10.1109/7.937465
[94]	王雪松. 雷达极化技术研究现状与展望[J]. 雷达学报, 2016, 5(2): 119–131. doi: 10.12000/JR16039 WANG Xuesong. Status and prospects of radar polarimetry techniques[J]. Journal of Radars, 2016, 5(2): 119–131. doi: 10.12000/JR16039
[95]	施龙飞, 任博, 马佳智, 等. 雷达极化抗干扰技术进展[J]. 现代雷达, 2016, 38(4): 1–7, 29. doi: 10.16592/j.cnki.1004-7859.2016.04.001 SHI Longfei, REN Bo, MA Jiazhi, et al. Recent developments of radar anti-interference techniques with polarimetry[J]. Modern Radar, 2016, 38(4): 1–7, 29. doi: 10.16592/j.cnki.1004-7859.2016.04.001
[96]	胡航. 现代相控阵雷达阵列处理技术[M]. 北京: 国防工业出版社, 2017. HU Hang. Array Processing Techniques for Modern Phased Array Radar[M]. Beijing: National Defense Industry Press, 2017.
[97]	邱朝阳, 雷丽丽, 胡航, 等. 子阵级相控阵差波束旁瓣抑制新方法[J]. 电波科学学报, 2011, 26(1): 13–17, 202. doi: 10.3969/j.issn.1005-0388.2011.01.003 QIU Chaoyang, LEI Lili, HU Hang, et al. Novel approach of sidelobe suppression for difference beam of phased array at subarray level[J]. Chinese Journal of Radio Science, 2011, 26(1): 13–17, 202. doi: 10.3969/j.issn.1005-0388.2011.01.003
[98]	李荣锋, 饶灿, 戴凌燕, 等. 子阵间约束自适应和差单脉冲测角算法[J]. 华中科技大学学报(自然科学版), 2013, 41(9): 6–10. doi: 10.13245/j.hust.2013.09.013 LI Rongfeng, RAO Can, DAI Lingyan, et al. Algorithm for constrained adaptive sum-difference monopulse among sub-arrays[J]. Journal of Huazhong University of Science and Technology (Natural Science Edition), 2013, 41(9): 6–10. doi: 10.13245/j.hust.2013.09.013
[99]	CHEN Xinzhu, SHU Ting, YU K B, et al. Enhanced ADBF architecture for monopulse angle estimation in multiple jamming[J]. IEEE Antennas and Wireless Propagation Letters, 2017, 16: 2684–2687. doi: 10.1109/LAWP.2017.2740958
[100]	CHENG Ziyang, HE Zishu, DUAN Xiang, et al. Adaptive monopulse approach with joint linear constraints for planar array at subarray level[J]. IEEE Transactions on Aerospace and Electronic Systems, 2018, 54(3): 1432–1441. doi: 10.1109/TAES.2018.2793318
[101]	周必雷, 李荣锋, 戴凌燕, 等. 和差四通道及辅助阵元联合自适应单脉冲方法[J]. 系统工程与电子技术, 2017, 39(9): 1905–1914. doi: 10.3969/j.issn.1001-506X.2017.09.01 ZHOU Bilei, LI Rongfeng, DAI Lingyan, et al. Adaptive monopulse algorithm combining four-channel sum-difference beam and auxiliary elements[J]. Systems Engineering and Electronics, 2017, 39(9): 1905–1914. doi: 10.3969/j.issn.1001-506X.2017.09.01
[102]	陈功, 谢文冲, 王永良. 基于空时联合约束的机载雷达STAP单脉冲角度估计方法[J]. 电子学报, 2015, 43(3): 489–495. doi: 10.3969/j.issn.0372-2112.2015.03.011 CHEN Gong, XIE Wenchong, and WANG Yongliang. Space-time adaptive monopulse angle estimation approach for airborne radar based on space-time joint constraint[J]. Acta Electronica Sinica, 2015, 43(3): 489–495. doi: 10.3969/j.issn.0372-2112.2015.03.011
[103]	刘义, 赵志超, 王雪松, 等. 反辐射导弹复合测角抗诱偏干扰方法[J]. 宇航学报, 2009, 30(5): 2122–2127. doi: 10.3873/j.issn.1000-1328.2009.05.063 LIU Yi, ZHAO Zhichao, WANG Xuesong, et al. Approach of anti-decoy based on complex angle measuring system[J]. Journal of Astronautics, 2009, 30(5): 2122–2127. doi: 10.3873/j.issn.1000-1328.2009.05.063
[104]	宋立众, 乔晓林, 孟宪德. 一种单脉冲雷达中的极化估值与滤波算法[J]. 系统工程与电子技术, 2005, 27(5): 764–766. doi: 10.3321/j.issn:1001-506X.2005.05.002 SONG Lizhong, QIAO Xiaolin, and MENG Xiande. Algorithm of polarization estimation and filtering for mono-pulse radar[J]. Systems Engineering and Electronics, 2005, 27(5): 764–766. doi: 10.3321/j.issn:1001-506X.2005.05.002
[105]	MA Jiazhi, SHI Longfei, LI Yongzhen, et al. Angle estimation of extended targets in main-lobe interference with polarization filtering[J]. IEEE Transactions on Aerospace and Electronic Systems, 2017, 53(1): 169–189. doi: 10.1109/TAES.2017.2649783
[106]	MA Jiazhi, SHI Longfei, LI Yongzhen, et al. Angle estimation with polarization filtering: A single snapshot approach[J]. IEEE Transactions on Aerospace and Electronic Systems, 2018, 54(1): 257–268. doi: 10.1109/TAES.2017.2756218
[107]	YANG Yong, XIAO Shunping, FENG Dejun, et al. Polarisation oblique projection for radar seeker tracking in chaff centroid jamming environment without prior knowledge[J]. IET Radar, Sonar & Navigation, 2014, 8(9): 1195–1202. doi: 10.1049/iet-rsn.2013.0388
[108]	王文涛, 张剑云, 李小波, 等. Fast ICA应用于雷达抗主瓣干扰算法研究[J]. 信号处理, 2015, 31(4): 497–503. doi: 10.3969/j.issn.1003-0530.2015.04.016 WANG Wentao, ZHANG Jianyun, LI Xiaobo, et al. A study on radar mainlobe jamming suppression algorithm based on fast ICA[J]. Journal of Signal Processing, 2015, 31(4): 497–503. doi: 10.3969/j.issn.1003-0530.2015.04.016
[109]	温媛媛, 陈豪. 基于时域卷积盲信号分离的雷达干扰抑制算法[J]. 中国科学院大学学报, 2013, 30(4): 523–527. doi: 10.7523/j.issn.2095-6134.2013.04.014 WEN Yuanyuan and CHEN Hao. Algorithm of radar interference suppression based on blind signal separation in the time domain[J]. Journal of University of Chinese Academy of Sciences, 2013, 30(4): 523–527. doi: 10.7523/j.issn.2095-6134.2013.04.014
[110]	WASHIZAWA Y, YAMASHITA Y, TANAKA T, et al. Blind extraction of global signal from multi-channel noisy observations[J]. IEEE Transactions on Neural Networks, 2010, 21(9): 1472–1481. doi: 10.1109/TNN.2010.2052828
[111]	王瑜, 李小波, 周青松, 等. 联合BSS和FRFT的雷达抗主瓣干扰新方法[J]. 现代雷达, 2016, 38(7): 72–77. doi: 10.16592/j.cnki.1004-7859.2016.07.018 WANG Yu, LI Xiaobo, ZHOU Qingsong, et al. A new method of radar main lobe interference based on the combination of BSS and FRFT[J]. Modern Radar, 2016, 38(7): 72–77. doi: 10.16592/j.cnki.1004-7859.2016.07.018
[112]	LI Jiong, ZHANG Hang, and ZHANG Jiang. Fast adaptive BSS algorithm for independent/dependent sources[J]. IEEE Communications Letters, 2016, 20(11): 2221–2224. doi: 10.1109/LCOMM.2016.2598144
[113]	周必雷, 李荣锋, 陈风波, 等. 基于盲分离的空时联合处理抗复合干扰方法[J]. 系统工程与电子技术, 2018, 40(11): 2393–2402. doi: 10.3969/j.issn.1001-506X.2018.11.01 ZHOU Bilei, LI Rongfeng, CHEN Fengbo, et al. Space-time complex-jamming suppression algorithm based on the BSS[J]. Systems Engineering and Electronics, 2018, 40(11): 2393–2402. doi: 10.3969/j.issn.1001-506X.2018.11.01
[114]	LEE S H, LEE S J, CHOI I O, et al. ICA-based phase-comparison monopulse technique for accurate angle estimation of multiple targets[J]. IET Radar, Sonar & Navigation, 2018, 12(3): 323–331. doi: 10.1049/iet-rsn.2017.0156
[115]	周伟江, 王培强, 张进, 等. 雷达射频掩护信号分析及对抗方法研究[J]. 航天电子对抗, 2013, 29(5): 47–50. doi: 10.3969/j.issn.1673-2421.2013.05.014 ZHOU Weijiang, WANG Peiqiang, ZHANG Jin, et al. Analysis and countermeasures of radar radio frequency-screen signal[J]. Aerospace Electronic Warfare, 2013, 29(5): 47–50. doi: 10.3969/j.issn.1673-2421.2013.05.014
[116]	金珊珊, 王春阳, 邱程, 等. 对抗应答式干扰的射频掩护脉冲设计[J]. 中国电子科学研究院学报, 2014, 9(4): 377–381. doi: 10.3969/j.issn.1673-5692.2014.04.010 JIN Shanshan, WANG Chunyang, QIU Cheng, et al. Design of RF protecting signal for transponder jamming suppression[J]. Journal of CSEIT, 2014, 9(4): 377–381. doi: 10.3969/j.issn.1673-5692.2014.04.010
[117]	李凤从. 雷达抗干扰波形优化设计的研究[D]. [博士论文], 哈尔滨工业大学, 2014. LI Fengcong. Research on anti-interference waveform optimization for radar[D]. [Ph.D. dissertation], Harbin Institute of Technology, 2014.
[118]	MA Jiazhi, SHI Longfei, XIAO Shunping, et al. Mitigation of cross-eye jamming using a dual-polarization array[J]. Journal of Systems Engineering and Electronics, 2018, 29(3): 491–498. doi: 10.21629/JSEE.2018.03.06
[119]	白渭雄, 唐宏, 陶建峰. 拖曳式诱饵对单脉冲雷达的干扰分析[J]. 电子信息对抗技术, 2007, 22(6): 39–42. doi: 10.3969/j.issn.1674-2230.2007.06.010 BAI Weixiong, TANG Hong, and TAO Jianfeng. Analysis of towed decoy jamming on monopulse radar[J]. Electronic Information Warfare Technology, 2007, 22(6): 39–42. doi: 10.3969/j.issn.1674-2230.2007.06.010
[120]	廖云, 何松华, 张军. 脉冲多普勒雷达抗拖曳式干扰方法研究[J]. 雷达科学与技术, 2009, 7(5): 325–328. doi: 10.3969/j.issn.1672-2337.2009.05.001 LIAO Yun, HE Songhua, and ZHANG Jun. Method research on mono-pulse Doppler radar countering towed decoy jamming[J]. Radar Science and Technology, 2009, 7(5): 325–328. doi: 10.3969/j.issn.1672-2337.2009.05.001
[121]	YANG Yong, FENG Dejun, ZHANG Wenming, et al. Detection of chaff centroid jamming aided by GPS/INS[J]. IET Radar, Sonar & Navigation, 2013, 7(2): 130–142. doi: 10.1049/iet-rsn.2012.0101
[122]	徐娟, 姚如贵, 陈赟, 等. 频域空时域级联导航抗干扰技术研究[J]. 弹箭与制导学报, 2015, 35(2): 137–141. doi: 10.15892/j.cnki.djzdxb.2015.02.035 XU Juan, YAO Rugui, CHEN Yun, et al. Cascaded frequency and spatial-time domain anti-jamming technique in navigation systems[J]. Journal of Projectiles,Rockets,Missiles and Guidance, 2015, 35(2): 137–141. doi: 10.15892/j.cnki.djzdxb.2015.02.035
[123]	WANG LUO Shengbin, XU Zhenhai, LIU Xinghua, et al. Subarray-based frequency diverse array for target range-angle localization with monopulse processing[J]. IEEE Sensors Journal, 2018, 18(14): 5937–5947. doi: 10.1109/JSEN.2018.2844280
[124]	龙杰. 单脉冲前斜SAR成像信息处理技术研究[D]. [博士论文], 北京理工大学, 2014. LONG Jie. Research on monopulse squint SAR imaging information processing technique[D]. [Ph.D. dissertation], Beijing Institute of Technology, 2014.