一种基于误差反向传播优化的多通道SAR相位误差估计方法

崔磊; 仇晓兰; 郭嘉逸; 温雪娇; 杨俊莹; 付琨

doi:10.12000/JR20096

一种基于误差反向传播优化的多通道SAR相位误差估计方法

DOI: 10.12000/JR20096

崔磊^1, ,,
仇晓兰^{1, 2, 3, ,},
郭嘉逸^{2, 3},
温雪娇¹,
杨俊莹^{2, 3},
付琨^{2, 3}

1.
中国科学院电子学研究所苏州研究院苏州 215123
2.
中国科学院空天信息创新研究院北京 100190
3.
微波成像技术国家级重点实验室北京 100190

基金项目: 国家自然科学基金(61991420, 61991421)

详细信息

作者简介:
崔　磊(1989–)，男，甘肃天水人，2016年在北京理工大学信息与电子学院获得硕士学位，中国科学院电子学研究所苏州研究院助理研究员。主要研究领域为SAR数据预处理。E-mail: cuilei1167@163.com

仇晓兰(1982–)，女，中国科学院空天信息创新研究院研究员，博士生导师，主要研究领域为SAR成像处理、SAR图像理解，IEEE高级会员、IEEE地球科学与遥感快报副主编、雷达学报青年编委。E-mail: xlqiu@mail.ie.ac.cn

通讯作者:
崔磊 cuilei1167@163.com

仇晓兰 xlqiu@mail.ie.ac.cn

责任主编：陈杰 Corresponding Editor: CHEN Jie
中图分类号: TN957.52
计量
- 文章访问数: 1855
- HTML全文浏览量: 559
- PDF下载量: 127
- 被引次数: 0
出版历程
- 收稿日期: 2020-07-07
- 修回日期: 2020-09-04
- 网络出版日期: 2020-10-28

Multi-channel Phase Error Estimation Method Based on an Error Backpropagation Algorithm for a Multichannel SAR

CUI Lei^{1
, ,},
QIU Xiaolan^{1, 2, 3
, ,},
GUO Jiayi^{2, 3},
WEN Xuejiao¹,
YANG Junying^{2, 3},
FU Kun^{2, 3}

1.
Institute of Electronics, Chinese Academy of Sciences, Suzhou Research Center, Suzhou 215123, China
2.
Aerospace Information Research Institute, Chinese Academy of Sciences, Beijing 100190, China
3.
National Key Lab of Microwave Imaging Technology, Beijing 100190, China

Funds: The National Natural Science Foundation of China (61991420, 61991421)

More Information

Corresponding author: CUI lei, cuilei1167@163.com; QIU Xiaolan, xlqiu@mail.ie.ac.cn

摘要

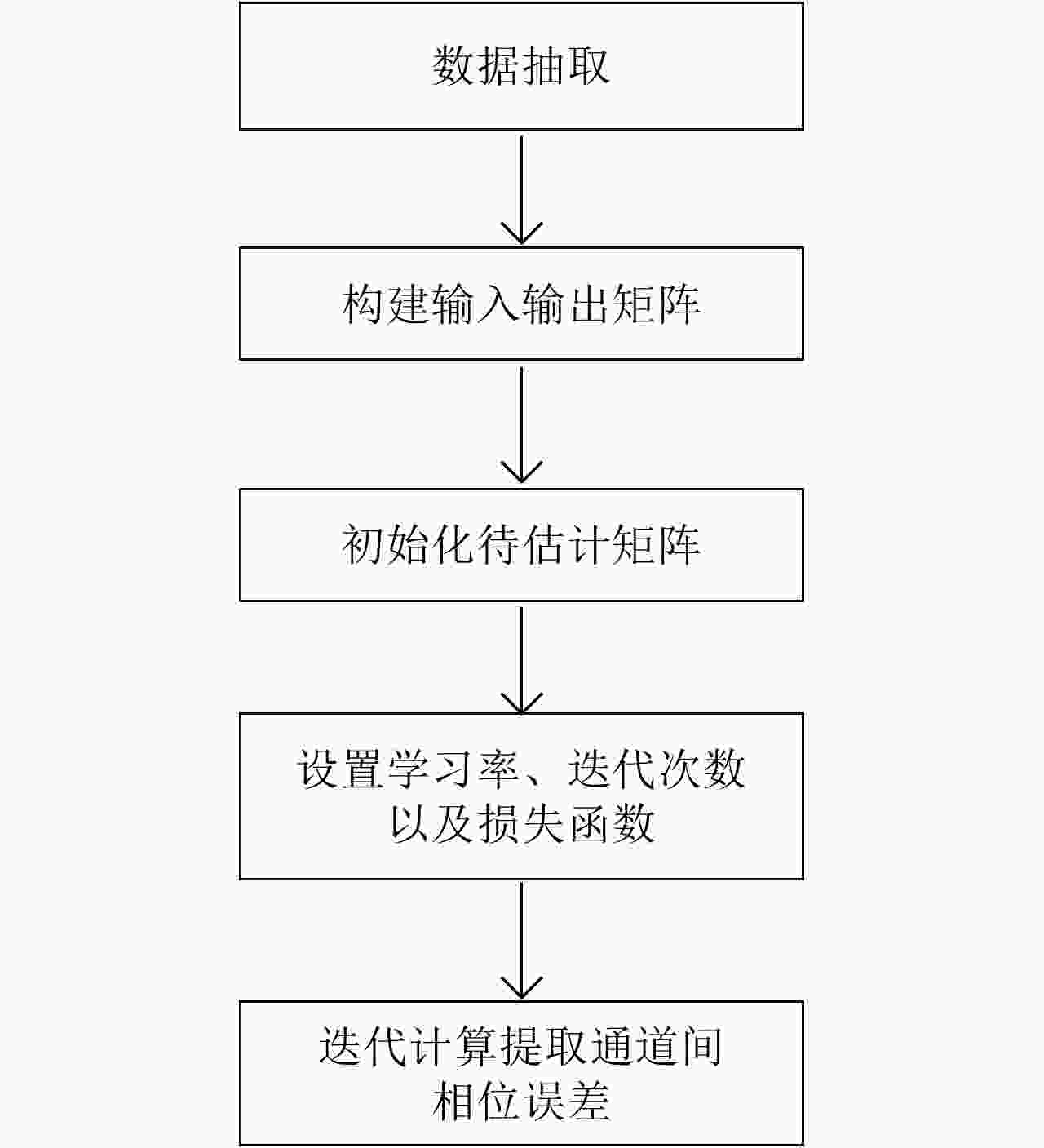
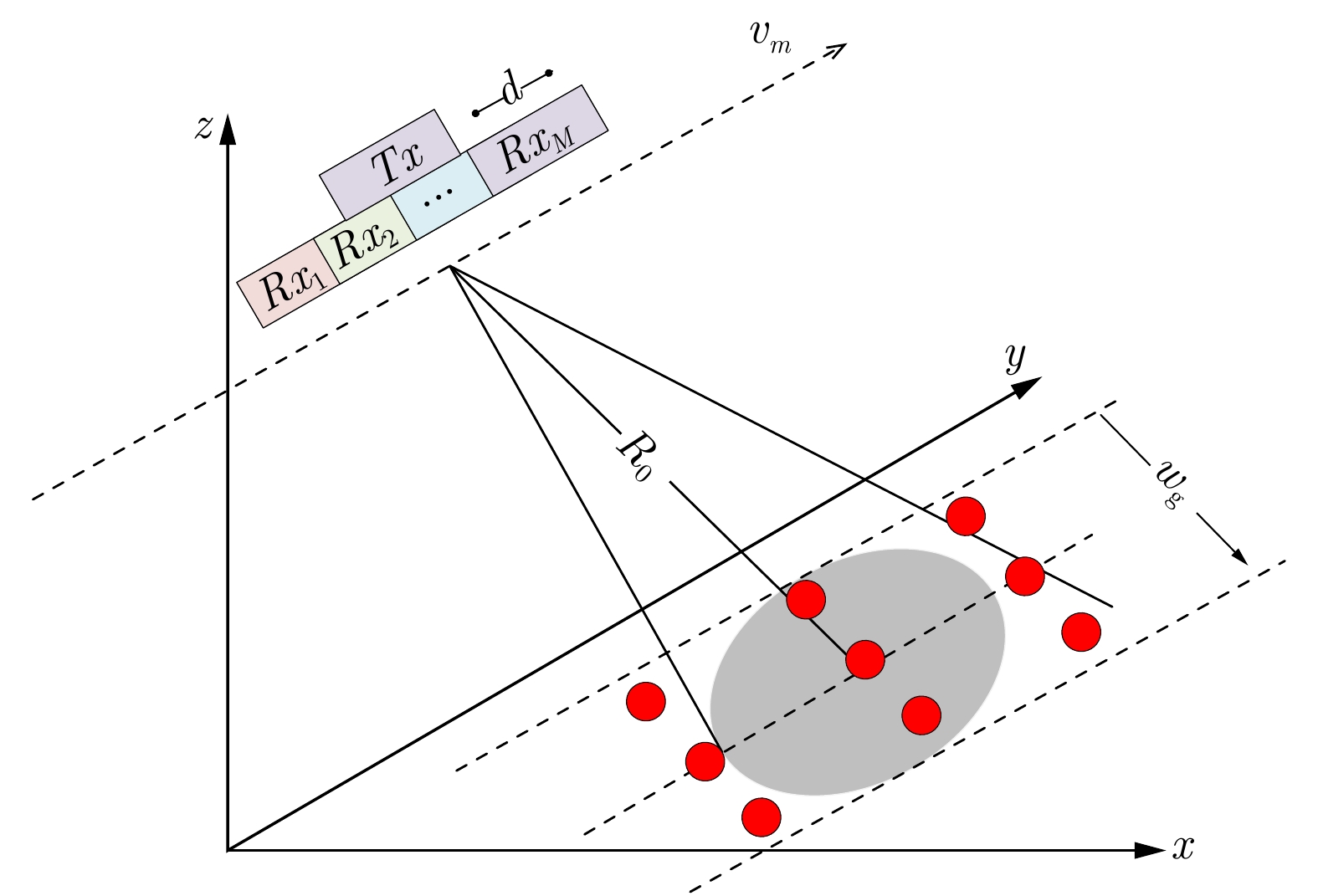
摘要: 方位向多通道合成孔径雷达(SAR)可实现高分辨率宽测绘带成像，准确估计通道间相位误差是保障成像质量的关键。该文提出了基于误差反向传播训练优化的通道相位误差估计方法，该方法根据多通道SAR回波生成的物理过程，构建含有通道间相位误差待估计参数的观测矩阵，通过初始化的通道误差和初始化的目标散射系数参数生成初始化的SAR回波，并计算该回波与多通道SAR实测回波之间的误差，通过深度学习中常用的误差反向传播的方法，不断训练优化上述参数，最终获得通道间相位误差的估计值，同时也得到了对稀疏目标散射系数的估计。该方法基于误差反向传播方法，并将该方法与通道误差的形成原理相结合，在稀疏假设下同时完成了相位估计和成像，为多通道SAR误差估计提供了一种全新的思路。多通道SAR仿真数据验证了该文算法的有效性。
- 合成孔径雷达 /
- 高分辨率宽测绘带 /
- 误差反向传播 /
- 多通道SAR /
- 相位误差估计
Abstract: An azimuth multi-channel Synthetic Aperture Radar (SAR) can be used to obtain high-resolution wide-swath SAR images. Accurate estimation of the phase error between channels is the key to ensuring image quality. In this study, we present a channel phase error estimation method based on the error backpropagation algorithm. During the physical process of a multi-channel SAR echo generation, this method constructs an observation matrix with the parameters to be estimated including the phase error between channels. The initial SAR echo is generated using the initial channel error matrix and initial target scattering coefficient matrix, and the error between the echo and measured multi-channel SAR echo is calculated. Using the backpropagation algorithm commonly used in deep learning, the abovementioned parameters are continuously trained and optimized. Finally, the estimation of the phase error between channels is obtained along with the target scattering coefficient. This method combines the error backpropagation method with the principle of multi-channel SAR channel error. Phase estimation and imaging are realized based on the sparsity assumption, which provides a new approach for estimating an error in a multi-channel SAR. The effectiveness of the presented method is validated using multi-channel SAR simulation data.
- Synthetic Aperture Radar (SAR) /
- High-resolution wide-swath /
- Error backpropagation algorithm /
- Multi-channel SAR /
- Phase error estimation

HTML全文

图 1 方位多通道SAR系统对地观测成像构型示意图

Figure 1. Ground observation imaging configuration of azimuth multi-channel SAR system

下载: 全尺寸图片幻灯片

图 2 基于反向传播优化的多通道SAR相位误差估计算法流程图

Figure 2. Flow chart of channel phase error estimation method based on backpropagation algorithm for multichannel SAR

下载: 全尺寸图片幻灯片

图 3 场景目标示意图

Figure 3. Scene target diagram

下载: 全尺寸图片幻灯片

图 4 回波抽取示意图

Figure 4. Schematic diagram of echo data extraction

下载: 全尺寸图片幻灯片

图 5 无噪声估计结果

Figure 5. Noiseless estimation results

下载: 全尺寸图片幻灯片

图 6 估计结果(SNR=0 dB)

Figure 6. Estimation results (SNR=0 dB)

下载: 全尺寸图片幻灯片

图 7 估计结果(SNR=–5 dB)

Figure 7. Estimation results (SNR=–5 dB)

下载: 全尺寸图片幻灯片

表 1 仿真实验系统参数

Table 1. Parameters of simulation experiment system

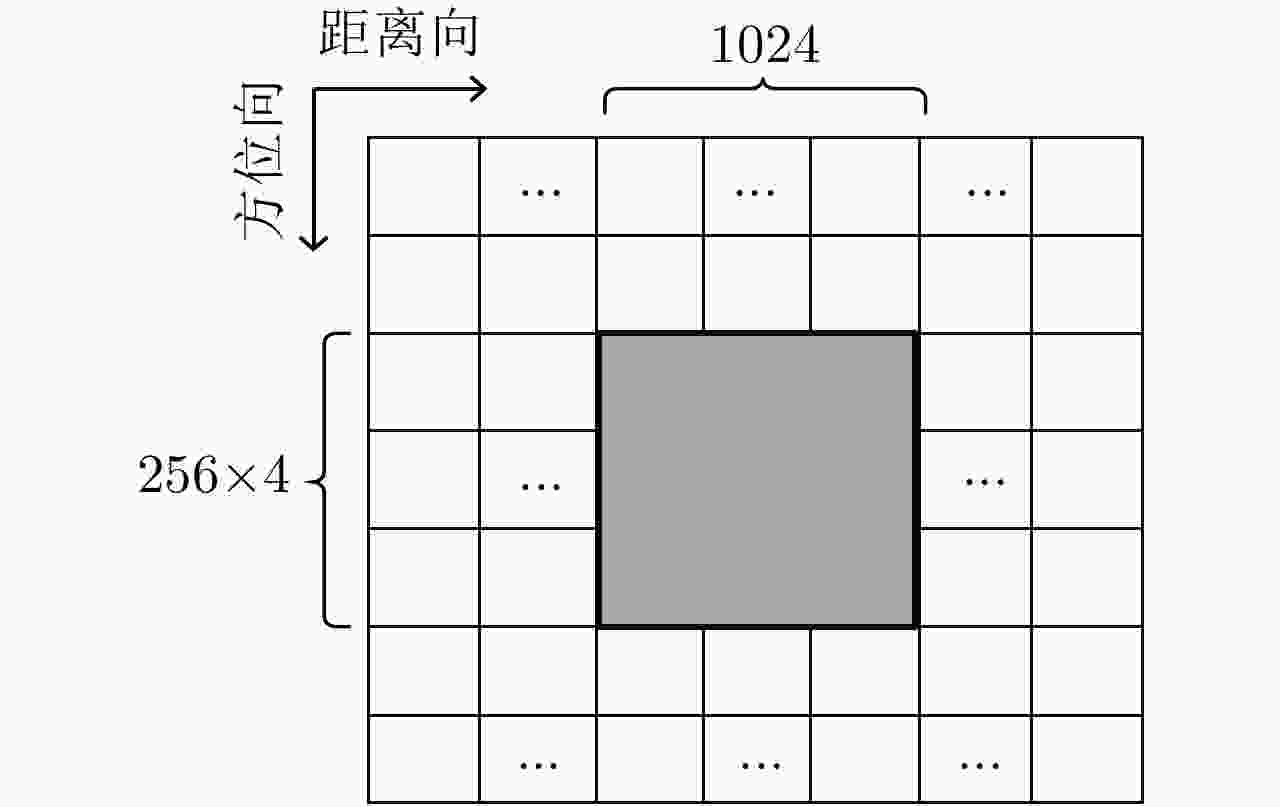
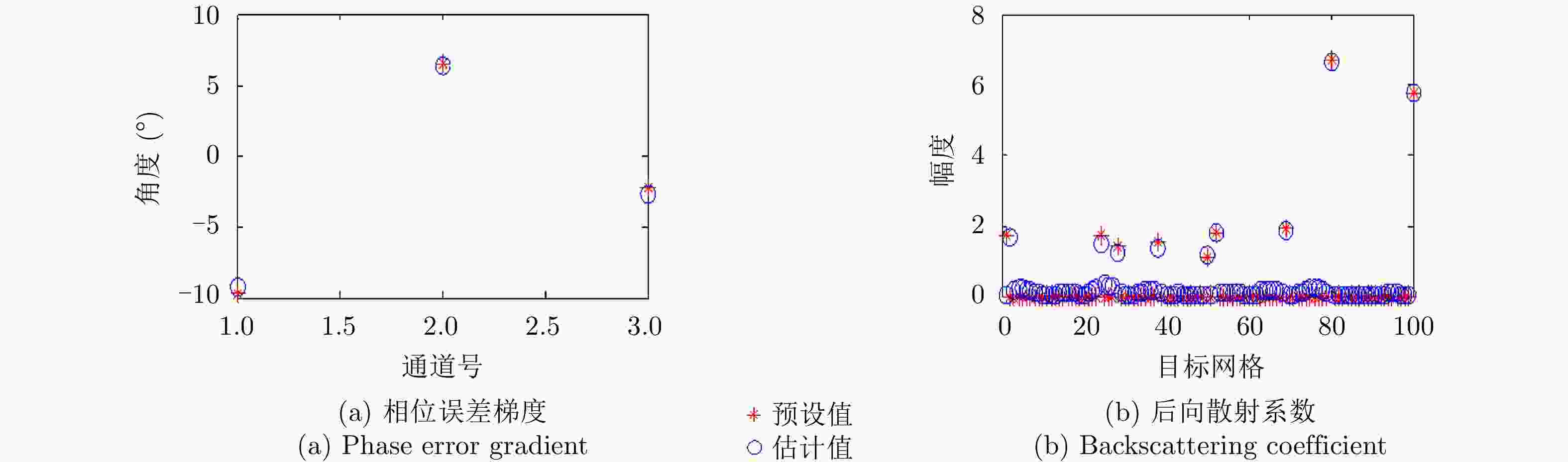
参数	取值
通道数	4
雷达载频(GHz)	5.35
信号带宽(MHz)	210.00
脉冲重复频率(Hz)	335.10
方位向波束宽度(°)	5.14
雷达高度(m)	4950.00
雷达距场景中心的距离(m)	7000.00
雷达方位向等效速度(m/s)	123.00
场景方位向宽度(m)	20.00
场景地距向宽度(m)	20.00
距离采样个数	4096
方位回波个数	3166

下载: 导出CSV

表 2 通道间相位误差估计结果(°)

Table 2. Estimation results of channel phase error (°)

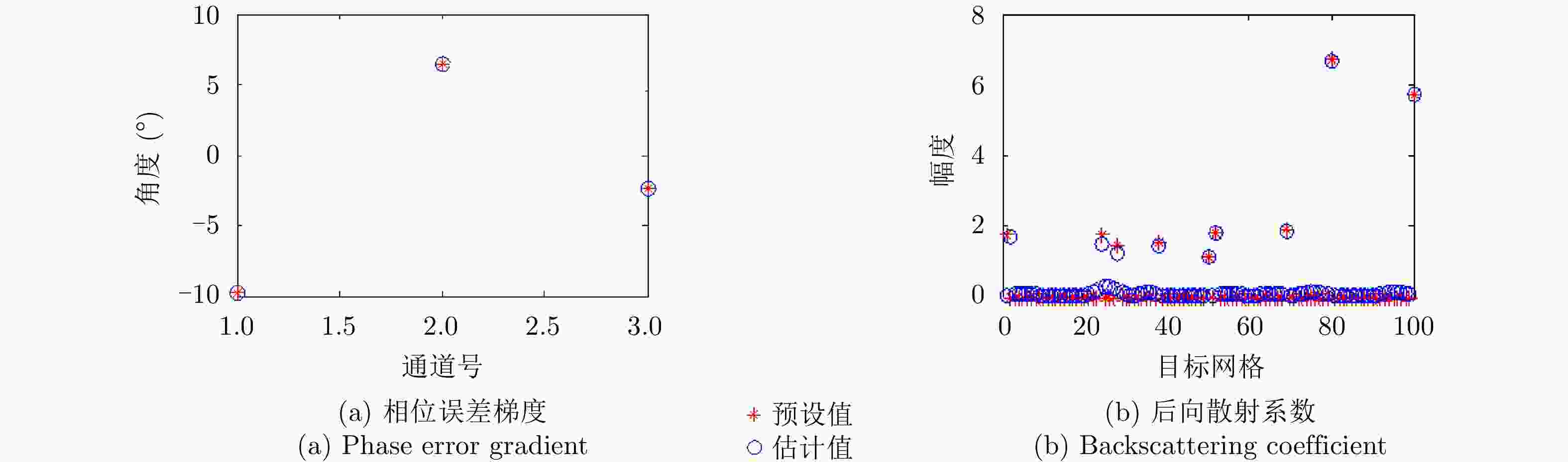
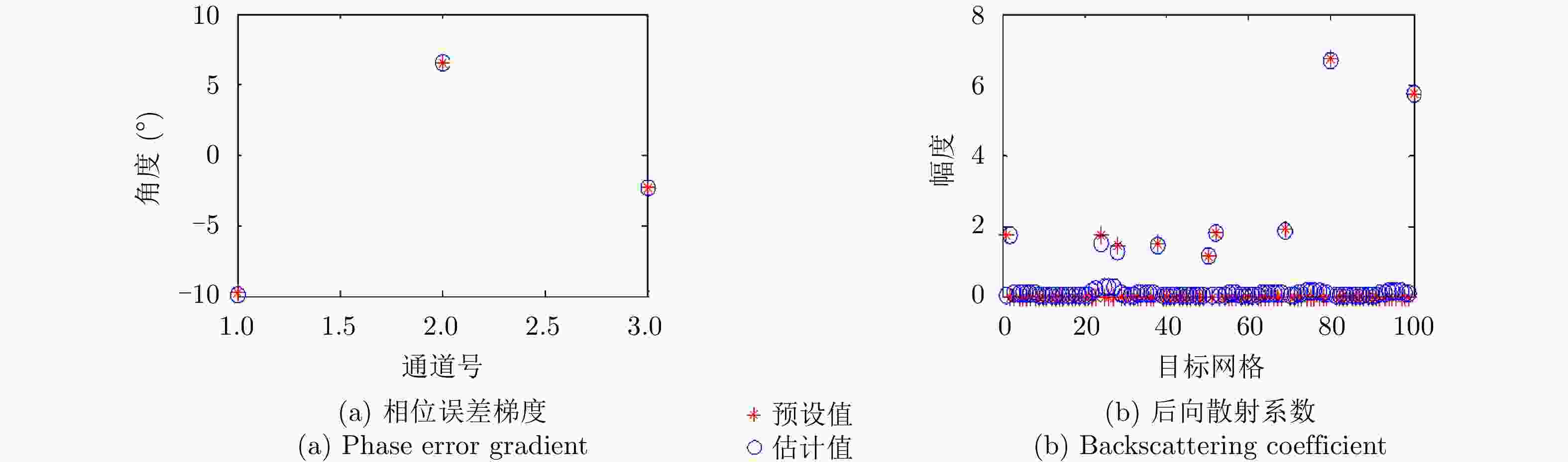
相位误差	通道1/2相位误差	通道2/3相位误差	通道3/4相位误差
实际相位偏差	–9.82	6.44	–2.34
无噪估计	–9.81	6.44	–2.34
SNR=0 dB估计	–9.87	6.46	–2.33
SNR=–5 dB估计	–9.35	6.30	–2.76

下载: 导出CSV

参考文献(19)

[1]	FREEMAN A, JOHNSON W T K, HUNEYCUTT B, et al. The “Myth” of the minimum SAR antenna area constraint[J]. IEEE Transactions on Geoscience and Remote Sensing, 2000, 38(1): 320–324. doi: 10.1109/36.823926
[2]	MOREIRA J, SCHWABISCH M, FORNARO G, et al. X-SAR interferometry: First results[J]. IEEE Transactions on Geoscience and Remote Sensing, 1995, 33(4): 950–956. doi: 10.1109/36.406681
[3]	JIN Tingting, QIU Xiaolan, HU Donghui, et al. Unambiguous imaging of static scenes and moving targets with the first Chinese Dual-Channel spaceborne SAR sensor[J]. Sensors, 2017, 17(8): 1709. doi: 10.3390/s17081709
[4]	刘艳阳, 李真芳, 杨桃丽, 等. 一种单星方位多通道高分辨率宽测绘带SAR系统通道相位偏差时域估计新方法[J]. 电子与信息学报, 2012, 34(12): 2913–2919. doi: 10.3724/SP.J.1146.2012.00562 LIU Yanyang, LI Zhenfang, YANG Taoli, et al. A novel channel phase bias estimation method for spaceborne along-track multi-channel HRWS SAR in time-domain[J]. Journal of Electronics &Information Technology, 2012, 34(12): 2913–2919. doi: 10.3724/SP.J.1146.2012.00562
[5]	LI Zhenfang, BAO Zheng, WANG Hongyang, et al. Performance improvement for constellation SAR using signal processing techniques[J]. IEEE Transactions on Aerospace and Electronic Systems, 2006, 42(2): 436–452. doi: 10.1109/TAES.2006.1642562
[6]	张磊, 全英汇, 邢孟道, 等. 一种子空间投影的高分辨宽测绘带SAR成像通道均衡方法[J]. 电子与信息学报, 2010, 32(1): 1–6. doi: doi:10.3724/SP.J.1146.2008.01821 ZHANG Lei, QUN Yinghui, XING Mengdao, et al. An SSP based channel calibration for high-resolution and wide-swath sar imagery[J]. Journal of Electronics &Information Technology, 2010, 32(1): 1–6. doi: doi:10.3724/SP.J.1146.2008.01821
[7]	杨桃丽, 李真, 刘艳阳, 等. 两种星载高分辨宽测绘带SAR系统通道相位误差估计方法[J]. 电子学报, 2013, 41(5): 931–935. doi: 10.3969/j.issn.0372-2112.2013.05.016 YANG Taoli, LI Zhen, LIU Yanyang, et al. Two channel phase error estimation methods for spaceborne HRWS SAR system[J]. Acta Electronica Sinica, 2013, 41(5): 931–935. doi: 10.3969/j.issn.0372-2112.2013.05.016
[8]	LIU Yanyang, LI Zhenfang, YANG Taoli, et al. An adaptively weighted least square estimation method of channel mismatches in phase for multichannel SAR systems in azimuth[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(2): 439–443. doi: 10.1109/LGRS.2013.2264771
[9]	JIN Tingting, QIU Xiaolan, and HU Donghui. Estimation accuracy and Cramér–Rao Lower bounds for errors in multichannel HRWS SAR systems[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(12): 1772–1776. doi: 10.1109/LGRS.2016.2608386
[10]	ZHANG L, XING M D, QIU C W, et al. Adaptive two-step calibration for high resolution and wide-swath SAR imaging[J]. IET Radar, Sonar & Navigation, 2010, 4(4): 548–559.
[11]	GEBERT N and KRIEGER G. Azimuth phase center adaptation on transmit for high-resolution wide-swath SAR imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2009, 6(4): 782–786. doi: 10.1109/LGRS.2009.2025245
[12]	王志斌, 刘艳阳, 李真芳, 等. 基于多普勒谱优化的HRWS SAR系统通道相位偏差估计算法[J]. 电子与信息学报, 2016, 38(12): 3026–3033. doi: 10.11999/JEIT161038 WANG Zhibin, LIU Yanyang, LI Zhenfang, et al. Phase bias estimation algorithm for HRWS SAR system in azimuth based on Doppler spectrum optimization[J]. Journal of Electronics &Information Technology, 2016, 38(12): 3026–3033. doi: 10.11999/JEIT161038
[13]	束宇翔. 分布式卫星多相位中心SAR-GMTI技术研究[D]. [博士论文], 西安电子科技大学, 2014. SHU Yuxiang. Research on techniques of SAR-GMTI for distributed satellite SAR systems with multiple phase centers[D]. [Ph. D. dissertation], Xidian University, 2014.
[14]	JANOTH J, JOCHUM M, PETRAT L, et al. High resolution wide swath–the next generation X-band mission[C]. 2019 IEEE International Geoscience and Remote Sensing Symposium, Yokohama, Japan, 2019: 3535–3537.
[15]	HAWKES M, NEHORAI A, STOICA P. Performance breakdown of subspace-based methods: Prediction and cure[C]. 2001 IEEE International Conference on Acoustics, Speech, and Signal. Salt Lake City, USA, 2001.
[16]	SHANG Mingyang, QIU Xiaolan, HAN Bing, et al. Channel imbalances and along-track baseline estimation for the GF-3 azimuth multichannel mode[J]. Remote Sensing, 2019, 11(11): 1297. doi: 10.3390/rs11111297
[17]	ZHANG Linjian, GAO Yesheng, and LIU Xingzhao. Robust channel phase error calibration algorithm for multichannel high-resolution and wide-swath SAR imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2017, 14(5): 649–653. doi: 10.1109/LGRS.2017.2668390
[18]	YANG Taoli, LI Zhenfang, LIU Yanyang, et al. Channel error estimation methods for multi-channel HRWS SAR systems[C]. 2013 IEEE International Geoscience and Remote Sensing Symposium, Melbourne, Australia, 2013.
[19]	DE ALMEIDA F Q, YOUNIS M, KRIEGER G, et al. An analytical error model for Spaceborne SAR multichannel azimuth reconstruction[J]. IEEE Geoscience and Remote Sensing Letters, 2018, 15(6): 853–857. doi: 10.1109/LGRS.2018.2811786