PSF Analysis and Ground Test Results of a Novel Circular Array 3-D SAR System
-
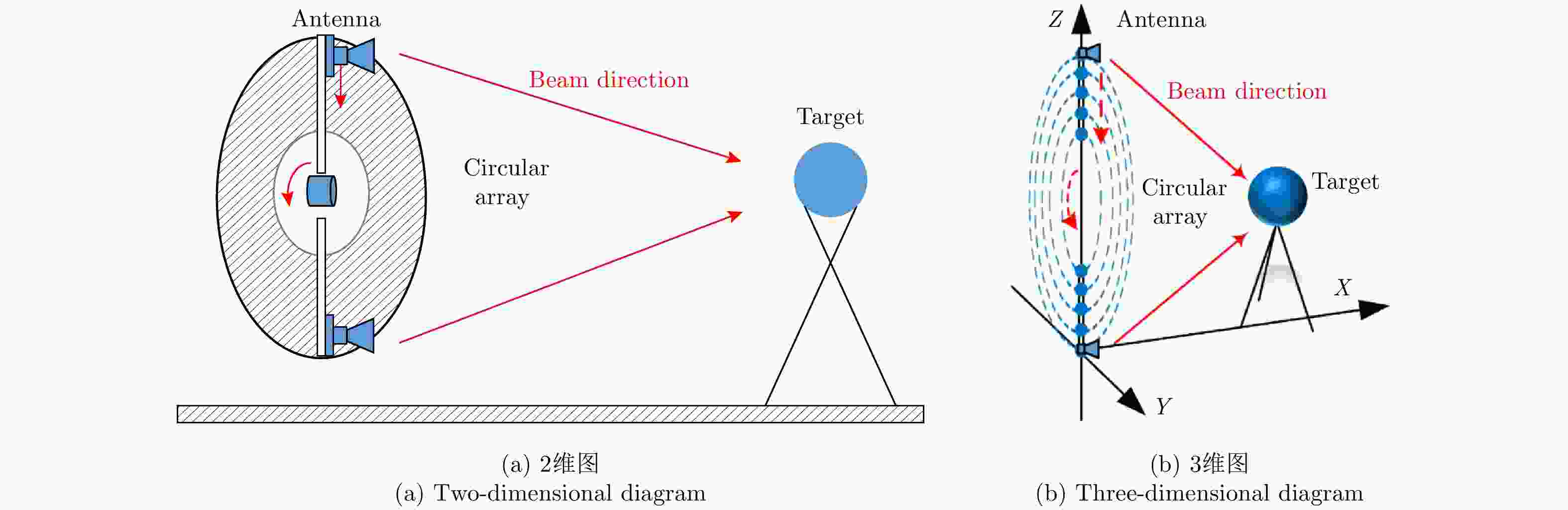
摘要: 阵列合成孔径雷达(ASAR)具备3维成像能力,是3维SAR成像领域的研究热点之一。该文针对线阵SAR在高分辨率成像方面和圆周SAR在旁瓣抑制方面的问题,提出一种新型圆迹阵列合成孔径雷达(CASAR)系统用于3维高分辨率雷达成像。首先推导基于CASAR系统的点扩散函数模型,从理论上分析圆迹阵列这一新型构型在3维成像中的优势。在此基础上构建原型CASAR实验系统,通过点扩散函数仿真实验和室外实测3维CASAR成像实验验证了3维CASAR成像的有效性,与线阵SAR和圆周SAR实验结果相比,证明CASAR系统可获得3维高分辨率SAR图像以及有效的旁瓣抑制能力。Abstract: Array Synthetic Aperture Radar (ASAR) system radar imaging field has gained attention because of its three-dimensional (3-D) imaging ability. A novel Circular Array SAR (CASAR) system is employed for 3-D high-resolution radar imaging to overcome the drawbacks exhibited by linear array SAR in high-resolution imaging and by circular SAR in side-lobe suppression. Point Spread Function (PSF) model based on the CASAR system is derived, and the advantages of the new configuration of the circular array in 3-D imaging are theoretically analyzed. Prototype CASAR experimental system is built on the basis of the abovementioned analysis. PSF simulation experiments and outdoor 3-D CASAR imaging experiments under outdoor conditions are performed to verify performance of the CASAR system in 3-D SAR imaging. Compared imaging results acquired using linear array SAR, circular SAR, and CASAR system proves that the CASAR system has high-resolution 3-D SAR images and demonstrates effective side-lobe suppression capability.
-
Key words:
- Synthetic Aperture Radar (SAR) /
- Circular array /
- 3-D imaging /
- High-resolution /
- Side-lobe suppression
-
表 1 仿真参数
Table 1. Simulation parameters
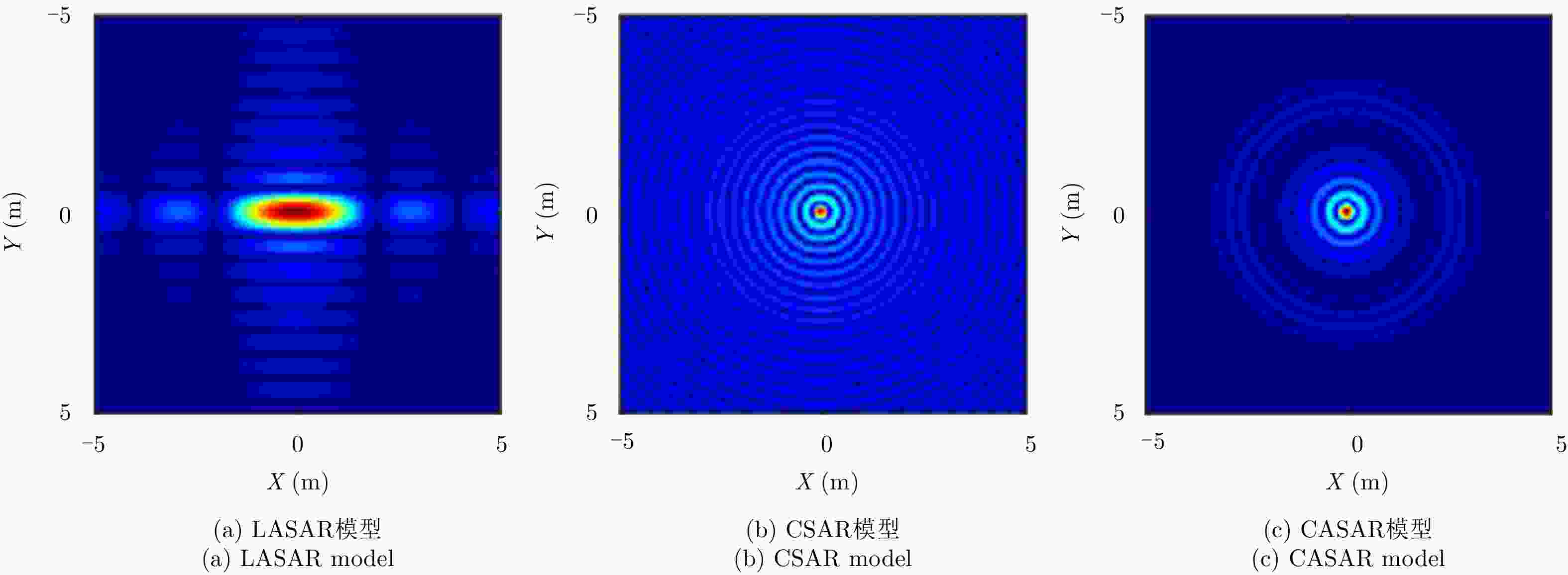
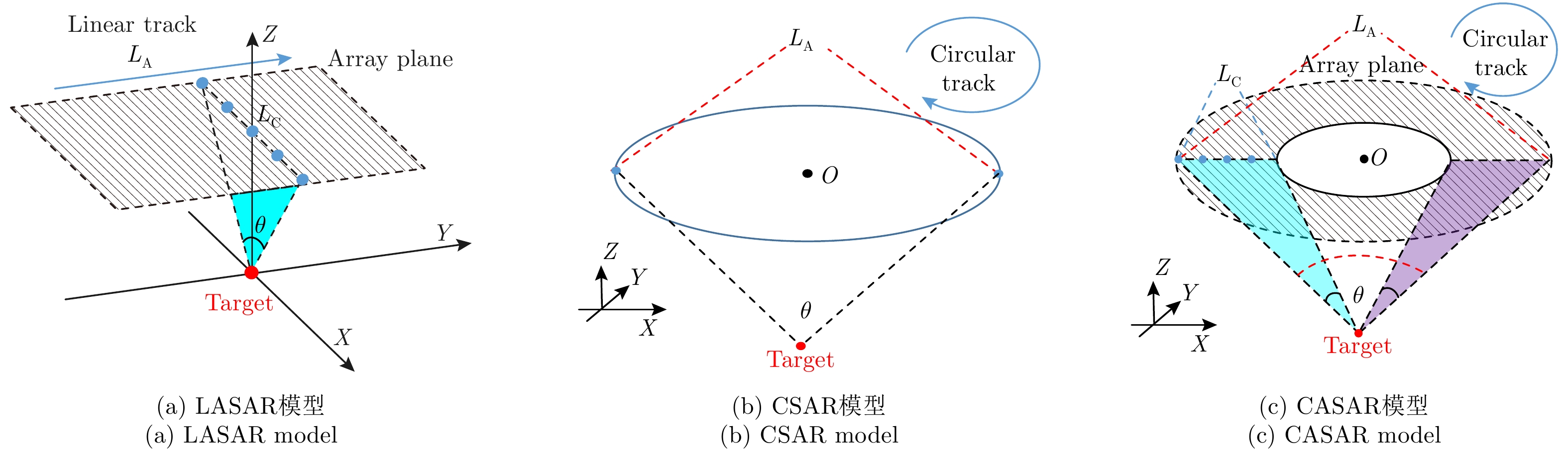
仿真参数 SAR系统 LASAR CSAR CASAR 信号 单脉冲 单脉冲 单脉冲 载频(GHz) 5 5 5 参考距离(m) 1000 1000 1000 PRF (Hz) 10 10 10 平台速度(m/s) 6 6 6 合成孔径时间(s) 105 105 105 目标位置 (0, 0, 0) (0, 0, 0) (0, 0, 0) 线阵单元数 500 1 500 线阵长度(m) 30 – 30 圆周半径 $r$ (m) – 100 70~100 合成孔径长度 – 2 ${{π}}$r 2 ${{π}}$r 表 2 切航迹向性能比较
Table 2. Cross-track performance comparison
实验模型 3 dB带宽(m) PSLR(dB) ISLR(dB) LASAR 1.80 –13.28 –11.60 CSAR 0.22 –7.89 –1.58 CASAR 0.25 –8.56 –5.31 表 3 实验参数
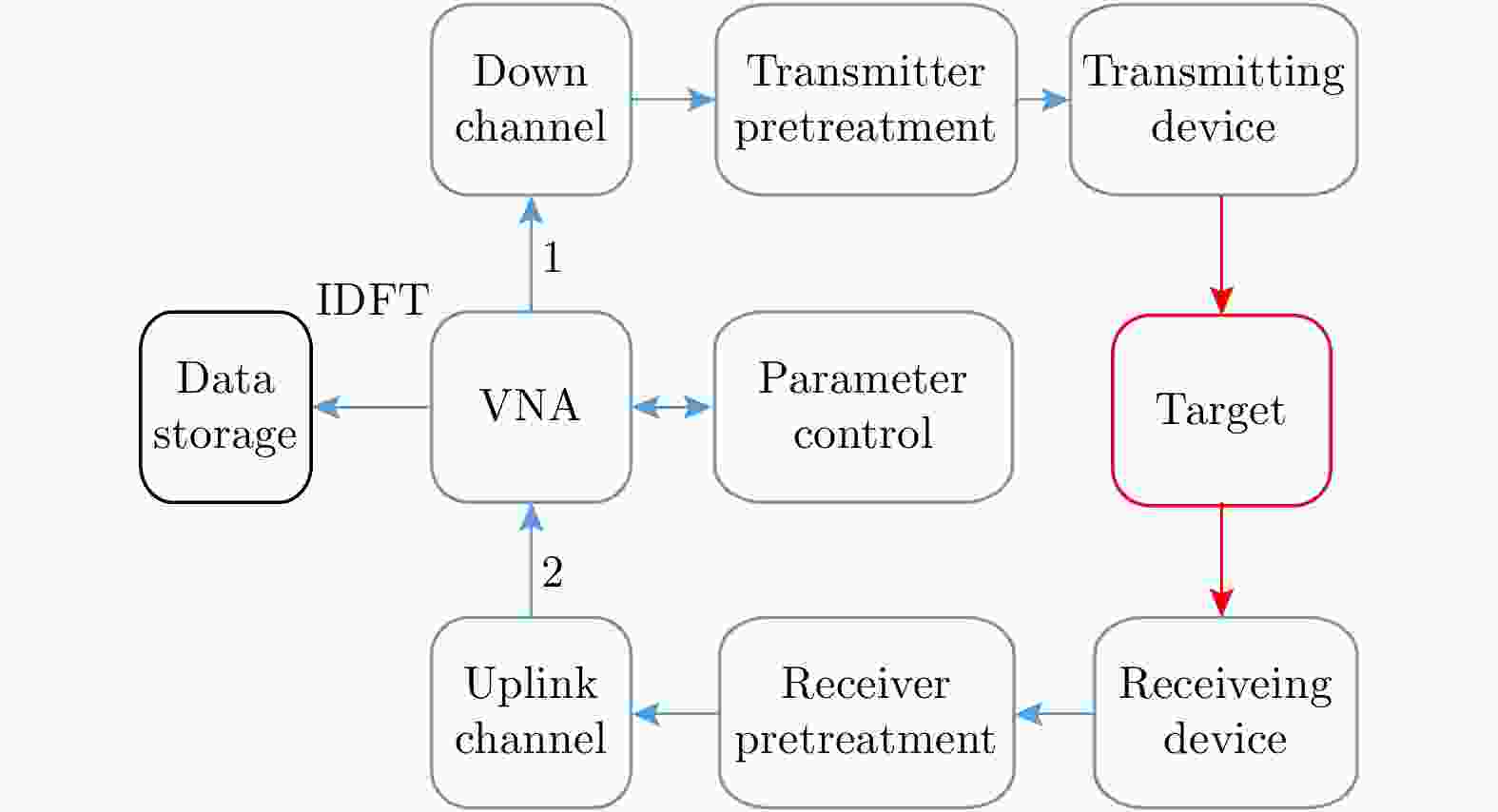
Table 3. Experiment parameters
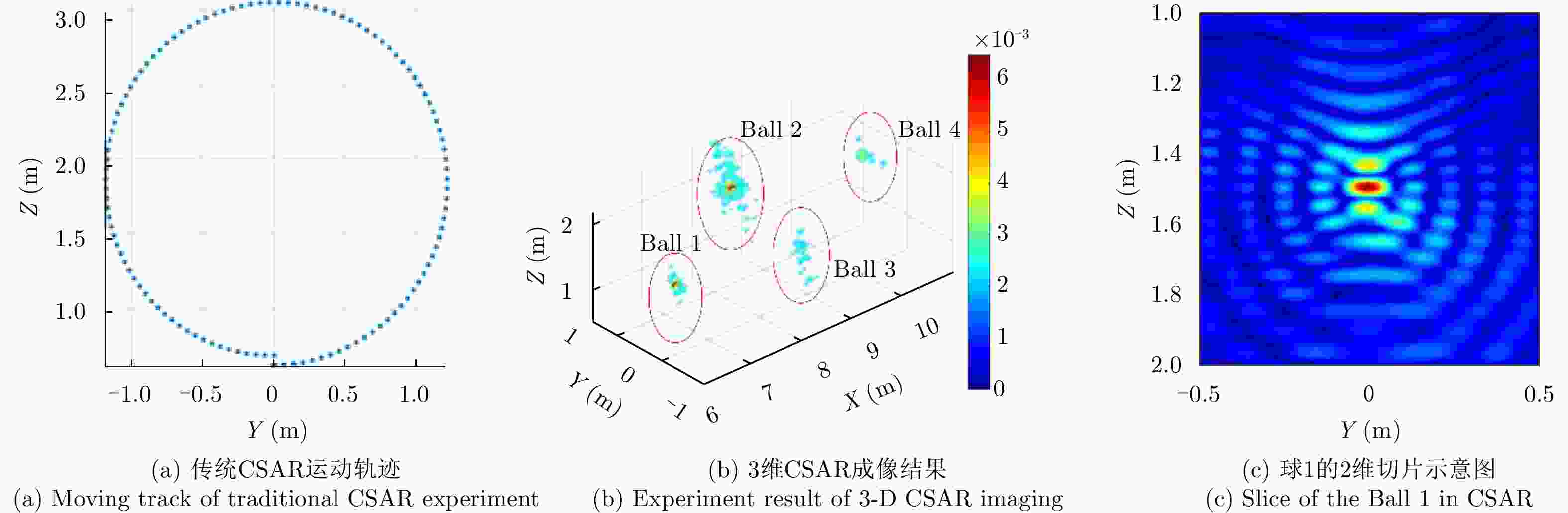
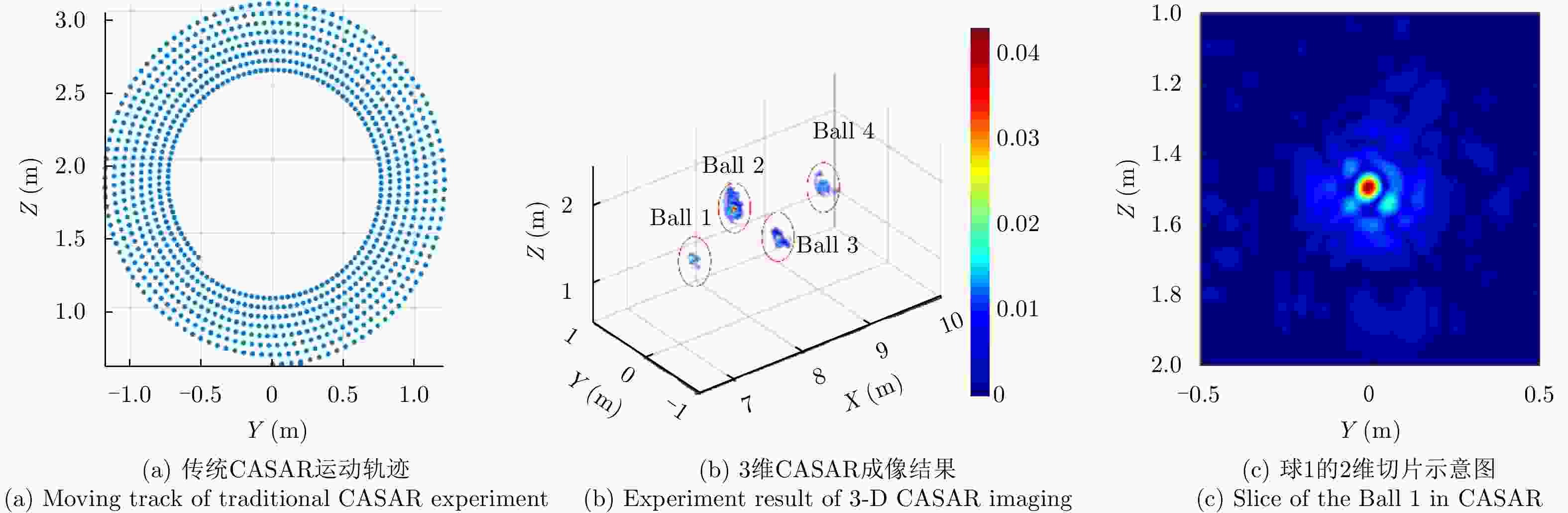
CASAR参数 值 起始频率(GHz) 6.5 终止频率(GHz) 8.5 SF信号步数 801 圆迹阵列最大半径(m) 1.3 圆迹阵列最小半径(m) 0.8 目标位置 球1:(6.7, 0, 1.4) m 球2:(8.5, 0.9, 1.6) m 球3:(8.5, –0.8, 1.6) m 球4:(10.9, 0.5, 1.8) m 表 4 性能比较
Table 4. Performance comparison
实验模型 分辨率(m) PSLR(dB) ISLR(dB) CSAR 0.05 –8.325 –2.999 CASAR 0.05 –9.191 –6.433 -
[1] Devadithya S, Pedross-Engel A, Watts C M, et al. GPU-accelerated enhanced resolution 3-D SAR imaging with dynamic metamaterial antennas[J]. IEEE Transactions on Microwave Theory and Techniques, 2017, 65(12): 5096–5103. DOI: 10.1109/TMTT.2017.2766060 [2] Ren X Z, Qin Y, and Tian L J. Three-dimensional imaging algorithm for tomography SAR based on multiple signal classification[C]. Proceedings of 2014 IEEE International Conference on Signal Processing, Communications and Computing, Guilin, China, 2014: 120–123. DOI: 10.1109/ICSPCC.2014.6986165. [3] Xie H T, Shi S Y, An D X, et al. Fast factorized backprojection algorithm for one-stationary bistatic spotlight circular SAR image formation[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2017, 10(4): 1494–1510. DOI: 10.1109/JSTARS.2016.2639580 [4] Chen L P, An D X, and Huang X T. A backprojection-based imaging for circular synthetic aperture radar[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2017, 10(8): 3547–3555. DOI: 10.1109/JSTARS.2017.2683497 [5] Liu X, Yu J, Zhao H, et al.. An along-track sparse imaging method for forward-looking array SAR[C]. Proceedings of IET International Radar Conference 2015, Hangzhou, China, 2015: 1–5. DOI: 10.1049/cp.2015.0996. [6] Shi J, Zhang X L, Yang J Y, et al. APC trajectory design for " one-active” linear-array three-dimensional imaging SAR[J]. IEEE Transactions on Geoscience and Remote Sensing, 2010, 48(3): 1470–1486. DOI: 10.1109/TGRS.2009.2031430 [7] Wei S J, Zhang X L, and Shi J. Spaceborne-airborne bistatic linear array SAR high resolution 3-D imaging based on sparsity exploiting[C]. Proceedings of the 2016 19th International Conference on Information Fusion, Heidelberg, Germany, 2016: 1518–1522. [8] Axelsson S R J. Beam characteristics of three-dimensional SAR in curved or random paths[J]. IEEE Transactions on Geoscience and Remote Sensing, 2004, 42(10): 2324–2334. DOI: 10.1109/TGRS.2004.834802 [9] Tang L, Zhu Y F, and Fu Q. Stepped frequency SAR imaging based on compensation in Doppler domain[C]. Proceedings of 2014 IEEE International Conference on Signal Processing, Communications and Computing, Guilin, China, 2014: 627–631. DOI: 10.1109/ICSPCC.2014.6986269. [10] Li Y C, Zhang L, Liu B C, et al. Stepped-frequency inverse synthetic aperture radar imaging based on adjacent pulse correlation integration and coherent processing[J]. IET Signal Processing, 2011, 5(7): 632–642. DOI: 10.1049/iet-spr.2009.0301 [11] Shi J, Zhang X L, Sun H, et al.. Explanation of synthetic aperture 3-D imaging technique via EFIE[C]. Proceedings of the 2011 3rd International Asia-Pacific Conference on Synthetic Aperture Radar, Seoul, South Korea, 2011: 1–4. -



 作者中心
作者中心 专家审稿
专家审稿 责编办公
责编办公 编辑办公
编辑办公
 下载:
下载: