作者中心
专家审稿
责编办公
编辑办公
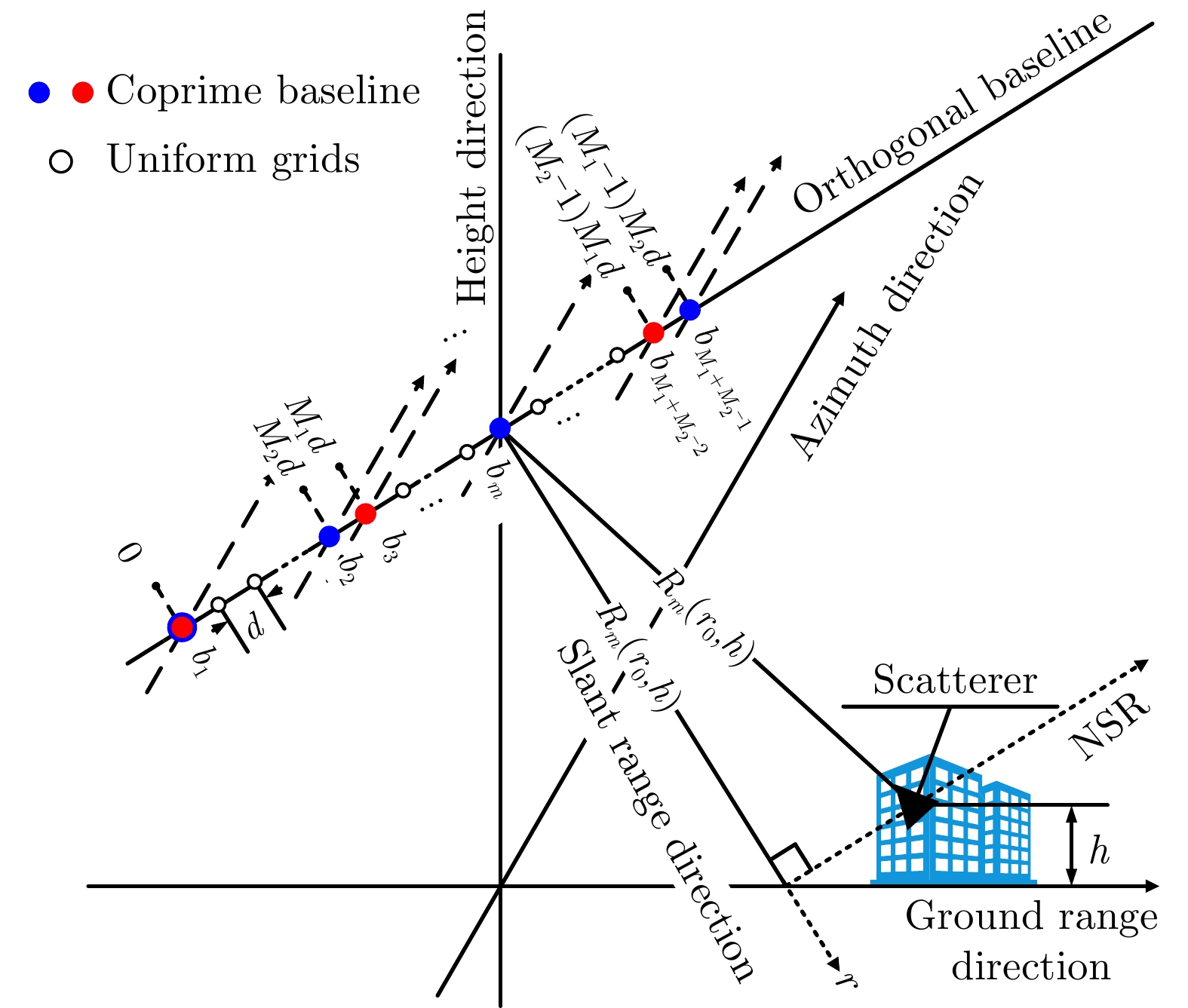
Estimation of the Minimum Number of Acquisitions for Coprime Tomographic Synthetic Aperture Radar
-
摘要: 在层析SAR技术的实际应用中，航过数量通常受高昂成本等因素的限制。互质层析SAR技术通过稀疏分布航过位置、延长基线孔径长度，可以降低所需的航过数量。当采用子空间方法开展互质层析SAR重构处理时，为了获得可靠的层析图，研究最少航过数量估计问题。考虑到子空间方法的重构性能受多个参数的影响，因此航过数量的选择必须综合考虑所有相关参数对重构结果的影响。为此，通过样本特征值分析方式建立子空间方法的可靠性保证条件。根据这个可靠性保证条件，提出了一种估计最少航过数量的方法。与传统的最少航过数量估计方法相比，所提方法的优势在于：同时考虑所有的相关参数，且具有解析的数学描述式。最后，仿真实验证实由所提方法估算的航过数量确实接近最小，且能够保证重构结果可靠。
-
关键词:
- 层析合成孔径雷达 /
- 互质阵列 /
- 最少航过数量 /
- 样本协方差矩阵的特征值 /
- 散射点数量估计
Abstract: In the practical application of Tomographic Synthetic Aperture Radar (TomoSAR), the number of acquisitions is usually restricted due to their expensive cost. A coprime TomoSAR technique was proposed to reduce the required number of acquisitions by sparsely distributing acquisitions and elongating baseline aperture. To guarantee a reliable tomogram, this study aims to determine the minimum number of acquisitions for the coprime TomoSAR when adopting a subspace method for performing the tomographic reconstruction. However, the performance of the subspace method depends on multiple parameters. In light of this, the selection of acquisition times has to comprehensively weigh the effects of all these parameters on the reconstruction performance. To this end, a prerequisite for reliable reconstruction is established by quantifying the relationship between the sample eigenvalues and all related parameters. Compared with conventional estimation approaches for the minimum number of acquisitions, the proposed approach has twofold advantages of containing all related parameters and of having a closed-form expression. Finally, the simulation experiments verify that the number of acquisitions estimated by our approach is close to the minimum and can guarantee reconstruction reliability. -
1. 引言
高分3号(GaoFen-3, GF-3)是我国第1颗自主研制的民用C波段多极化合成孔径雷达(Synthetic Aperture Radar, SAR)卫星，具有高分辨率、大成像幅宽、多成像模式、长寿命运行等特点，可有效改变我国民用高分辨率SAR图像依赖进口的现状。GF-3号卫星通过全天候、全天时监测全球海洋资源，提供高质量和高精度的稳定观测数据，对海洋强国建设具有重大意义。GF-3自2016年8月发射以来，逐步进行在轨测试定标，数据质量逐渐稳定完善，可提供长序列多模态SAR数据。后续我国将持续发射多颗民用多极化SAR卫星，与GF-3号进行组网观测，提高覆盖频率，为国家海域使用动态实时监测提供契机。
近些年，高分辨率SAR理论与应用得到长足发展，在海洋领域诸如海洋灾害溢油、绿潮等，海洋波浪反演，海上目标船只、海冰识别，海域使用动态监测等各方面都具有广泛的应用。海洋SAR卫星遥感影像因为存在随机波浪等不同海况的影响，相对于陆地SAR遥感影像，包含了大量非高斯的，非平稳的相干斑噪声，空间可视性较差，对海域使用动态监测提出难点。针对海岸线变化，经典阈值分割、边缘梯度算子等常规方法无法获得准确结果；而围填海变化也无法采用经典变化检测思路，因为每幅图像海水背景随海况不断发生变化，直接使用会将海况变化错误地分析为围填海变化；养殖浮筏会导致后向散射系数的增强，然而这种现象与海水表面所刮的风和表面自身的活动有关，引发这种变化可能是涌浪、内波、海洋水深测量等不同海况因素相互作用的结果[1,2]，因此难以从复杂的海水背景中精确识别海水养殖目标。
本文首先概述国家海域使用动态遥感监测，进而分析GF-3 SAR成像模式和标准化预处理，然后对海岸线围填海和海水浮筏养殖的监测工作进行总结和归纳，根据测试数据给出研究团队部分处理结果，最后对GF-3未来的应用发展方向做了展望。
2. 国家海域使用遥感动态监测
国家海域使用动态监测采用高、中、低分辨率遥感影像，通过遥感影像处理、遥感信息提取、地理信息系统等多种技术手段，编制海洋区域用海规划，实现对全国海水养殖、海岸线、围填海和疑点疑区等海域使用要素进行动态监测，如图1所示，准确获得全国沿海用海现状分布情况，建立海域海岛动态遥感监测本底库，并及时更新到国家海域动态监视监测管理系统，保持全国海域海岛管理数据的现势性[3,4]。
从2006年开始，对全海域范围进行宏观低分辨率的卫星监测，对内水及领海海域进行中高分辨率的卫星监测，对近岸重点海域进行高分辨率的无人机遥感动态监视监测。10～30 m的低分辨率遥感数据包括环境、高分、中巴、Landsat等；2～5 m的高分辨率遥感数据包括高分、资源、遥感系列、SPOT5/6/7、Rapideye等；优于1 m的高分切片数据包括 QuickBird、WorldView-1/2、GF2等。目前海域动态系统获取的原始遥感数据超过9000景。其中，低分辨率原始遥感数据1500余景，高分辨率原始遥感数据7500余景；高分切片数据20余万平方公里。低分辨率遥感数据按季度实现全国沿海全覆盖每年4次，高分辨率遥感数据实现全海域覆盖按年1次。
由于沿海地区常为多云阴雨天气，南方海域此特点更加明显，导致部分地区一年也无法完全覆盖，覆盖频率受到极大限制，高分辨率SAR 遥感影像不受天气条件影响，可有效解决这一难题。我国机载SAR研制较早，始于1976年，1979年9月获得的第1批SAR图像受脉冲压缩技术的限制，距离分辨率不高，只有180 m，方位分辨率30 m[5]。星载SAR系统主要有我国于2012年11月发射的HJ-1-C卫星，是我国首颗S波段小型合成孔径雷达卫星，主要用于环境监测与灾害预警，2016年8月10日，我国首颗C波段全极化SAR卫星在太原卫星发射中心升空，可全天时全天候监测海洋陆地信息，为国家海域建设提供极大数据支持. 实现海域使用开发利用活动实时科学管理[6]。
3. 高分三号海域使用监测模式
GF-3号提供新型C波段SAR遥感数据，具有多种成像模式，包括聚束、条带、扫描、波浪、全球观测、高低入射角等12种成像模式，最高分辨率可达1 m，其参数属性信息如表1所示。
表 1 GF-3卫星SAR数据属性信息Table 1. Attribute information of SAR data on GF-3序号 成像模式 分辨率(m) 成像幅宽(km) 入射角范围(°) 极化方式 标称 方位向 距离向 标称 范围 1 聚束 1 1.0～1.5 0.9～2.5 10×10 10×10 20～50 可选单极化 2 超精细条带 3 3 2.5～5.0 30 30 20～50 可选单极化 3 精细条带Ⅰ 5 5 4～6 50 50 19～50 可选双极化 4 精细条带Ⅱ 10 10 8～12 100 95～110 19～50 可选双极化 5 标准条带 25 25 15～30 130 95～150 17～50 可选双极化 6 窄幅扫描Ⅰ 50 50～60 30～60 300 300 17～50 可选双极化 7 窄幅扫描Ⅱ 100 100 50～110 500 500 17～50 可选双极化 8 全极化条带Ⅰ 8 8 6～9 30 20～35 20～41 全极化 9 全极化条带Ⅱ 25 25 15～30 40 35～50 20～38 全极化 10 波成像模式 10 10 8～12 5×5 5×5 20～41 全极化 11 全球观测成像模式 500 500 350～700 650 650 17～53 可选双极化 12 扩展入射角 低入射角 25 25 15～30 130 120～150 10～20 可选双极化 高入射角 25 25 20～30 80 70～90 50～60 可选双极化 基于国家海域使用遥感动态监测精度要求，通常选用空间分辨率优于5 m的影像作为监测数据，将GF-3号聚束模式、精细条带模式和全极化条带Ⅰ模式数据作为备选数据，截止2017年8月，全国近岸海域数据覆盖情况如图2所示。此外，GF-3可提供全极化模式SAR遥感影像，可以有效描述目标的电磁散射特性(能量特性、相位特性和极化特性)，其中极化特性可以更容易反映海水背景和海域使用目标的表面粗糙度差异、强二面角散射等其它雷达参数不能提供的信息，更利于表征海域使用目标特性。
海域使用动态监测需要严格精确的预处理操作，像控点误差必须在1个像素点之内。高分三号卫星数据通过国家卫星海洋应用中心分发，到本地后需要进行模运算、投影变换、系统几何校正，水平/垂直镜像、像控点几何精校正，陆地掩膜等相关预处理，除此以外，还需要进行SAR数据特有的多视处理、相干斑降噪等处理，通过上述处理操作完成遥感数据标准预处理，形成海域使用信息提取条件。
4. GF-3 SAR海岸线围填海变化监测
在全球范围内围填海主要分布于4个区域，分别是东亚及东南亚沿岸(中国、日本、新加坡等)；波斯湾沿岸(迪拜、卡塔尔等)；欧洲沿岸(荷兰、德国、英国等)；美洲沿岸(美国东海岸、墨西哥湾沿岸等)。不同国家根据其围填海资源环境影响，实施围填海规划与管理。荷兰为了抗衡洪水、拓展生存空间，开展了近800年围填海活动，面积达到5200 km2；迪拜等波斯湾沿岸国家围填海都采用整体规划、仿自然生态设计等优美造型，因此在国内外开展围填海变化监测研究仍具有广泛的应用价值。
依据《海域使用分类体系》对围填海做出了明确定义：填海是指筑堤围割海域填成土地，并形成有效岸线的用海方式；围海是指通过筑堤或其他手段，以全部或部分闭合形式围割海域进行海洋开发的用海方式，两者统称为围填海。从定义可以得出，围填海变化信息提取的关键是海岸线有效识别，当获得精确海岸线之后，与历史基准遥感岸线相比对，即可获得围填海变化区域面积。围填海遥感监测研究最早起源于图像分割，常规图像分割的主要方法有：(1)阈值分割法，该方法通过直方图分割法或最大类间方差法确定阈值，将图像二值化从而区分目标和背景，该方法常用于目标和背景灰度值差较大的图像；(2)边缘检测法，该方法通过计算每个像元的梯度值构建边缘梯度算子，检测任一像元与邻近像元之间的差异，根据目标边缘处灰度值梯度的不连续性检测目标与背景之间的边界；(3)小波变换法，该方法先将图像信息转换为数字信号，通过小波变换对信号进行细化分析，识别信号中的奇异点，从而确定海岸线位置。(4)主动轮廓模型，该方法分为参数主动轮廓和几何主动轮廓两类，算法思想是通过最小化能量泛函将包括了目标在内的封闭曲线驱动到目标的边缘，实现目标边缘检测。
然而，海浪和悬浮泥沙等因素改变了海面对电磁波的反射能力，会在海洋SAR遥感影像上产生相干斑噪声。因此，对于海洋SAR遥感影像的处理，需要考虑海洋SAR遥感影像中相干斑噪声等因素的影响。国内学者如杨虎等[7]使用Sobel算子对对比度较低的SAR图像提取出了水边线信息，陆立明等[8]提出了基于方位向子孔径相关和RDD域散射能量特征相结合的海岸线提取方法并提取出海岸线，林维诗[9]采用基本水平集方法和基于区域特征的水平集方法进行边缘信息的有效提取，赵伟舟[10]设计了一种基于模糊集理论的SAR图像分割方法并取得良好的分割效果，李映等[11]提出并使用一种基于小波能量和邻域统计的无监督聚类方法进行SAR图像分割取得较好的分类结果，荆浩等[12]提出一种基于边缘特征海岸线检测方法、该算法能够在考虑海杂波的情况下实现岸线的高精度提取。
国外学者Kass[13]首先提出Snake主动轮廓模型，实现应用曲线逼近目标轮廓，之后Lee提出边界追踪算法实现了海岸线轮廓提取，Moctezuma[14]将Markovian分割法和多项式变换相结合将海洋SAR遥感影像降噪后提取出岸线信息，Sethianhe和Osher等[15]将水平集(Level Set)方法引入主动轮廓模型并提出几何活动轮廓(Geometric Active Contour, GAC)模型。范剑超团队针对海洋SAR遥感影像中的不规则相干斑噪声，提出基于区域的距离正则化几何主动轮廓模型(Regional Distance Regularized Geometric Active Contour models, RDRGAC)，该算法构建区域面积项系数与等效视数(Equivalent Number of Looks, ENL)之间的联系，能够根据每景影像中的噪声水平进行参数的自动设定，实现岸线的高精度高速度自动提取。
随着GF-3卫星的成功发射，我国民用SAR数据质量得到有效提升，使得围填海信息提取成为可能。范剑超团队采用RDRGAC算法自动提取遥感影像中的海岸线，通过对遥感影像的解译，对比分析岸线变化，形成围填海专题图，通过外业现场验证其有效性，实现海域使用情况的动态监测，相应流程图如图3所示。针对海南省海口区域和辽宁大连金州湾区域，分别进行围填海信息自动提取，分别如图4和图5所示。
图 3 GF-3 SAR围填海动态监测流程图Figure 3. Dynamic monitoring process flow chart of reclamation in SAR image
图 4 海口地区SAR影像基础原始岸线提取Figure 4. The basic result of coastline extraction result of Haikou area
图 5 根据处理流程进行SAR围填海信息提取Figure 5. Coastline information extraction according to dynamic monitoring process为了海岸线提取结果的精确性，采用均方根误差(Root Mean Squared Error, RMSE)来计算海岸线提取精度，并将本文所提方法提取岸线与经过外业验证的手动提取岸线进行对比，计算公式如下：
RMSE=√n−1∑k=0[(xk−ˉxk)2+(yk−ˉyk)2]/n (1) 其中， xk,yk,ˉxk,ˉyk分别表示本文方法RDRGAC模型和手动提取岸线的横纵坐标，并随机抽查50个像素点，通过ArcGIS测量工具统计其准确率，统计结果如表2所示。
表 2 精度评价结果Table 2. The results of precision assessment区域 RMSE波动范围(像素数) 位置相同像素点 位置不同像素点 准确率 大连金州湾 0.5～1.5 46 4 92% 其中围填海信息提取算法测试结果与现场调查结果进行叠加比对结果如图5(g)所示，金州湾围填海用地类型绝大部分为建设填海用地，通过直接测量解译两者之间的差别为4个像素，2007年4月–2016年12月金州湾区域围填海面积为22.86 km2，专题图如图6所示。
5. GF-3 SAR海水浮筏养殖监测
我国浅海和滩涂资源丰富，海水养殖业繁荣，浮筏养殖是海域使用动态监测的重要类型，在国民经济发展中具有巨大作用。浮筏养殖是在浅海与潮间带设置浮动筏架，筏上挂养养殖对象的一种生产方式，多以浮球悬挂吊笼方式养殖扇贝、牡蛎等贝类。然而随着养殖规模的逐年扩大，一些贝类养殖海区出现贝类产量降低和死亡率增加的情况，贝类持续高密度地养殖增加了局部海域的环境压力[16]，造成生态环境污染。因此，系统深入地研究浮筏养殖用海面积范围提取，实现浮筏养殖动态监测，利于合理使用养殖资源，营造健康平衡的海水生态环境，具有十分重要的意义[17]。浮筏养殖范围面积过大，而且浮筏会随海洋动力情况发生漂移，给现场调查带来巨大的困难，传统可见光遥感影像主要表征目标的反射信号，而养殖浮筏在海上分布稀疏，导致浮筏养殖区域的混合像元无法完全准确反映[18]。
5.1 养殖浮筏极化散射机理分析
筏式养殖分为网箱养殖、延绳式养殖和浮筏养殖。网箱养殖链接成片，体积较大，当雷达波发射时，由于海水的回波能量较弱，而养殖用的基座、围栏和网箱等回波能量较强，色调比周围的海水更亮，二者对比度更大，所以在Radarsat-2影像中可以十分清晰地识别[19]。延绳式养殖用于养殖藻类，建立近海养殖区快速检测的光谱特征指数，可以通过多光谱波段线性组合实现其面积的有效识别[20]。由于养殖浮筏在水面上基本以浮球漂浮为主，相对网箱养殖体积小(直径30 cm)，即使是2 m高分辨率可见光影像也无法完全发现。此外，相对藻类，贝类与海水的光谱反射特性差异不强，所以波段运算无法实现浮筏养殖信息提取，如何有效地发现养殖浮筏成为研究的难点。
国外学者多集中于潮间带固定基或滩涂上底播贝类的识别，属于自然养殖。Van der Wal等[21]分析潮间带之间不同地物表面粗糙度和后向散射特性之间的关系。Lee等[22]采用AIRSAR和JERS-1 对固定基座贝类养殖进行不同位置的后向散射特征分析，获得不同拍摄方向不同基座结构的后向散射特性，从而发现HH极化更有利于发现滩涂上的贝类。Choe等[23]通过多频率Radarsat-2和ALOS PALSAR的极化SAR微波特征获得潮间带低潮期不同地物类型后向散射特征。目前，Gade等[24]在此研究基础之上，获得多时相SAR图像可以探测到潮间带贝类养殖和生态变化的结论，并展望多极化数据在贝类养殖物提取的可能性。国内浮筏养殖属于人工养殖，多采用浮球吊笼模式，较难直接发现，而且现有的浮筏养殖信息提取的研究资料较少，均为本课题组的研究成果。初佳兰等[25]对长海县贝类养殖进行遥感监测的初步研究，根据浮筏分布特性进行专家解译，获得养殖分布专题图。范剑超团队等[26]对北戴河邻近海域浮筏养殖区域进行2年长序列可见光和SAR遥感图像监测，发现Landsat-8、ZY-3和GF-1等可见光遥感影像均无法发现浮筏养殖区域，仅有极少数SAR影像可以识别养殖浮筏目标，浮筏养殖极化散射机理尚不清晰。
海水养殖均以浮球悬挂吊笼方式养殖为主，其主要要素分为浮球、吊笼、养殖物和海水海况，各要素对浮筏养殖的后向散射强度影响成因不同。浮球是白色或黑色橡胶材质，直径约为30 cm，浮球间距约为75 cm，吊笼直径约为20 cm，长度4～6 m，养殖物主要以扇贝、牡蛎等贝类为主，其材质、类型、空间分布均影响后向散射强度；海水海况影响表面粗糙度，导致后向散射强度发生变化。海上养殖现场结构和示意图如图7所示。
由于养殖浮筏在水面上基本以浮球漂浮为主，相对网箱养殖体积小(直径 30 cm)，即使是2 m高分辨率可见光影像也无法完全发现，如图8(a)所示。此外，对于SAR影像，如图8(b)所示，养殖浮筏与海水背景的后向散射差异不强，所以经典算法无法精确实现浮筏养殖信息提取，如何有效地发现养殖浮筏成为研究的难点。
以浮筏养殖物理实际为基础，讨论目标极化分解类型，以海水为界划分为海面部分与水下部分，直观可分解为6类不同散射，如图9所示。海面部分包括表面散射、二面角散射和螺旋体散射，海水表面随机粗糙度以布拉格散射为主，浮球亦以表面散射为主，随机海面和浮球可产生二面角散射和螺旋体散射；水下部分根据电磁波在海水的穿透性，无法产生直接作用，所以⑤和⑥散射不存在，水下部分通过间接改变海表粗糙度产生影响。
图 9 浮筏养殖的极化散射机制示意图(①海水表面散射，②浮球表面散射，③海水浮球二面角散射，④海水浮球螺旋体散射，⑤水下表面散射，⑥水下体散射)Figure 9. Schematic diagram of floating raft aquaculture scattering mechanism (① Scattering method of sea surface, ② Scattering method of floating raft, ③ Scattering method of dihedral caused by raft and sea surface, ④ Scattering method of helix structure, ⑤ Scattering method of surface under water, ⑥ Scattering method of body under water)采用GF3号全极化模式Ⅰ数据，对日本明海区域进行Nonlocal滤波，Yamaguchi极化分解、Freeman分解，H/A/Alpha分解、Krogager分解和伪彩色图合成，分解结果如图10所示。
图 10 日本明海区域海水养殖极化分解结果Figure 10. Polar decomposition of floating raft aquaculture in Japan从图10中可以发现在日本明海区域有十分规则的固定结构筏式养殖区域，形成十分典型的偶次散射区域，海表波浪以表面散射为主，陆上山地区域以体散射为主，城区部分为多种类型混合散射，GF-3号全极化SAR数据通过极化分解，可以非常有效地表征各类散射体，其中Yamaguchi更能明显区分出浮筏养殖区域与其他陆地海水背景区域。图11为典型中国区域浮筏养殖区域，以养殖牡蛎，扇贝为主，浮筏养殖结构示例如图7所示。采用Nonlocal滤波，Yamaguchi极化分解和伪彩色图合成，从图中可以看出海上浮筏养殖区域以表面散射和二次散射混合为主，与图9极化散射结构相同。
图 11 大连市浮筏养殖Yamaguchi极化分解结果Figure 11. Yamaguchi polar decomposition of floating raft aquaculture in Dalian5.2 养殖浮筏信息提取
近些年，极化SAR图像的识别分类得到了广泛地研究，主要可以分为非监督和有监督两大类方法[27]。非监督方法仅仅根据遥感数据自身特性，无需人工辅助实现最终聚类，更适合于大范围目标识别[28,29]。有监督分类方法不需要任何假设条件，针对精细化分类，可以获得较高的精度[30]。
5.2.1 非监督浮筏养殖监测
非监督算法对遥感图像聚类多引入空间域特征分布的方法，假设邻近区域属于同一类的概率较高，可解决孤岛效应和噪声干扰[31]。Wu等[32]采用Wishart 分布描述数据的协方差矩阵，实现基于区域的全极化SAR图像聚类。Yu等[33]采用带有边界惩罚项的区域增长方法进行SAR图像分割。上述两种方法属于空间域的图像分类方法。范剑超等[34]利用部分先验知识，提出单点逼近型初始聚类中心选择方法，得到不同属性的权重，解决同谱异物的问题，进而提出两阶段模糊聚类[35]，提高SAR图像处理效率。相对陆地SAR图像，海洋SAR遥感图像因为存在海面大量不规则波浪等不同海况，单一特征严重受到相干斑噪声的影响，因此，需要考虑引入更多图像特征用于目标识别。纹理特征[36]、灰度共生矩阵[37]、Gabor变换特征和小波变换特征[38]广泛应用于SAR图像目标识别的特征提取过程中，可以获得较好的尺度和方向性的后向散射特征。Cunha等[39]提出一种非下采样轮廓波变换(NonSubsampled Contourlet Transform, NSCT)具有平移不变性的特点，能够很好地提取图像的边缘轮廓信息。焦李成等[40]根据SAR数据的上下文空间统计信息进行非监督分层迭代聚类，将特征域和空间域方法结合，获得了较好的效果。徐新等[41]对SAR图像进行过分割，再进行图像区域级和像素级的特征提取，得到用于表示图像的特征向量，再采用隐马尔科夫模型聚类。如上所述，多源特征集成提高SAR图像目标提取精度已经成为目前研究的热点，然而多集中于特征选择阶段或同类型特征合成，如何将不同类型的特征有效融入统一的框架下进行学习却鲜有报道。此外，传统聚类方法仅对球状分布的目标样本数据具有较好的聚类效果，然而在实际SAR图像处理过程中通常无法提前获知浮筏养殖分布类型。近些年，Chen等[42]在此基础上提出多核框架，将不同统计分布的数据在一个分类器中进行计算，取得较好的结果。Huang等[43]将多核函数与经典带有空间约束或目标函数带有惩罚项的核方法进行比较，发现经典聚类方式是多核框架的一种特殊形式。
选用GF-3全极化模式数据，选取5个研究区域，将L1A级产品转换为L2级，进行图像水平镜像，几何校正和图像滤波等相应预处理。并根据浮筏分布情况建立数据切片，GF-3影像和数据切片情况如图12所示。针对区域1和区域2进行无监督模糊聚类，将单极化特征、Yamaguchi极化特征和H/A/Alpha极化特征进行聚类分析，结果分别如图13和图14所示，聚类结果整体精度如表3所示，Yamaguchi极化特征相对单极化数据可以获得更好的聚类结果，而H/A/Alpha极化特征结果较差，因此获得更适合海水养殖目标提取极化特征十分重要。
表 3 精度评价结果Table 3. The results of precision assessment区域 单极化聚类结果(%) Yamaguchi极化特征聚类结果(%) H/A/Alpha极化特征聚类结果(%) 区域1 71.33 78.69 62.28 区域2 71.54 79.17 61.31 5.2.2 有监督深度学习网络浮筏养殖监测
深度学习在人工智能研究中表现出优异的效果，其具有在大规模数据上有效的特征提取与表达能力，在遥感图像分类识别任务中有很大的潜力。深度学习是一种特征学习方法，通过学习输入数据本身的结构来初始化网络参数，从而解决反向传播神经网络无法加深的问题，并获得更高层次的、更加抽象的数据表达[44–46]。对于遥感分类识别任务，高层次的语义表达能够提升输入数据的区分能力，并且削弱不相关因素的影响[47]。目前，国内外学者开展了基于深度学习算法的SAR图像分类及识别等研究，经典的深度网络结构如深度置信网络[48]、卷积神经网络[49]和堆叠自动编码器[47]都应用于SAR图像分类识别问题中。何楚等[50]提出一种基于软概率的池化方法，结合多层反卷积网络，学习目标的高层结构特征，并将其用于SAR图像分类。陈渤等[51]提出一种相似性约束的受限玻尔兹曼机模型，提高了SAR图像目标识别的精度。Jiao等[52]提出Wishart深度堆叠网络进行极化SAR图像分类，将Wishart距离用于隐含层映射中，加快了计算速度并提高了分类精度。Gong等[53]采用受限玻尔兹曼机对多时相SAR图像训练学习，实现变化检测。Qin等[54]提出基于受限玻尔兹曼机的集成分类模型，适合于样本有效条件下的分类。Jiao等[55]提出判别式深度置信网络，学习SAR图像高层次信息，取得优异的分类效果。本课题组也开展了基于深度学习神经网络的单极化SAR图像分类的研究，提出了一种深度卷积编码网络[56]进行SAR图像特征提取并分类，所提模型具有特征自主学习的能力并能抑制相干斑噪声干扰，取得了优异的分类结果；提出了一种基于监督收缩编码器的深度网络[57]，对SAR图像初始特征进行优化，通过加入对编码函数惩罚的收缩项来增强局部不变性，通过加入样本标签的监督项来引入高层语义信息，进一步提高了SAR图像分类精度。同时，本课题组提出了一种深度协同稀疏编码网络进行养殖浮筏目标识别[58]，将超像素分割后的SAR图像像素点对应的特征输入到所提模型进行联合优化，使得同个超像素块的像素特征更趋于相似并平滑掉相干斑噪声，取得了较好的养殖浮筏识别效果。
针对上述讨论和提高识别率的考虑，结合对深度学习网络的讨论，提出利用深度学习作为核心的有监督浮筏养殖信息提取算法。该算法将超像素分割后的SAR图像像素点对应的特征输入到所提及模型进行联合优化，使得同个超像素模块的像素特征更趋于相似并平滑掉相干斑噪声，根据SAR遥感影像的自身特点，通过训练样本进行学习，实现不同类型目标的分类，结果如图15–图17所示。
为了对比分析非监督算法和有监督算法的优缺点，采用8 m空间分辨率全极化模式和5 m空间分辨率超精细条带模式GF-3 SAR数据进行整图处理，分别如图18和图19所示。无监督算法仅根据数据特性进行识别，不需要人工选取学习样本，对于图18和图19，可以实现分钟级识别效率，而对于深度学习网络需要小时数量级处理，但是识别精度受海况影响较大，如HH极化右下区域，浮筏养殖目标信息和海水背景混杂，识别精度会受到影响。而有监督深度学习方法对不同养殖分布状态、都具有更好的识别效果。针对GF-3长序列数据，可以提供不同海况下浮筏养殖目标的后向散射状态，增加深度学习网络训练样本的丰富性，从而对于海洋背景变化较大的情况下，均可以获得较好监测精度。
图 18 极化浮筏养殖识别结果(全极化模式Ⅰ)Figure 18. Floating raft recognition result under full polarmetric mode Ⅰ6. 结论和展望
随着SAR卫星的不断发射，覆盖频率大幅提高，GF-3 号SAR数据将在国家海域使用动态监测中发挥重大作用。本文对国家海域使用遥感动态监测和高分三号监测模式进行论述，重点对海岸线围填海变化监测、海水浮筏养殖极化散射机理、非监督/有监督信息提取算法进行了详细讨论，不同类型信息提取算法可根据实际需求发挥各自优势，从部分研究结果可以发现GF-3不同模式数据均可以实现海域使用信息的有效提取。
综合当前国内外技术发展和应用水平，可以开展GF-3号 SAR遥感影像在围填海用地类型分类方面的应用，包括养殖区、盐地、岸滩等。进而开展GF-3号全极化SAR数据分析研究，利用不同极化特征对围填海不同用地类型进行识别分类，实现GF-3号多时相围填海变化监测，自动提取围填海信息。
此外，针对GF-3号可以提供长序列监测数据的优势，可以有效获取不同海况下海上目标的后向散射特征，使得对于需要海量多样的学习样本进行训练的复杂分类器，例如深度学习网络、迁移学习等，得到有效的学习，充分提升网络泛化能力，实现不同海域不同海况海上目标的精确监测，具有广泛的科学应用价值。
-
图 2 本文提出的最少航过数量估计方法的流程图
Figure 2. The flow chart for the propose approach to estimate the minimum number of acquisitions
图 3 基于样本特征值检测方法的正确检测概率随航过数量的变化曲线(K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
Figure 3. Successful detection rate varies with the number of acquisitions (K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
图 4 高度估计的RMSE随航过数量的变化(K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
Figure 4. The RMSE of the height estimation varies with the number of acquisitions (K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
图 5 基于样本特征值的信号检测方法的正确检测概率随高度间隔的变化曲线(K=2; SNR1=0 dB, SNR2=10 dB; ρh=1 m)
Figure 5. Successful detection rate varies with the height separation (K=2; SNR1=0 dB, SNR2=10 dB; ρh=1 m)
图 6 高度估计的RMSE随高度间隔的变化曲线(K=2; SNR1=0 dB, SNR2=10 dB; ρh=1 m)
Figure 6. The RMSE of the height estimation varies with the height separation (K=2; SNR1=0 dB, SNR2=10 dB; ρh=1 m)
图 7 基于样本特征值检测方法的正确检测概率随航过数量的变化曲线(Δh=1 m; L=20；所有散射点信噪比均等于0 dB)
Figure 7. Successful detection rate varies with the number of acquisitions (Δh=1 m; L=20; all the SNRs=0 dB)
图 8 高度估计的RMSE随航过数量的变化曲线(Δh=1 m; L=20；所有散射点信噪比均等于0 dB)
Figure 8. The RMSE of the height estimation varies with the number of acquisitions (Δh=1 m; L=20; all the SNRs=0 dB)
图 9 基于样本特征值检测方法的正确检测概率随高度间隔的变化曲线(L=20；所有散射点信噪比均等于0 dB；ρh=1 m)
Figure 9. Successful detection rate varies with the height separation (L=20; all the SNRs=0 dB; ρh=1 m)
图 10 高度估计的RMSE随高度间隔的变化曲线(L=20；所有散射点信噪比均等于0 dB；ρh=1 m)
Figure 10. The RMSE of the height estimation varies with the height separation (L=20; all the SNRs=0 dB; ρh=1 m)
图 11 最少航过数量与信噪比之间的约束关系(K=2; Δh=1 m; L=10)
Figure 11. The constraint relationship between the minimum number of acquisitions and the SNR (K=2; Δh=1 m; L=10)
图 12 |<a1, a2>|/M随航过数量的变化(d=7.5 m; K=2; Δh=1 m)
Figure 12. |<a1, a2>|/M varies with the number of acquisitions (d=7.5 m; K=2; Δh=1 m)
图 13 高度估计RMSE随航过数量和信噪比的相变图(K=2; Δh=1 m; L=10)
Figure 13. The phase transition of the RMSE as a function of the number of acquisitions and the SNR (K=2; Δh=1 m; L=10)
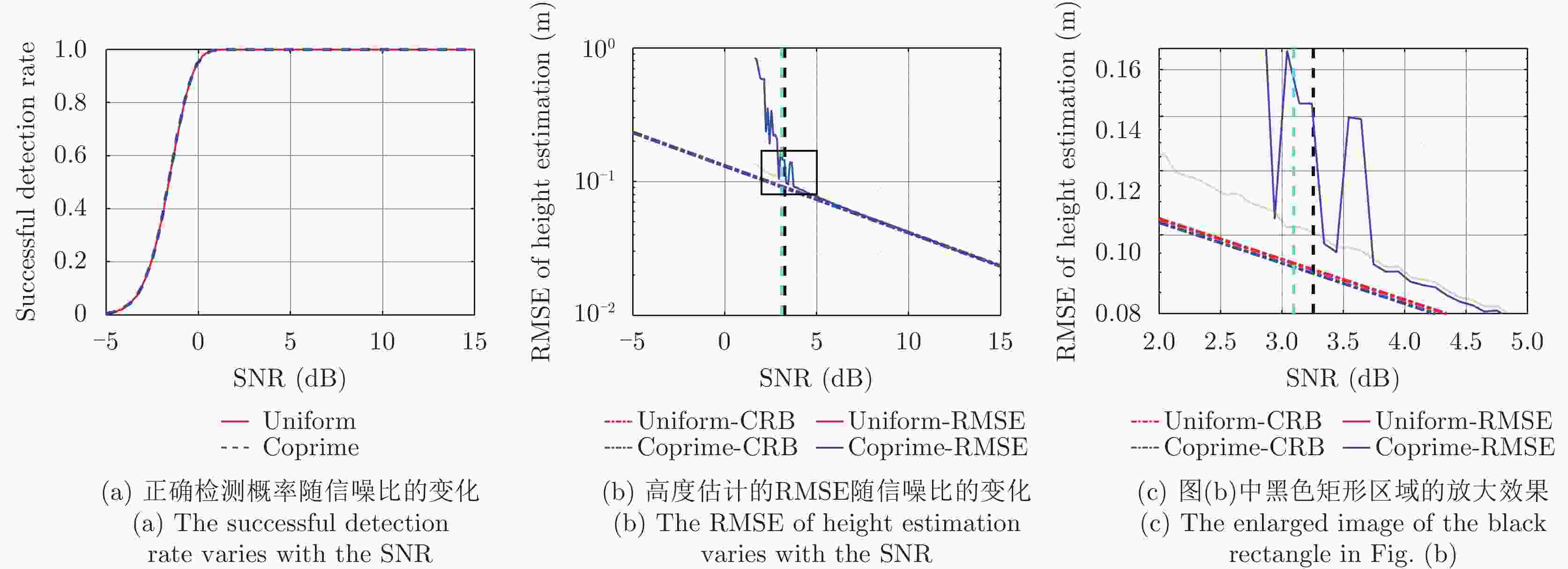
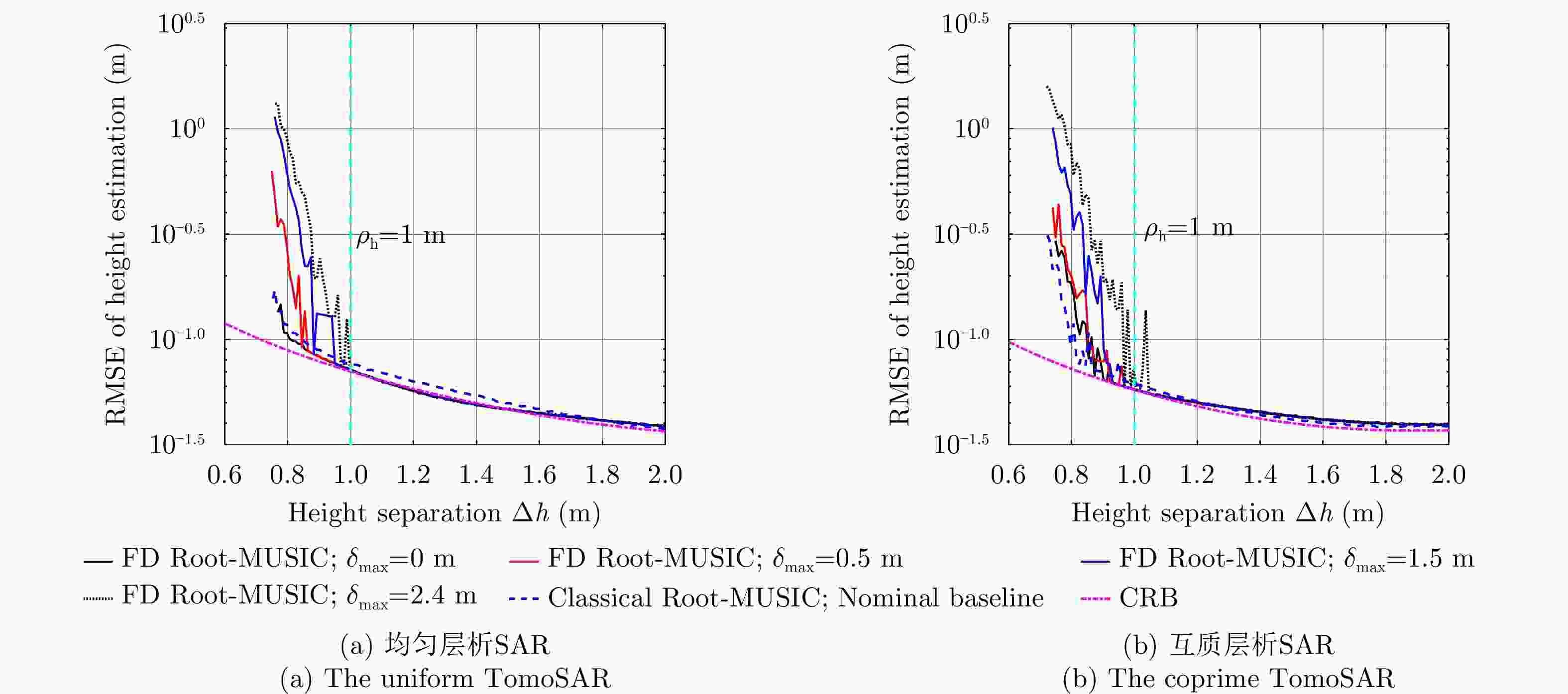
图 14 由文献[9]方法设计的均匀层析SAR和互质层析SAR的重构结果[均匀层析SAR (M=16; d=7.5 m; B=112 m)；互质层析SAR (M1=9, M2=8; d=1.8 m; B=112 m)]
Figure 14. The reconstruction results from the uniform TomoSAR and the coprime TomoSAR which are designed by Ref. [9] vary with the SNR [the uniform TomoSAR (M=16; d=7.5 m; B=112 m); the coprime TomoSAR (M1=9, M2=8; d=1.8 m; B=112 m)]
图 15 存在基线偏差情况下和理想情况下的正确检测概率(K=2；所有散射点的信噪比均等于0 dB；L=20；ρh=1 m)
Figure 15. Successful detection rate calculated from the deviated tracks and the nominal tracks (K=2; all the SNRs=0 dB; L=20; ρh=1 m)
图 16 存在基线偏差情况下和理想情况下的高度估计的RMSE(实验参数的设置与图 15中的参数相等)
Figure 16. RMSE of the height estimation calculated from the deviated tracks and the nominal tracks (the simulation parmeters are seted as the same as in Fig. 15)
表 1 由本文方法计算得到的最少航过数量和最小航过间隔(K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
Table 1. The minimum number of acquisitions and the minimum inter-acquisition spacing calculated by the proposed approach (K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
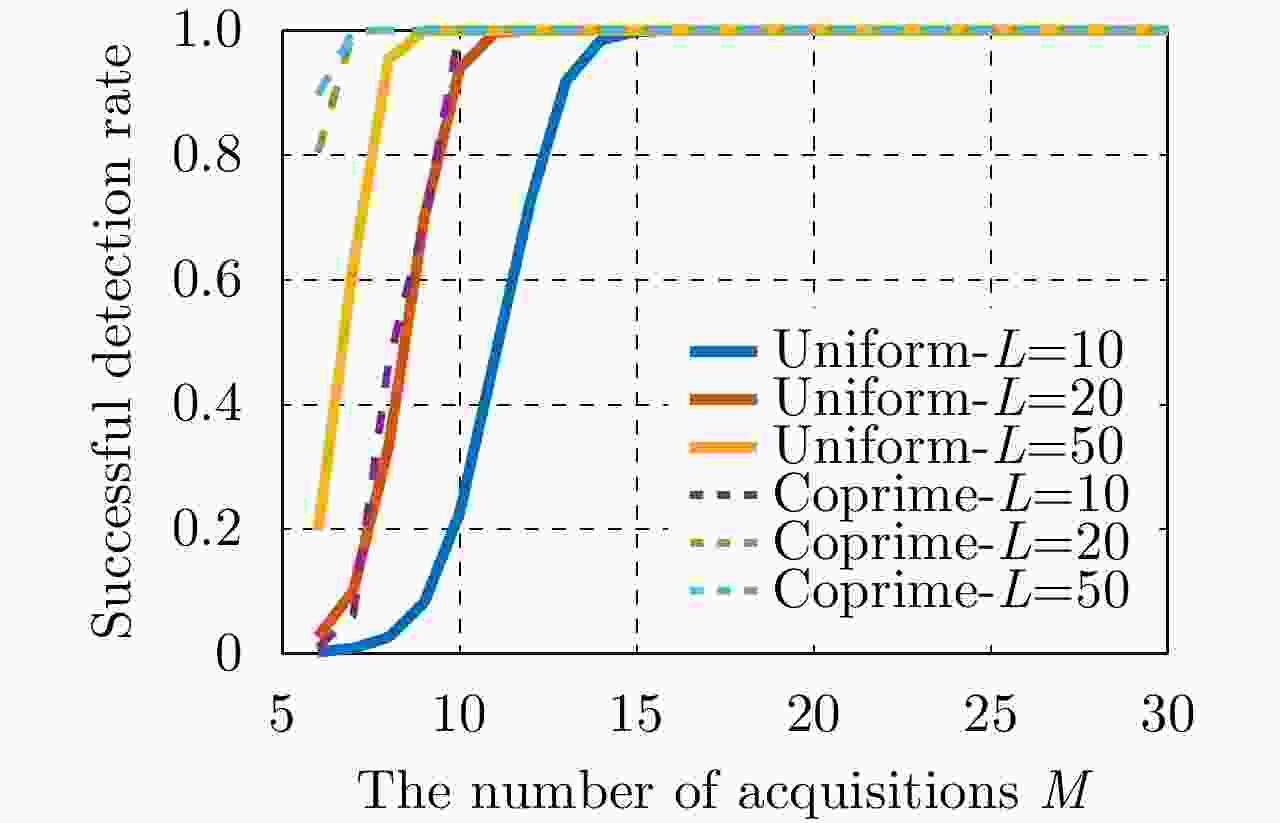
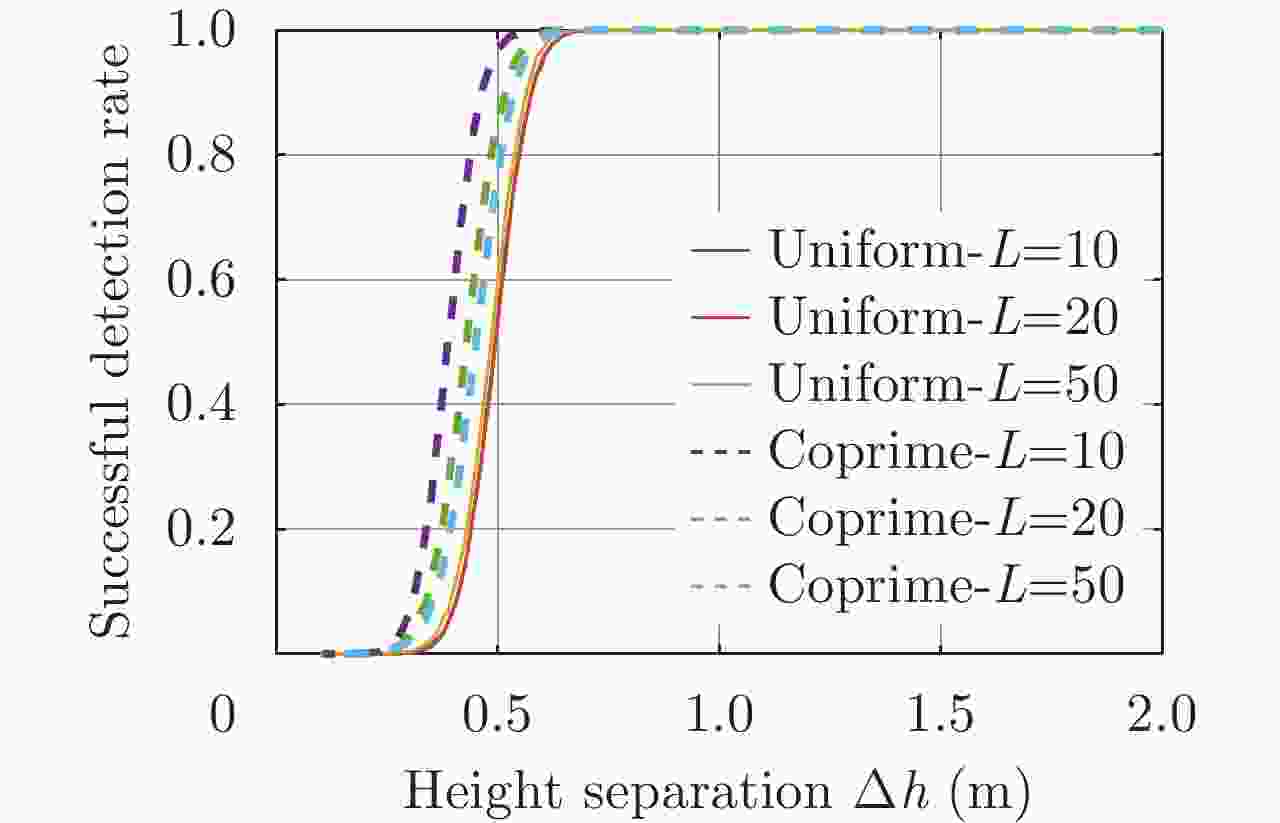
视数 均匀层析SAR 互质层析SAR L=10 Mmin=20; dM=7.0 m Mmin=13;dM=4.6 m L=20 Mmin=15; dM=7.3 m Mmin=9; dM=7.3 m L=50 Mmin=12; dM=7.0 m Mmin=8; dM=5.5 m 
下载: 导出CSV
表 2 基于样本特征值检测方法所需的最少航过数量的理论估计值和实测估计值(K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
Table 2. The theoretical estimate and the measured estimate of the minimum number of acquisitions in the sense of the sample-eigenvalue-based detection (K=2; SNR1=0 dB, SNR2=10 dB; Δh=1 m)
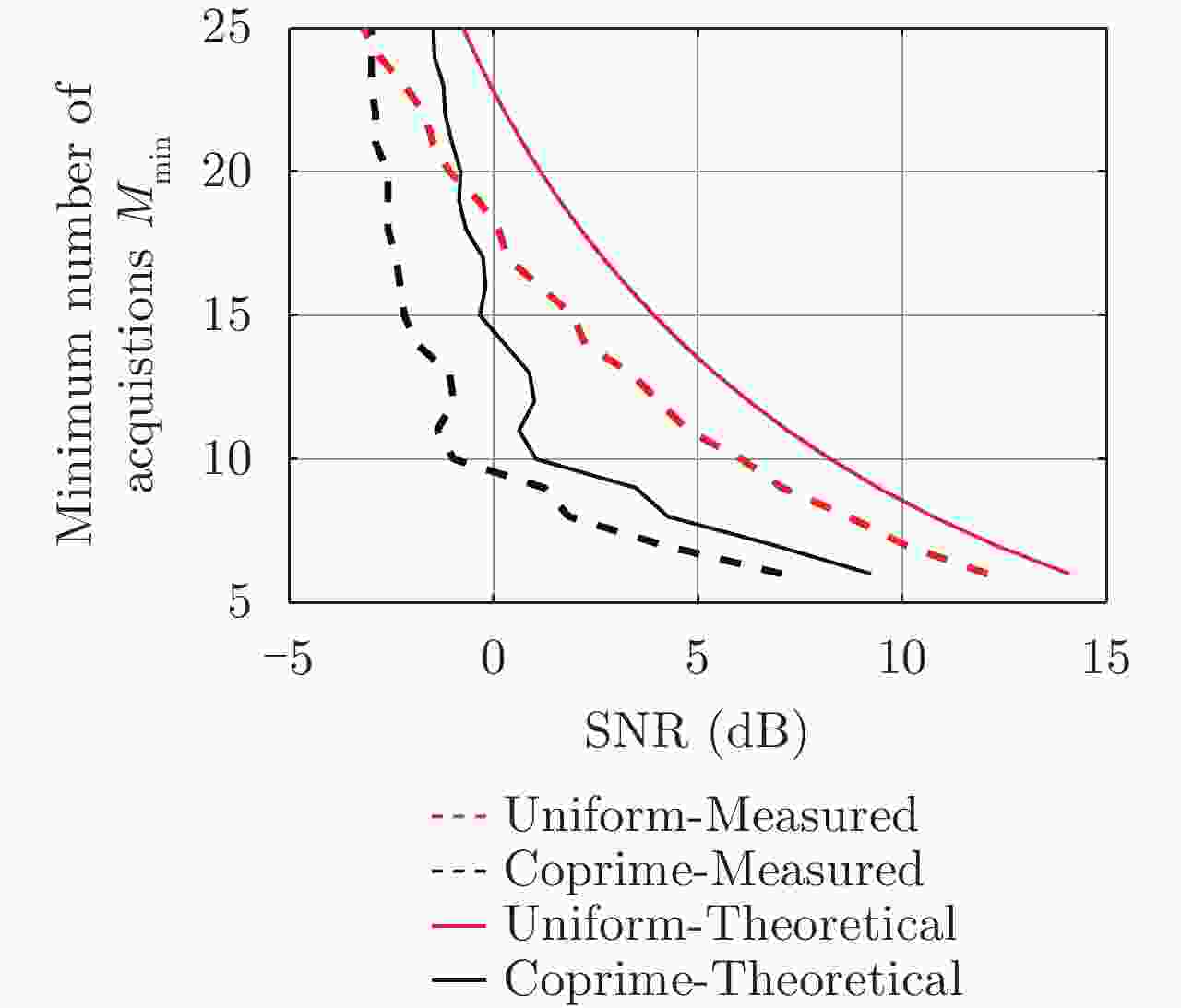
视数 均匀层析SAR 互质层析SAR 理论值 实测值 理论值 实测值 L=10 20 16 13 11 L=20 15 13 9 8 L=50 12 10 8 7
下载: 导出CSV
表 3 基于样本特征值检测方法的实测分辨率(K=2; SNR1=0 dB, SNR2=10 dB; ρh=1 m)
Table 3. The measured tomographic resolution of the sample-eigenvalue-based detection (K=2; SNR1=0 dB, SNR2=10 dB; ρh=1 m)
实验参数 分辨的实测值(m) 瑞利分辨率(m) L=10；均匀层析SAR
(Mmin=20, dM=7.0 m)0.73 1.69 L=10；互质层析SAR
(Mmin=13; dM=4.6 m)0.64 1.22 L=20；均匀层析SAR
(Mmin=15; dM=7.3 m)0.73 2.19 L=20；互质层析SAR
(Mmin=9; dM=7.3 m)0.69 1.71 L=50；均匀层析SAR
(Mmin=12; dM=7.0 m)0.70 2.91 L=50；互质层析SAR
(Mmin=8; dM=5.5 m)0.69 2.55
下载: 导出CSV
表 4 本文方法计算所得的最少航过数量和最小航过间隔(Δh=1 m; L=20；所有散射点信噪比均等于0 dB)
Table 4. The minimum number of acquisitions and the minimum inter-acquisition spacing calculated by the proposed approach (Δh=1 m; L=20; all the SNRs=0 dB)
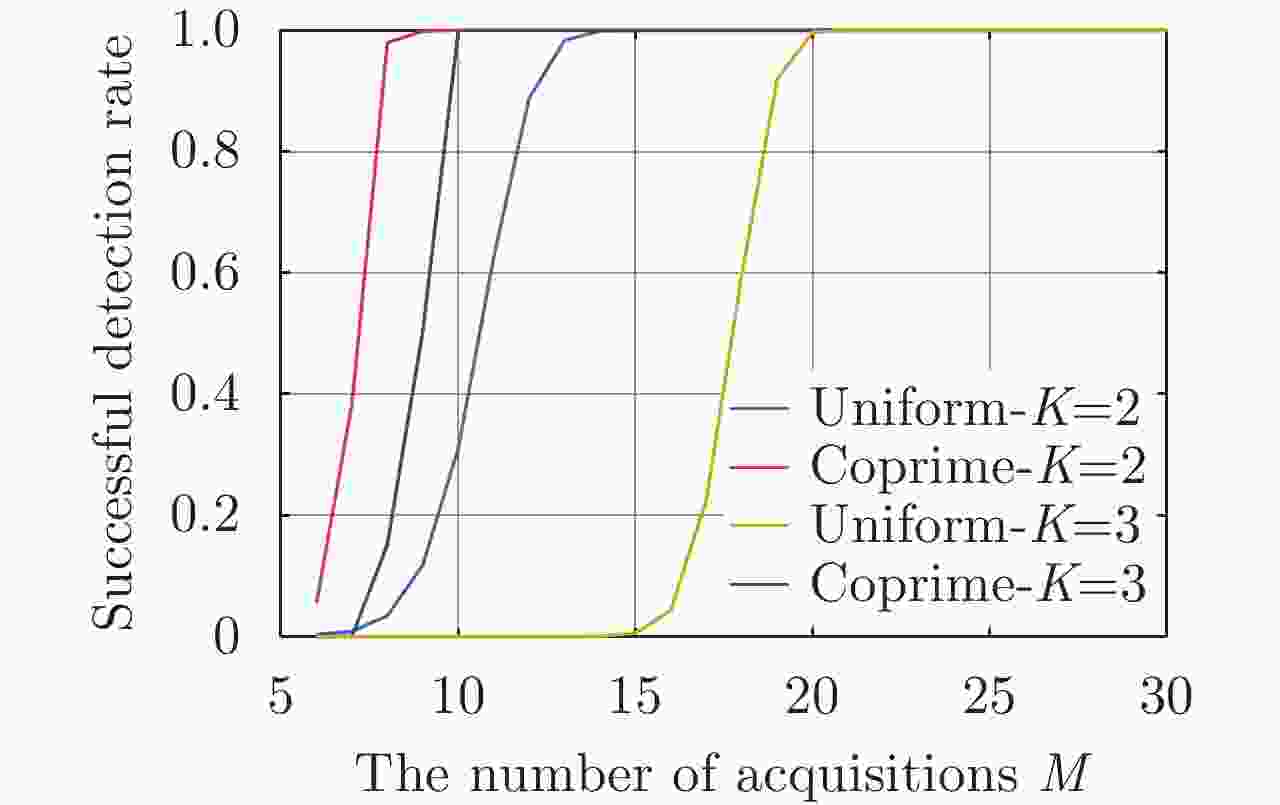
散射点数量 均匀层析SAR 互质层析SAR K=2 Mmin=18; dM=7.4 m Mmin=10; dM=6.1 m K=3 Mmin=23; dM=7.2 m Mmin=10; dM=7.1 m
下载: 导出CSV
表 5 基于样本特征值检测方法所需的最少航过数量的理论估计值和实测估计值(Δh=1 m; L=20；所有散射点信噪比均等于0 dB)
Table 5. The theoretical estimate and the measured estimate of the minimum number of acquisitions in the sense of the sample-eigenvalue-based detection (Δh=1 m; L=20; all the SNRs=0 dB)
视数 均匀层析SAR 互质层析SAR 理论值 实测值 理论值 实测值 K=2 18 15 10 10 K=3 23 21 10 10
下载: 导出CSV
表 6 基于样本特征值检测方法的实测分辨率(L=20；所有散射点信噪比均等于0 dB；ρh=1 m)
Table 6. The measured tomographic resolution of the sample-eigenvalue-based detection (L=20; all the SNRs=0 dB; ρh=1 m)
实验参数 分辨的实测值(m) 瑞利分辨率(m) K=2；均匀层析SAR
(Mmin=18;dM=7.4 m)0.75 1.78 K=2；互质层析SAR
(Mmin=10; dM=6.1 m)0.72 1.47 K=3；均匀层析SAR
(Mmin=23; dM=7.2 m)0.90 1.42 K=3；互质层析SAR
(Mmin=10; dM=7.1 m)0.88 1.26
下载: 导出CSV
-
[1] RAMBOUR C, BUDILLON A, JOHNSY A C, et al. From interferometric to tomographic SAR: A review of synthetic aperture radar tomography-processing techniques for scatterer unmixing in urban areas[J]. IEEE Geoscience and Remote Sensing Magazine, 2020, 8(2): 6–29. doi: 10.1109/MGRS.2019.2957215 [2] REIGBER A and MOREIRA A. First demonstration of airborne SAR tomography using multibaseline L-band data[J]. IEEE Transactions on Geoscience and Remote Sensing, 2000, 38(5): 2142–2152. doi: 10.1109/36.868873 [3] YU Longlong, HUANG Xiaotao, FENG Dong, et al. Coprime synthetic aperture radar tomography[C]. 2021 2nd China International SAR Symposium (CISS), Shanghai, China, 2021: 1–6. [4] YU Longlong, FENG Dong, WANG Jian, et al. An efficient reconstruction approach based on atomic norm minimization for coprime tomographic SAR[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4503705. doi: 10.1109/LGRS.2022.3143662 [5] VAIDYANATHAN P P and PAL P. Sparse sensing with Co-prime samplers and arrays[J]. IEEE Transactions on Signal Processing, 2011, 59(2): 573–586. doi: 10.1109/TSP.2010.2089682 [6] LOMBARDINI F and PARDINI M. 3-D SAR tomography: The multibaseline sector interpolation approach[J]. IEEE Geoscience and Remote Sensing Letters, 2008, 5(4): 630–634. doi: 10.1109/LGRS.2008.2001283 [7] NANNINI M, REIGBER A, and SCHEIBER R. A study on irregular baseline constellations in SAR tomography[C]. 8th European Conference on Synthetic Aperture Radar, Aachen, Germany, 2010: 1–4. [8] WEI Lianhuan, FENG Qiuyue, LIU Shanjun, et al. Minimum redundancy array—A baseline optimization strategy for urban SAR tomography[J]. Remote Sensing, 2020, 12(18): 3100. doi: 10.3390/rs12183100 [9] NANNINI M, SCHEIBER R, and MOREIRA A. Estimation of the minimum number of tracks for SAR tomography[J]. IEEE Transactions on Geoscience and Remote Sensing, 2009, 47(2): 531–543. doi: 10.1109/TGRS.2008.2007846 [10] ZHU Xiaoxiang and BAMLER R. Super-resolution power and robustness of compressive sensing for spectral estimation with application to spaceborne tomographic SAR[J]. IEEE Transactions on Geoscience and Remote Sensing, 2012, 50(1): 247–258. doi: 10.1109/TGRS.2011.2160183 [11] ZHU Xiaoxiang, GE Nan, and SHAHZAD M. Joint sparsity in SAR tomography for urban mapping[J]. IEEE Journal of Selected Topics in Signal Processing, 2015, 9(8): 1498–1509. doi: 10.1109/JSTSP.2015.2469646 [12] NADLER B. Finite sample approximation results for principal component analysis: A matrix perturbation approach[J]. The Annals of Statistics, 2008, 36(6): 2791–2817. doi: 10.1214/08-aos618 [13] NADAKUDITI R R and EDELMAN A. Sample eigenvalue based detection of high-dimensional signals in white noise using relatively few samples[J]. IEEE Transactions on Signal Processing, 2008, 56(7): 2625–2638. doi: 10.1109/TSP.2008.917356 [14] FORNARO G, VERDE S, REALE D, et al. CAESAR: An approach based on covariance matrix decomposition to improve multibaseline-multitemporal interferometric SAR processing[J]. IEEE Transactions on Geoscience and Remote Sensing, 2015, 53(4): 2050–2065. doi: 10.1109/TGRS.2014.2352853 [15] NAVNEET S, KIM J W, and LU Zhong. A new InSAR persistent scatterer selection technique using top eigenvalue of coherence matrix[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(4): 1969–1978. doi: 10.1109/TGRS.2017.2771386 [16] PAUCIULLO A, REALE D, FRANZÉ W, et al. Multi-look in GLRT-based detection of single and double persistent scatterers[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(9): 5125–5137. doi: 10.1109/TGRS.2018.2809538 [17] D’HONDT O, LÓPEZ-MARTÍNEZ C, GUILLASO S, et al. Nonlocal filtering applied to 3-D reconstruction of tomographic SAR data[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(1): 272–285. doi: 10.1109/TGRS.2017.2746420 [18] ZHU Xiaoxiang and BAMLER R. Superresolving SAR tomography for multidimensional imaging of urban areas: Compressive sensing-based TomoSAR inversion[J]. IEEE Signal Processing Magazine, 2014, 31(4): 51–58. doi: 10.1109/MSP.2014.2312098 [19] JOHNSON B A, ABRAMOVICH Y I, and MESTRE X. MUSIC, G-MUSIC, and maximum-likelihood performance breakdown[J]. IEEE Transactions on Signal Processing, 2008, 56(8): 3944–3958. doi: 10.1109/TSP.2008.921729 [20] SHAGHAGHI M and VOROBYOV S A. Subspace leakage analysis and improved DOA estimation with small sample size[J]. IEEE Transactions on Signal Processing, 2015, 63(12): 3251–3265. doi: 10.1109/TSP.2015.2422675 [21] FRIEDLANDER B. The root-MUSIC algorithm for direction finding with interpolated arrays[J]. Signal Processing, 1993, 30(1): 15–29. doi: 10.1016/0165-1684(93)90048-F [22] RUBSAMEN M and GERSHMAN A B. Direction-of-arrival estimation for nonuniform sensor arrays: From manifold separation to fourier domain MUSIC methods[J]. IEEE Transactions on Signal Processing, 2009, 57(2): 588–599. doi: 10.1109/TSP.2008.2008560 [23] STOICA P and NEHORAI A. MUSIC, maximum likelihood, and Cramer-Rao bound[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1989, 37(5): 720–741. doi: 10.1109/29.17564 期刊类型引用(19)
1. CHEN Xuewei，CHEN Jianping，WANG Genhou，ZHANG Qian，ZHENG Yanwei. Mining Subsidence Based on Integrated SBAS-InSAR and Unmanned Aerial Vehicles Technology. Journal of Ocean University of China. 2025(01): 113-129 . 
必应学术2. 向俞明，滕飞，王林徽，焦念刚，王峰，尤红建. 基于快速异源配准的高分辨率SAR影像海岛区域正射校正. 雷达学报. 2024(04): 866-884 . 
本站查看3. 袁新哲，叶小敏，刘亚东，赵良波，韩冰，仲利华，安文韬，崔利民. 高分三号卫星赋能海洋强国建设. 卫星应用. 2024(08): 12-19 . 百度学术4. 庾露. 基于SNAP通用架构的高分三号卫星数据转换方法研究. 计算机应用与软件. 2023(09): 19-24 . 百度学术5. 孙学娇，李俊杰，陈卫荣，严薇. 高分三号卫星助力海洋强国建设. 卫星应用. 2023(12): 20-27 . 百度学术6. 柳崇斌，徐佳，王冬梅，陈媛媛. 基于GF-3全极化SAR影像多特征优选的水产养殖塘提取. 农业工程学报. 2022(04): 206-214 . 百度学术7. 朱默研，侯景伟，孙诗琴，王彦卷. 基于深度学习的遥感影像识别国内研究进展. 测绘与空间地理信息. 2021(05): 67-73+85 . 百度学术8. 张艳梅，蒙印，黄国满，王萍，杨书成，张荞. 高分三号数据在地形图测制中的应用研究. 地理空间信息. 2020(01): 20-24+40+8 . 百度学术9. 邓云凯，禹卫东，张衡，王伟，刘大成，王宇. 未来星载SAR技术发展趋势. 雷达学报. 2020(01): 1-33 . 本站查看10. 李佳慧，王晓晨. 高分三号全极化SAR散射特性分析与精度评价. 中国激光. 2020(03): 275-282 . 百度学术11. 陈志新，聂娜. 高分三号数据在防城港海岛岸线监测的应用研究. 南方国土资源. 2020(08): 45-48+52 . 百度学术12. 杨泽楠，黄亮，王枭轩. 结合DEM的面向对象高分三号SAR影像高原山区水系提取. 昆明理工大学学报(自然科学版). 2019(01): 39-46 . 百度学术13. 吴元. 一种基于参数更新的机载SAR图像目标定位方法. 电子与信息学报. 2019(05): 1063-1068 . 百度学术14. 田东，禹卫东. 电离层色散效应对星载SAR成像的影响. 中国科学院大学学报. 2019(03): 376-384 . 百度学术15. 蔡宣宣，张永红，崔斌. 利用高分三号SAR影像进行双侧变化检测. 遥感信息. 2019(03): 62-69 . 百度学术16. 程前，王华斌，汪韬阳，李玉. 基于RFM模型的叠掩区域定位方法. 航天返回与遥感. 2019(05): 95-105 . 百度学术17. 罗格，卫征. 航天遥感与中国空间信息产业发展. 航天返回与遥感. 2018(04): 10-17 . 百度学术18. 陈淼焱，冯琦，刘克俭. 遥感技术在公安业务中的应用及展望. 公安学研究. 2018(03): 64-78+124 . 百度学术19. 田东，禹卫东，李宁，邹航. 高分辨率机载SAR多子带合成误差补偿方法. 雷达科学与技术. 2018(06): 589-595 . 百度学术其他类型引用(26)
-
下载:
必应学术
计量
- 文章访问数: 713
- HTML全文浏览量: 394
- PDF下载量: 92
- 被引次数: 45
