作者中心
专家审稿
责编办公
编辑办公
Multicarrier Waveform Optimization Method for an Intelligent Reflecting Surface-assisted Dual-function Radar-communication System
-
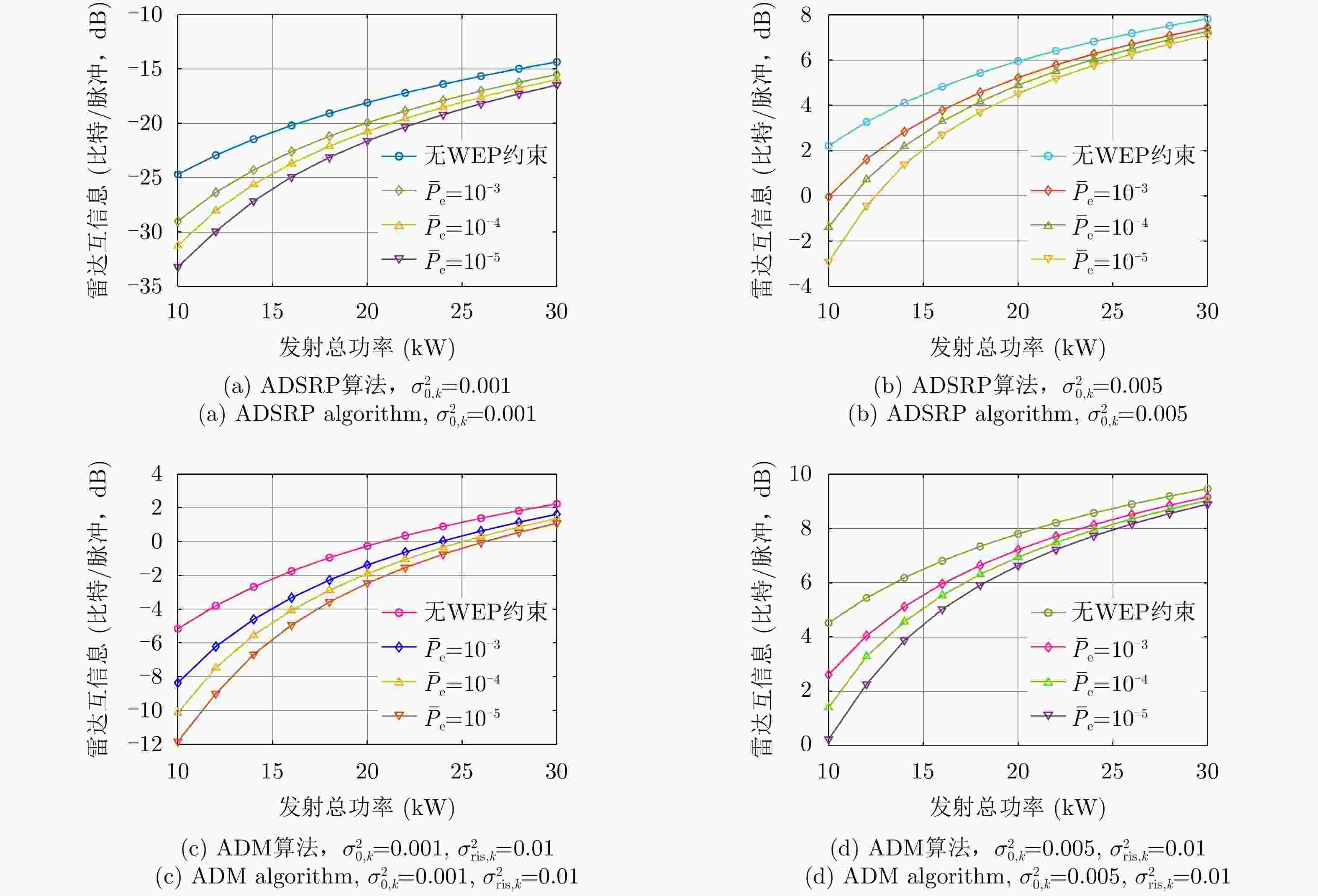
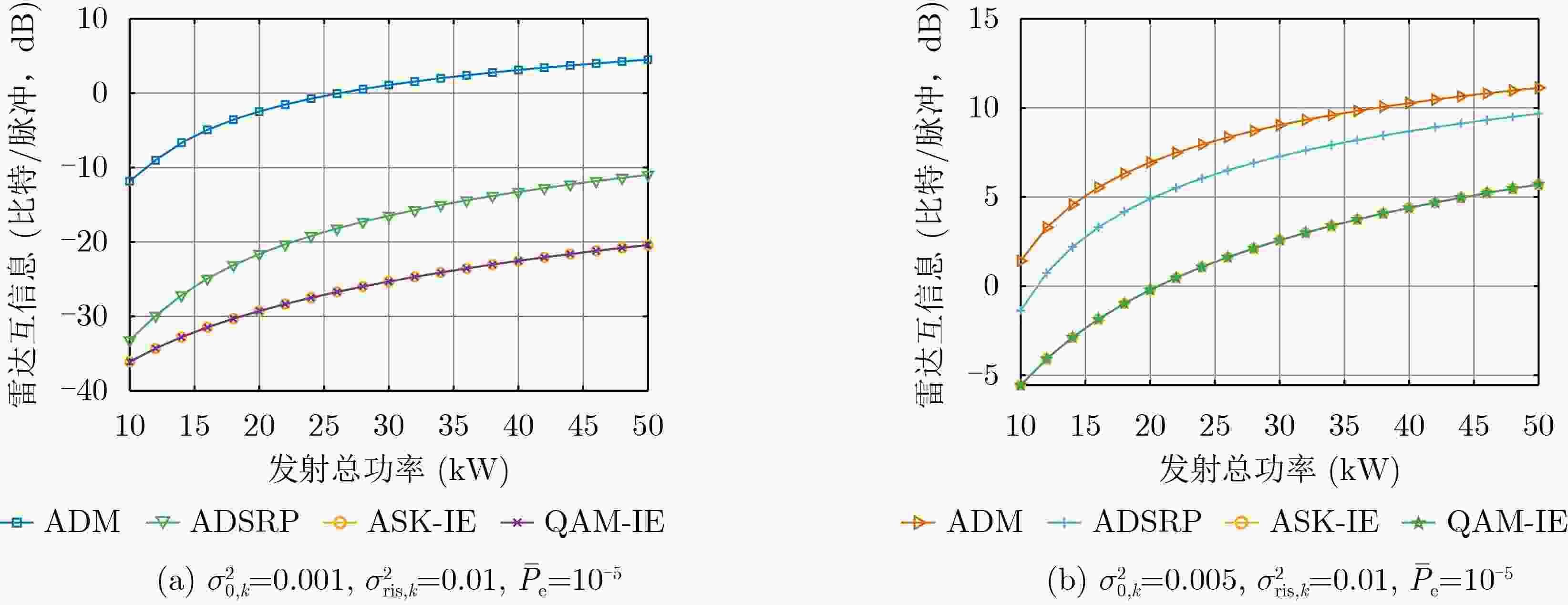
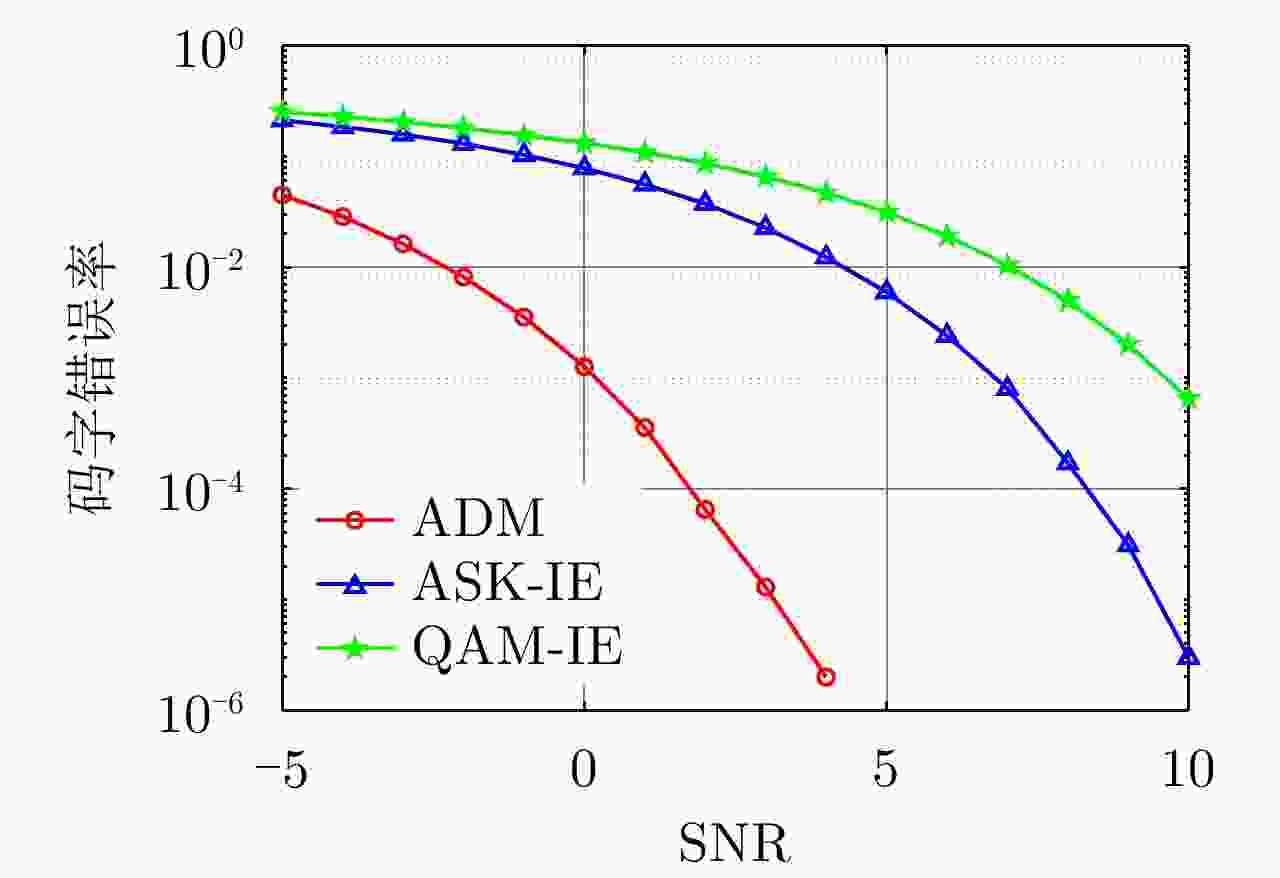
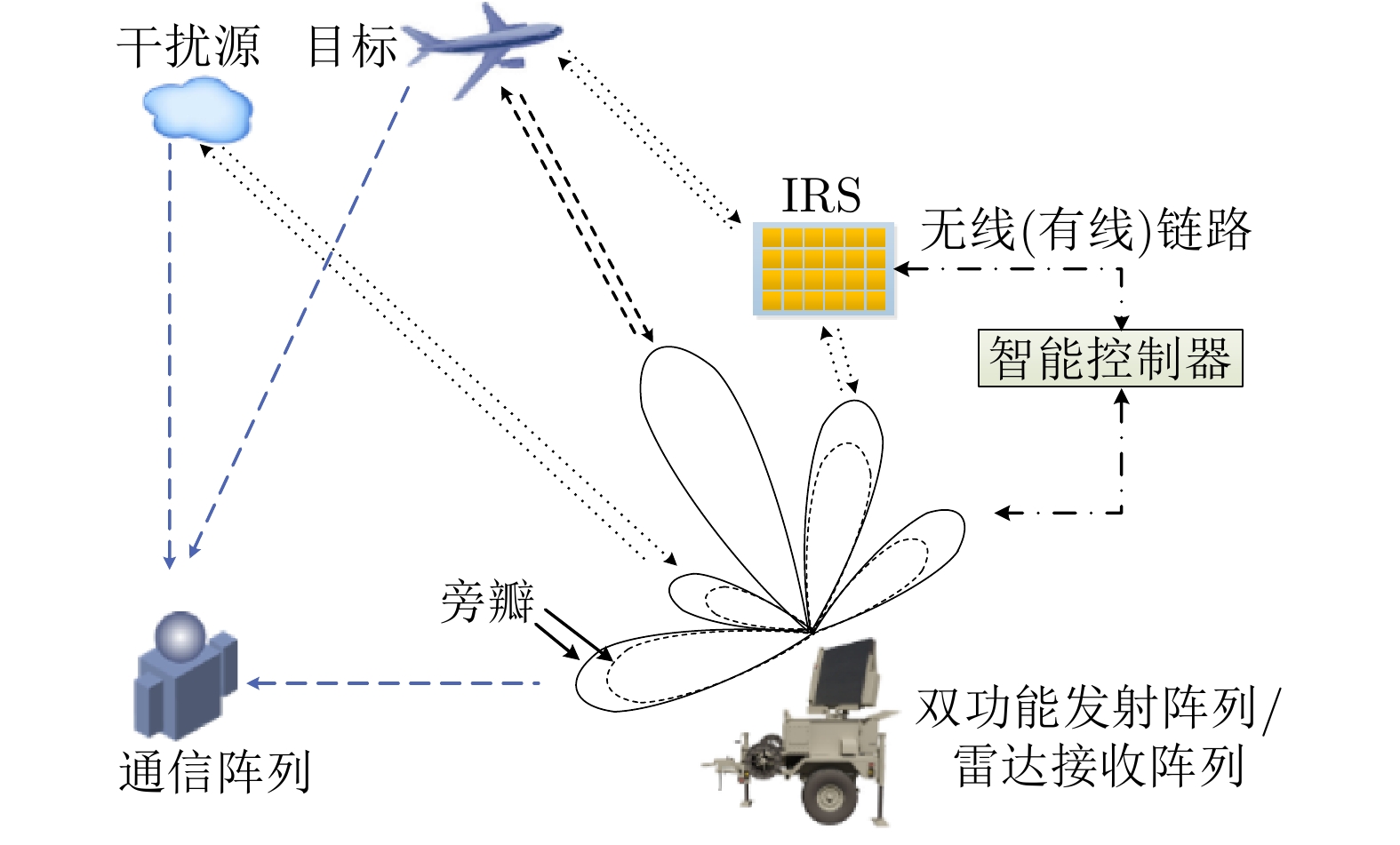
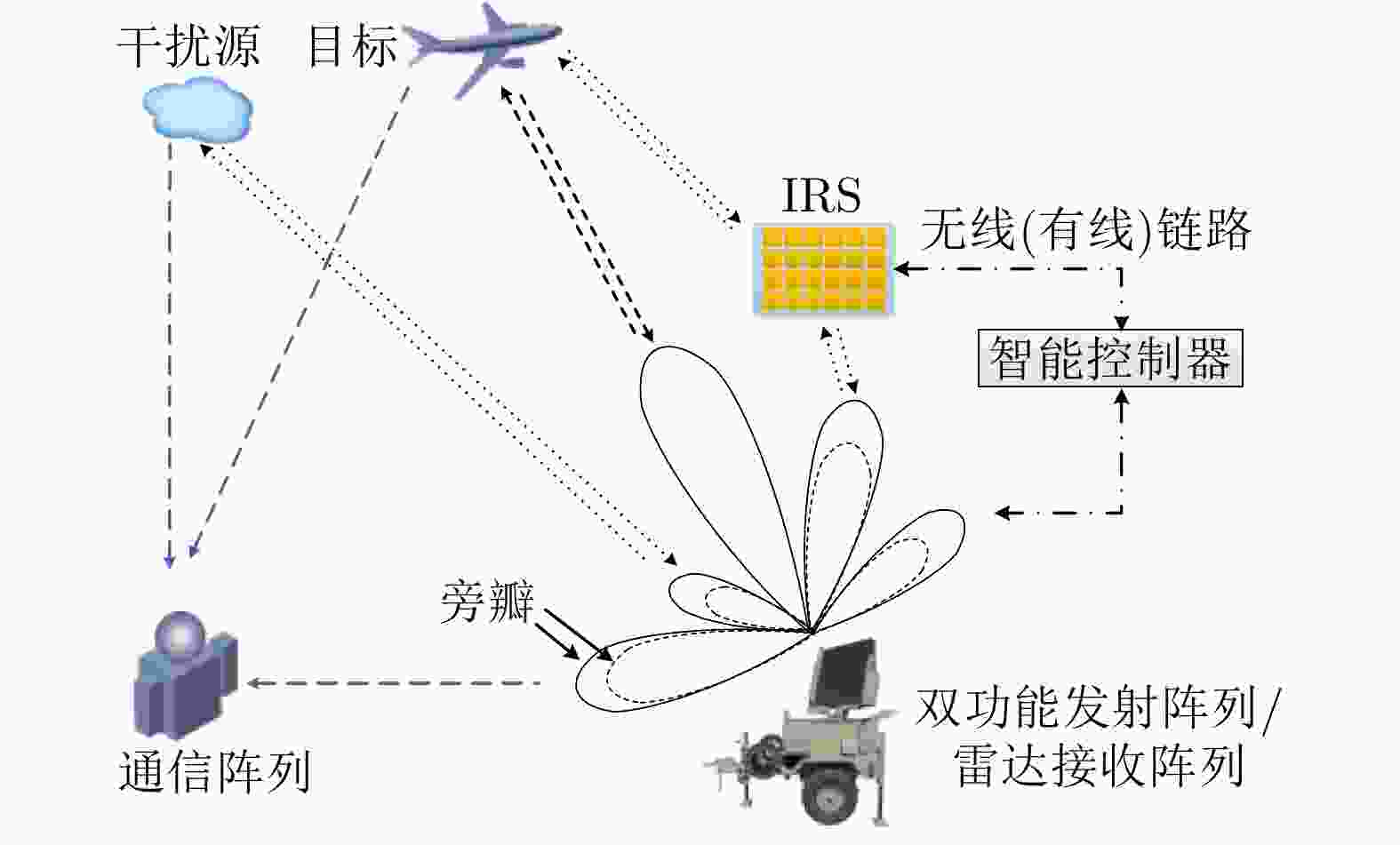
摘要: 雷达通信一体化是解决频谱资源拥挤问题的有效途径之一，而共享波形设计是同时实现雷达与通信功能的关键技术，该文旨在解决智能反射面(IRS)辅助雷达通信双功能(DRC)系统的多载波波形优化问题。首先，通过最大化传输功率、通信码字错误率(WEP)、旁瓣幅度与IRS反射系数约束下的雷达互信息(RMI)，构建了双功能发射波形、IRS反射单元、雷达与通信接收波束联合优化模型。其次，提出了基于交替方向最大化(ADM)的多载波波形优化算法，通过将原非凸优化问题分解为若干低复杂度子问题并迭代优化，获得了多载波波形功率分配策略的局部最优解。最后，仿真结果表明，ADM算法能同时实现雷达与通信功能；相较于现有方法有效提升了IRS辅助DRC系统的雷达与通信性能。Abstract: Radar-communication integration is an effective way to solve the congestion problem of spectrum resource. Sharing waveform design is the key technology that realizes the radar and communication functions simultaneously. This study solves the multicarrier waveform optimization problem for an Intelligent Reflecting Surface (IRS)-assisted Dual-function Radar-Communication (DRC) system. First, by maximizing Radar Mutual Information (RMI) along with the constraints of transmission power, Word Error Probability (WEP), sidelobe amplitude and IRS reflection coefficient, a joint optimization model with dual-functional transmit waveform, IRS reflecting units, radar and communication receiving beampattern is constructed. Second, a multicarrier waveform optimization algorithm based on Alternating Direction Maximization (ADM) is proposed. The original non-convex optimization problem is decomposed into several low-complexity subproblems and then iteratively optimized to obtain the local power allocation strategy of the multicarrier waveform. Finally, the simulation results show that the radar and communication functions can be simultaneously realized using the ADM algorithm. For the IRS-assisted DRC system, both the radar and communication performances can be effectively improved compared with those of the existing methods.
-
图 2 I-DRC多载波波形设计的仿真场景
Figure 2. Simulation scenario of multi-carrier waveform design for I-DRC
图 3 通信方位角旁瓣幅度分别为–10 dB和–20 dB的发射波束
Figure 3. Transmit beampatterns with sidelobe amplitudes of –10 dB and –20 dB, respectively, towards the communication direction
图 6 I-DRC多载波波形功率分配方案(
${{{P}}_{\text{t}}}{\text{ = 12}}\;{\text{kW}}$ )Figure 6. Power allocation scheme of multi-carrier waveform for I-DRC (
${{{P}}_{\text{t}}}{\text{ = 12}}\;{\text{kW}}$ )
图 7 不同WEP要求下RMI随着发射总功率变化曲线图
Figure 7. Curve of relationship between RMI and total transmit power under different WEP requirements
图 8 ADM, ADSRP, ASK-IE和QAM-IE算法RMI性能比较
Figure 8. RMI Performance comparison for ADM, ADSRP, ASK-IE and QAM-IE algorithms
图 9 WEP随着信噪比变化曲线图(
$ L' = 4 $ )Figure 9. Curve of relationship between WEP and SNR (
$ L' = 4 $ )表 1 基于ADM的多载波波形优化算法
Table 1. ADM based multicarrier waveform optimization method
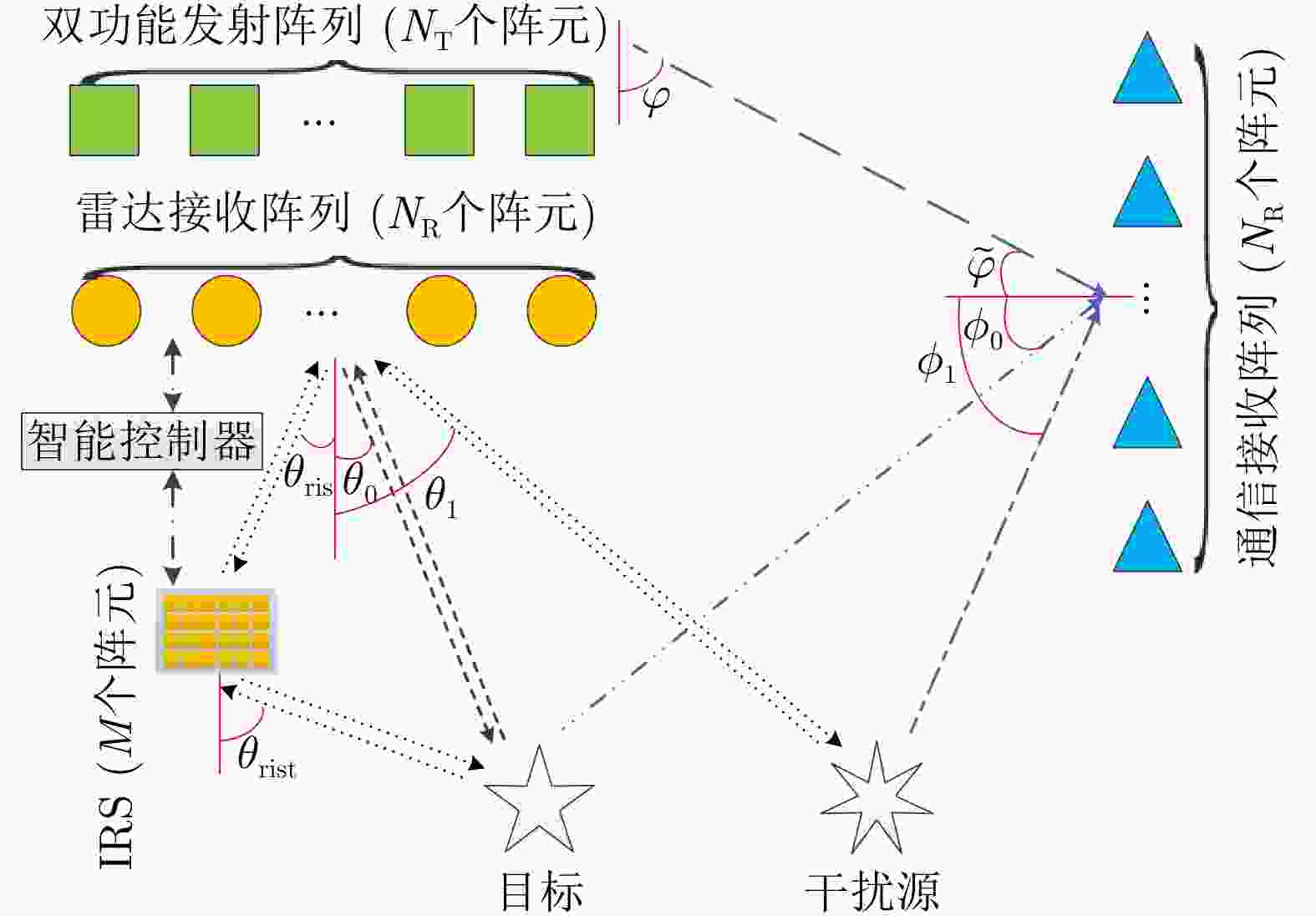
1 输入：发射和接收阵元数$ {N_{\text{T}}} $和$ {N_{\text{R}}} $；IRS阵元数$ M $；方位角$ {\theta _0} $, $ {\theta _1} $, $ {\theta _{{\text{ris}}}} $, $ {\theta _{{\text{rist}}}} $, $ \varphi $, $ \tilde \varphi $, $ {\phi _0} $和$ {\phi _1} $；子载波数$ K $；
传输总功率${ {{P} }_{\text{t} } }$；信道系数方差$ \sigma _{{\text{r}},k}^2 $, $ \sigma _{{\text{c}},k}^2 $, $ \sigma _{0,k}^2 $, $ \sigma _{1,k}^2 $, $ \sigma _{{\text{ris}},k}^2 $, $ \sigma _{{\text{rist}},k}^2 $, $ \sigma _{\beta ,0,k}^2 $, $ \sigma _{\beta ,1,k}^2 $和$ \sigma _{\gamma ,k}^2 $；旁瓣幅度数$ L $；停止准则$ \varepsilon $。2 输出：双功能发射波束形成矢量$ {{\boldsymbol u}_k} $；接收波束形成矢量$ {{\boldsymbol w}_k} $；RMI。 3 初始化：$ {\boldsymbol u}_k^0 $, $ {{\boldsymbol Q}^0} $, $ {\boldsymbol v}_k^0 $和$ {\boldsymbol w}_k^0 $；迭代索引$ j $。 4 求解松弛优化问题(16)获得$ {\boldsymbol u}_k^j $； 5 求解半正定凸问题(25)获得$ {{\boldsymbol Q}^j} $； 6 根据式(29)获得$ {\boldsymbol v}_k^j $； 7 根据式(32)获得$ {\boldsymbol w}_k^j $； 8 判断式(10)的收敛条件是否满足；若满足，停止迭代；反之，$ j = j + 1 $，并转向步骤4。 
下载: 导出CSV
-
[1] 刘凡, 袁伟杰, 原进宏, 等. 雷达通信频谱共享及一体化: 综述与展望[J]. 雷达学报, 2021, 10(3): 467–484. doi: 10.12000/JR20113LIU Fan, YUAN Weijie, YUAN Jinhong, et al. Radar-communication spectrum sharing and integration: Overview and prospect[J]. Journal of Radars, 2021, 10(3): 467–484. doi: 10.12000/JR20113 [2] CAA. Public sector spectrum release programme: Radar planning and spectrum sharing in the 2.7～2.9 GHz bands[EB/OL]. https://www.caa.co.uk/commercial-industry/airspace/communication-navigation-and-surveillance/spectrum. [3] TAVIK G C, HILTERBRICK C L, EVINS J B, et al. The advanced multifunction RF concept[J]. IEEE Transactions on Microwave Theory and Techniques, 2005, 53(3): 1009–1020. doi: 10.1109/TMTT.2005.843485 [4] MAZUMDER S, DURAND J P, MEYER S L, et al. High-band digital preprocessor (HBDP) for the AMRFC test-bed[J]. IEEE Transactions on Microwave Theory and Techniques, 2005, 53(3): 1065–1071. doi: 10.1109/TMTT.2005.843511 [5] PAUL B, CHIRIYATH A R, and BLISS D W. Survey of RF communications and sensing convergence research[J]. IEEE Access, 2017, 5: 252–270. doi: 10.1109/ACCESS.2016.2639038 [6] OZKAPTAN C D, EKICI E, and ALTINTAS O. Adaptive waveform design for communication-enabled automotive radars[J]. IEEE Transactions on Wireless Communications, 2021. doi: 10.1109/TWC.2021.3125924. [7] MA Dingyou, SHLEZINGER N, HUANG Tianyao, et al. Joint radar-communication strategies for autonomous vehicles: Combining two key automotive technologies[J]. IEEE Signal Processing Magazine, 2020, 37(4): 85–97. doi: 10.1109/MSP.2020.2983832 [8] QUAN Siji, QIAN Weiping, GUO Junhai, et al. Radar-communication integration: An overview[C]. The 7th IEEE/International Conference on Advanced Infocomm Technology (ICAIT), Fuzhou, China, 2014: 98–103. doi: 10.1109/ICAIT.2014.7019537. [9] 刘永军. 基于OFDM的雷达通信一体化设计方法研究[D]. [博士论文], 西安电子科技大学, 2019.LIU Yongjun. Study on integrated radar and communications design method based on OFDM[D]. [Ph. D. dissertation], Xidian University, 2019. [10] CAGER R, LAFLAME D, and PARODE L. Orbiter Ku-band integrated radar and communications subsystem[J]. IEEE Transactions on Communications, 1978, 26(11): 1604–1619. doi: 10.1109/TCOM.1978.1094004 [11] HAN Liang and WU Ke. 24-GHz integrated radio and radar system capable of time-agile wireless communication and sensing[J]. IEEE Transactions on Microwave Theory and Techniques, 2012, 60(3): 619–631. doi: 10.1109/TMTT.2011.2179552 [12] HAN Liang and WU Ke. Multifunctional transceiver for future intelligent transportation systems[J]. IEEE Transactions on Microwave Theory and Techniques, 2011, 59(7): 1879–1892. doi: 10.1109/TMTT.2011.2138156 [13] MOGHADDASI J and WU Ke. Multifunctional transceiver for future radar sensing and radio communicating data-fusion platform[J]. IEEE Access, 2016, 4: 818–838. doi: 10.1109/ACCESS.2016.2530979 [14] MISHRA A K and INGGS M. FOPEN capabilities of commensal radars based on whitespace communication systems[C]. 2014 IEEE International Conference on Electronics, Computing and Communication Technologies, Bangalore, India, 2014: 1–5. doi: 10.1109/CONECCT.2014.6740313. [15] WINKLER V and DETLEFSEN J. Automotive 24 GHz pulse radar extended by a DQPSK communication channel[C]. The 4th European Radar Conference, Munich, Germany, 2007: 138–141. doi: 10.1109/EURAD.2007.4404956. [16] SURENDER S C, NARAYANAN R M, and DAS C R. Performance analysis of communications & radar coexistence in a covert UWB OSA system[C]. 2010 IEEE Global Telecommunications Conference, Miami, USA, 2010: 1–5. doi: 10.1109/GLOCOM.2010.5683837. [17] 李晓柏, 杨瑞娟, 程伟. 基于频率调制的多载波Chirp信号雷达通信一体化研究[J]. 电子与信息学报, 2013, 35(2): 406–412. doi: 10.3724/SP.J.1146.2012.00567LI Xiaobai, YANG Ruijuan, and CHENG Wei. Integrated radar and communication based on multicarrier frequency modulation chirp signal[J]. Journal of Electronics &Information Technology, 2013, 35(2): 406–412. doi: 10.3724/SP.J.1146.2012.00567 [18] TAKASE H and SHINRIKI M. A dual-use radar and communication system with complete complementary codes[C]. 2014 15th International Radar Symposium, Gdansk, Poland, 2014: 1–4. doi: 10.1109/IRS.2014.6869268. [19] LI Xiaobai, YANG Ruijuan, ZHANG Zunquan, et al. Research of constructing method of complete complementary sequence in integrated radar and communication[C]. 2012 IEEE 11th International Conference on Signal Processing, Beijing, China, 2012: 1729–1732. doi: 10.1109/ICoSP.2012.6491914. [20] XU Shaojian, CHEN Yan, and ZHANG Peng. Integrated radar and communication based on DS-UWB[C]. 2006 3rd International Conference on Ultrawideband and Ultrashort Impulse Signals, Sevastopol, Ukraine, 2006: 142–144. doi: 10.1109/UWBUS.2006.307182. [21] GARMATYUK D, SCHUERGER J, MORTON Y T, et al. Feasibility study of a multi-carrier dual-use imaging radar and communication system[C]. The 37th European Microwave Conference, Munich, Germany, 2007: 1473–1476. doi: 10.1109/EUMC.2007.4405484. [22] RUGGIANO M and VAN GENDEREN P. Wideband ambiguity function and optimized coded radar signals[C]. The 4th European Radar Conference, Munich, Germany, 2007: 142–145. doi: 10.1109/EURAD.2007.4404957. [23] 刘少华, 黄志星. 基于扩频的雷达通信一体化信号的设计[J]. 雷达科学与技术, 2014, 12(1): 69–75. doi: 10.3969/j.issn.1672-2337.2014.01.012LIU Shaohua and HUANG Zhixing. Design of integrated radar-communication signal based on spread spectrum[J]. Radar Science and Technology, 2014, 12(1): 69–75. doi: 10.3969/j.issn.1672-2337.2014.01.012 [24] 赵玉振, 陈龙永, 张福博, 等. 一种基于OFDM-chirp的雷达通信一体化波形设计与处理方法[J]. 雷达学报, 2021, 10(3): 453–466. doi: 10.12000/JR21028ZHAO Yuzhen, CHEN Longyong, ZHANG Fubo, et al. A new method of joint radar and communication waveform design and signal processing based on OFDM-chirp[J]. Journal of Radars, 2021, 10(3): 453–466. doi: 10.12000/JR21028 [25] GARMATYUK D, SCHUERGER J, KAUFFMAN K, et al. Wideband OFDM system for radar and communications[C]. 2009 IEEE Radar Conference, Pasadena, USA, 2009: 1–6. doi: 10.1109/RADAR.2009.4977024. [26] LIU Yongjun, LIAO Guisheng, YANG Zhiwei, et al. Multiobjective optimal waveform design for OFDM integrated radar and communication systems[J]. Signal Processing, 2017, 141: 331–342. doi: 10.1016/j.sigpro.2017.06.026 [27] SIT Y L, REICHARDT L, STURM C, et al. Extension of the OFDM joint radar-communication system for a multipath, multiuser scenario[C]. 2011 IEEE Radar Conference, Kansas City, USA, 2011: 718–723. doi: 10.1109/RADAR.2011.5960632. [28] 李自琦, 梅进杰, 胡登鹏, 等. 基于分组格雷编码的OFDM雷达通信一体化系统峰均功率比抑制[J]. 雷达学报, 2014, 3(5): 548–555. doi: 10.3724/SP.J.1300.2014.14059LI Ziqi, MEI Jinjie, HU Dengpeng, et al. Peak-to-Average power ratio reduction for integration of radar and communication systems based on OFDM signals with block Golay coding[J]. Journal of Radars, 2014, 3(5): 548–555. doi: 10.3724/SP.J.1300.2014.14059 [29] NOWAK M, WICKS M, ZHANG Zhiping, et al. Co-designed radar-communication using linear frequency modulation waveform[J]. IEEE Aerospace and Electronic Systems Magazine, 2016, 31(10): 28–35. doi: 10.1109/MAES.2016.150236 [30] GAGLIONE D, CLEMENTE C, ILIOUDIS C V, et al. Waveform design for communicating radar systems using fractional Fourier transform[J]. Digital Signal Processing, 2018, 80: 57–69. doi: 10.1016/j.dsp.2018.05.002 [31] LIU Fan, ZHOU Longfei, MASOUROS C, et al. Toward dual-functional radar-communication systems: Optimal waveform design[J]. IEEE Transactions on Signal Processing, 2018, 66(16): 4264–4279. doi: 10.1109/TSP.2018.2847648 [32] EDARA I P, HASSANIEN A, AMIN M G, et al. Ambiguity function analysis for dual-function radar communications using PSK signaling[C]. 2018 52nd Asilomar Conference on Signals, Systems, and Computers, Pacific Grove, USA, 2018: 900–904. doi: 10.1109/ACSSC.2018.8645328. [33] HASSANIEN A, AMIN M G, ZHANG Y D, et al. A dual function radar-communications system using sidelobe control and waveform diversity[C]. 2015 IEEE Radar Conference, Arlington, USA, 2015: 1260–1263. doi: 10.1109/RADAR.2015.7131188. [34] HASSANIEN A, AMIN M G, ZHANG Y D, et al. Dual-function radar-communications: Information embedding using sidelobe control and waveform diversity[J]. IEEE Transactions on Signal Processing, 2016, 64(8): 2168–2181. doi: 10.1109/TSP.2015.2505667 [35] HASSANIEN A, AMIN M G, ZHANG Y D, et al. Dual-function radar-communications using phase-rotational invariance[C]. 2015 23rd European Signal Processing Conference, Nice, France, 2015: 1346–1350. doi: 10.1109/EUSIPCO.2015.7362603. [36] AHMED A, ZHANG Y D, and GU Yujie. Dual-function radar-communications using QAM-based sidelobe modulation[J]. Digital Signal Processing, 2018, 82: 166–174. doi: 10.1016/j.dsp.2018.06.018 [37] TIAN Tuanwei, LI Guchong, and ZHOU Tao. Power distribution for an OFDM-based dual-function Radar-Communication sensor[J]. IEEE Sensors Letters, 2020, 4(11): 5501504. doi: 10.1109/LSENS.2020.3033044 [38] TIAN Tuanwei, ZHANG Tianxian, KONG Lingjiang, et al. Transmit/receive beamforming for MIMO-OFDM based dual-function radar and communication[J]. IEEE Transactions on Vehicular Technology, 2021, 70(5): 4693–4708. doi: 10.1109/TVT.2021.3072094 [39] WU Qingqing and ZHANG Rui. Towards smart and reconfigurable environment: Intelligent reflecting surface aided wireless network[J]. IEEE Communications Magazine, 2020, 58(1): 106–112. doi: 10.1109/MCOM.001.1900107 [40] DI RENZO M, ZAPPONE A, DEBBAH M, et al. Smart radio environments empowered by reconfigurable intelligent surfaces: How it works, state of research, and the road ahead[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(11): 2450–2525. doi: 10.1109/JSAC.2020.3007211 [41] HU Jingzhi, ZHANG Hongliang, DI Boya, et al. Reconfigurable intelligent surface based rf sensing: Design, optimization, and implementation[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(11): 2700–2716. doi: 10.1109/JSAC.2020.3007041 [42] HUANG Kewen and WANG Huiming. Passive beamforming for IRS aided wireless networks[J]. IEEE Wireless Communications Letters, 2020, 9(12): 2035–2039. doi: 10.1109/LWC.2020.3011596 [43] DE JESUS TORRES A, SANGUINETTI L, and BJÖRNSON E. Electromagnetic interference in RIS-aided communications[J]. IEEE Wireless Communications Letters, in press, 2021. doi: 10.1109/LWC.2021.3124584. [44] WANG Jun, LIANG Yingchang, HAN Shiying, et al. Robust beamforming and phase shift design for IRS-enhanced multi-user MISO downlink communication[C]. 2020 IEEE International Conference on Communications, Dublin, Ireland, 2020: 1–6. doi: 10.1109/ICC40277.2020.9148947. [45] ZHAO Jie, YANG Xi, DAI Junyan, et al. Programmable time-domain digital-coding metasurface for non-linear harmonic manipulation and new wireless communication systems[J]. National Science Review, 2019, 6(2): 231–238. doi: 10.1093/nsr/nwy135 [46] DAI Junyan, TANG Wankai, ZHAO Jie, et al. Wireless communications through a simplified architecture based on time-domain digital coding metasurface[J]. Advanced Materials Technologies, 2019, 4(7): 1900044. doi: 10.1002/admt.201900044 [47] TANG Wankai, DAI Junyan, CHEN Mingzheng, et al. MIMO transmission through reconfigurable intelligent surface: System design, analysis, and implementation[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(11): 2683–2699. doi: 10.1109/JSAC.2020.3007055 [48] TANG Wankai, CHEN Mingzheng, CHEN Xiangyu, et al. Wireless communications with reconfigurable intelligent surface: Path loss modeling and experimental measurement[J]. IEEE Transactions on Wireless Communications, 2021, 20(1): 421–439. doi: 10.1109/TWC.2020.3024887 [49] WANG Fangzhou, LI Hongbin, and FANG Jun. Joint active and passive beamforming for IRS-assisted radar[J]. IEEE Signal Processing Letters, 2021. [50] LU Wei, LIN Qiang, SONG Ningzhe, et al. Target detection in intelligent reflecting surface aided distributed MIMO radar systems[J]. IEEE Sensors Letters, 2021, 5(3): 7000804. doi: 10.1109/LSENS.2021.3061534 [51] AUBRY A, DE MAIO A, and ROSAMILIA M. Reconfigurable intelligent surfaces for N-LOS radar surveillance[J]. IEEE Transactions on Vehicular Technology, 2021, 70(10): 10735–10749. doi: 10.1109/TVT.2021.3102315 [52] 施宏宇, 李国强, 刘康, 等. 基于反射型超表面的太赫兹偏折涡旋波束生成[J]. 雷达学报, 2021, 10(5): 785–793. doi: 10.12000/JR21070SHI Hongyu, LI Guoqiang, LIU Kang, et al. Deflective vortex beams generation based on metasurfaces in the terahertz band[J]. Journal of Radars, 2021, 10(5): 785–793. doi: 10.12000/JR21070 [53] BUZZI S, GROSSI E, LOPS M, et al. Foundations of MIMO radar detection aided by reconfigurable intelligent surfaces[EB/OL]. https://arxiv.org/abs/2105.09250, 2021. [54] WANG Xinyi, FEI Zesong, GUO Jing, et al. RIS-assisted spectrum sharing between MIMO radar and MU-MISO communication systems[J]. IEEE Wireless Communications Letters, 2021, 10(3): 594–598. doi: 10.1109/LWC.2020.3039369 [55] WANG Xinyi, FEI Zesong, ZHENG Zhong, et al. Joint Waveform design and passive beamforming for RIS-assisted dual-functional radar-communication system[J]. IEEE Transactions on Vehicular Technology, 2021, 70(5): 5131–5136. doi: 10.1109/TVT.2021.3075497 [56] BELL M R. Information theory and radar waveform design[J]. IEEE Transactions on Information Theory, 1993, 39(5): 1578–1597. doi: 10.1109/18.259642 [57] AN Lin, LI Ming, ZHANG Peng, et al. Multicontextual mutual information data for SAR image change detection[J]. IEEE Geoscience and Remote Sensing Letters, 2015, 12(9): 1863–1867. doi: 10.1109/LGRS.2015.2432071 [58] ZHANG Haowei, ZONG Binfeng, and XIE Junwei. Power and bandwidth allocation for multi-target tracking in collocated MIMO radar[J]. IEEE Transactions on Vehicular Technology, 2020, 69(9): 9795–9806. doi: 10.1109/TVT.2020.3002899 [59] 王璐璐. 基于信息论的自适应波形设计[D]. [博士论文], 国防科学技术大学, 2015. doi: 10.7666/d.D01107893.WANG Lulu. Adaptive waveform design based on information theory[D]. [Ph. D. dissertation], National University of Defense Technology, 2015. doi: 10.7666/d.D01107893. [60] 张钰. 基于最大互信息准则的认知雷达波形优化算法研究[D]. [硕士论文], 西安电子科技大学, 2012. doi: 10.7666/d.d216380.ZHANG Yu. Study on the waveform design algorithm for cognitive radar based on maximum mutual information rule[D]. [Master dissertation], Xidian University, 2012. doi: 10.7666/d.d216380. [61] TANG Bo and LI Jian. Spectrally constrained MIMO radar waveform design based on mutual information[J]. IEEE Transactions on Signal Processing, 2019, 67(3): 821–834. doi: 10.1109/TSP.2018.2887186 [62] 崔国龙, 余显祥, 杨婧, 等. 认知雷达波形优化设计方法综述[J]. 雷达学报, 2019, 8(5): 537–557. doi: 10.12000/JR19072CUI Guolong, YU Xianxiang, YANG Jing, et al. An overview of waveform optimization methods for cognitive radar[J]. Journal of Radars, 2019, 8(5): 537–557. doi: 10.12000/JR19072 [63] TIAN Tuanwei, ZHANG Tianxian, LI Guchong, et al. Mutual information-based power allocation and co-design for multicarrier radar and communication systems in coexistence[J]. IEEE Access, 2019, 7: 159300–159312. doi: 10.1109/ACCESS.2019.2950890 [64] TIAN Tuanwei, ZHANG Tianxian, KONG Lingjiang, et al. Mutual information based partial band coexistence for joint radar and communication system[C]. 2019 IEEE Radar Conference, Boston, USA, 2019: 1–5. doi: 10.1109/RADAR.2019.8835671. [65] TKACENKO A and VAIDYANATHAN P P. Iterative greedy algorithm for solving the FIR paraunitary approximation problem[J]. IEEE Transactions on Signal Processing, 2006, 54(1): 146–160. doi: 10.1109/TSP.2005.861054 [66] OMIDVAR M N, YANG Ming, MEI Yi, et al. DG2: A faster and more accurate differential grouping for large-scale black-box optimization[J]. IEEE Transactions on Evolutionary Computation, 2017, 21(6): 929–942. doi: 10.1109/TEVC.2017.2694221 [67] SUN Ying, BABU P, and PALOMAR D P. Majorization-minimization algorithms in signal processing, communications, and machine learning[J]. IEEE Transactions on Signal Processing, 2017, 65(3): 794–816. doi: 10.1109/TSP.2016.2601299 [68] GRANT M C. CVX Research, Inc. is here[EB/OL]. http://www.cvxr.com/cvx.r, 2012. [69] CAPON J. High-resolution frequency-wavenumber spectrum analysis[J]. Proceedings of the IEEE, 1969, 57(8): 1408–1418. doi: 10.1109/PROC.1969.7278 [70] DU Xiaolin, AUBRY A, DE MAIO A, et al. Hidden convexity in robust waveform and receive filter bank optimization under range unambiguous clutter[J]. IEEE Signal Processing Letters, 2020, 27: 885–889. doi: 10.1109/LSP.2020.2992323 [71] CHEN Chunyang and VAIDYANATHAN P. MIMO radar waveform optimization with prior information of the extended target and clutter[J]. IEEE Transactions on Signal Processing, 2009, 57(9): 3533–3544. doi: 10.1109/TSP.2009.2021632 [72] LIU Jun, LI Hongbin, and HIMED B. Joint optimization of transmit and receive beamforming in active arrays[J]. IEEE Signal Processing Letters, 2014, 21(1): 39–42. doi: 10.1109/LSP.2013.2289325 -
图(9) / 表(1)
计量
- 文章访问数: 2060
- HTML全文浏览量: 1441
- PDF下载量: 217
- 被引次数: 0
