作者中心
专家审稿
责编办公
编辑办公
-
摘要: 该文提出一种基于四元数的宽带鲁棒自适应波束形成方法。在利用四元数构造阵元输出的基础上，通过期望信号复包络对齐技术，建立四元数域宽带对合增广宽线性信号模型，以联合利用四元数阵列输出矢量的3种对合信息和2阶统计特性以及信号非圆信息，采用信号加干扰子空间投影方式，有效提取期望信号，抑制多个不相关干扰和噪声，进而实现四元数域宽带鲁棒自适应波束形成。同其它宽带波束形成方法相比，该方法对非圆信号的接收性能提升，可以实现阵列虚拟孔径扩展，有效克服指向误差带来的性能下降问题。计算机仿真结果验证了该方法的性能。Abstract: A robust quaternion-valued wideband adaptive beamformer is proposed, in which a quaternion is utilized to arrange the output of the array element. By exploiting the augmented envelope alignment technique, adopting the three involutions of quaternion, and incorporating the noncircular information of the signal simultaneously, a quaternion-valued wideband augmented signal model is established to achieve the robust adaptive beamforming based on signal subspace projection. Compared with other wideband beamformers, the proposed scheme exhibits a better performance in extracting noncircular signals by array aperture extension, and is insensitive to the pointing error. The simulation results verify the efficiency of the proposed beamformer.
-
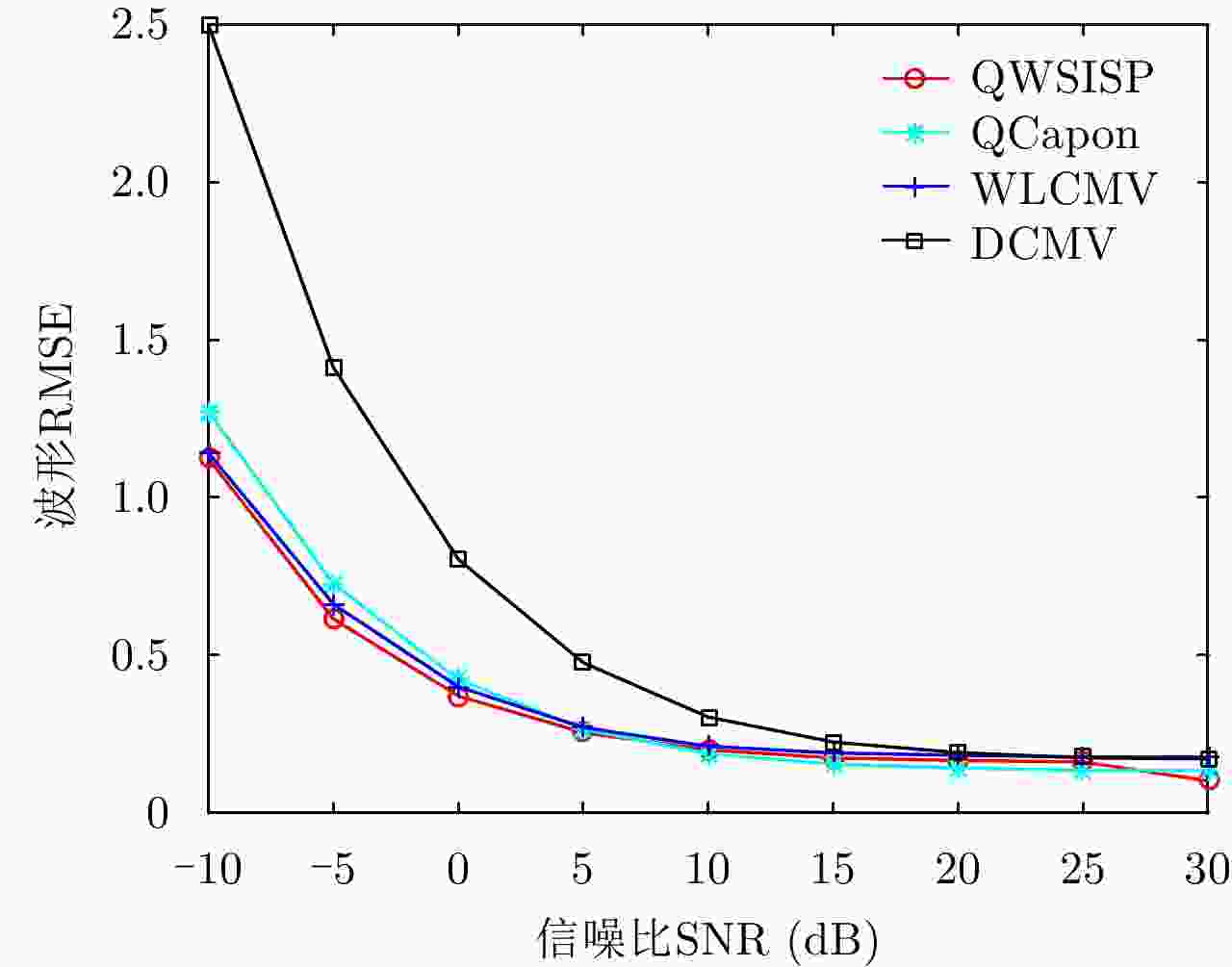
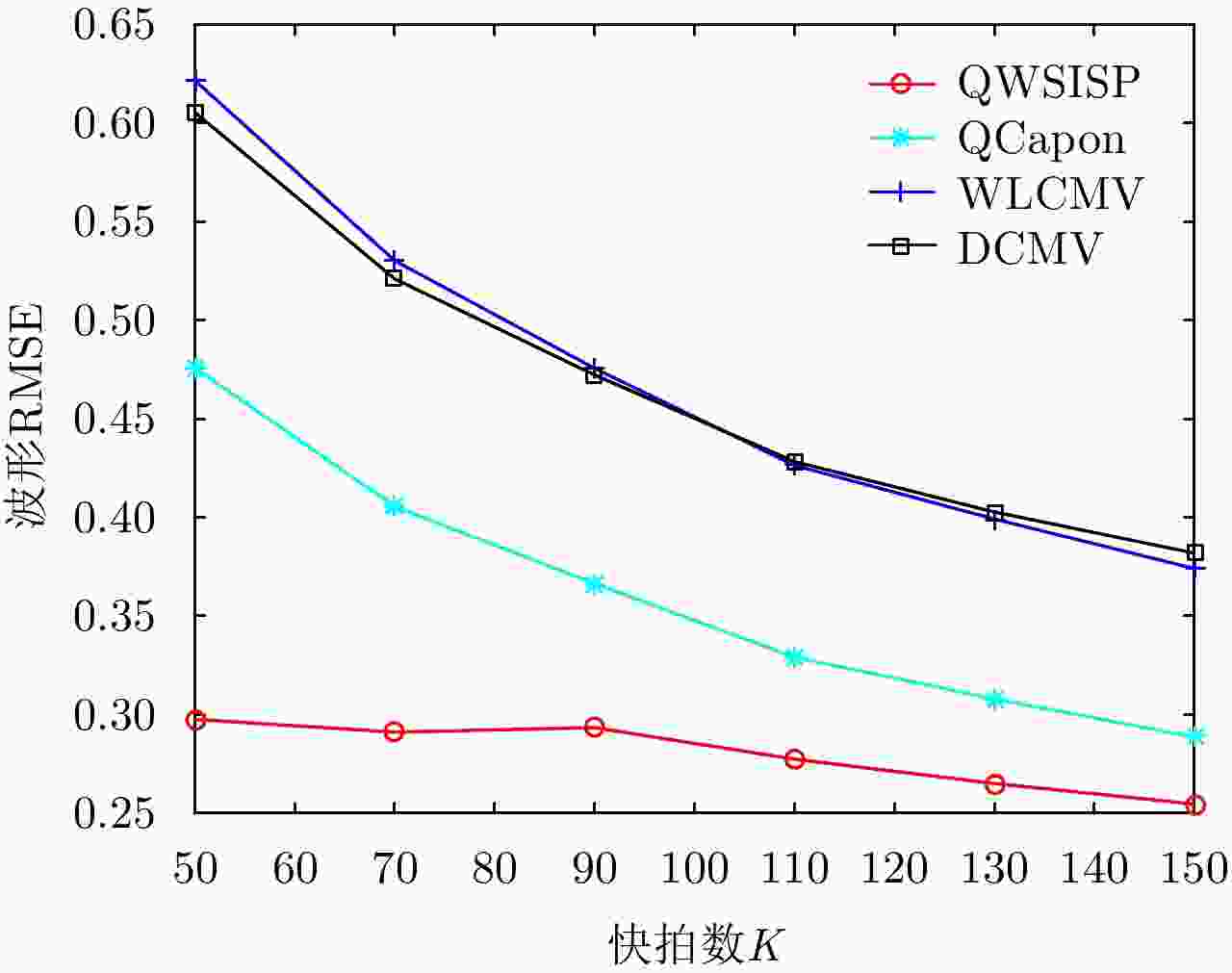
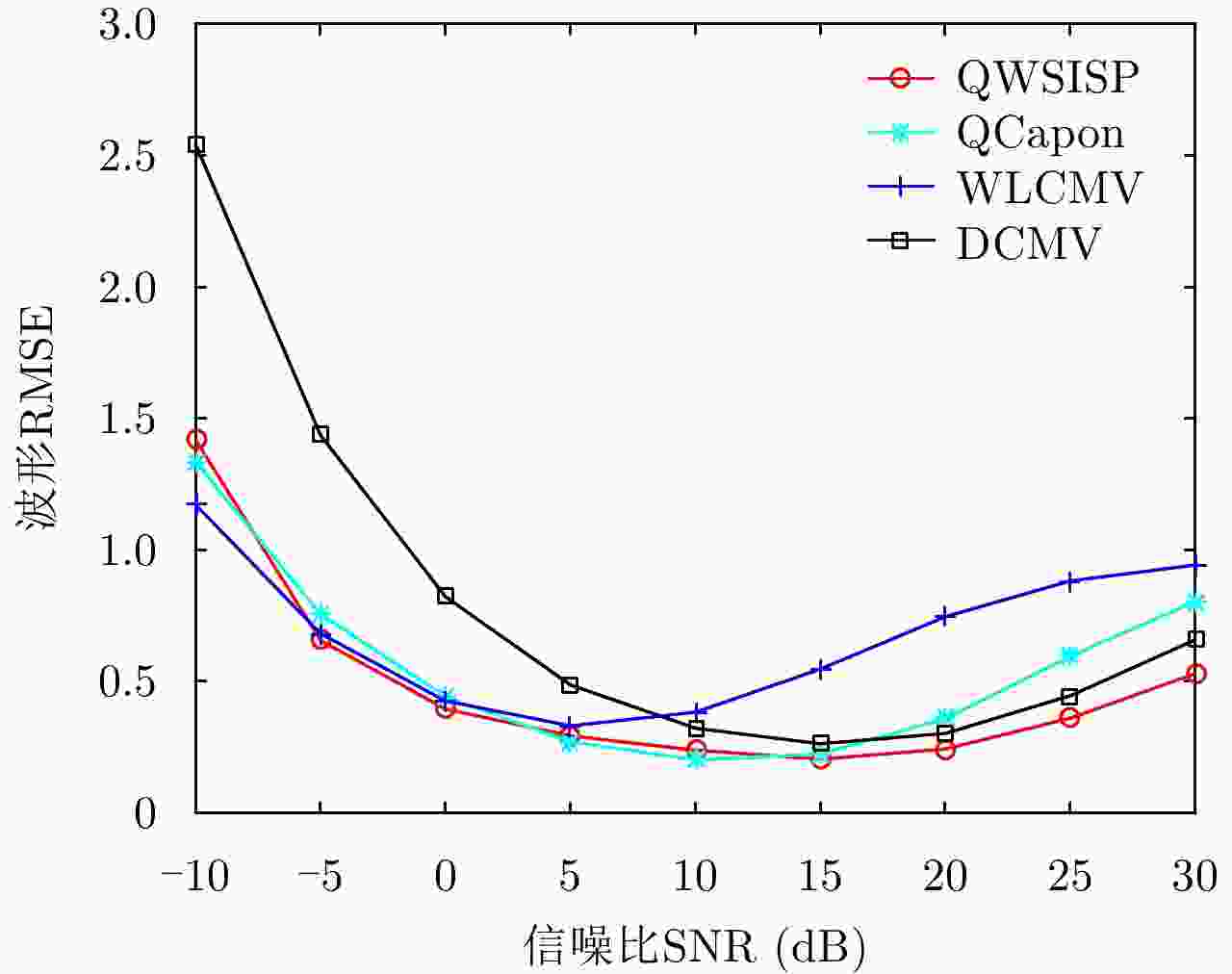
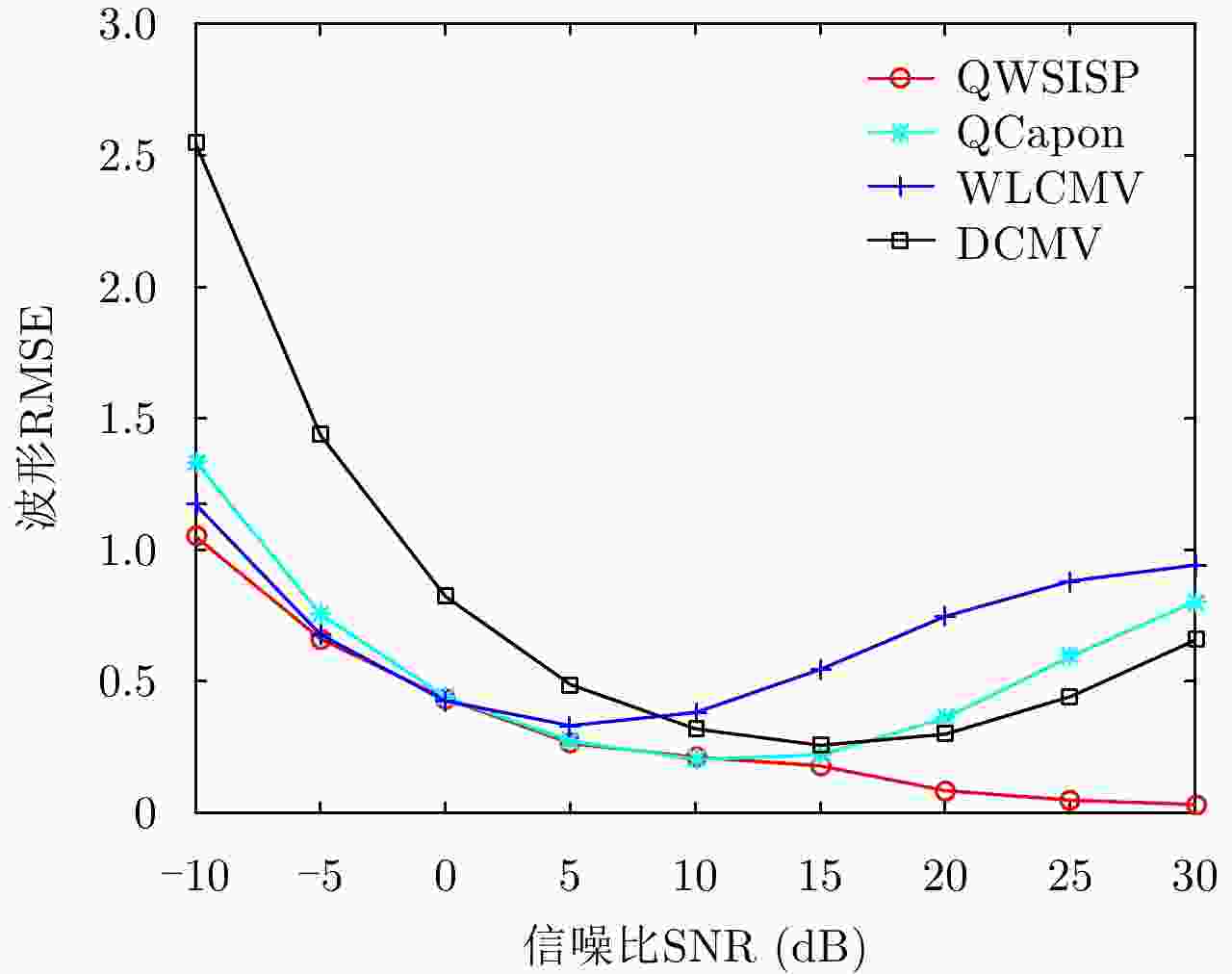
[1] GOU X M, XU Y G, LIU Z W, et al. Quaternion-capon beamformer using crossed-dipole arrays[C]. Proceedings of the 4th IEEE International Symposium on Microwave, Antenna, Propagation and EMC Technologies for Wireless Communications, Beijing, China, 2011: 34–37. doi: 10.1109/MAPE.2011.6156140. [2] TAO J W and CHANG W X. The MVDR beamformer based on hypercomplex processes[C]. Proceedings of 2012 International Conference on Computer Science and Electronics Engineering, Hangzhou, China, 2012: 273–277. doi: 10.1109/ICCSEE.2012.430. [3] TAO J W and CHANG W X. A novel combined beamformer based on hypercomplex processes[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(2): 1276–1289. doi: 10.1109/TAES.2013.6494413 [4] ZHANG X R, LIU W, XU Y G, et al. Quaternion-valued robust adaptive beamformer for electromagnetic vector-sensor arrays with worst-case constraint[J]. Signal Processing, 2014, 104: 274–283. doi: 10.1016/j.sigpro.2014.04.006 [5] LIU Z W, WANG Y X, ZHANG X R, et al. Spatially smoothed Quaternion-Capon beamforming in the presence of coherent interferences[J]. Journal of Beijing Institute of Technology, 2016, 25(2): 225–230. doi: 10.15918/j.jbit1004-0579.201625.0210 [6] TOOK C C and MANDIC D P. A quaternion widely linear adaptive filter[J]. IEEE Transactions on Signal Processing, 2010, 58(8): 4427–4431. doi: 10.1109/TSP.2010.2048323 [7] ZHANG X R, LIU Z W, FAN Z Y, et al. Quaternion-valued robust adaptive beamformer based on widely linear processing[C]. Proceedings of the 19th International Conference on Digital Signal Processing, Hong Kong, China, 2014: 719–724. doi: 10.1109/ICDSP.2014.6900758. [8] 刘志文, 王荔, 徐友根. 四元数域对合增广宽线性自适应波束形成[J]. 电子与信息学报, 2017, 39(7): 1525–1531. doi: 10.11999/JEIT160988LIU Zhi-wen, WANG Li, and XU You-gen. Quaternion-valued widely linear adaptive beamforming via involution augmentation[J]. Journal of Electronics &Information Technology, 2017, 39(7): 1525–1531. doi: 10.11999/JEIT160988 [9] FROST O L. An algorithm for linearly constrained adaptive array processing[J]. Proceedings of the IEEE, 1972, 60(8): 926–935. doi: 10.1109/PROC.1972.8817 [10] MENG E and CANTONI A. Derivative constraints for broad-band element space antenna array processors[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1983, 31(6): 1378–1393. doi: 10.1109/TASSP.1983.1164219 [11] BUCKLEY K M. Spatial/spectral filtering with linearly constrained minimum variance beamformers[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1987, 35(3): 249–266. doi: 10.1109/TASSP.1987.1165142 [12] CHEVALIER P and BLIN A. Widely linear MVDR beamformers for the reception of an unknown signal corrupted by noncircular interferences[J]. IEEE Transactions on Signal Processing, 2007, 55(11): 5323–5336. doi: 10.1109/TSP.2007.899394 [13] WANG G H, LIE J P, and SEE C M S. A robust approach to optimum widely linear MVDR beamformer[C]. Proceedings of 2012 IEEE International Conference on Acoustics, Speech and Signal Processing, Kyoto, Japan, 2012: 2593–2596. doi: 10.1109/ICASSP.2012.6288447. [14] XU Y G, MA J Y, LIU Z W, et al. A class of diagonally loaded robust Capon beamformers for noncircular signals of interest[J]. Signal Processing, 2014, 94: 670–680. doi: 10.1016/j.sigpro.2013.07.013 [15] CHEVALIER P, DELMAS J P, and OUKACI A. Optimal widely linear MVDR beamforming for noncircular signals[C]. Proceedings of 2009 IEEE International Conference on Acoustics, Speech and Signal Processing, Taipei, China, 2009: 3573–3576. doi: 10.1109/ICASSP.2009.4960398. [16] HAMILTON W R. On a new species of imaginary quantities connected with a theory of quaternions[J]. Proceedings of the Royal Irish Academy, 1843, 2: 424–434. [17] WARD J P. Quaternions and Cayley Numbers: Algebra and Applications[M]. Dordrecht: Kluwer, 1997: 54–102. doi: 10.1007/978-94-011-5768-1. [18] ELL T A and SANGWINE S J. Quaternion involutions and anti-involutions[J]. Computers & Mathematics with Applications, 2007, 53(1): 137–143. doi: 10.1016/j.camwa.2006.10.029 [19] JIANG M D, LI Y, and LIU W. Properties of a general quaternion-valued gradient operator and its applications to signal processing[J]. Frontiers of Information Technology & Electronic Engineering, 2016, 17(2): 83–95. doi: 10.1631/FITEE.1500334 -
下载:
图(7)
计量
- 文章访问数: 2576
- HTML全文浏览量: 577
- PDF下载量: 137
- 被引次数: 0
