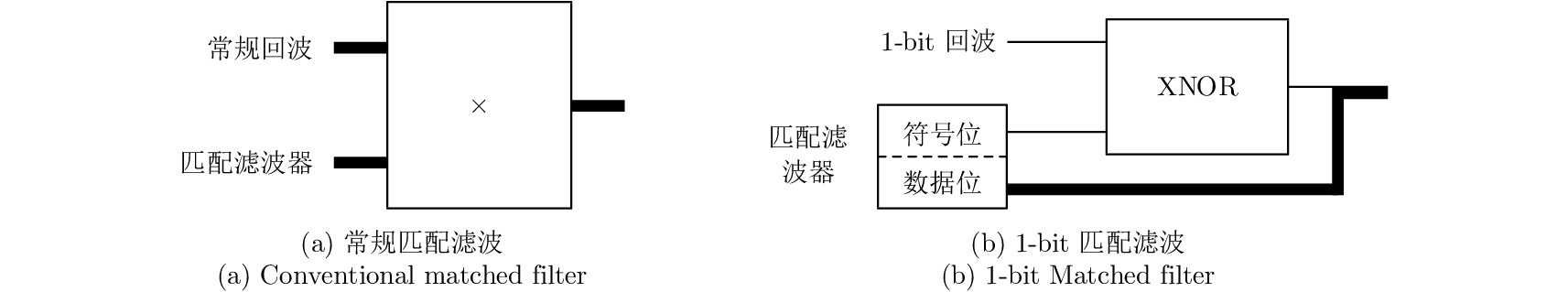
1-bit SAR Imaging Method Based on Single-frequency Time-varying Threshold
-
摘要: 该文提出一种基于单频时变阈值的1-bit合成孔径雷达(SAR)成像方法,通过将回波数据与时变阈值比较,将其量化为1-bit采样数据,从而降低SAR回波数据的位宽,达到简化系统、提升效率的目的。传统的1-bit采样将信号与0阈值比较,这将造成信号相对幅度的非线性失真,影响成像质量。而随机时变阈值虽然能够保留幅度信息,却会引入额外的类噪声干扰。单频时变阈值将能够有效地保留1-bit采样量化中丢失的相对幅度信息,同时避免引入类噪声干扰,有效地提高了1-bit采样量化下的SAR成像质量。通过仿真实验定量分析了算法的成像聚焦质量、幅度信息保持能力,并通过对场景目标的成像验证了算法的有效性。Abstract: This paper proposes a 1-bit Synthetic Aperture Radar (SAR) imaging method based on a single-frequency time-varying threshold. Synthetic aperture radar echoes are quantized to 1-bit sampling data by comparing the data with the threshold; this reduces the data-width of the SAR echoes, consequently simplifying the system and improving efficiency. The conventional 1-bit sampling compares the signal to a zero threshold, bringing nonlinear distortion to the relative amplitude and degrading the imaging quality. The random threshold can keep the amplitude information, but it introduces additional noise-like interferences. In contrary, the single-frequency time-varying threshold can maintain the amplitude information lost during the 1-bit sampling and quantization, and at the same time, eliminate noise-like interferences; thus, the imaging quality of SAR using 1-bit sampling and quantization can be improved. The focusing quality and the amplitude-maintaining ability of the proposed approach is quantitatively analyzed, and the effectiveness of the approach is verified by an imaging experiment on a scene.
-
Key words:
- Synthetic Aperture Radar (SAR) /
- 1-bit sampling /
- Time-varying threshold
-
表 1 SAR参数
Table 1. SAR parameters
参数名称 参数值 信号带宽(MHz) 300 脉冲宽度(μs) 1 采样率(GHz) 6.9 载频(GHz) 37.6 单频阈值频率(GHz) 16.2 信号阈值比(dB) 0 场景中心斜距(km) 10 天线孔径(m) 1 载机速度(m/s) 50 脉冲重复频率(Hz) 400 表 2 单散射点聚焦质量指标
Table 2. Focusing quality indexes of the single scatterer
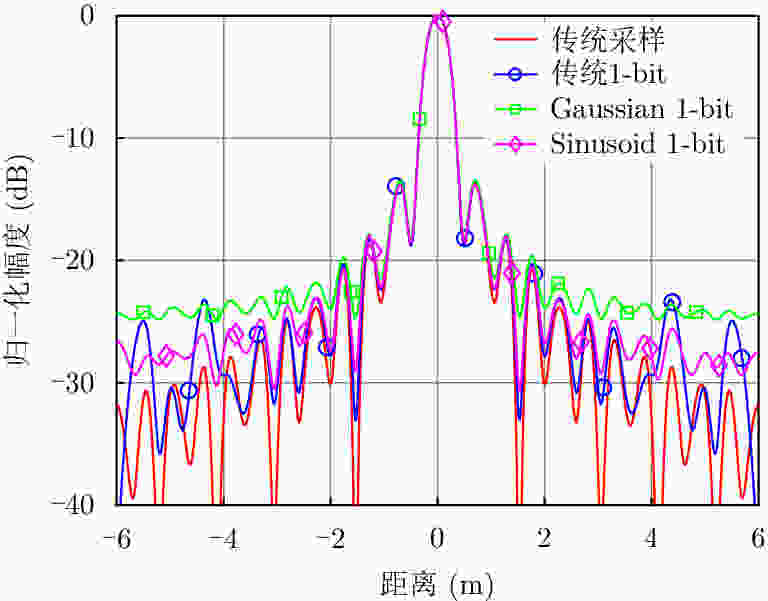
采样方法 PSLR (dB) ISLR (dB) IRW (m) 均值 方差 均值 方差 均值 方差 传统采样 –13.7217 – –10.1301 – 0.4435 – 传统1-bit采样 –13.4556 – –9.3684 – 0.4435 – Gaussian 1-bit采样 –13.4054 0.0059 –8.0347 0.0007 0.4435 0.2163×10–5 Sinusoid 1-bit采样 –13.8106 0.0016 –9.3048 0.0005 0.4474 0.1621×10–5 表 3 多散射点幅度质量指标
Table 3. Amplitude quality indexes of multiple scatterers
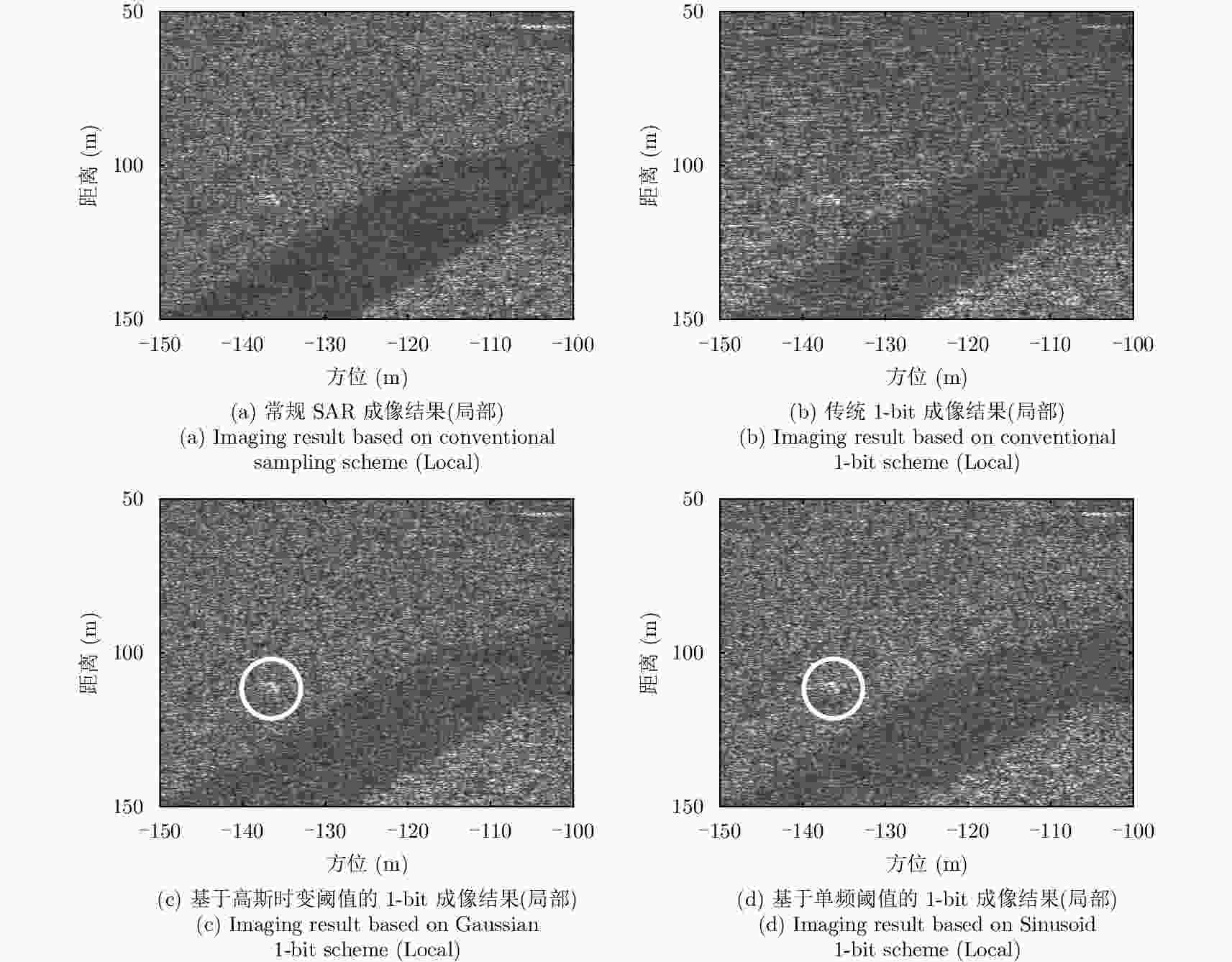
采样方法 幅度1 幅度2 幅度3 均值 误差 方差 均值 误差 方差 均值 误差 方差 传统采样 0.9729 2.71% – 1.9683 1.58% – 2.9775 0.75% – 传统1-bit采样 0.1818 – – 0.3185 – – 0.5067 – – 传统1-bit采样(缩放) 1.1311 13.11% – 1.7710 11.45% – 3.0834 2.78% – Gaussian 1-bit采样 1.0477 4.77% 0.0633 1.9870 0.65% 0.0629 2.9920 0.27% 0.0617 Sinusoid 1-bit采样 1.0181 1.81% 0.0377 2.0186 0.93% 0.0189 2.9812 0.63% 0.0108 -
[1] 保铮, 邢孟道, 王彤. 雷达成像技术[M]. 北京: 电子工业出版社, 2005: 1–20Bao Zheng, Xing Meng-dao, and Wang Tong. Radar Imaging Technology[M]. Beijing: Publishing House of Electronics Industry, 2005: 1–20 [2] 邢涛, 胡庆荣, 李军, 等. 毫米波高分辨SAR成像算法性能分析[J]. 现代防御技术, 2015, 43(1): 81–86. DOI: 10.3969/j.issn.1009-086x.2015.01.014Xing Tao, Hu Qing-rong, Li Jun, et al. Analysis of millimeter wave high resolution SAR imaging algorithm performances[J]. Modern Defence Technology, 2015, 43(1): 81–86. DOI: 10.3969/j.issn.1009-086x.2015.01.014 [3] 王辉, 赵凤军, 邓云凯. 毫米波合成孔径雷达的发展及其应用[J]. 红外与毫米波学报, 2015, 34(4): 452–459. DOI: 10.11972/j.issn.1001-9014.2015.04.013Wang Hui, Zhao Feng-jun, and Deng Yun-kai. Development and application of the millimeter wave SAR[J]. Journal of Infrared and Millimeter Waves, 2015, 34(4): 452–459. DOI: 10.11972/j.issn.1001-9014.2015.04.013 [4] 邢涛, 胡庆荣, 李军, 等. 机载毫米波高分辨大斜视合成孔径雷达成像[J]. 浙江大学学报(工学版), 2015, 49(12): 2355–2362. DOI: 10.3785/j.issn.1008-973X.2015.12.016Xing Tao, Hu Qing-rong, Li Jun, et al. Synthetic aperture radar imaging of airborne millimeter wave with high resolution and high squint[J]. Journal of Zhejiang University(Engineering Science) , 2015, 49(12): 2355–2362. DOI: 10.3785/j.issn.1008-973X.2015.12.016 [5] 费鹏, 方维海, 温鑫, 等. 用于人员安检的主动毫米波成像技术现状与展望[J]. 微波学报, 2015, 31(2): 91–96Fei Peng, Fang Wei-hai, Wen Xin, et al. State of the art and future prospect of the active millimeter wave imaging technique for personnel screening[J]. Journal of Microwaves, 2015, 31(2): 91–96 [6] 马超, 张小虎, 杨建超, 等. 基于MLBF的毫米波双站SAR前视Omega-k成像算法[J]. 红外与毫米波学报, 2017, 36(4): 490–497. DOI: 10.11972/j.issn.1001-9014.2017.04.018Ma Chao, Zhang Xiao-hu, Yang Jian-chao, et al. Omega-k algorithm based on MLBF for millimeter wave bistatic forward-looking SAR imaging[J]. Journal of Infrared and Millimeter Waves, 2017, 36(4): 490–497. DOI: 10.11972/j.issn.1001-9014.2017.04.018 [7] Franceschetti G, Pascazio V, and Schirinzi G. Processing of signum coded SAR signal: Theory and experiments[J]. IEE Proceedings F-Radar and Signal Processing, 1991, 138(3): 192–198. DOI: 10.1049/ip-f-2.1991.0025 [8] Franceschetti G, Tesauro M, and Wall S. SAR and one-bit coding: New ideas[C]. IEEE International Geoscience and Remote Sensing Symposium, Lincoln, USA, 1996: 51–53 [9] Franceschetti G, Impagnatiello F, Rubertone F, et al.. Results of the X-SAR real time one-bit processor[C]. IEEE International Geoscience and Remote Sensing Symposium, Taking the Pulse of the Planet: The Role of Remote Sensing in Managing the Environment, Honolulu, USA, 2000: 99–101 [10] 黄杰文, 祁海明, 李杨, 等. DBF-SAR系统1比特量化设计[J]. 宇航学报, 2011, 32(11): 2387–2394. DOI: 10.3873/j.issn.1000-1328.2011.11.013Huang Jie-wen, Qi Hai-ming, Li Yang, et al. One-bit quantization for DBF-SAR[J]. Journal of Astronautics, 2011, 32(11): 2387–2394. DOI: 10.3873/j.issn.1000-1328.2011.11.013 [11] Boufounos P T and Baraniuk R G. 1-bit Compressive sensing[C]. Proceedings of the 42nd Annual Conference on Information Sciences and Systems, Princeton, USA, 2008: 16–21. DOI: 10.1109/CISS.2008.4558487 [12] Karahanoglu N B and Erdogan H. Compressed sensing signal recovery via forward-backward pursuit[J]. Digital Signal Processing, 2013, 23(5): 1539–1548. DOI: 10.1016/j.dsp.2013.05.007 [13] Laska J N, Wen Z W, Yin W T, et al. Trust, but verify: Fast and accurate signal recovery from 1-bit compressive measurements[J]. IEEE Transactions on Signal Processing, 2011, 59(11): 5289–5301. DOI: 10.1109/TSP.2011.2162324 [14] Plan Y and Vershynin R. One-bit compressed sensing by linear programming[J]. Communications on Pure and Applied Mathematics, 2013, 66(8): 1275–1297. DOI: 10.1002/cpa.v66.8 [15] Jacques L, Laska J N, Boufounos P T, et al. Robust 1-bit compressive sensing via binary stable embeddings of sparse vectors[J]. IEEE Transactions on Information Theory, 2013, 59(4): 2082–2102. DOI: 10.1109/TIT.2012.2234823 [16] Plan Y and Vershynin R. Robust 1-bit compressed sensing and sparse logistic regression: A convex programming approach[J]. IEEE Transactions on Information Theory, 2013, 59(1): 482–494. DOI: 10.1109/TIT.2012.2207945 [17] Dong X and Zhang Y H. A MAP approach for 1-bit compressive sensing in synthetic aperture radar imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2015, 12(6): 1237–1241. DOI: 10.1109/LGRS.2015.2390623 [18] 周崇彬. 单比特合成孔径雷达稀疏成像技术的研究[D]. [博士论文], 中国科学技术大学, 2016: 29–47Zhou Chong-bin. Studies on 1-bit coded synthetic aperture radar sparse imaging[D]. [Ph.D. dissertation], University of Science and Technology of China, 2016: 29–47 [19] Gianelli C, Xu L Z, Li J, et al.. One-bit compressive sampling with time-varying thresholds for sparse parameter estimation[C]. Sensor Array and Multichannel Signal Processing Workshop, Rio de Janerio, Brazil, 2016: 1–5. DOI: 10.1109/SAM.2016.7569634 [20] Qian C and Li J. ADMM for harmonic retrieval from one-bit sampling with time-varying thresholds[C]. IEEE International Conference on Acoustics, Speech and Signal Processing, New Orleans, USA, 2017: 3699–3703. DOI: 10.1109/ICASSP.2017.7952847 [21] Stein M S. Signal parameter estimation with 1-bit ADC: Performance bounds, methods and system design[D]. [Ph.D. dissertation], Technische Universität München, 2016: 19–24 [22] Abramowitz M. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables[M]. Washington, USA: Government Printing Office, 1972: 361–556 [23] Zhao B, Huang L, Li J, et al. Deceptive SAR jamming based on 1-bit sampling and time-varying thresholds[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2018, 11(3): 939–950. DOI: 10.1109/JSTARS.2018.2793247 [24] Brunet D, Vrscay E R, and Wang Z. On the mathematical properties of the structural similarity index[J]. IEEE Transactions on Image Processing, 2012, 21(4): 1488–1499. DOI: 10.1109/TIP.2011.2173206 -



 作者中心
作者中心 专家审稿
专家审稿 责编办公
责编办公 编辑办公
编辑办公
 下载:
下载: