作者中心
专家审稿
责编办公
编辑办公
-
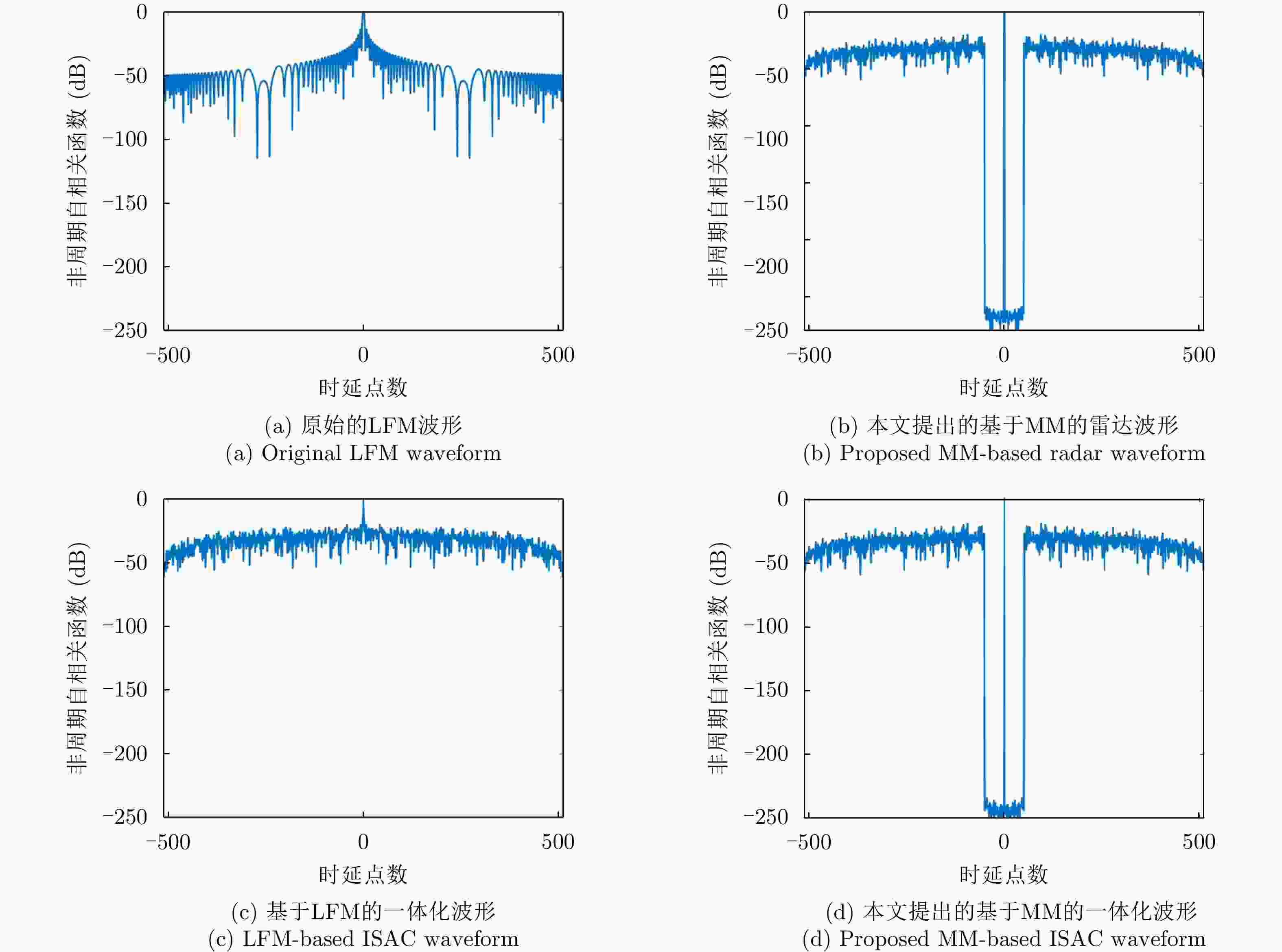
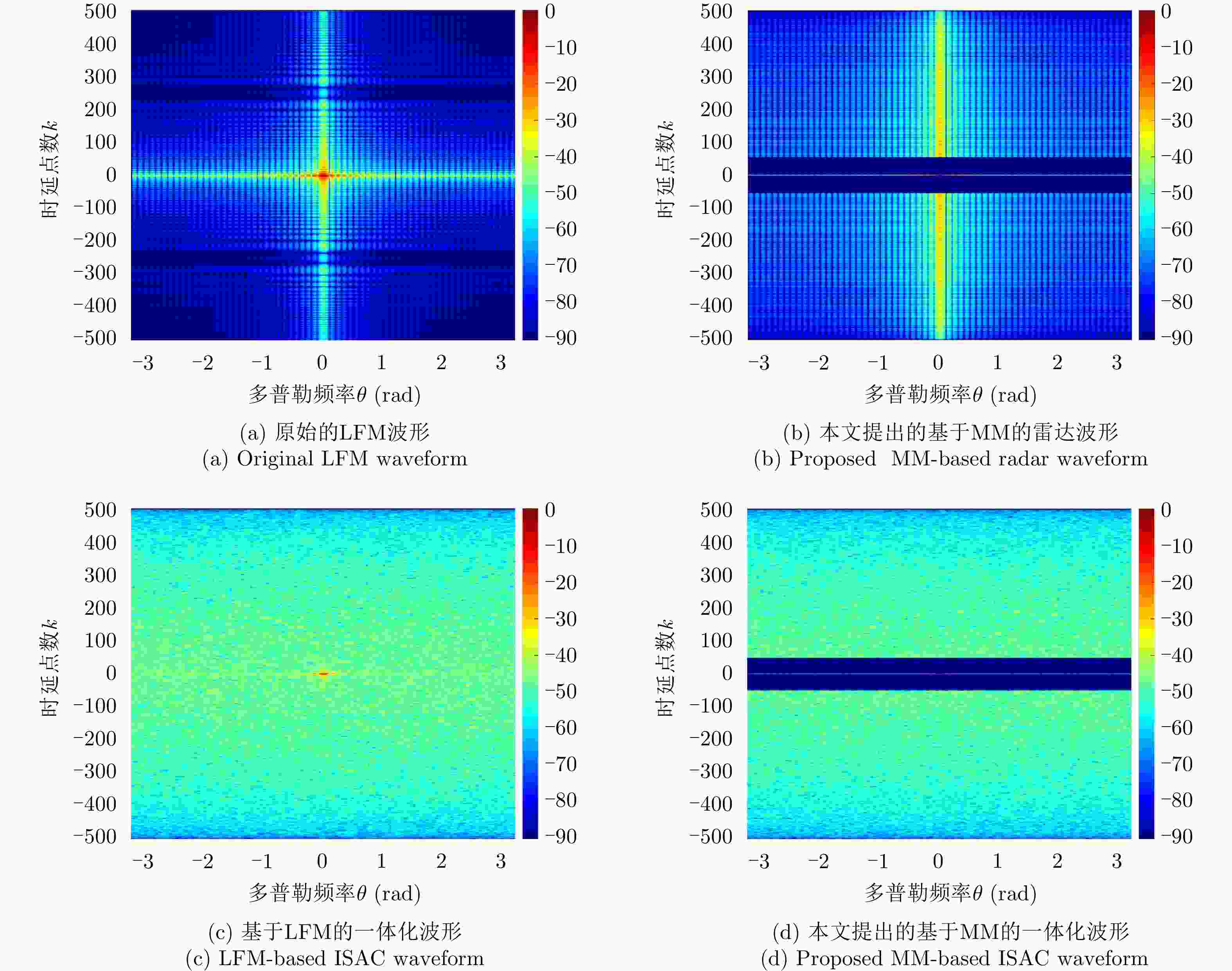
摘要: 针对现有联合设计的通感一体化波形对运动目标探测性能不足的问题，该文提出了一种具有多普勒容忍性的通感一体化波形联合设计方案。首先，基于脉冲串模糊函数，推导了构造多普勒容忍波形等价于波形在相关区内具有极低的积分旁瓣电平。基于此，构建了以最小化一体化波形的加权积分旁瓣电平为优化准则，以发射波形的能量、峰均功率比以及与通信波形之间的相位差为约束条件的优化问题，从而实现具有多普勒容忍性的通感一体化波形的构造。由于该优化问题的非凸性，该文提出一种基于优化最小化的迭代优化算法对其进行求解。数值仿真实验表明，相比传统一体化波形，该文提出的一体化波形具有更高的多普勒容忍性和更低的误符号率，在保证通信质量的前提下显著提升了通感一体化系统对运动目标的探测性能。Abstract: Because Doppler resilience is limited in the existing joint design of Integrated Sensing And Communication (ISAC) waveforms, a new Doppler resilient ISAC waveform design is proposed based on a joint design. First, with the pulse train ambiguity function, a construction of the Doppler resilient pulse train is deduced, which is equivalent to designing a waveform with a very low integral sidelobe level in a correlation zone. Accordingly, to construct the Doppler resilient ISAC pulse train, an optimization problem is proposed that takes minimizing the weighted integral sidelobe level of the ISAC waveform as the objective function and takes the energy of the transmitted waveform, the peak-to-average power ratio, and the phase difference between the transmitted ISAC waveform and the communication data modulated waveform as constraints. Because the optimization problem is nonconvex, an iterative optimization algorithm based on the Majorization-Minimization (MM) framework is proposed to solve it. Numerical simulation experiments show that compared with the traditional ISAC waveform design method, the ISAC waveform proposed in this paper has higher Doppler resilience and a lower symbol error rate, and the detection performance of the ISAC system for moving targets is considerably improved without loss of communication quality.
-
图 3 基于MM的一体化波形在不同参数下的SER比较
Figure 3. SER comparison under various parameters based on MM-based ISAC waveform
图 4 基于MM和基于LFM的一体化波形SER性能比较
Figure 4. SER comparison between MM-based and LFM-based ISAC waveform
表 1 基于FFT/IFFT快速计算
${\boldsymbol{a}}_n^{(t)}$ [30]Table 1. Compute
${\boldsymbol{a}}_n^{(t)}$ based on FFT/IFFT[30]1. 输入：$ {\boldsymbol{x}}_n^{(t)} $, $ \{ {\omega _l}\} _{l = - L + 1}^{L - 1} $，执行： 2. ${\boldsymbol{fx} } = {\rm{fft} }({[{\boldsymbol{x} }_n^{(t)},{ {\bf{0} }_{1 \times L} }]^{\rm{T} } })$ 3. ${\boldsymbol{r} } = {\rm{ifft} }({\left| { {\boldsymbol{fx} } } \right|^2})$ 4. $ {\boldsymbol{c}} = {\boldsymbol{r}} \circ {[0,{\omega _1}, \cdots ,{\omega _{L - 1}},0,{\omega _{L - 1}}, \cdots ,{\omega _1}]^{\text{T}}} $ 5. ${\boldsymbol{\mu}} = {\rm{fft} }({\boldsymbol{c} })$ 6. ${\bf{tmp} } = {\rm{ifft} }\left( {\mu \circ ({\boldsymbol{fx} })} \right)$ 7. ${\boldsymbol{Rx} }_n^{(t)} = {\bf{tm} }{ {\bf{p} }_{1:L} }$ 8. ${\lambda }_{J}=\mathop {\max }\limits_{k}\{ {\omega }_{k}(L-k):k=1,2，\cdots ,L-1\}$ 9. ${\lambda _u} = \dfrac{1}{2}\left( { { \mathop {\max }\limits_{1 \le i \le L} }{\mu _{2i} } + { \mathop {\max }\limits_{1 \le i \le L} }{\mu _{2i - 1} } } \right)$ 10. $ {\boldsymbol{a}}_n^{(t)} = - {\boldsymbol{R}}{{\boldsymbol{x}}^{(t)}} + ({\lambda _J}L + {\lambda _u}){{\boldsymbol{x}}^{(t)}} $ 11. 输出：$ {\boldsymbol{a}}_n^{(t)} $ 
下载: 导出CSV
表 2 二分法求
$ {\boldsymbol{\delta}} $ Table 2. Bisection method for
${\boldsymbol{ \delta }}$ 1. 初始化：设置搜索区间$ ({\delta _L},{\delta _U}) $。设置$ {\delta _L} = 0 $以及
$ {\delta _U} = \dfrac{{{\alpha _U}}}{{\min \{ |{a_{n,l}}| \ne 0,l = 1,2, \cdots ,L\} }} $, 令$ \delta \in ({\delta _L},{\delta _U}) $2. While $ |{\delta _U} - {\delta _L}| > {\text{eps}} \cdot |{\delta _U}| $ 3. $ \delta = ({\delta _L} + {\delta _U})/2 $ 4. If $ {\text{sign}}({f_L}(\delta )) = {\text{sign}}({f_L}({\delta _U})) $ 5. $ {\delta _U} = \delta $ 6. or 7. $ {\delta _L} = \delta $ 8. End if 9. End while，输出：$ \delta $
下载: 导出CSV
表 3 基于MM算法的一体化波形设计
Table 3. MM-based algorithm for ISAC waveform design
1. 输入：$ \alpha $, $\epsilon$, $ {\omega _l} $, $ {{\boldsymbol{e}}_n} $，以及$ {\boldsymbol{x}}_n^{(0)} = {{\boldsymbol{e}}_n} $ 2. for $ t = 0,1,2, \cdots $执行 3. 计算 $ {\boldsymbol{a}}_n^{(t)} $(根据表1执行) 4. 计算 $\bar {\boldsymbol{x} } _n^{(t + 1)}$(根据式(40)) 5. 计算 ${\boldsymbol{x} }_n^{(t + 1)} = \Pi (\bar {\boldsymbol{x} } _n^{(t + 1)})$(根据式(41)) 6. end for (当收敛时) 7. 输出：$ {\boldsymbol{x}}_n^{(t + 1)} $
下载: 导出CSV
-
[1] 田团伟, 邓浩, 鲁建华, 等. 智能反射面辅助雷达通信双功能系统的多载波波形优化方法[J]. 雷达学报, 2022, 11(2): 240–254. doi: 10.12000/JR21138TIAN Tuanwei, DENG Hao, LU Jianhua, et al. Multicarrier waveform optimization method for an intelligent reflecting surface-assisted dual-function radar-communication system[J]. Journal of Radars, 2022, 11(2): 240–254. doi: 10.12000/JR21138 [2] 刘凡, 袁伟杰, 原进宏, 等. 雷达通信频谱共享及一体化: 综述与展望[J]. 雷达学报, 2021, 10(3): 467–484. doi: 10.12000/JR20113LIU Fan, YUAN Weijie, YUAN Jinhong, et al. Radar-communication spectrum sharing and integration: Overview and prospect[J]. Journal of Radars, 2021, 10(3): 467–484. doi: 10.12000/JR20113 [3] YU Xianxiang, YAO Xue, YANG Jing, et al. Integrated waveform design for MIMO radar and communication via spatio-spectral modulation[J]. IEEE Transactions on Signal Processing, 2022, 70: 2293–2305. doi: 10.1109/TSP.2022.3170687 [4] LIU Fan, CUI Yuanhao, MASOUROS C, et al. Integrated sensing and communications: Toward dual-functional wireless networks for 6G and beyond[J]. IEEE Journal on Selected Areas in Communications, 2022, 40(6): 1728–1767. doi: 10.1109/JSAC.2022.3156632 [5] TIAN Tuanwei, ZHANG Tianxian, KONG Lingjiang, et al. Transmit/Receive beamforming for MIMO-OFDM based dual-function radar and communication[J]. IEEE Transactions on Vehicular Technology, 2021, 70(5): 4693–4708. doi: 10.1109/TVT.2021.3072094 [6] TIAN Tuanwei, LI Guchong, DENG Hao, et al. Adaptive bit/power allocation with beamforming for dual-function radar-communication[J]. IEEE Wireless Communications Letters, 2022, 11(6): 1186–1190. doi: 10.1109/LWC.2022.3160674 [7] 马丁友, 刘祥, 黄天耀, 等. 雷达通信一体化: 共用波形设计和性能边界[J]. 雷达学报, 2022, 11(2): 198–212. doi: 10.12000/JR21146MA Dingyou, LIU Xiang, HUANG Tianyao, et al. Joint radar and communications: Shared waveform designs and performance bounds[J]. Journal of Radars, 2022, 11(2): 198–212. doi: 10.12000/JR21146 [8] JOHNSTON J, VENTURINO L, GROSSI E, et al. MIMO OFDM dual-function radar-communication under error rate and beampattern constraints[J]. IEEE Journal on Selected Areas in Communications, 2022, 40(6): 1951–1964. doi: 10.1109/JSAC.2022.3156651 [9] KESKIN M F, WYMEERSCH H, and KOIVUNEN V. MIMO-OFDM joint radar-communications: Is ICI friend or foe?[J]. IEEE Journal of Selected Topics in Signal Processing, 2021, 15(6): 1393–1408. doi: 10.1109/JSTSP.2021.3109431 [10] SADDIK G N, SINGH R S, and BROWN E R. Ultra-wideband multifunctional communications/radar system[J]. IEEE Transactions on Microwave Theory and Techniques, 2007, 55(7): 1431–1437. doi: 10.1109/TMTT.2007.900343 [11] GAGLIONE D, CLEMENTE C, ILIOUDIS C V, et al. Waveform design for communicating radar systems using fractional Fourier transform[J]. Digital Signal Processing, 2018, 80: 57–69. doi: 10.1016/j.dsp.2018.05.002 [12] GEMECHU A Y, CUI Guolong, and YU Xianxiang. Spectral-compatible transmit beampattern design with minimum peak sidelobe for narrowband MIMO radar[J]. IEEE Transactions on Vehicular Technology, 2022: 1–12. doi: 10.1109/TVT.2022.3193720 [13] CUI Guolong, DE MAIO A, FARINA A, et al. Radar Waveform Design Based on Optimization Theory[M]. London, U.K.: Institution of Engineering and Technology, 2020. [14] CUI Guolong, LI Hongbin, and RANGASWAMY M. MIMO radar waveform design with constant modulus and similarity constraints[J]. IEEE Transactions on Signal Processing, 2014, 62(2): 343–353. doi: 10.1109/TSP.2013.2288086 [15] WANG Fulai, PANG Chen, ZHOU Jian, et al. Design of complete complementary sequences for ambiguity functions optimization with a PAR constraint[J]. IEEE Geoscience and Remote Sensing Letters, 2021, 19: 3505705. doi: 10.1109/LGRS.2021.3071249 [16] WANG Fulai, PANG Chen, WU Hao, et al. Designing constant modulus complete complementary sequence with high doppler tolerance for simultaneous polarimetric radar[J]. IEEE Signal Processing Letters, 2019, 26(12): 1837–1841. doi: 10.1109/LSP.2019.2949686 [17] FAN Wen, LIANG Junli, YU Guoyang, et al. Minimum local peak sidelobe level waveform design with correlation and/or spectral constraints[J]. Signal Processing, 2020, 171: 107450. doi: 10.1016/j.sigpro.2019.107450 [18] FAN Wen, LIANG Junli, SO H C, et al. Min-max metric for spectrally compatible waveform design via log-exponential smoothing[J]. IEEE Transactions on Signal Processing, 2020, 68: 1075–1090. doi: 10.1109/TSP.2020.2969043 [19] FAN Wen, LIANG Junli, LU Guangshan, et al. Spectrally-agile waveform design for wideband MIMO radar transmit beampattern synthesis via majorization-ADMM[J]. IEEE Transactions on Signal Processing, 2021, 69: 1563–1578. doi: 10.1109/TSP.2021.3052997 [20] SHI Qiao, ZHANG Tianxian, YU Xianxiang, et al. Waveform designs for joint radar-communication systems with OQAM-OFDM[J]. Signal Processing, 2022, 195: 108462. doi: 10.1016/j.sigpro.2022.108462 [21] LIU Fan, ZHOU Longfei, MASOUROS C, et al. Toward dual-functional radar-communication systems: Optimal waveform design[J]. IEEE Transactions on Signal Processing, 2018, 66(16): 4264–4279. doi: 10.1109/TSP.2018.2847648 [22] LIU Xiang, HUANG Tianyao, SHLEZINGER N, et al. Joint transmit beamforming for multiuser MIMO communications and MIMO radar[J]. IEEE Transactions on Signal Processing, 2020, 68: 3929–3944. doi: 10.1109/TSP.2020.3004739 [23] CHEN Li, WANG Zhiqin, DU Ying, et al. Generalized transceiver beamforming for DFRC with MIMO radar and MU-MIMO communication[J]. IEEE Journal on Selected Areas in Communications, 2022, 40(6): 1795–1808. doi: 10.1109/JSAC.2022.3155515 [24] LIU Xiang, HUANG Tianyao, and LIU Yimin. Transmit design for joint MIMO radar and multiuser communications with transmit covariance constraint[J]. IEEE Journal on Selected Areas in Communications, 2022, 40(6): 1932–1950. doi: 10.1109/JSAC.2022.3155512 [25] PEZESHKI A, CALDERBANK A R, MORAN W, et al. Doppler resilient Golay complementary waveforms[J]. IEEE Transactions on Information Theory, 2008, 54(9): 4254–4266. doi: 10.1109/TIT.2008.928292 [26] CHI Yuejie, PEZESHKI A, CALDERBANK R, et al. Range sidelobe suppression in a desired Doppler interval[C]. 2009 International Waveform Diversity and Design Conference, Orlando, FL, USA, 2009: 258–262. [27] WANG Jiahuan, FAN Pingzhi, ZHOU Zhengchun, et al. Quasi-orthogonal Z-complementary pairs and their applications in fully polarimetric radar systems[J]. IEEE Transactions on Information Theory, 2021, 67(7): 4876–4890. doi: 10.1109/TIT.2021.3063764 [28] WU Zhongjie, WANG Chenxu, ZHOU Zhiquan, et al. Design of (quasi) complementary waveform with Doppler resilience for range sidelobe suppression[C]. 2020 IEEE Radar Conference, Florence, Italy, 2020: 1–6. [29] 崔国龙, 余显祥, 杨婧, 等. 认知雷达波形优化设计方法综述[J]. 雷达学报, 2019, 8(5): 537–557. doi: 10.12000/JR19072CUI Guolong, YU Xianxiang, YANG Jing, et al. An overview of waveform optimization methods for cognitive radar[J]. Journal of Radars, 2019, 8(5): 537–557. doi: 10.12000/JR19072 [30] SONG Junxiao, BABU P, and PALOMAR D P. Sequence design to minimize the weighted integrated and peak sidelobe levels[J]. IEEE Transactions on Signal Processing, 2016, 64(8): 2051–2064. doi: 10.1109/TSP.2015.2510982 [31] CUI Guolong, FU Yue, YU Xianxiang, et al. Local ambiguity function shaping via unimodular sequence design[J]. IEEE Signal Processing Letters, 2017, 24(7): 977–981. doi: 10.1109/LSP.2017.2700396 [32] FAN Pingzhi, SUEHIRO N, KUROYANAGI N, et al. Class of binary sequences with zero correlation zone[J]. Electronics Letters, 1999, 35(10): 777–779. doi: 10.1049/el:19990567 [33] XIAO Zhiqiang and ZENG Yong. Waveform design and performance analysis for full-duplex integrated sensing and communication[J]. IEEE Journal on Selected Areas in Communications, 2022, 40(6): 1823–1837. doi: 10.1109/JSAC.2022.3155509 [34] MOLER C B. Numerical Computing with MATLAB[M]. Philadelphia: Society for Industrial and Applied Mathematics, 2004: 2–3. [35] YU Xianxiang, FAN Tao, QIU Hui, et al. Constrained transceiver design with expanded mainlobe for range sidelobe reduction[J]. IEEE Transactions on Aerospace and Electronic Systems, 2022, 58(5): 4803–4813. doi: 10.1109/TAES.2022.3163120 -
图(6) / 表(3)
计量
- 文章访问数: 1719
- HTML全文浏览量: 1171
- PDF下载量: 340
- 被引次数: 0
