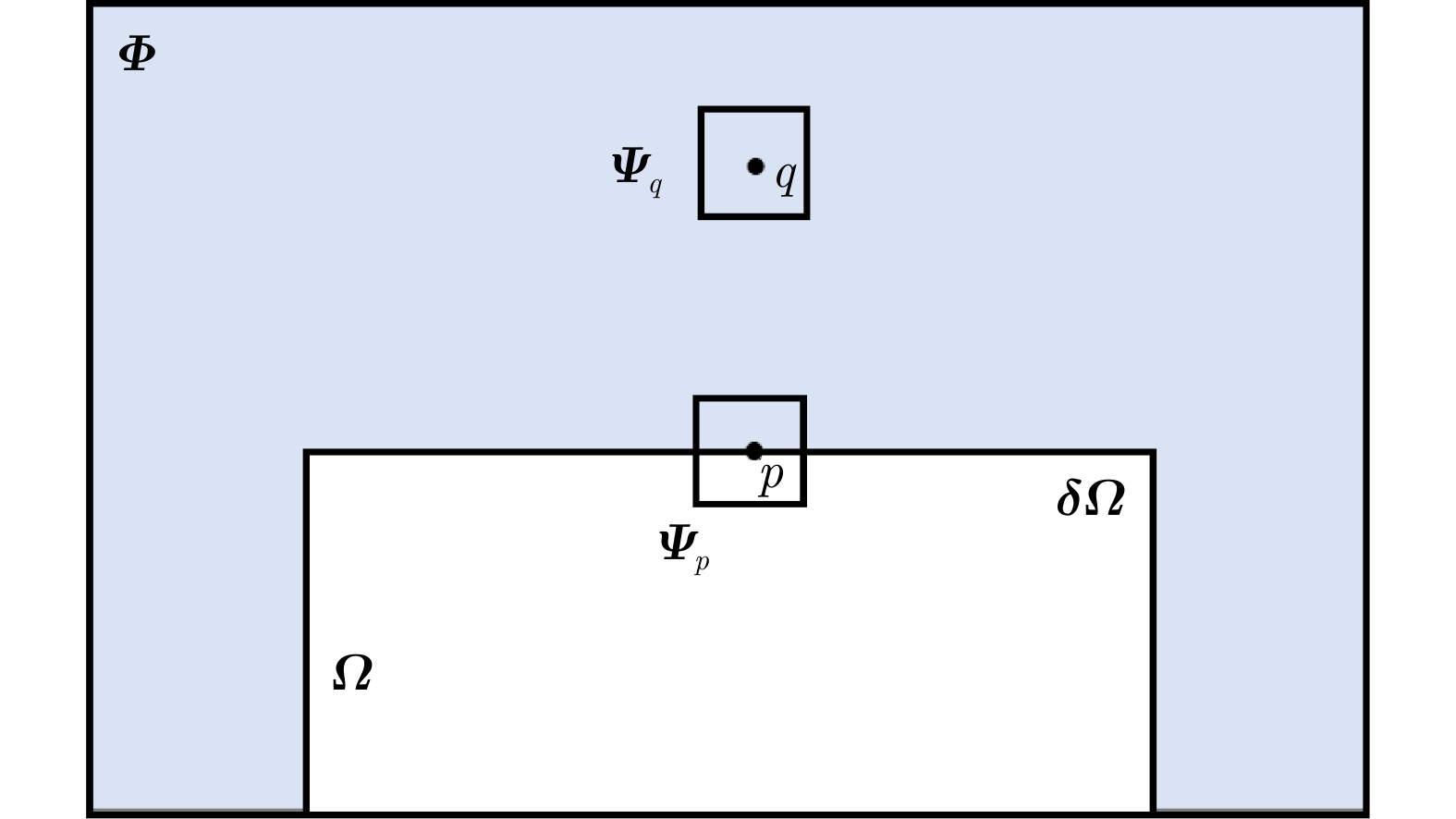
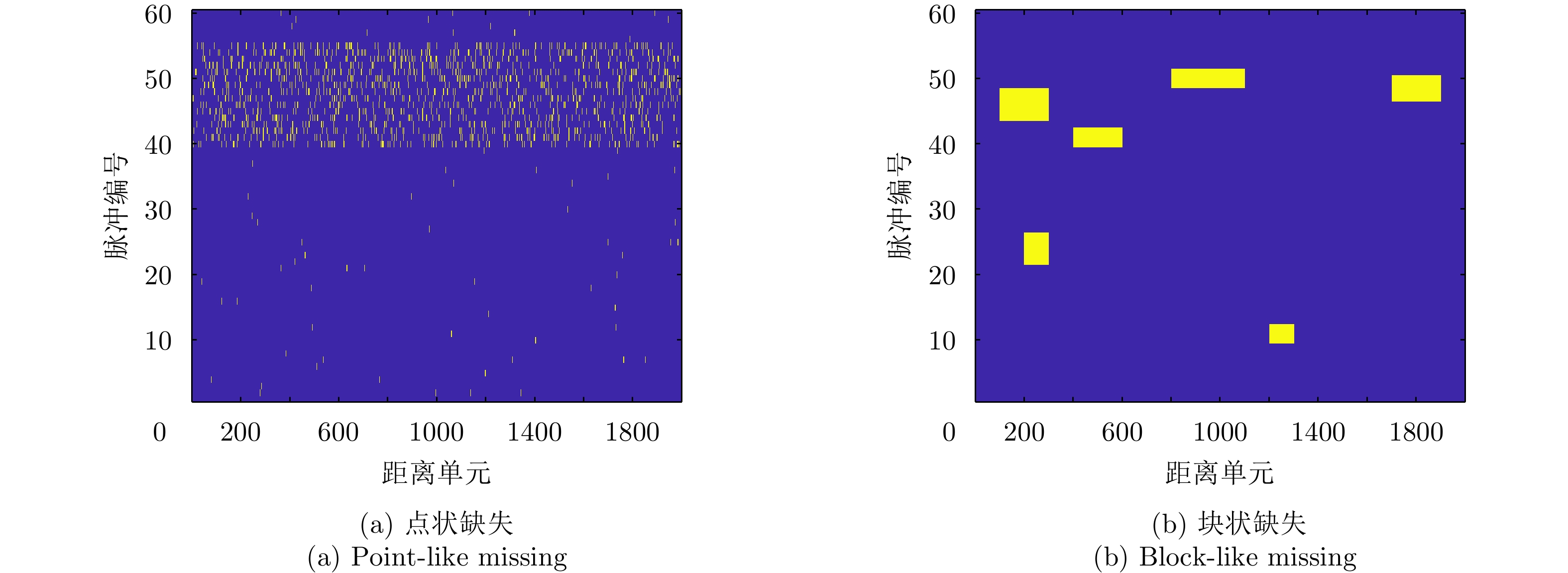
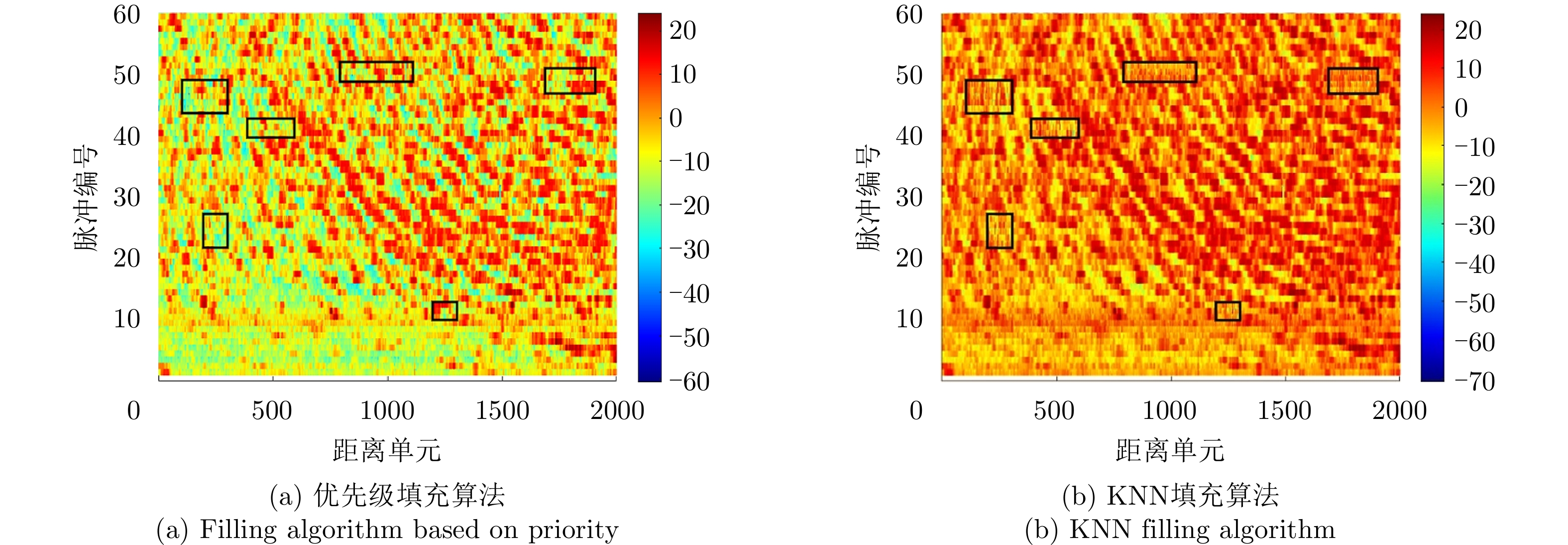
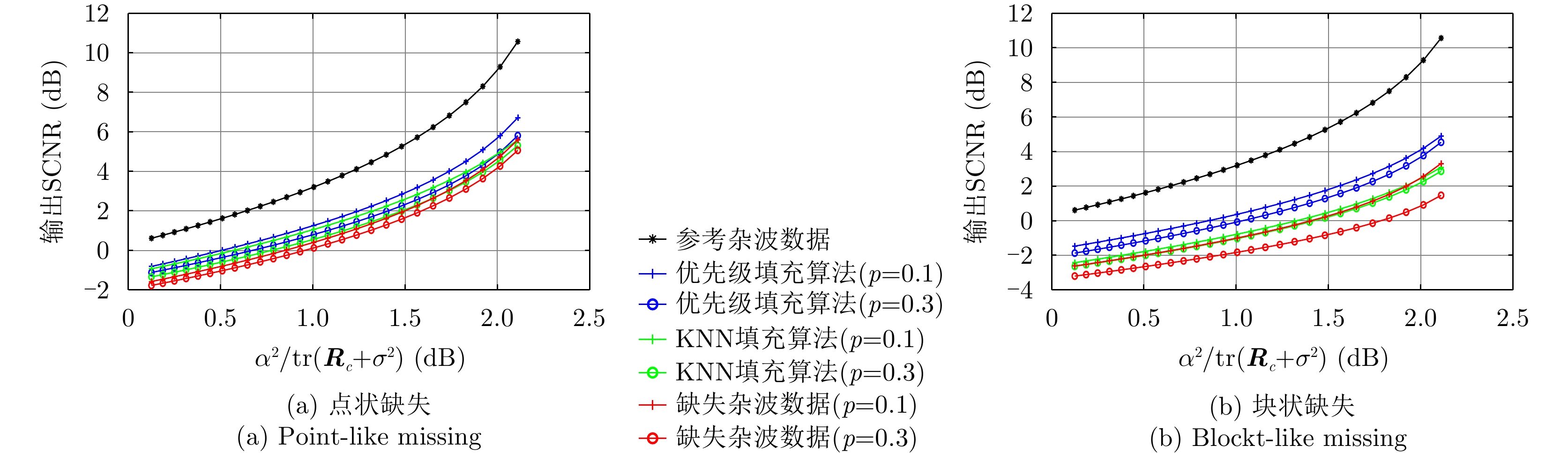
| [1] |
TANG Bo and TANG Jun. Joint design of transmit waveforms and receive filters for MIMO radar space-time adaptive processing[J]. IEEE Transactions on Signal Processing, 2016, 64(18): 4707–4722. doi: 10.1109/TSP.2016.2569431 |
| [2] |
TANG Bo, TUCK J, and STOICA P. Polyphase waveform design for MIMO radar space time adaptive processing[J]. IEEE Transactions on Signal Processing, 2020, 68: 2143–2154. doi: 10.1109/TSP.2020.2983833 |
| [3] |
YU Xianxiang, CUI Guolong, YANG Jing, et al. Wideband MIMO radar waveform design[J]. IEEE Transactions on Signal Processing, 2019, 67(13): 3487–3501. doi: 10.1109/TSP.2019.2916732 |
| [4] |
WU Linlong and PALOMAR D P. Radar Waveform Design Via the Majorization-Minimization Framework[M]. CUI Guolong, DE MAIO A, FARINA A, et al. Radar Waveform Design Based on Optimization Theory. London: The Institution of Engineering and Technology, 2020: 185–220.
|
| [5] |
O’ROURKE S M, SETLUR P, RANGASWAMY M, et al. Quadratic semidefinite programming for waveform-constrained joint filter-signal design in STAP[J]. IEEE Transactions on Signal Processing, 2020, 68: 1744–1759. doi: 10.1109/TSP.2020.2977271 |
| [6] |
TANG Bo, NAGHSH M M, and TANG Jun. Relative entropy-based waveform design for MIMO radar detection in the presence of clutter and interference[J]. IEEE Transactions on Signal Processing, 2015, 63(14): 3783–3796. doi: 10.1109/TSP.2015.2423257 |
| [7] |
WANG Yikai, XIA Wei, HE Zishu, et al. Polarimetric detection in compound Gaussian clutter with Kronecker structured covariance matrix[J]. IEEE Transactions on Signal Processing, 2017, 65(17): 4562–4576. doi: 10.1109/TSP.2017.2716912 |
| [8] |
SUN Guohao, HE Zishu, TONG Jun, et al. Mutual information-based waveform design for MIMO radar space-time adaptive processing[J]. IEEE Transactions on Geoscience and Remote Sensing, 2021, 59(4): 2909–2921. doi: 10.1109/TGRS.2020.3008320 |
| [9] |
崔国龙, 余显祥, 杨婧, 等. 认知雷达波形优化设计方法综述[J]. 雷达学报, 2019, 8(5): 537–557. doi: 10.12000/JR19072CUI Guolong, YU Xianxiang, YANG Jing, et al. An overview of waveform optimization methods for cognitive radar[J]. Journal of Radars, 2019, 8(5): 537–557. doi: 10.12000/JR19072 |
| [10] |
王珽, 赵拥军, 胡涛. 机载MIMO雷达空时自适应处理技术研究进展[J]. 雷达学报, 2015, 4(2): 136–148. doi: 10.12000/JR14091WANG Ting, ZHAO Yongjun, and HU Tao. Overview of space-time adaptive processing for airborne MIMO radar[J]. Journal of Radars, 2015, 4(2): 136–148. doi: 10.12000/JR14091 |
| [11] |
AUBRY A, DE MAIO A, MARANO S, et al. Structured covariance matrix estimation with missing-(complex) data for radar applications via expectation-maximization[J]. IEEE Transactions on Signal Processing, 2021, 69: 5920–5934. doi: 10.1109/TSP.2021.3111587 |
| [12] |
HIPPERT-FERRER A, EL KORSO M N, BRELOY A, et al. Robust low-rank covariance matrix estimation with a general pattern of missing values[J]. Signal Processing, 2022, 195: 108460. doi: 10.1016/j.sigpro.2022.108460 |
| [13] |
PAVEZ E and ORTEGA A. Covariance matrix estimation with non uniform and data dependent missing observations[J]. IEEE Transactions on Information Theory, 2021, 67(2): 1201–1215. doi: 10.1109/TIT.2020.3039118 |
| [14] |
ZHANG Ying, LIE J P, NG B P, et al. Robust minimum ℓ1-norm adaptive beamformer against intermittent sensor failure and steering vector error[J]. IEEE Transactions on Antennas and Propagation, 2010, 58(5): 1796–1801. doi: 10.1109/TAP.2010.2044353 |
| [15] |
XIONG Can, XIAO Gaobiao, HOU Yibei, et al. A compressed sensing-based element failure diagnosis method for phased array antenna during beam steering[J]. IEEE Antennas and Wireless Propagation Letters, 2019, 18(9): 1756–1760. doi: 10.1109/LAWP.2019.2929353 |
| [16] |
GAO Yongchan, LIAO Guisheng, and LIU Weijian. High-resolution radar detection in interference and nonhomogeneous noise[J]. IEEE Signal Processing Letters, 2016, 23(10): 1359–1363. doi: 10.1109/LSP.2016.2597738 |
| [17] |
LIM D, GIANELLI C D, and LI Jian. Automatic target recognition in missing data cases[J]. IEEE Aerospace and Electronic Systems Magazine, 2017, 32(7): 40–49. doi: 10.1109/MAES.2017.150273 |
| [18] |
SHEN Lei, LIU Zhiwen, XU Yougen, et al. Robust polarimetric adaptive detector against target steering matrix mismatch[J]. IEEE Transactions on Aerospace and Electronic Systems, 2020, 56(1): 442–455. doi: 10.1109/TAES.2019.2916708 |
| [19] |
LOUNICI K. High-dimensional covariance matrix estimation with missing observations[J]. Bernoulli, 2014, 20(3): 1029–1058. doi: 10.3150/12-BEJ487 |
| [20] |
LIU Junyan and PALOMAR D P. Regularized robust estimation of mean and covariance matrix for incomplete data[J]. Signal Processing, 2019, 165: 278–291. doi: 10.1016/j.sigpro.2019.07.009 |
| [21] |
XU Danlei, DU Lan, LIU Hongwei, et al. Compressive sensing of stepped-frequency radar based on transfer learning[J]. IEEE Transactions on Signal Processing, 2015, 63(12): 3076–3087. doi: 10.1109/TSP.2015.2421473 |
| [22] |
LV Qinzhe, QUAN Yinghui, WEI Feng, et al. Radar deception jamming recognition based on weighted ensemble CNN with transfer learning[J]. IEEE Transactions on Geoscience and Remote Sensing, 2022, 60: 5107511. doi: 10.1109/TGRS.2021.3129645 |
| [23] |
JIANG Wei, HAIMOVICH A M, and SIMEONE O. Joint design of radar waveform and detector via end-to-end learning with waveform constraints[J]. IEEE Transactions on Aerospace and Electronic Systems, 2022, 58(1): 552–567. doi: 10.1109/TAES.2021.3103560 |
| [24] |
LI Jian, GUERCI J R, and XU Luzhou. Signal waveform’s optimal-under-restriction design for active sensing[J]. IEEE Signal Processing Letters, 2006, 13(9): 565–568. doi: 10.1109/LSP.2006.874465 |
| [25] |
BELLMAN R. A Markovian decision process[J]. Journal of Mathematics and Mechanics, 1957, 6(5): 679–684.
|
| [26] |
CRIMINISI A, PEREZ P, and TOYAMA K. Region filling and object removal by exemplar-based image inpainting[J]. IEEE Transactions on Image Processing, 2004, 13(9): 1200–1212. doi: 10.1109/TIP.2004.833105 |
| [27] |
|




 Submit Manuscript
Submit Manuscript Peer Review
Peer Review Editor Work
Editor Work





 DownLoad:
DownLoad: