作者中心
专家审稿
责编办公
编辑办公
Anti-interrupted Sampling Repeater Jamming Method in the Waveform Domain before Matched Filtering
-
摘要: 间歇采样转发干扰属于一类脉内相干欺骗干扰，其运用欠采样原理，在距离维上产生多个虚假的目标峰，从而干扰真实目标的检测与跟踪。为了解决这一问题，该文提出了一种基于波形域的匹配滤波前抗间歇采样转发干扰方法。首先，考虑到间歇采样转发干扰的部分匹配特性，该文在匹配滤波过程中引入了扩展域，即波形域，以研究干扰信号与真实目标回波信号元素的局部特征，并在每个波形域上定义了自适应的阈值函数。其次，引入卡尔曼滤波对波形域信号进行状态估计，通过自适应阈值检测筛选出波形域信号中的有效积分元素与无效积分元素，并建立关于有效积分元素的估计状态空间。最后，在抑制波形域信号中的无效积分元素的同时，从有效积分元素的估计状态空间中补充相应长度的积分元素，保留剩余的有效积分元素，通过积分得到不含虚假目标的距离像结果。该文所提方法不倚赖于任何干扰机参数等先验信息，即可有效抑制间歇采样转发干扰。仿真实验表明，与传统方法相比，该文方法实现的抗间歇采样转发干扰性能更优。Abstract: Interrupted Sampling Repeater Jamming (ISRJ) falls within the category of intrapulse coherent deception interference. ISRJ employs the principle of undersampling to engender multiple spurious target peaks on the range profile, thereby disrupting the detection and tracking of genuine targets. To address this challenge, this study introduces a novel method grounded in the waveform domain to mitigate ISRJ before matched filtering. First, considering the partial matching attributes of ISRJ, an expanded domain, specifically the waveform domain, is incorporated into the matched filtering. This augmentation enables the investigation of local features within the interference signals and components of authentic target echo signals. Moreover, adaptive threshold functions are defined for each waveform domain. Subsequently, the introduction of the Kalman filter enables the state estimation of waveform domain signals. Additionally, valid and invalid integral elements are discriminated within the waveform domain signals via adaptive threshold detection, and a state space estimation is formulated, specifically concerning the valid integral elements. In conclusion, by suppressing the invalid integral elements within the waveform domain signals, the proposed approach simultaneously supplements the estimated state space of valid integral elements with their corresponding length components. This preservation of residual valid integral elements, coupled with integration operation, yields a range profile outcome devoid of deceptive interference artifacts. Importantly, the approach proposed herein operates independently of any prior information regarding the interference device parameters, thereby substantially reducing the effect of ISRJ. Simulation experiments illustrate that, in comparison with traditional methodologies, the method proposed in this study exhibits remarkably superior resistance against the ISRJ interference challenges.
-
图 1 时域、波形域和距离域的信号表征形式(线性调频信号为例)
Figure 1. Signal representation in time, waveform, and range domains (using linear frequency modulated signal as an example)
图 2
$ {t}_{1} $ ,$ {t}_{2} $ ,$ {t}_{3} $ 时刻下，使用波形域的IMM-KF方法进行估计的结果以及相应的波形域标记结果Figure 2. Results of estimation using waveform domain IMM-KF method at
$ {t}_{1} $ ,$ {t}_{2} $ , and$ {t}_{3} $ ,along with corresponding waveform domain labels
图 4 干扰场景下不同方法的输出结果
Figure 4. Output results of different methods in the presence of interference acenarios
图 5 不同输入信噪比和信干比条件下本文方法匹配滤波输出平均峰值
Figure 5. Average peak values of matched filter output for various input SNRs and SJRs using the proposed method
图 6 不同间歇采样周期和采样占空比条件下的干扰平均峰值电平变化曲线
Figure 6. Curves of average peak interference levels under various intermittent sampling periods and sampling duty cycles
图 7 重复转发与循环转发干扰模式下波形域抗干扰输出结果
Figure 7. Waveform domain anti-Jamming output results under repetitive retransmission and cyclic retransmission interference modes
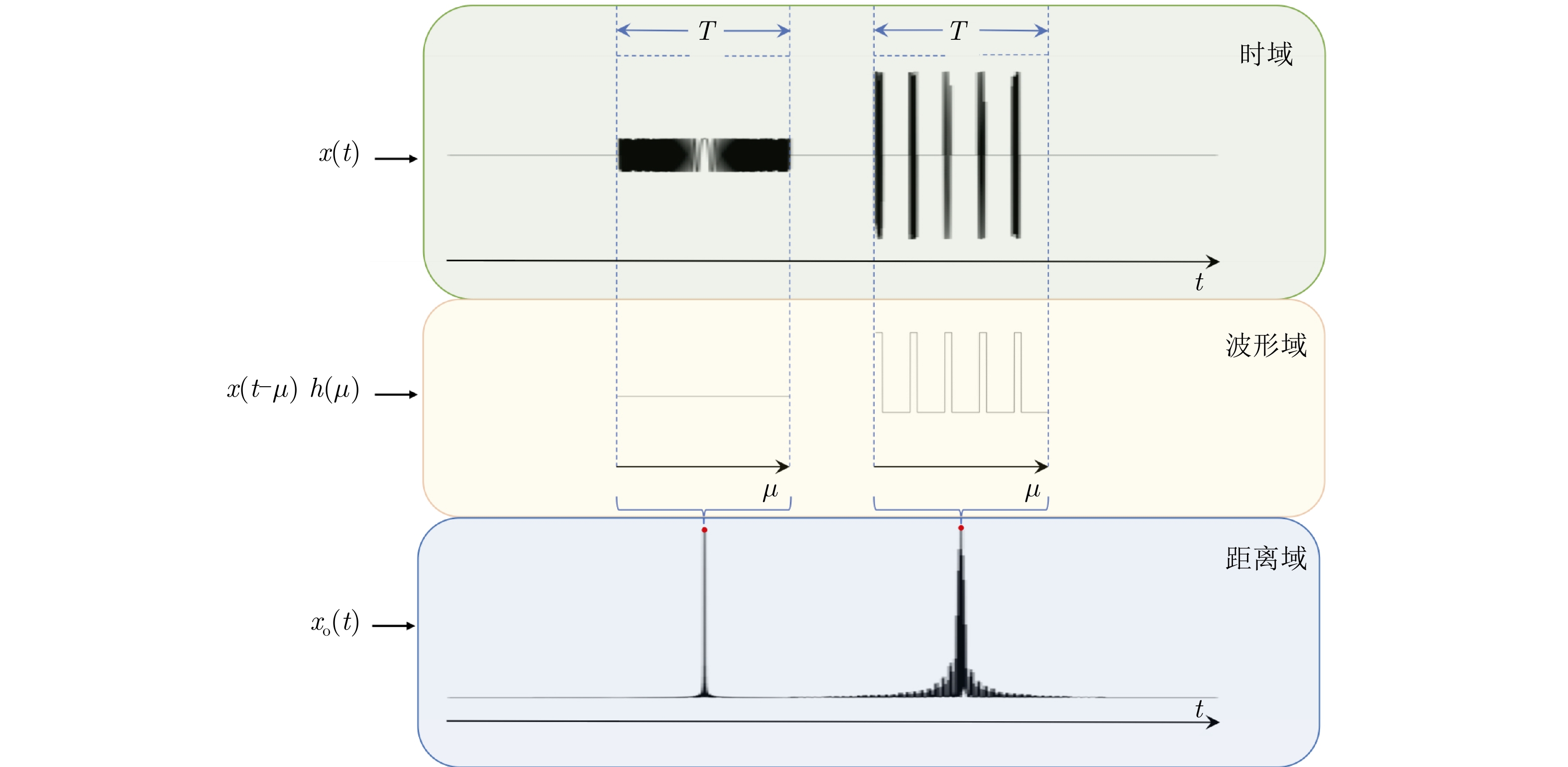
表 1 不同信号表征形式的比较与联系
Table 1. Comparison and correlation of different signal representation forms.
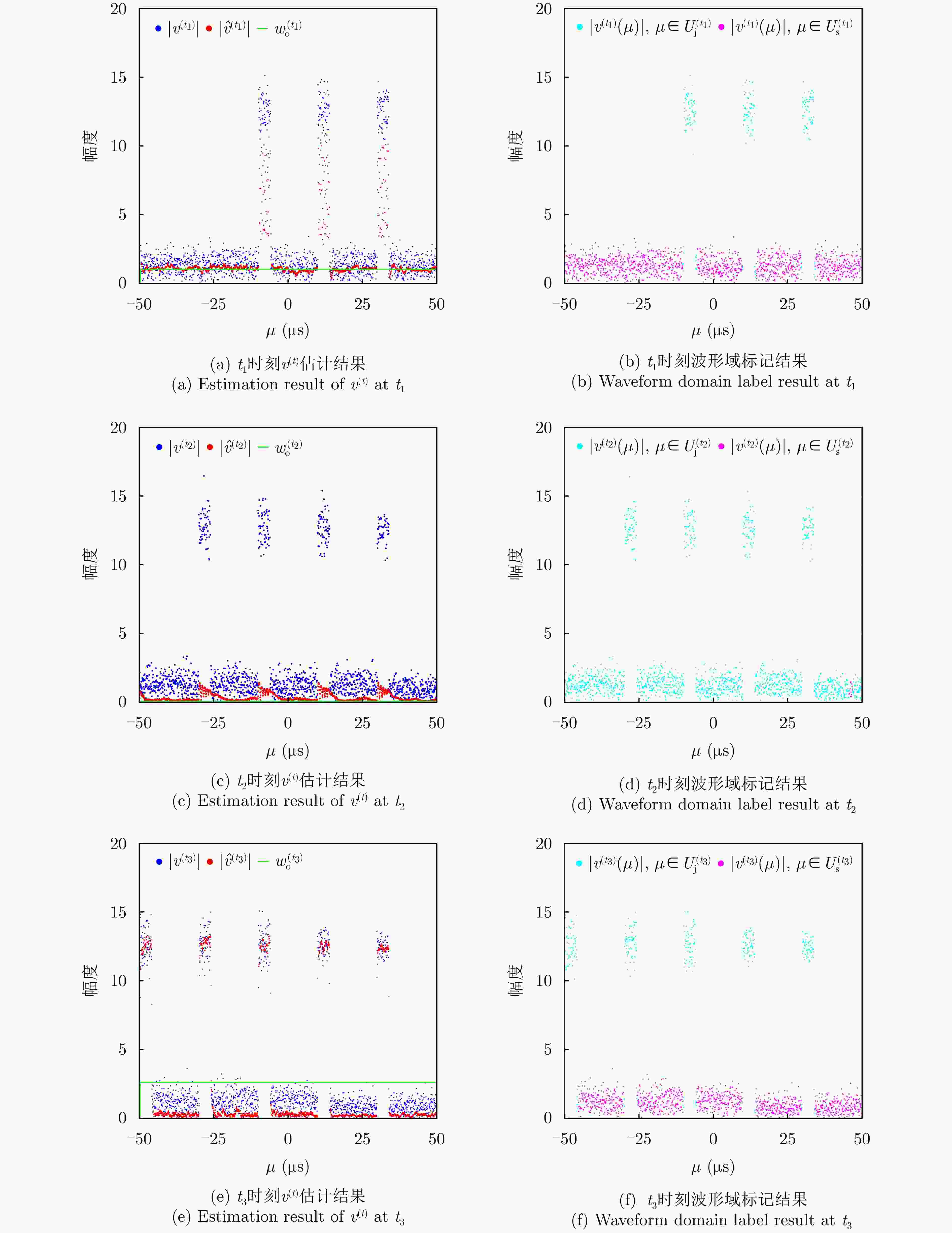
信号表征形式 特点 时域 1. 区间长度无限长。2. 表征信号的时域波形。 距离域 1. 区间长度是信号的脉冲重复周期。2. 表征信号的匹配滤波结果，即一维距离像。3. 距离域时刻与时域快时间一一对应。 波形域 1. 区间长度为无限长。2. 表征$ {\upsilon }^{\left(t\right)}\left(\mu \right) $的处理过程。3. 区间与时域快时间和距离域时刻一一对应，
即每个时刻t都对应着一个无限长的波形域，可视为每个时刻下的拓展子空间。
下载: 导出CSV
表 2 干扰场景的仿真参数
Table 2. Simulation parameters of the jamming scene
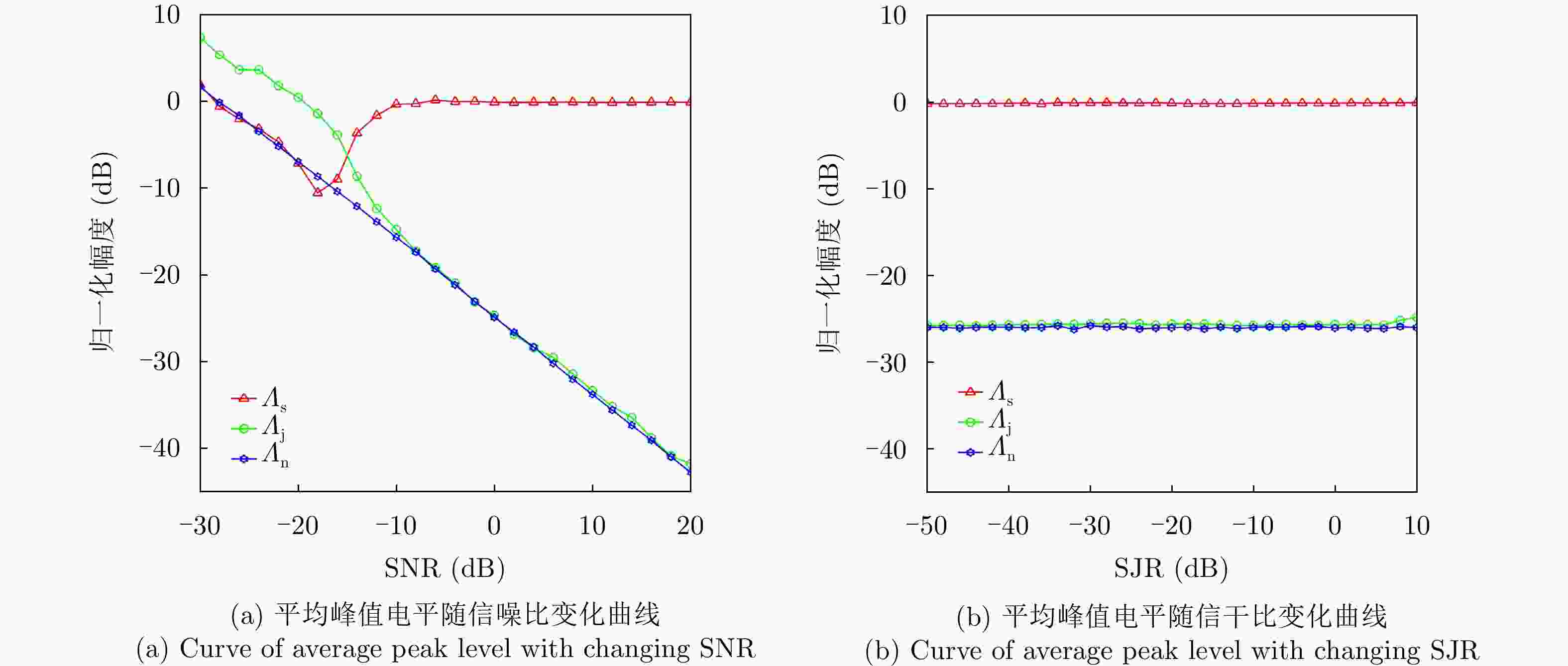
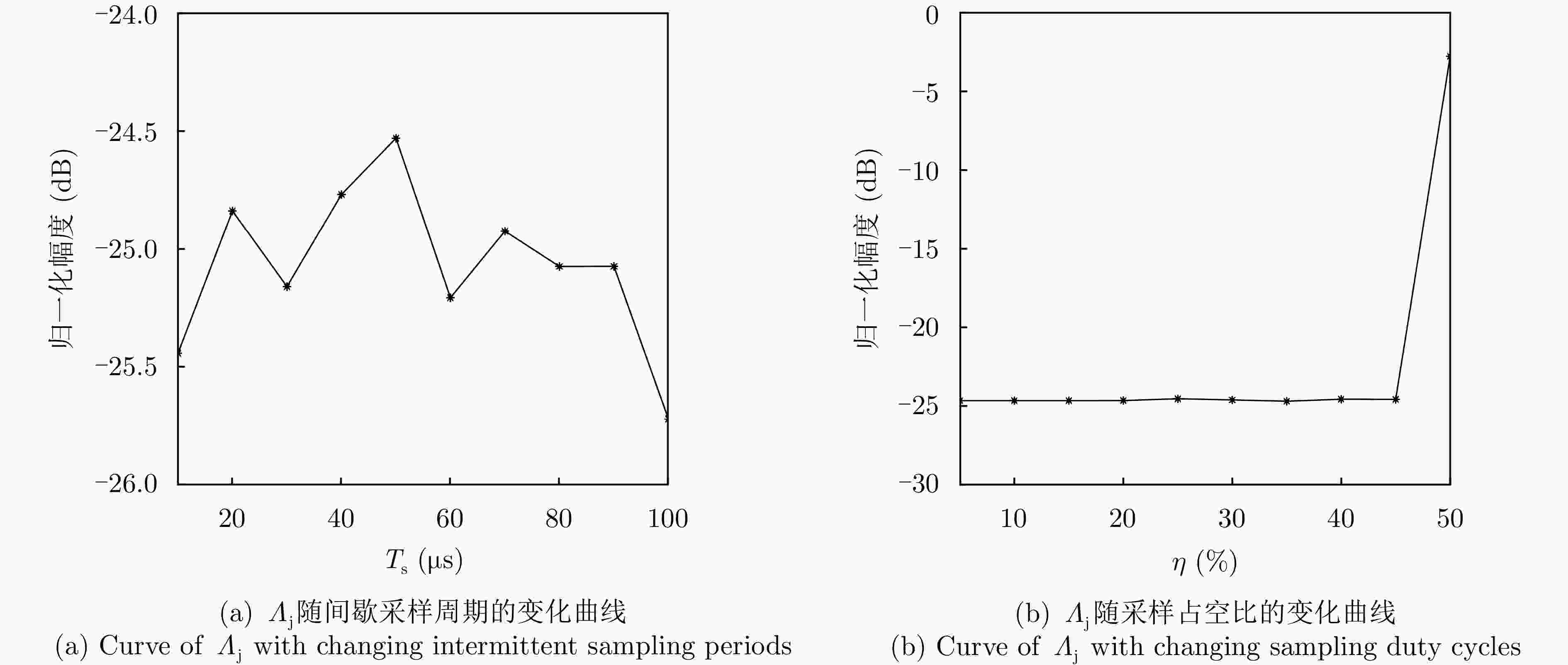
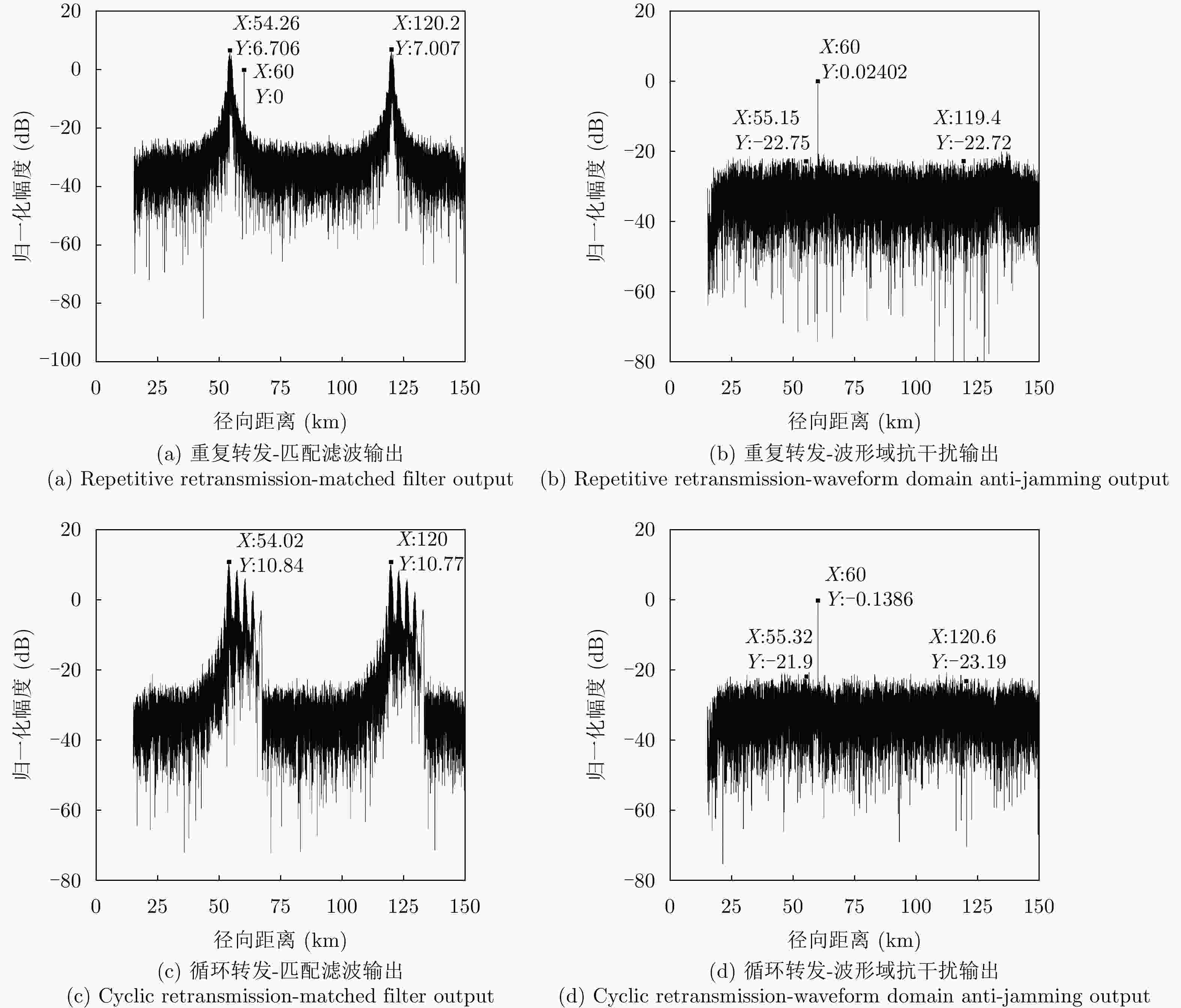
参数 数值 载频$ {f}_{0}\left(\mathrm{G}\mathrm{H}\mathrm{z}\right) $ 2 脉冲重复频率$ \mathrm{P}\mathrm{R}\mathrm{F}\left(\mathrm{k}\mathrm{H}\mathrm{z}\right) $ 1 目标径向距离$ {d}_{0}\left(\mathrm{k}\mathrm{m}\right) $ $ 60 $ 目标径向速度$ \vartheta_0(\mathrm{m}/\mathrm{s}) $ $ 300 $ 干扰径向距离$ {d}_{1}\left(\mathrm{k}\mathrm{m}\right),{d}_{2}\left(\mathrm{k}\mathrm{m}\right) $ $ \mathrm{54,20} $ 干扰径向速度$ {\vartheta }_{1}(\mathrm{m}/\mathrm{s}),{\vartheta }_{2}(\mathrm{m}/\mathrm{s}) $ $ -300,\mathrm{ }300 $ 输入信噪比$ \mathrm{S}\mathrm{N}\mathrm{R}\left(\mathrm{d}\mathrm{B}\right) $ 0 输入信干比$ \mathrm{S}\mathrm{J}\mathrm{R}\left(\mathrm{d}\mathrm{B}\right) $ $ -20 $
下载: 导出CSV
-
[1] ROOME S J. Digital radio frequency memory[J]. Electronics & Communication Engineering Journal, 1990, 2(4): 147–153. [2] 王雪松, 刘建成, 张文明, 等. 间歇采样转发干扰的数学原理[J]. 中国科学E辑 信息科学, 2006, 36(8): 891–901. doi: 10.1007/s11432-007-2017-y.WANG Xuesong, LIU Jiancheng, ZHANG Wenming, et al. Mathematic principles of interrupted-sampling repeater jamming (ISRJ)[J]. Science in China Series E: Information Sciences, 2006, 36(8): 891–901. doi: 10.1007/s11432-007-2017-y. [3] 沈阳, 陈永光, 李修和, 等. 多基地雷达反隐身分布式检测融合算法研究[J]. 电子学报, 2007, 35(3): 506–510. doi: 10.3321/j.issn:0372-2112.2007.03.025SHEN Yang, CHEN Yongguang, LI Xiuhe, et al. Study on fusion arithmetic of multi radar distributed detection system against stealthy targets[J]. Acta Electronica Sinica, 2007, 35(3): 506–510. doi: 10.3321/j.issn:0372-2112.2007.03.025 [4] 徐裴为. 随队支援干扰效果分析[D]. [硕士论文], 电子科技大学, 2012.XU Peiwei. Analysis of the effectiveness of Escort Jamming (ESJ)[D]. [Master dissertation], University of Electronic Science and Technology of China, 2012. [5] GONG Shixian, WEI Xizhang, and LI Xiang. ECCM scheme against interrupted sampling repeater jammer based on time-frequency analysis[J]. Journal of Systems Engineering and Electronics, 2014, 25(6): 996–1003. doi: 10.1109/JSEE.2014.00114. [6] XIONG Wei, ZHANG Gong, and LIU Wenbo. Efficient filter design against interrupted sampling repeater jamming for wideband radar[J]. EURASIP Journal on Advances in Signal Processing, 2017, 2017(1): 9. doi: 10.1186/s13634-017-0446-3 [7] YUAN Hui, WANG Chunyang, LI Xin, et al. A method against interrupted-sampling repeater jamming based on energy function detection and band-pass filtering[J]. International Journal of Antennas and Propagation, 2017, 2017: 6759169. doi: 10.1155/2017/6759169 [8] CHEN Jian, WU Wenzhen, XU Shiyou, et al. A band pass filter design against interrupted-sampling repeater jamming based on time-frequency analysis[J]. IET Radar, Sonar & Navigation, 2019, 13(10): 1646–1654. doi: 10.1049/iet-rsn.2018.5658 [9] 周畅, 汤子跃, 朱振波, 等. 抗间歇采样转发干扰的波形设计方法[J]. 电子与信息学报, 2018, 40(9): 2198–2205. doi: 10.11999/JEIT171236ZHOU Chang, TANG Ziyue, ZHU Zhenbo, et al. Anti-interrupted sampling repeater jamming waveform design method[J]. Journal of Electronics & Information Technology, 2018, 40(9): 2198–2205. doi: 10.11999/JEIT171236 [10] 周畅, 汤子跃, 余方利, 等. 基于脉内正交的抗间歇采样转发干扰方法[J]. 系统工程与电子技术, 2017, 39(2): 269–276. doi: 10.3969/j.issn.1001-506X.2017.02.06ZHOU Chang, TANG Ziyue, YU Fangli, et al. Anti intermittent sampling repeater jamming method based on intrapulse orthogonality[J]. Systems Engineering and Electronics, 2017, 39(2): 269–276. doi: 10.3969/j.issn.1001-506X.2017.02.06 [11] 周凯, 李德鑫, 粟毅, 等. 基于雷达发射波形和非匹配滤波联合设计的间歇采样转发干扰抑制方法[J]. 电子与信息学报, 2021, 43(7): 1939–1946. doi: 10.11999/JEIT200299ZHOU Kai, LI Dexin, SU Yi, et al. Joint transmitted waveform and mismatched filter design against interrupted-sampling repeater jamming[J]. Journal of Electronics & Information Technology, 2021, 43(7): 1939–1946. doi: 10.11999/JEIT200299 [12] 王福来, 庞晨, 殷加鹏, 等. 一种多普勒容忍的抗间歇采样转发干扰恒模互补波形和接收滤波器联合设计方法[J]. 雷达学报, 2022, 11(2): 278–288. doi: 10.12000/JR22020WANG Fulai, PANG Chen, YIN Jiapeng, et al. Joint design of Doppler-tolerant complementary sequences and receiving filters against interrupted sampling repeater jamming[J]. Journal of Radars, 2022, 11(2): 278–288. doi: 10.12000/JR22020 [13] MENG Yunyun, YU Lei, WEI Yinsheng, et al. A novel parameter estimation method of interrupted sampling repeater jamming[C]. 2019 IEEE International Conference on Signal, Information and Data Processing (ICSIDP), Chongqing, China, 2019: 1–5. [14] WANG Chaoyu, WANG Xinhai, and ZHANG Jindong. A novel parameter estimation method for ISRJ[C]. The 2nd International Conference on Electronic Materials and Information Engineering, Hangzhou, China, 2022: 1–4. [15] WANG Chaoyu, HU Wanwan, GENG Zhe, et al. Parameter estimation for interrupted sampling repeater jamming based on ADMM[J]. Sensors, 2021, 21(24): 8277. doi: 10.3390/s21248277 [16] WEI Zhenhua, LIU Zhen, PENG Bo, et al. ECCM Scheme against interrupted sampling repeater jammer based on parameter-adjusted waveform design[J]. Sensors, 2018, 18(4): 1141. [17] ZHOU Chao, LIU Quanhua, and CHEN Xinliang. Parameter estimation and suppression for DRFM-based interrupted sampling repeater jammer[J]. IET Radar, Sonar & Navigation, 2018, 12(1): 56–63. doi: 10.1049/iet-rsn.2017.0114 [18] CHEN Jian, XU Shiyou, ZOU Jiangwei, et al. Interrupted- sampling repeater jamming suppression based on stacked bidirectional gated recurrent unit network and infinite training[J]. IEEE Access, 2019, 7: 107428–107437. doi: 10.1109/ACCESS.2019.2932793 [19] WU Wenzhen, ZOU Jiangwei, CHEN Jian, et al. False-target recognition against interrupted-sampling repeater jamming based on integration decomposition[J]. IEEE Transactions on Aerospace and Electronic Systems, 2021, 57(5): 2979–2991. doi: 10.1109/TAES.2021.3068443 [20] DAI Huahua, ZHAO Yingxiao, SU Hanning, et al. Research on an intra-pulse orthogonal waveform and methods resisting interrupted-sampling repeater jamming within the same frequency band[J]. Remote Sensing, 2023, 15(14): 3673. doi: 10.3390/rs15143673 [21] BLACKMAN S S. Multiple hypothesis tracking for multiple target tracking[J]. IEEE Aerospace and Electronic Systems Magazine, 2004, 19(1): 5–18. doi: 10.1109/MAES.2004.1263228 -
计量
- 文章访问数: 593
- HTML全文浏览量: 293
- PDF下载量: 188
- 被引次数: 0
