作者中心
专家审稿
责编办公
编辑办公
Monopulse Forward-looking Imaging Based on Doppler Estimation Using Fast Iterative Interpolated Beamforming Algorithm
-
摘要: 在单脉冲前视成像技术中，同分辨单元内多目标的辨识一直是单脉冲雷达的研究热点。尽管多普勒处理可以提高对前斜视多目标的分辨能力，但在真实目标数量未知、强点目标能量泄露的情况下，多普勒频率的精确估计面临巨大挑战。针对以上问题，该文在单脉冲前视成像中引入具有目标个数估计和单快拍处理能力的快速迭代插值波束形成(FIIB)算法，结合信息论准则估计目标个数，实现对多普勒频率的无偏估计。点目标仿真数值分析结果显示，FIIB对于同分辨单元内的目标数估计和参数估计性能优于调频Z变换(CZT)算法，能实现对±5°外点目标的准确估计。场景仿真和实测数据成像结果表明基于FIIB的单脉冲前视成像算法聚焦能力强，图像对比度更高，并能有效抑制背景杂波。
-
关键词:
- 单脉冲雷达 /
- 前视成像 /
- 多普勒频率估计 /
- 快速迭代插值波束形成算法
Abstract: Distinguishing multiple targets in the same resolution cell is an important and challenging task in the forward-looking imaging process of monopulse radar. Although Doppler processing can improve the recognition performance for multiple targets at high squint angles, the precise estimation of Doppler frequency remains challenging under conditions with unknown target numbers and energy leakage from strong point targets. To address these issues, this paper proposes a Fast Iterative Interpolated Beamforming (FIIB) algorithm with model order estimation and single snapshot processing for monopulse forward-looking imaging, which combines information theory to unbiasedly estimate the number of targets and Doppler frequencies. The simulation results show the superiority of the proposed FIIB algorithm over the Chirp-Z Transform (CZT) algorithm for estimating target numbers and Doppler frequencies within the same resolution cell in the presence of multiple point targets. In addition, the proposed FIIB algorithm can accurately estimate point targets beyond a ±5° azimuth angle in monopulse angle measurement tasks. Real-data experiments also reveal that FIIB-based monopulse forward-looking imaging has high focusing capability and imaging contrast and can effectively suppress background clutter. -
图 2 强点目标仿真结果(
${W_1}/{W_2} = 20\;{\text{dB}}$ )Figure 2. Simulation result of strong point targets (
${W_1}/{W_2} = 20\;{\text{dB}}$ )
图 3 相邻点目标仿真结果 (
$\Delta f = 1/N$ )Figure 3. Simulation result of neighboring point targets (
$\Delta f = 1/N$ )
图 4 多点目标仿真结果(
$L = 10$ )Figure 4. Simulation result of multiple point targets (
$L = 10$ )
图 5 不同迭代次数下同多普勒单元内两个目标的FIIB仿真结果
Figure 5. Simulation results of FIIB for two targets situated in a Doppler bin by different iterations
图 7 距离向1700 m处目标方位向剖面图
Figure 7. Azimuthal contour plots for point targets at the range cell 1700 m
图 8 仿真场景前视成像效果对比
Figure 8. Comparison of simulation results in forward-looking imaging
图 9 实测数据前视成像效果对比
Figure 9. Comparison of experimental results in forward-looking imaging
初始化： 令 ${\hat f_l} = 0$, ${\hat A_l} = 0$, $l = 1,2, \cdots ,L$ 设 $q = 0$ ${\boldsymbol{X}} = {\text{FFT}}({\boldsymbol{x}},N)$ 迭代(Q次)： 对于 $l = 1,2, \cdots ,L$ IF $q = 1$(粗估计)： $\tilde X(k) = X(k) - \displaystyle\sum \limits_{i = 1,i \ne l}^L {\hat A_i}{\hat S_i}(k),k = 0,1, \cdots ,N - 1$ (i) $ {\hat m_l} =\arg \mathop{\max}\limits_{0 \le k \le N - 1 } {\left| {\tilde X(k)} \right|^2} $ (ii) ${\hat f_l} = \dfrac{1}{N}{\hat m_l}$ (iii) END IF (精估计):
$ {\tilde X_p}({\hat f_l}) = {X_p}({\hat f_l}) - \displaystyle\sum \limits_{i = 1,i \ne l}^L {\hat A_i}{\hat S_i} \left( {{{\hat f}_l} + \dfrac{p}{N}} \right),p = \pm \dfrac{1}{2} $ (iv)其中， ${X_p}({\hat f_l}) = X\left( {{{\hat f}_l} + \dfrac{p}{N}} \right)$ (v) $ \delta = \dfrac{1}{2}{{\mathrm{Re}}} \left[ {\dfrac{{{{\tilde X}_{0.5}}({{\hat f}_l}) + {{\tilde X}_{ - 0.5}}({{\hat f}_l})}}{{{{\tilde X}_{0.5}}({{\hat f}_l}) - {{\tilde X}_{ - 0.5}}({{\hat f}_l})}}} \right] $ (vi) ${\hat f_l} \leftarrow {\hat f_l} + \dfrac{\delta }{N}$ (vii) $ {\hat A_l} = \dfrac{1}{N} \left\{ {\displaystyle\sum \limits_{k = 0}^{N - 1} x(k){{\text{e}}^{ - {\text{j}}{\textstyle\frac{{2\pi }}{N}}k{{\hat f}_l}}} - \displaystyle\sum \limits_{i = 1,i \ne l}^L {{\hat A}_i}{{\hat S}_i}({{\hat f}_l})} \right\} $ (viii) $q \leftarrow q + 1$ 输出: $\left\{ {{{\hat f}_l},{{\hat A}_l}} \right\},l = 1,2, \cdots ,L$ 
下载: 导出CSV
2 具有目标个数估计功能的FIIB算法
2. The FIIB algorithm with model order estimation
初始化： 设 ${L_{\max }}$, Q 循环: 对于 $L = 1,2, \cdots ,{L_{\max }}$ $\left\{ {{{\hat f}_l},{A_l}} \right\}_{l = 1}^L = {\text{FIIB(}}{\boldsymbol{x}},L,Q)$ 计算 ${C_{{\text{ITC}}}}(L)$ 结束 取: $ \hat{L}=\mathrm{arg}\mathop{ \mathrm{max}}\limits_{L=1,2,\cdots, {L}_{\mathrm{max}} }\{{C}_{\text{ITC}}(L)\} $ 输出: $\hat L,\{ {\hat f_l},{\hat A_l}\} ,l = 1,2, \cdots ,\hat L$
下载: 导出CSV
表 1 点目标仿真参数
Table 1. Simulation parameters of point targets
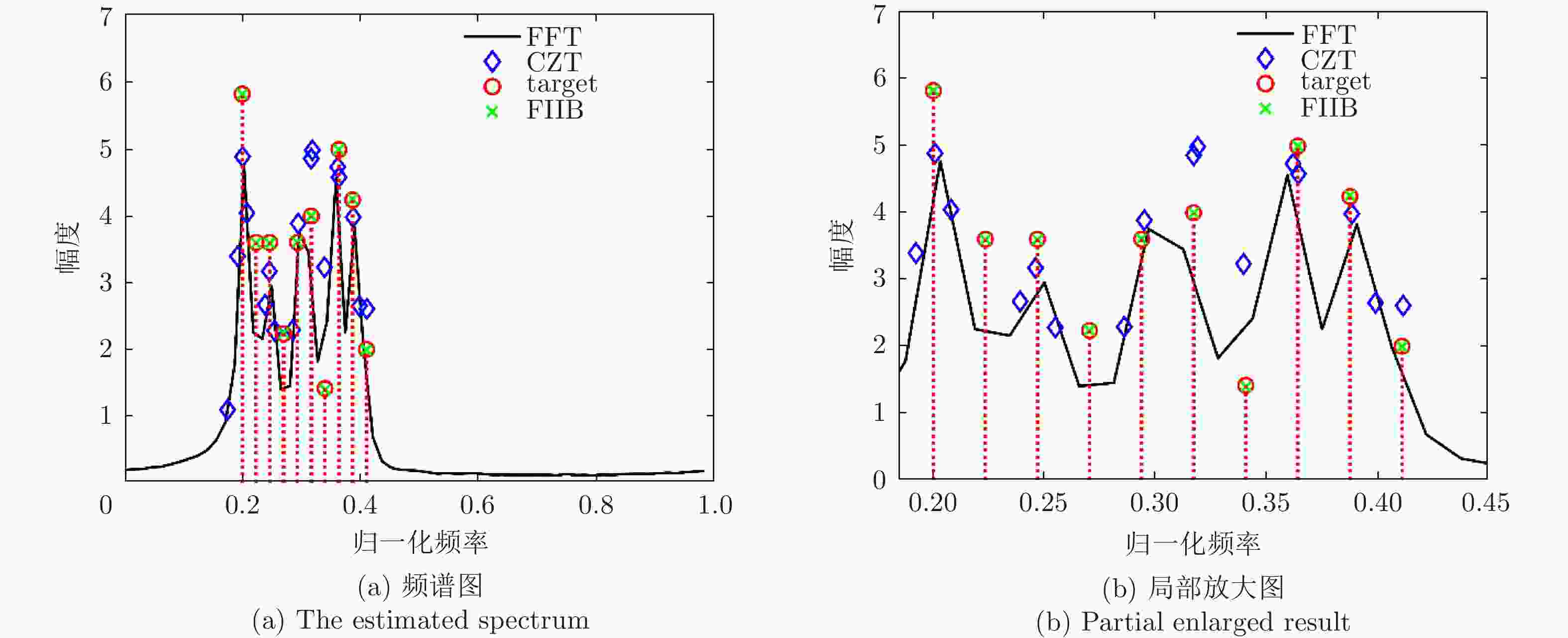
参数 数值 参数 数值 频率值${f_1}$ 0.2687 信号长度N 64 复振幅${A_1}$ 5.0000+3.0000j 迭代次数Q 10 频率值${f_2}$ 0.3000 设定最大目标数${L_{\max}}$ 5 复振幅${A_2}$ 0.5000–0.3000j 信噪比$\rho $ (dB) 20
下载: 导出CSV
表 2 强点目标参数估计结果(
${\boldsymbol{W}}_{\bf{1}}/{\boldsymbol{W}}_{\bf{2}} $ =20 dB)Table 2. Estimation result of strong point targets (W1/W2=20 dB)
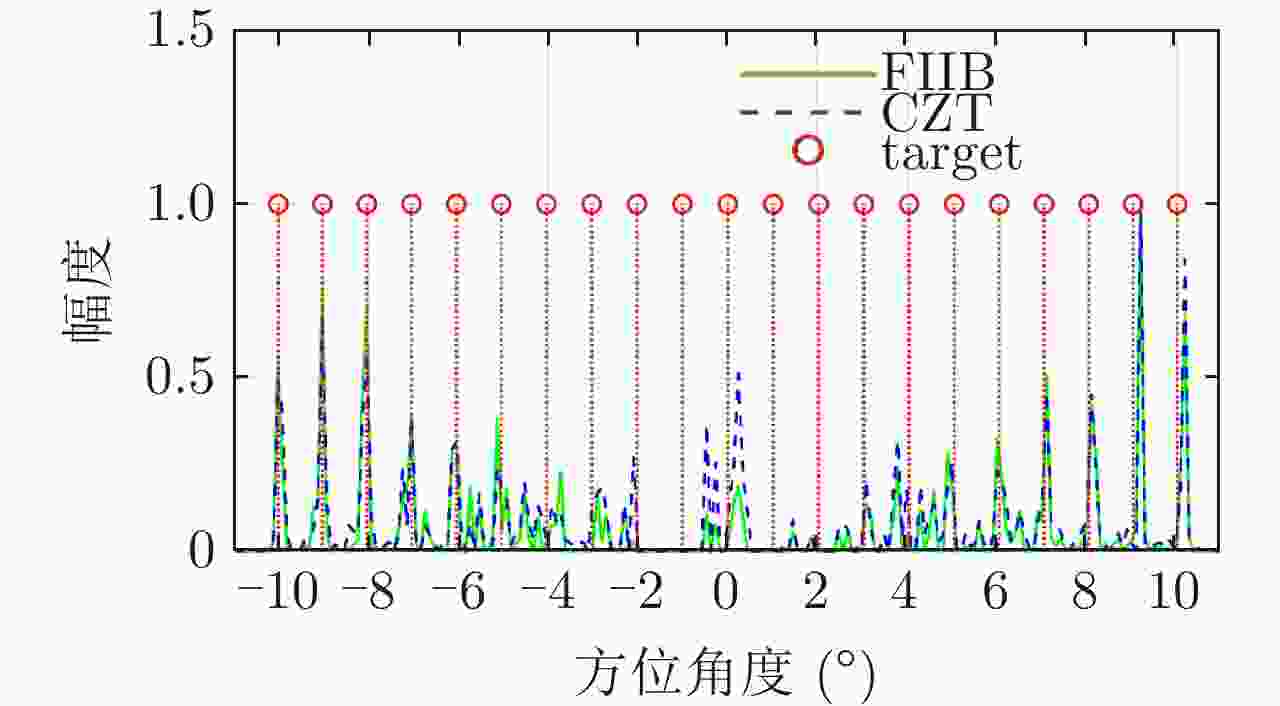
参数 方法 目标1 目标2 假目标 假目标 假目标 假目标 频率 True 0.2687 0.3000 / / / / FIIB 0.2687 0.3000 / / / / CZT 0.2689 0.2737 0.2578 0.2912 0.3063 0.3232 FFT 0.2656 / / / / / 复振幅 True 5.0000+3.0000j 0.5000–0.3000j / / / / FIIB 4.9906+3.0169j 0.4905–0.3066j / / / / CZT 4.9230+3.1262j 4.6314–1.8004j –1.8767+0.8188j 1.0581–0.5353 0.5413–0.8405j 0.3429–0.4851j FFT 2.2325+5.0000j / / / / /
下载: 导出CSV
表 3 相邻点目标参数估计结果(
${\boldsymbol{\Delta}} {\boldsymbol{f}} = {\bf{1}}/{\boldsymbol{N}}$ )Table 3. Estimation result of neighboring point targets (
${\boldsymbol{\Delta}} {\boldsymbol{f}} = {\bf{1}}/{\boldsymbol{N}}$ )参数 方法 目标1 目标2 假目标 假目标 假目标 假目标 频率 True 0.2844 0.3000 / / / / FIIB 0.2844 0.3000 / / / / CZT 0.2828 0.3021 0.3049 0.2734 0.3227 0.2550 FFT 0.2813 0.2969 / / / / 复振幅 True 5.0000+3.0000j 5.0000–3.0000j / / / / FIIB 4.9852+3.0081j 4.9980–3.0238j / / / / CZT 4.1085+4.3364j 4.1257–4.3441j 1.5993–5.3751j –1.9113+1.6013j 0.2152–1.7055j –0.5516+0.9638j FFT 3.0812+5.0246j 4.9664–0.8527j / / / /
下载: 导出CSV
表 4 不同迭代次数下同多普勒单元内两个目标的FIIB参数估计结果
Table 4. Estimation result of FIIB for two targets situated in a Doppler bin by different iterations
迭代次数Q ${f_1}$ ${f_2}$ ${A_1}$ ${A_2}$ 50 0.2899 0.2980 2.4221+2.4827j 7.3413–2.0001j 100 0.2915 0.2994 4.0880+2.9784j 5.9220–2.9249j 200 0.2920 0.2999 4.7896+2.9998j 5.2107–2.9917j 300 0.2922 0.3000 4.9617+2.9887j 5.0442–2.9834j (真实值) 0.2922 0.3000 5.0000+3.0000j 5.0000–3.0000j
下载: 导出CSV
表 5 前视扫描成像实验仿真参数
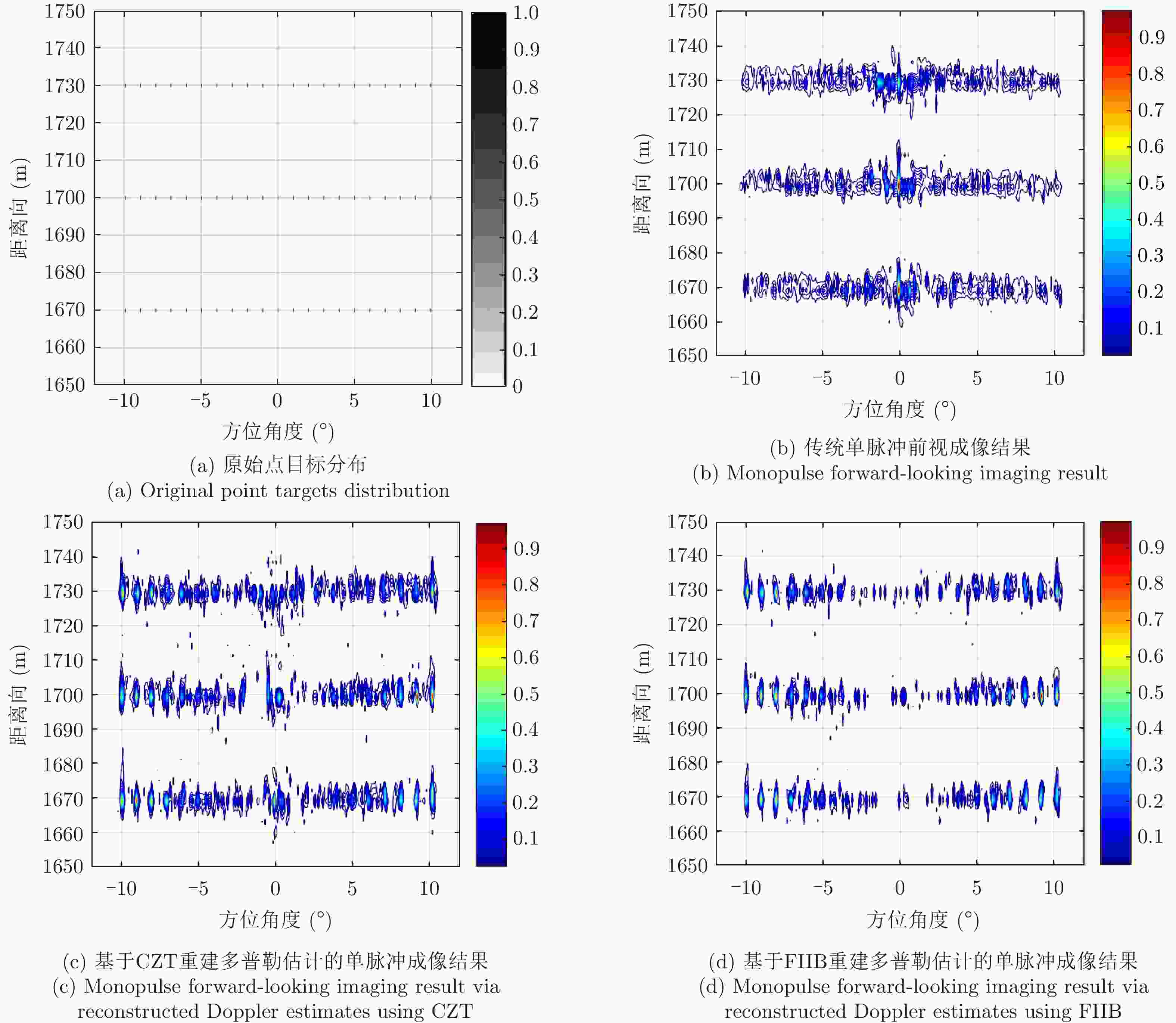
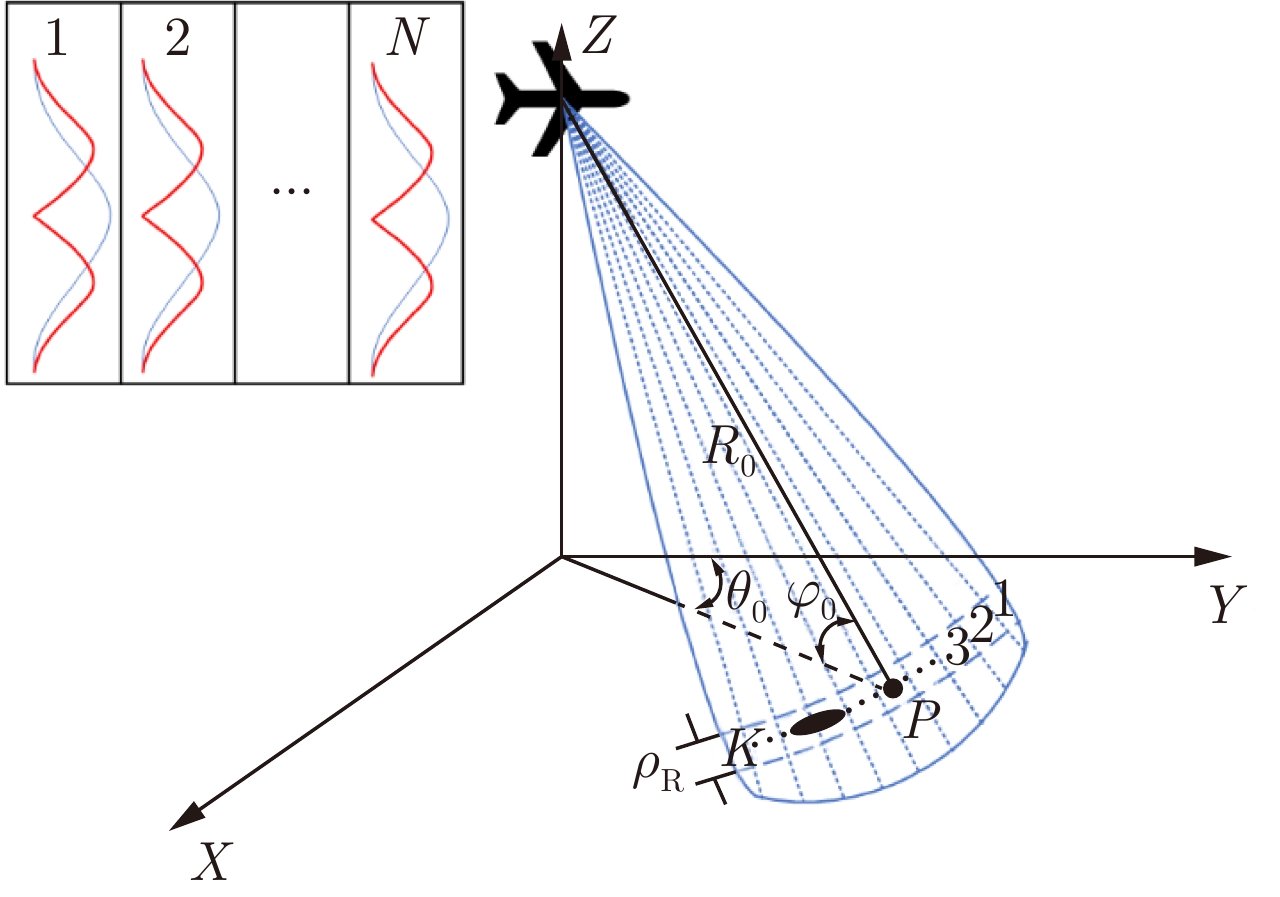
Table 5. Simulation parameters of a forward-looking scanning radar
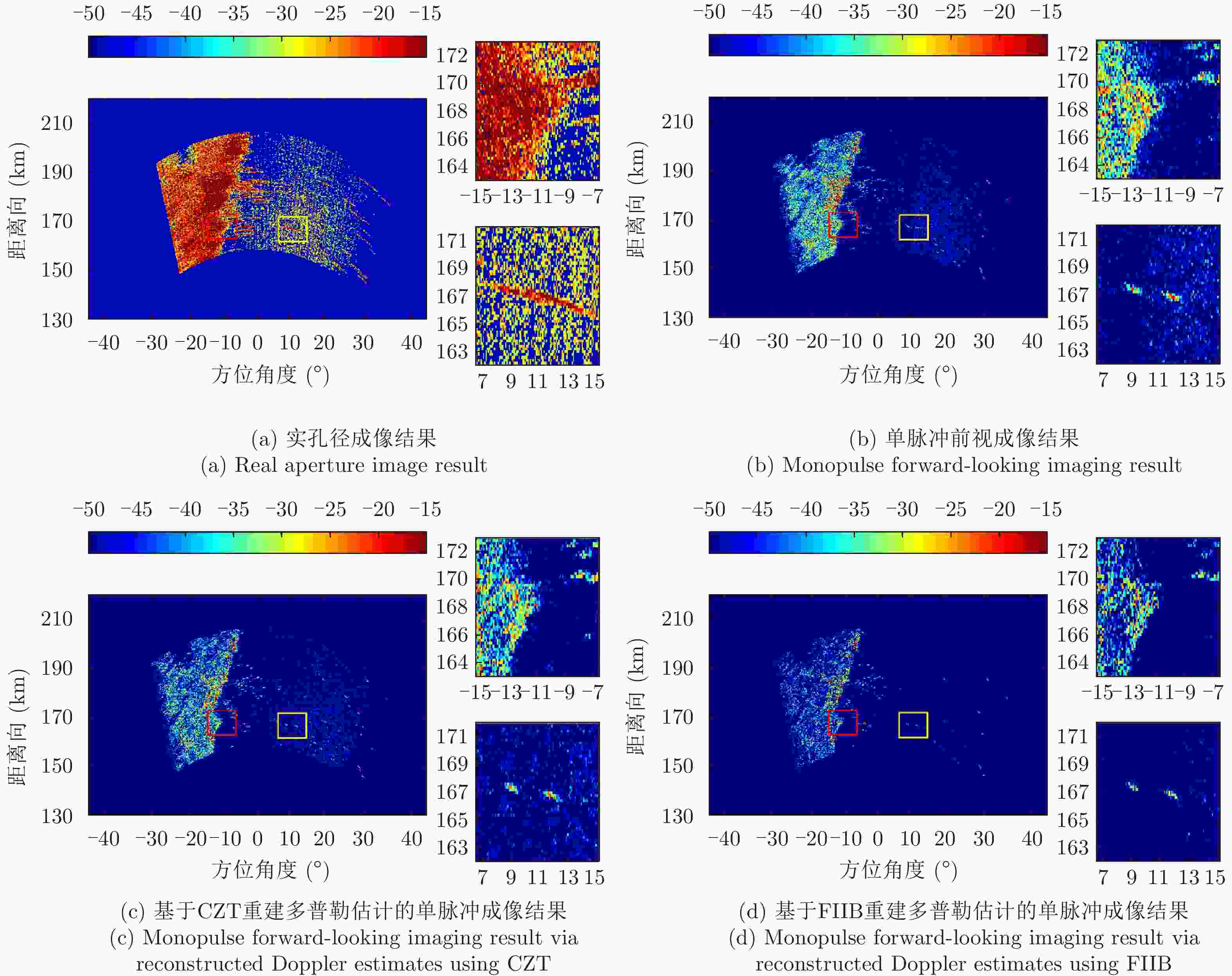
参数 数值 参数 数值 平台飞行速度(m/s) 100 场景中心地距(m) 1700 雷达中心频率(GHz) 18 距离×方位分辨单元 (m×m) 3×3 信号带宽(MHz) 50 和通道3 dB波束宽度(°) 5 信号脉宽(μs) 1 波束扫描范围(°) –15～15 脉冲重复频率PRF (Hz) 2000 天线扫描速度(°/s) 30
下载: 导出CSV
表 6 场景仿真不同方法的定量评价
Table 6. Quantitative evaluation of different methods of scenario simulation
方法 MSE C 传统单脉冲前视成像 $0.00663$ $51.4994$ 基于CZT重建多普勒估计的单脉冲成像 $0.00394$ $52.3441$ 基于FIIB重建多普勒估计的单脉冲成像 $0.00387$ $ 59.7068 $
下载: 导出CSV
表 7 实测数据不同方法的定量评价
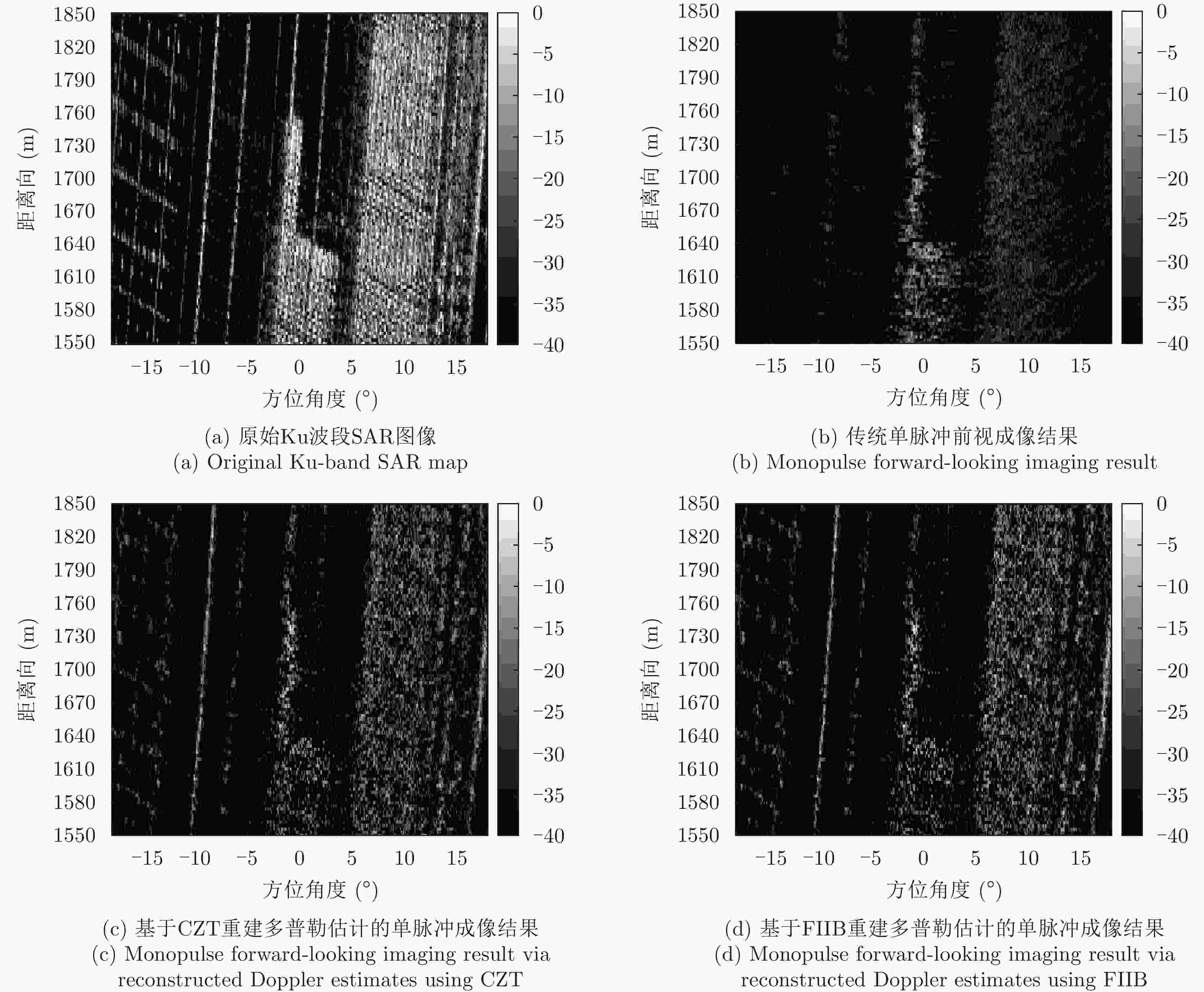
Table 7. Quantitative evaluation of different methods of real data
方法 $ {\text{ENT}} $ C 实孔径图像 $4.2660$ $411.9599$ 传统单脉冲前视成像 ${\text{3}}{\text{.6825}}$ $213.4888$ 基于CZT重建多普勒估计的单脉冲成像 $3.5228$ $233.1903$ 基于FIIB重建多普勒估计的单脉冲成像 $2.9651$ $292.8872$
下载: 导出CSV
-
[1] 李亚超, 王家东, 张廷豪, 等. 弹载雷达成像技术发展现状与趋势[J]. 雷达学报, 2022, 11(6): 943–973. doi: 10.12000/JR22119LI Yachao, WANG Jiadong, ZHANG Tinghao, et al. Present situation and prospect of missile-borne radar imaging technology[J]. Journal of Radars, 2022, 11(6): 943–973. doi: 10.12000/JR22119 [2] CHEN Hongmeng, LI Yachao, GAO Wenquan, et al. Bayesian forward-looking superresolution imaging using Doppler deconvolution in expanded beam space for high-speed platform[J]. IEEE Transactions on Geoscience and Remote Sensing, 2022, 60: 5105113. doi: 10.1109/TGRS.2021.3107717 [3] LI Yueli, LIU Jianguo, JIANG Xiaoqing, et al. Angular superresol for signal model in coherent scanning radars[J]. IEEE Transactions on Aerospace and Electronic Systems, 2019, 55(6): 3103–3116. doi: 10.1109/TAES.2019.2900133 [4] 马佳智, 施龙飞, 徐振海, 等. 单脉冲雷达多点源参数估计与抗干扰技术进展[J]. 雷达学报, 2019, 8(1): 125–139. doi: 10.12000/JR18093MA Jiazhi, SHI Longfei, XU Zhenhai, et al. Overview of multi-source parameter estimation and jamming mitigation for monopulse radars[J]. Journal of Radars, 2019, 8(1): 125–139. doi: 10.12000/JR18093 [5] 吴迪, 杨成杰, 朱岱寅, 等. 一种用于单脉冲成像的自聚焦算法[J]. 电子学报, 2016, 44(8): 1962–1968. doi: 10.3969/j.issn.0372-2112.2016.08.027WU Di, YANG Chengjie, ZHU Daiyin, et al. An autofocusing algorithm for monopulse imaging[J]. Acta Electronica Sinica, 2016, 44(8): 1962–1968. doi: 10.3969/j.issn.0372-2112.2016.08.027 [6] 吴迪, 朱岱寅, 田斌, 等. 单脉冲成像算法性能分析[J]. 航空学报, 2012, 33(10): 1905–1914.WU Di, ZHU Daiyin, TIAN Bin, et al. Performance evaluation for Monopulse imaging algorithm[J]. Acta Aeronautica et Astronautica Sinica, 2012, 33(10): 1905–1914. [7] 胡艳芳, 陈伯孝, 吴传章. 基于单脉冲三维成像的抗交叉眼干扰方法[J]. 系统工程与电子技术, 2022, 44(4): 1188–1194. doi: 10.12305/j.issn.1001-506X.2022.04.15HU Yanfang, CHEN Baixiao, and WU Chuanzhang. Anti-cross-eye jamming method based on monopulse radar 3-D imaging[J]. Systems Engineering and Electronics, 2022, 44(4): 1188–1194. doi: 10.12305/j.issn.1001-506X.2022.04.15 [8] LONG Teng, LU Zheng, DING Zegang, et al. A DBS Doppler centroid estimation algorithm based on entropy minimization[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(10): 3703–3712. doi: 10.1109/TGRS.2011.2142316 [9] CHEN Hongmeng, LI Ming, WANG Zeyu, et al. Cross-range resolution enhancement for DBS imaging in a scan mode using aperture-extrapolated sparse representation[J]. IEEE Geoscience and Remote Sensing Letters, 2017, 14(9): 1459–1463. doi: 10.1109/LGRS.2017.2710082 [10] 马长征, 张守宏. 超分辨在单脉冲雷达三维成像中的应用[J]. 西安电子科技大学学报, 1999, 26(3): 379–382. doi: 10.3969/j.issn.1001-2400.1999.03.027MA Changzheng and ZHANG Shouhong. Applications of super-resolution signal processing on monopulse radar three dimensional imaging[J]. Journal of Xidian University, 1999, 26(3): 379–382. doi: 10.3969/j.issn.1001-2400.1999.03.027 [11] 李悦丽, 马萌恩, 赵崇辉, 等. 基于单脉冲雷达和差通道多普勒估计的前视成像[J]. 雷达学报, 2021, 10(1): 131–142. doi: 10.12000/JR20111LI Yueli, MA Meng’en, ZHAO Chonghui, et al. Forward-looking imaging via Doppler estimates of sum-difference measurements in scanning monopulse radar[J]. Journal of Radars, 2021, 10(1): 131–142. doi: 10.12000/JR20111 [12] STOICA P and NEHORAI A. MUSIC, maximum likelihood, and Cramer-Rao bound[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1989, 37(5): 720–741. doi: 10.1109/29.17564 [13] SHAH S M, SAMAR R, KHAN N M, et al. Fractional-order adaptive signal processing strategies for active noise control systems[J]. Nonlinear Dynamics, 2016, 85(3): 1363–1376. doi: 10.1007/s11071-016-2765-6 [14] ROY R and KAILATH T. ESPRIT-estimation of signal parameters via rotational invariance techniques[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1989, 37(7): 984–995. doi: 10.1109/29.32276 [15] 叶沙琳, 张永, 杨桃丽, 等. 基于稀疏空间谱估计的星载SAR DBF算法[J]. 上海航天, 2018, 35(6): 65–70. doi: 10.19328/j.cnki.1006-1630.2018.06.011YE Shalin, ZHANG Yong, YANG Taoli, et al. An algorithm for spaceborne SAR DBF based on sparse spatial spectrum estimation[J]. Aerospace Shanghai, 2018, 35(6): 65–70. doi: 10.19328/j.cnki.1006-1630.2018.06.011 [16] LI Feng, GAO Yunpeng, CAO Yijia, et al. Improved teager energy operator and improved Chirp-Z transform for parameter estimation of voltage flicker[J]. IEEE Transactions on Power Delivery, 2016, 31(1): 245–253. doi: 10.1109/TPWRD.2015.2448943 [17] WANG Kai, WANG Lanlan, YAN Bao, et al. Efficient frequency estimation algorithm based on Chirp-Z transform[J]. IEEE Transactions on Signal Processing, 2022, 70: 5724–5737. doi: 10.1109/TSP.2022.3224648 [18] ABOUTANIOS E, HASSANIEN A, AMIN M G, et al. Fast iterative interpolated beamforming for accurate single-snapshot DOA estimation[J]. IEEE Geoscience and Remote Sensing Letters, 2017, 14(4): 574–578. doi: 10.1109/LGRS.2017.2661315 [19] 许可, 王玲, 万建伟. 信号处理仿真实验[M]. 2版. 北京: 清华大学出版社, 2020: 106–107.XU Ke, WANG Ling, and WAN Jianwei. Emulation for Signal Processing[M]. 2nd ed. Beijing: Tsinghua University Press, 2020: 106–107. [20] 柏果, 程郁凡, 唐万斌. 基于两阶段加窗插值的多音信号频率估计算法[J]. 电子科技大学学报, 2021, 50(5): 682–688. doi: 10.12178/1001-0548.2021066BAI Guo, CHENG Yufan, and TANG Wanbin. Frequency estimation of multi-tone by two-stage windowed interpolation[J]. Journal of University of Electronic Science and Technology of China, 2021, 50(5): 682–688. doi: 10.12178/1001-0548.2021066 [21] 杨超, 李波, 胡绪权, 等. 基于迭代离散时间傅里叶变换插值的高精度频率估计[J]. 电网技术, 2021, 45(10): 3955–3963. doi: 10.13335/j.1000-3673.pst.2020.2285YANG Chao, LI Bo, HU Xuquan, et al. High-precision frequency estimation based on iterative DTFT interpolation[J]. Power System Technology, 2021, 45(10): 3955–3963. doi: 10.13335/j.1000-3673.pst.2020.2285 [22] YE Shanglin and ABOUTANIOS E. An algorithm for the parameter estimation of multiple superimposed exponentials in noise[C]. IEEE International Conference on Acoustics, Speech and Signal Processing, South Brisbane, Australia, 2015: 3457–3461. [23] ABOUTANIOS E. On the convergence of the fast iterative interpolated beamformer[C]. The 53rd Asilomar Conference on Signals, Systems, and Computers, Pacific Grove, USA, 2019: 630–634. [24] HASSANIEN A and ABOUTANIOS E. Single-snapshot beamforming using fast iterative adaptive techniques[C]. The 11th Sensor Array and Multichannel Signal Processing Workshop, Hangzhou, China, 2020: 1–4. [25] MILLS K R, AHMAD F, and ABOUTANIOS E. Coarray-domain iterative direction-of-arrival estimation with coprime arrays[J]. Digital Signal Processing, 2022, 122: 103332. doi: 10.1016/j.dsp.2021.103332 [26] ABOUTANIOS E and HASSANIEN A. Low-cost beamforming-based DOA estimation with model order determination[C]. The 11th Sensor Array and Multichannel Signal Processing Workshop, Hangzhou, China, 2020: 1–5. [27] LIU F, ZHAO F, YU W, et al. Ship detection and speed estimation based on azimuth scanning mode of synthetic aperture radar[J]. IET Radar, Sonar & Navigation, 2012, 6(6): 425–431. doi: 10.1049/iet-rsn.2011.0139 [28] SHERMAN S M, BARTON D K, 周颖, 陈远征, 赵锋, 等译. 单脉冲测向原理与技术[M]. 2版. 北京: 国防工业出版社, 2013: 172–197.SHERMAN S M, BARTON D K, ZHOU Ying, CHEN Yuanzheng, ZHAO Feng, et al. translation. Monopulse Principles and Techniques[M]. 2nd ed. Beijing: National Defense Industry Press, 2013: 172–197. [29] ABOUTANIOS E and MULGREW B. Iterative frequency estimation by interpolation on Fourier coefficients[J]. IEEE Transactions on Signal Processing, 2005, 53(4): 1237–1242. doi: 10.1109/TSP.2005.843719 [30] AKAIKE H. A new look at the statistical model identification[J]. IEEE Transactions on Automatic Control, 1974, 19(6): 716–723. doi: 10.1109/TAC.1974.1100705 [31] ABOUTANIOS E. Frequency estimation for low earth orbit satellites[D]. [Ph.D. dissertation], University of Technology, 2002: 178–205. [32] WEI Shunjun, ZHOU Zichen, WANG Mou, et al. 3DRIED: A high-resolution 3-D millimeter-wave radar dataset dedicated to imaging and evaluation[J]. Remote Sensing, 2021, 13(17): 3366. doi: 10.3390/rs13173366 [33] ZHANG Shuanghui, LIU Yongxiang, and LI Xiang. Fast entropy minimization based autofocusing technique for ISAR imaging[J]. IEEE Transactions on Signal Processing, 2015, 63(13): 3425–3434. doi: 10.1109/TSP.2015.2422686 -
计量
- 文章访问数: 382
- HTML全文浏览量: 192
- PDF下载量: 100
- 被引次数: 0
