基于机载多通道雷达迭代超分辨估计的前视成像

任凌云; 吴迪; 朱岱寅; 孙伟杰

doi:10.12000/JR23085

基于机载多通道雷达迭代超分辨估计的前视成像

DOI: 10.12000/JR23085

南京航空航天大学电子信息工程学院南京 211106

基金项目: 国家自然科学基金(62271252)

详细信息

作者简介:
任凌云，博士生，主要研究方向为雷达前视成像、MIMO雷达信号处理

吴　迪，教授，主要研究方向为雷达前视成像、雷达信号处理、空时自适应处理技术等

朱岱寅，教授，主要研究方向为合成孔径雷达/逆合成孔径雷达(SAR/ISAR)成像以及自聚焦算法、MIMO雷达信号处理、干涉SAR成像、SAR地面动目标指示以及机载雷达空中动目标指示技术等

孙伟杰，硕士生，主要研究方向为雷达前视成像、阵列信号处理

通讯作者:
吴迪 wudi82@nuaa.edu.cn

朱岱寅 zhudy@nuaa.edu.cn

责任主编：李亚超 Corresponding Editor: LI Yachao
中图分类号: TN957.5; TN959.4
计量
- 文章访问数: 767
- HTML全文浏览量: 253
- PDF下载量: 266
- 被引次数: 1
出版历程
- 收稿日期: 2023-05-10
- 修回日期: 2023-07-10
- 网络出版日期: 2023-07-27
- 刊出日期: 2023-12-28

Forward-looking Imaging via Iterative Super-resolution Estimation in Airborne Multi-channel Radar

College of Electronic Information Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 211106, China

Funds: The National Natural Science Foundation of China (62271252)

More Information

Corresponding author: WU Di, wudi82@nuaa.edu.cn; ZHU Daiyin, zhudy@nuaa.edu.cn

摘要

摘要: 波达角估计算法用于机载多通道雷达前视成像时可以突破瑞利极限，实现同一波束主瓣宽度内的多目标分辨，改善成像的方位向分辨率，然而天线波束覆盖有限且其快速扫描使得可用于协方差矩阵估计的数据样本缺乏，导致对目标位置和幅度估计出现误差。该文提出了一种基于单快拍迭代超分辨处理的多通道雷达前视成像算法，通过对单个空域快拍的迭代谱估计可获得目标的准确位置和幅度信息，再通过多个脉冲的非相干累积得到前视方位高分辨成像。仿真和实测数据处理结果表明，所提算法具有分辨多目标的能力，相较于传统前视成像算法显著提高了前视图像的方位分辨率，同时保证了点目标的精确重构和面目标的轮廓重构。
- 波达角估计 /
- 前视成像 /
- 单快拍 /
- 迭代超分辨 /
- 迭代最小均方误差
Abstract: The direction of arrival estimation algorithm can overcome the Rayleigh limit, effectively separate multiple targets within the main lobe, and improve the azimuth resolution when applied to forward-looking imaging in airborne multi-channel radar. However, the limited antenna beam coverage and rapid scanning result in a scarcity of data samples available for covariance matrix estimation, leading to direction and amplitude estimation errors. Herein, we propose a forward-looking imaging algorithm based on single-snapshot iterative super-resolution estimation. The algorithm performs single-snapshot iterative spectral estimation to accurately determine the azimuth and amplitude of the target. Subsequently, a high-resolution image is achieved through non-coherent accumulation. Simulation and experimental data processing results show that the proposed algorithm can resolve multiple targets, significantly improving the azimuth resolution of the forward-looking image compared with traditional forward-looking imaging algorithms. Moreover, it ensures the accurate reconstruction of point targets and contour reconstruction of area targets.
- DOA estimation /
- Forward-looking imaging /
- Single snapshot /
- Iterative super-resolution /
- Iterative minimum mean square error

HTML全文

1. 引言

森林高度是估算森林蓄积量及生物量的重要基础数据，对于研究森林资源状况以及分析全球生态环境、气候变化具有重要意义。极化合成孔径雷达干涉测量技术(Polarimetric SAR Interferometry, PolInSAR)采用微波监测模式，其回波信号不仅记录垂直结构及其属性信息，且可以区分同一分辨单元内不同散射体高度的能力，已被视为大范围、高分辨率、高精度反演森林高度的有效手段之一^[1]。

为了实现利用PolInSAR观测量准确地提取森林高度，Thrauhft等人^[2]建立了随机地体二层散射模型(Random Volume over Ground, RVoG)，该模型将森林散射场景抽象为两层，即由随机均匀分布的散射体组成的植被层，以及微波信号不可穿透的地表层。随后，Papathanassiou等人^[3,4]进一步分析PolInSAR复相干性与RVoG模型的关联，建立了利用PolInSAR反演森林高度的框架。实质上，该框架是基于体散射去相干的模型表达来反演森林高度等参数的，并且利用不同PolInSAR数据都获得了较高的反演精度^[5-7]。

由于森林场景具有显著的时变性，具有长时间间隔的星载重轨干涉SAR(如ALOS-1至少为46天，ALOS-2为14天)散射场景内介电常数变化(如降雨)和风动都会产生严重的时间去相干。因此，除了体去相干的影响，时间去相干也是星载重轨极化干涉SAR数据中不可忽略的去相干因素，决定了森林参数反演的精度，甚至是反演成败的关键。为此，Yang等人^[8]在随机移动散射模型(Random Motion over Ground, RMoG)^[9]和体时去相干散射模型 (Volume Temporal Decorrelation, VTD)模型^[10]基础上，提出一种时间去相干半经验森林高度反演方法。该方法结合少量机载LiDAR森林高度数据辅助时间去相干半经验模型解算，利用ALOS-1 PARSAR-1 HV极化相干幅度成功实现了大尺度森林高度反演。

然而，该方法需假设HV极化不包含地表散射回波能量贡献，事实上，L波段SAR信号具有较强的穿透性，尤其当森林高度较低或密度较小时，HV极化方式会记录显著地表回波信号。此外，该方法只适用于单基线干涉数据，尚未考虑多基线条件下，如何充分利用观测几何的多样性提升反演结果的可靠性。因此本文的目的是针对上述反演方法的限制，利用ALOS-2 PARSAR-2多基线PolInSAR数据更为准确地提取森林高度。主要思路如下：首先利用相干最大分离算法(Maximum Coherence Difference, MCD)在极化空间内寻求具有最少地面散射能量贡献的极化方式，以获得更为纯净的森林冠层散射贡献。然后利用该极化方式的相干幅度，在少量森林高度地面调查数据辅助下基于时间去相干半经验模型进行森林高度反演。在此基础之上，结合多基线数据根据PolInSAR相干集在复数平面内的几何表达，甄选最优观测干涉数据的反演结果作为森林高度反演最终结果。

2. 多基线PolInSAR森林高度反演策略

2.1 时间去相干半经验模型

综合顾及垂直方向上散射体分布产生的体去相干、散射场景内介电特性改变和植被风动引起的时间去相干同时占主导地位，星载重轨PolInSAR复相干系数一般形式表示为^[8]

$\gamma (\omega ) = {{\rm e}^{i{\varphi _0}}} \cdot \frac{{\gamma _d^{\rm v} \cdot {\gamma _{{\rm{v/m}}}} + \gamma _d^{\rm g} \cdot \mu (\omega )}}{{1{\rm{ + }}\mu (\omega )}}$

(1)

式中， ${\varphi _0}$ 为地表相位； $\gamma _d^{\rm v}$ 和 $\gamma _d^{\rm g}$ 分别表示植被体层和地面层介电特性改变引起的时间去相干复因子； $\mu (\omega )$ 为地体幅度比，与极化方式有关； ${\gamma _{{\rm{v/m}}}}$ 为体散射去相干和时间去相干(植被风动引起)产生的耦合去相干

${\gamma _{\rm v/m}} = \frac{{\displaystyle\int_0^h {\exp \left[ - \dfrac{1}{2}{{\left(\dfrac{{4\pi }}{\lambda }\right)}^2}\sigma _r^2(z)\right] \!\cdot\! f(z) \cdot \exp (i{k_z}z){\rm{d}}z} }}{{\displaystyle\int_0^h {f(z){\rm{d}}z} }}$

(2)

其中， $h$ 表示森林高度； $f\left( z \right)$ 为指数形式的垂直结构函数，描述垂直方向 $z$ 上散射体的分布； ${\sigma _r}(z)$ 为散射体沿雷达视线方向的随机运动标准差，假定与森林高度呈线性关系

${\sigma _r}(z) = \frac{{{\sigma _r}}}{{{h_r}}}z$

(3)

式中， ${\sigma _r}$ 表示在参考高度 ${h_r}$ (根据先验信息一般设为15 m^[8,9])处的运动标准差。

为了解决上述模型过参数化问题，Yang等人^[8]对散射场景做如下假设：(1) 散射场景内时间去相干与消光系数在空间上具有一致性；(2) HV极化方式具有较小地面散射能量贡献，可假定其地体幅度比 ${\mu _{\min }}{\rm{ = 0}}$ ，此时对于该极化方式可忽略地面层介电常数改变引起的时间去相干；(3) 假定干涉场景为零空间基线理想情况(忽略森林垂直结构引起的体散射去相干)，即垂直有效波束 ${k_z} = 0$ ，此时对于式(2)适用积分第一中值定理(即对于在给定区间 $[a,b]$ 有连续函数 $f(x)$ 和同号可积函数 $g(x)$ ，区间内存在一点 $\varepsilon$ 满足 $\displaystyle\int_a^b {f(x)} g(x){\rm{d}}x = f(\varepsilon )\displaystyle\int_a^b {g(x)} {\rm{d}}x$ 。因此在上述假定条件下，式(1)可简化为时间去相干半经验模型^[8,11]

$\begin{split} \gamma =\,& {S_{{\rm{scene}}}} \cdot \exp \left[ - \frac{1}{2}{\left(\frac{{4\pi {\sigma _r}\alpha }}{{\lambda {h_r}}}\right)^2}{h^2}\right] \\ \approx\,& {S_{{\rm{scene}}}} \cdot {\rm{sinc}}\left(\frac{h}{{{C_{{\rm{scene}}}}}}\right) \end{split}$

(4)

其中， $\alpha$ 为中值 $\varepsilon$ 关于森林高度的比例因子，即 $\varepsilon = \alpha h$ ( $0 \le \alpha \le 1$ ); ${S_{{\rm{scene}}}}$ , ${C_{{\rm{scene}}}}$ 分别与植被体层介电特性改变和风动引起的时间去相干有关

${S_{{\rm{scene}}}} = \left| {\gamma _d^{\rm v}} \right|;\ {C_{{\rm{scene}}}} = \frac{{\lambda {h_r}}}{{2{\pi ^2}{\sigma _r}\alpha }}$

(5)

2.2 MCD相干优化算法

已有方法主要选用对森林冠层较为敏感的HV极化方式进行模型求解，但是ALOS-2 PALSAR-2发射具有较强穿透能力的L波段电磁波，HV极化方式回波信号中同样会记录显著地表回波信号。鉴于此，本文利用ALOS-2 PALSAR-2全极化数据结合极化相干最优理论，尽可能抑制地表回波信号的干扰。具体方法如下：

在主辅极化SAR影像散射机制相同的情况下，极化干涉SAR的复相干系数表示为^[12]

$\gamma = \frac{{\left\langle {{{{\omega}} ^{\rm{H}}}{{{\varOmega}} _{{\rm{12}}}}{{\omega}} } \right\rangle }}{{\sqrt {\left\langle {{{{\omega}} ^{\rm{H}}}{{{T}}_{{\rm{11}}}}{{\omega}} } \right\rangle \left\langle {{{{\omega}} ^{\rm{H}}}{{{T}}_{22}}{{\omega}} } \right\rangle } }}$

(6)

式中，自相关矩阵 ${{{T}}_{{\rm{11}}}}$ 和 ${{{T}}_{22}}$ 都是标准Hermitian相干矩阵，分别描述主辅影像的极化特性， ${{{\varOmega}} _{12}}$ 为互相关矩阵，不仅包含极化信息，还包含了主副影像不同极化通道间的干涉相位关系。 ${{\omega}}$ 为归一化复投影矢量，通过转换 ${{\omega}}$ 可以计算极化空间内任意极化基下对应散射机制的复相干系数，组成相干集。该复相干系数集合形成的区域边界范围可以看作将相干复平面旋转任意角度，得到的实部最大和最小相干系数^[13]

$\left. \begin{aligned} & {{\rm{Re}} (\gamma {{\rm e}^{i\phi }}) = \frac{{{{{\omega}} ^{\rm{H}}}{{A}}{{\omega}} }}{{{{{\omega}} ^{\rm H}}{{T}}{{\omega}} }}} \\ & {{{A}} = \frac{{{{\rm e}^{i\phi }}{{{\varOmega}} _{12}} + {{\rm e}^{ - i\phi }}{{\varOmega}} _{12}^{\rm{H}}}}{2},{{T}} = \frac{{{{{T}}_{11}} + {{{T}}_{22}}}}{2}} \end{aligned} \right\}$

(7)

式中， $\phi$ 为旋转相位，在 $\left[ {0,\pi } \right)$ 范围内等间隔采样角度。式(7)求极值可以转化为求解特征值问题即 ${{A}}{{\omega}} = \lambda {{T}}{{\omega}}$ ，进而得到最大和最小特征值分别对应的特征向量 ${{{\omega}} _1}$ 和 ${{{\omega}} _2}$ ，那么相干区域的一对边界点可以表示为^[14]

${\gamma _1} = \frac{{{{\omega}} _1^{\rm{H}}{{{\varOmega}} _{12}}{{{\omega}} _1}}}{{{{\omega}} _1^{\rm{H}}{{T}}{{{\omega}} _1}}},\ {\gamma _2} = \frac{{{{\omega}} _2^{\rm{H}}{{{\varOmega}} _{12}}{{{\omega}} _2}}}{{{{\omega}} _2^{\rm{H}}{{T}}{{{\omega}} _2}}}$

(8)

相比传统InSAR技术只能获取HH, HV或VV极化方式对应的复相干系数，相干集中包含了特定极化散射机理对应的复相干系数，为寻求极化空间内具有更为纯净森林冠层散射贡献的极化方式提供了可能。相干区域范围示意如图1所示，其中在相干区域成对边界点中距离最远的一对相干系数点 ${\gamma _{\rm{A}}}$ , ${\gamma _{\rm{B}}}$ (也就是相干区域长轴两端点)，可以表征植被层和地表层有效相位中心的最大分离^[15]。根据式(9)进一步确定体散射占优极化方式复相干 $\gamma ({\mu _{\min }})$ 与地表散射占优极化方式的复相干 $\gamma ({\mu _{\max }})$

图 1 不同干涉对相干区域在复平面上的示意图

Figure 1. Coherent regions on the complex plane for different interferometric pairs

下载: 全尺寸图片幻灯片

$\left. \begin{aligned} & {k_z} > 0: \\ & \quad {\rm{if\; arg(}}{\gamma _{\rm{A}}}\gamma _{\rm{B}}^ * {\rm{) > 0 \;then }}\;\gamma ({\mu _{\min }}){\rm{ = }}{\gamma _{\rm{A}}}{\rm{,}}\gamma ({\mu _{\max }}){\rm{ = }}{\gamma _{\rm{B}}} \\ & \quad {\rm{if\; arg(}}{\gamma _{\rm{A}}}\gamma _{\rm{B}}^ * {\rm{) < 0 \; then }}\;\gamma ({\mu _{\min }}){\rm{ = }}{\gamma _{\rm{B}}}{\rm{,}}\gamma ({\mu _{\max }}){\rm{ = }}{\gamma _{\rm{A}}} \\ & {k_z} < 0: \\ & \quad {\rm{if \;arg(}}{\gamma _{\rm{A}}}\gamma _{\rm{B}}^ * {\rm{) < 0 \; then }}\;\gamma ({\mu _{\min }}){\rm{ = }}{\gamma _{\rm{A}}}{\rm{,}}\gamma ({\mu _{\max }}){\rm{ = }}{\gamma _{\rm{B}}} \\ & \quad {\rm{if \;arg(}}{\gamma _{\rm{A}}}\gamma _{\rm{B}}^ * {\rm{) > 0 \; then }}\;\gamma ({\mu _{\min }}){\rm{ = }}{\gamma _{\rm{B}}}{\rm{,}}\gamma ({\mu _{\max }}){\rm{ = }}{\gamma _{\rm{A}}} \end{aligned} \right\}$

(9)

其中， ${\mu _{\min }}$ 表示具有最小地体幅度比的极化方式，对应体散射占优极化方式复相干； ${\mu _{\max }}$ 表示具有最大地体幅度比的极化方式，对应表面散射占优极化方式复相干； ${k_z}$ 为垂直有效波束，取决于成像相对几何关系(垂直基线 ${B_ \bot }$ ，斜距 $R$ ，入射角 $\theta$ 和雷达波长 $\lambda$ )^[16]

${k_z}{\rm{ = }}\frac{{4\pi {B_ \bot }}}{{\lambda R\sin \theta }}$

(10)

2.3 森林高度反演方法

即便简化了模型参数和采用多基线PolInSAR数据增加了观测量，利用传统多维非线性迭代求解时间去相干半经验模型仍存在秩亏问题。因此本文采用一种外部数据辅助反演法^[8]，即先利用小范围真实森林高度数据辅助解算出模型参数 ${S_{{\rm{scene}}}}$ 和 ${C_{{\rm{scene}}}}$ ，然后代入模型中即可得到整个散射场景范围的森林高度结果。模型参数求解具体思路如下：对于给定模型参数初始值，利用训练数据中的 ${\mu _{\min }}$ 极化方式相干幅度结合式(4)可以得到反演森林高度结果 ${h_{{\rm{invert}}}}$ ，它与对应真实森林高度数据 ${h_{{\rm{real}}}}$ 确定的散点图如图2所示。理想情况下，两者数据散点应沿虚线 $y = x$ 分布，但实际上在初始模型参数误差存在情况下，两者散点点阵椭圆主轴与 $y = x$ 并非一致，而是存在一定的偏差。因此通过利用训练数据对其调整来寻求散射场景最佳模型参数。

图 2 反演森林高度与真实森林高度散点点阵椭圆示意图

Figure 2. Scatters ellipse between invert height and real height

下载: 全尺寸图片幻灯片

主成分分析思想^[17]为实现上述思路提供了契机，即通过对训练数据中 ${h_{{\rm{real}}}}$ 与 ${h_{{\rm{invert}}}}$ 这两个二维数据的协方差矩阵进行特征值分解，可以确定该二维数据降维后的主轴(也就是散点点阵椭圆的长轴)斜率 $k$

$\left. \begin{aligned} &{{X}} = \left[ {\begin{array}{*{20}{c}} {{\rm{Var}}({h_{{\rm{real}}}})}&{{\rm{Cov}}({h_{{\rm{real}}}},{h_{{\rm{invert}}}})} \\ {{\rm{Cov}}({h_{{\rm{invert}}}},{h_{{\rm{real}}}})}&{{\rm{Var}}({h_{{\rm{invert}}}})} \end{array}} \right] \\ & \quad = \left[ {\begin{array}{*{20}{c}} {P_{11}}&{P_{12}} \\ {P_{21}}&{P_{22}} \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {\lambda _{1}}\!\!&\!\!0 \\ 0\!\!&\!\!{\lambda _{2}} \end{array}} \right]{\left[ {\begin{array}{*{20}{c}} {P_{11}}\!\!&\!\!{P_{12}} \\ {P_{21}}\!\!&\!\!{P_{22}} \end{array}} \right]^{ - 1}} \\ & k = \frac{{P_{21}}}{{P_{11}}} \end{aligned}\right\} \!\!\!\!$

(11)

其中 $\lambda_1$ 和 $\lambda_2$ 为按降序排列的特征值， $P$ 为特征值对应的特征向量的元素。而点阵椭圆质心与虚线 $y = x$ 的偏差 $b$ 可以表示为

$b = \frac{{M({h_{{\rm{real}}}}) - M({h_{{\rm{invert}}}})}}{{[M({h_{{\rm{real}}}}) + M({h_{{\rm{invert}}}})]/2}}$

(12)

式中，M 表示取平均运算。

散点点阵椭圆主轴确定后，显然可以通过建立使逼近参数 $k$ , $b$ 分别趋近于1, 0的目标函数

${(k - 1)^2} + {(b - 0)^2} = \min$

(13)

该目标函数可以利用高斯-牛顿迭代算法进行非线性最小二乘求解，如式(14)所示

$\left[ {\begin{array}{*{20}{c}} {S_{{\rm{scene}}}^ * } \\ {C_{{\rm{scene}}}^ * } \end{array}} \right] = {({{J}}_0^{\rm T}{{{J}}_0})^{ - 1}}{{J}}_0^{\rm T}\left[ {\begin{array}{*{20}{c}} {1 - {k_0}} \\ {0 - {b_0}} \end{array}} \right] + \left[ {\begin{array}{*{20}{c}} {{S_{{\rm{scene0}}}}} \\ {{C_{{\rm{scene0}}}}} \end{array}} \right]$

(14)

式中， $*$ 表示最终迭代次数；通过给定模型参数初始值 ${S_{{\rm{scene0}}}}$ , ${C_{{\rm{scene0}}}}$ ，结合上述主成分思想可以得到初始点相应的 ${k_0}$ , ${b_0}$ 以及雅克比矩阵 ${{{J}}_0}$

${{{J}}_0} = {\left. {\left[ {\begin{array}{*{20}{c}} {\dfrac{{{\partial _k}}}{{{\partial _{{S_{{\rm{scene}}}}}}}}}&{\dfrac{{{\partial _k}}}{{{\partial _{{C_{{\rm{scene}}}}}}}}} \\ {\dfrac{{{\partial _b}}}{{{\partial _{{S_{{\rm{scene}}}}}}}}}&{\dfrac{{{\partial _b}}}{{{\partial _{{C_{{\rm{scene}}}}}}}}} \end{array}} \right]} \right|_{\begin{array}{*{20}{c}} {{S_{{\rm{scene0}}}}} \\ {{C_{{\rm{scene0}}}}} \end{array}}}$

(15)

然后将得到修正后的模型参数作为新的初始点进行下一次迭代，经过多次迭代后即可获得最佳模型参数 $S_{{\rm{scene}}}^ *$ , $C_{{\rm{scene}}}^ *$ ，迭代终止条件为( $\varepsilon$ 为经验阈值，本文设为 ${10^{ - 6}}$ )

$\left| {\left[ {\begin{array}{*{20}{c}} {S_{{\rm{scene}}}^ * } \\ {C_{{\rm{scene}}}^ * } \end{array}} \right] - \left[ {\begin{array}{*{20}{c}} {S_{{\rm{scene}}}^{( * - 1)}} \\ {C_{{\rm{scene}}}^{( * - 1)}} \end{array}} \right]} \right| < \varepsilon$

(16)

在利用训练数据求得时间去相干半经验模型参数后，对每个像元求解一元非线性方程得到整个散射场景内的森林高度结果。

2.4 多基线融合策略

时间去相干、体去相干以及其他噪声等因素会共同影响PolInSAR复相干性在复平面单位圆上的几何表达^[18]。在多基线配置下，不同干涉对在同一分辨单元内往往呈现出不同的相干区域结构(如图1所示)。而相干特性 $P$ 可以作为评价相干区域结构的指标^[19]

$P = \left| {\gamma ({\mu _{\min }}) - \gamma ({\mu _{\max }})} \right|\left| {\gamma ({\mu _{\min }}) + \gamma ({\mu _{\max }})} \right|$

(17)

式中， $\gamma ({\mu _{\min }})$ , $\gamma ({\mu _{\max }})$ 为2.2节所述相干区域长轴的两端点，分别对应体散射极化通道与地表散射极化通道的复相干系数。 $\left| {\gamma ({\mu _{\min }}) - \gamma ({\mu _{\max }})} \right|$ 即为极化相干区域的长轴，反映了不同极化相干点在复数单位圆的分离程度； $\left| {\gamma ({\mu _{\min }}) + \gamma ({\mu _{\max }})} \right|$ 为相干区域质心到坐标原点距离的2倍，反映了相干区域整体相干性的平均水平。因此，P 值越大说明该干涉对具有更好的相干性质量与极化分离度，反演的结果更为可靠。

通过相干特性指标P 甄选出不同干涉对在同一分辨单元内反演出的最优森林高度值作为多基线PolInSAR森林高度融合结果，多基线PolInSAR融合反演框架可表示为

$\max \left\| \begin{array}{l} {P^1}\left( {{\gamma ^1}\left( {{\mu _{\min }}} \right),{\gamma ^1}\left( {{\mu _{\max }}} \right)} \right)\\ {P^2}\left( {{\gamma ^2}({\mu _{\min }}),{\gamma ^2}({\mu _{\max }})} \right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\vdots \\ {P^N}\left( {{\gamma ^N}({\mu _{\min }}),{\gamma ^N}({\mu _{\max }})} \right) \end{array} \right\|$

(18)

式中，N 为极化干涉SAR观测基线数。

3. 实验与分析

3.1 实验区及数据分析

研究区域黄丰桥国有林场(27° $05'$ —27° $24'$ N, 113° $35'$ —113° $55'$ E)呈带状分布，横跨湖南省攸县东西两部(如图3所示)。该林场属亚热带季风湿润性气候区，年平均气温17.8 °C，年降水量1410.8 mm，大部分降雨发生于春、夏季。林场境内森林茂盛，拥有森林蓄积量90.12×10⁴ m³，森林覆盖率达90%。林分类型以针叶林为主，包括杉木、油松、落叶松等。

图 3 实验区：绿线范围为黄丰桥(HFQ)林场研究区域，蓝色虚线为ALOS-2 PALSAR-2影像范围，圆点为地面实测林分样地

Figure 3. The test site: the green line indicates the study area of HuangFengQiao (HFQ) forestry center, the blue dotted line indicates the ALOS-2 PALSAR-2 image range, and the dots are field measurement plots

下载: 全尺寸图片幻灯片

地面实测数据由中南林业科技大学于2016年6～7月采集得到，通过在林区范围内选取60个相互独立的林分样地以确保避免空间自相关，每个林分样地规格为30×30 m。树高则基于单木测高原理利用激光测高仪测得，林分高度范围为4.60～20.20 m，平均高度为13.24 m。本文通过随机采样，将60个林分样地数据随机分为45个训练数据(图3黄点所示)和15个验证数据(图3红点所示)两组。

多基线星载重轨PolInSAR数据是利用日本宇航局(JAXA)提供的5景覆盖研究区域的ALOS-2 PALSAR-2 L波段全极化数据。该SAR影像范围如图3蓝色虚线所示，获取时间为2016年6月至8月，获取模式为StripMap2(SM2)，影像主要参数信息如表1所示。将5景SAR影像组成3个时间基线为14天的干涉影像对(BL1, BL2, BL3)，然后各自进行配准处理，并进行公共带通滤波以确保去除几何去相干。相干性以11×11窗口进行估计，并应用Boxcar滤波进行平滑处理以消除斑点效应。最后利用SRTM DEM对SAR影像进行地理编码，并将其重采样至与DEM空间分辨率一致(30×30 m)。图4为3个干涉对的HV极化和 ${\mu _{\min }}$ 极化的相干性统计图，由统计图可见不同干涉对的相干性均较低，说明研究区域受时间去相干影响较为严重。

表 1 ALOS-2 PALSAR-2参数信息

Table 1. Parameter information of ALOS-2 PALSAR-2

日期(2016年)	垂直有效波数(rad/m)	时间基线(天)	距离向/方位向分辨率(m)	中心入射角 (°)	极化方式
0616—0630 (BL1)	0.013～0.015
0630—0714 (BL2)	0.010～0.011	14	2.86/2.97	38.99	Full
0811—0825 (BL3)	0.009～0.010

下载: 导出CSV

| 显示表格

图 4 相干性统计图

Figure 4. The histograms of coherence

下载: 全尺寸图片幻灯片

3.2 实验结果与分析

以选取的15个验证林分的实测森林高度(H-field)对反演结果(H-invert)进行分析评价，图5为3个干涉对利用HV极化反演得到的散点图结果，均方根误差RMSE分别为：4.20 m, 4.03 m和3.42 m。利用 ${\mu _{\min }}$ 极化方式反演的验证结果如图6所示，3个干涉对的反演精度分别提高了：20%, 17%和12%，除此之外，相关系数 ${R^2}$ 也分别有所提高。分析认为采用全极化数据结合PolInSAR相干优化算法扩展了极化空间，相比已有方法中选用的HV极化， ${\mu _{\min }}$ 极化含有更少地表散射贡献，更贴近时间去相干半经验模型推导过程中基于“零”地体幅度比的关键假设。

图 5 单基线InSAR反演高度与验证数据散点图

Figure 5. Scatterplot comparison between inversion height of single baseline InSAR and validation data

下载: 全尺寸图片幻灯片

图 6 单基线PolInSAR反演高度与验证数据散点图

Figure 6. Scatterplot comparison between inversion height of single baseline PolInSAR and validation data

下载: 全尺寸图片幻灯片

从上述单基线森林高度反演结果看，不同干涉对反演整体精度较为接近，但是对于同一林分在利用不同干涉对反演的结果却存在明显差异。因此，当多基线数据可用时，我们进一步在单基线PolInSAR森林高度反演结果的基础上挖掘PolInSAR数据本身特性并对其森林高度反演能力进行评判。与时间去相干相关的参数 ${S_{{\rm{scene}}}}$ 和 ${C_{{\rm{scene}}}}$ 共同反映了散射场景内的时间去相干影响水平，其中 ${S_{{\rm{scene}}}}$ 与植被体层介电特性变化相关， ${C_{{\rm{scene}}}}$ 反映了植被体层随机运动引起时间去相关水平。表2即为单基线PolInSAR模型参数解算结果，对于不同干涉对， ${S_{{\rm{scene}}}}$ 越小，表明该基线在散射场景内植被体层介电变化(降水等引起)越显著； ${C_{{\rm{scene}}}}$ 越小，则表明植被体层随机运动(风动等引起)越强烈。从图4相干性统计图也可以看出，干涉对BL1相干性相对更低，受时间去相干的影响更为严重。因此，在不同时间去相干以及其他噪声影响下，每个干涉对在同一分辨单元内会具有不同的的相干特性，呈现出优劣不同的相干区域结构。

表 2 单基线PolInSAR模型参数解算结果

Table 2. Model parameter results of single baseline PolInSAR inversion

模型参数	BL1	BL2	BL3
${S_{{\rm{scene}}}}$	0.69	0.78	0.78
${C_{{\rm{scene}}}}$	9.88	10.08	11.14

下载: 导出CSV

| 显示表格

3个干涉对在验证林分的相干特性P值、反演森林高度值以及多基线融合森林高度值如表3所示，从整体看，根据相干特性P值大小从3个单基线PolInSAR反演结果中甄选出的森林高度结果更接近于实测真实森林高度。整个实验区的多基线PolInSAR融合反演结果以及精度评定如图7所示，均方根误差RMSE为2.05 m，相比于已有的方法，本文提出的多基线PolInSAR融合反演策略精度至少提高了40%(与图5中BL3基线结果对比)，同时，相关系数也提升至0.81。

表 3 3个干涉对的相干特性P值以及森林高度值

Table 3. Coherence characteristic P-value and forest heights for three interferometric pairs

林分样地编号	BL1 P值 / 森林高度(m)	BL2 P值 / 森林高度(m)	BL3 P值 / 森林高度(m)	多基线融合结果(m)	实测森林高度(m)
1	0.130 / 17.82	0.113 / 17.02	0.081 / 16.89	17.82	14.43
2	0.116 / 14.38	0.104 / 15.30	0.091 / 16.52	14.38	14.20
3	0.092 / 12.46	0.075 / 15.83	0.135 / 11.34	11.34	9.80
4	0.103 / 15.21	0.111 / 15.34	0.119 / 14.19	14.19	16.00
5	0.106 / 6.86	0.106 / 7.24	0.131 / 8.31	8.31	10.70
6	0.110 / 12.98	0.083 / 14.67	0.118 / 11.89	11.89	13.50
7	0.114 / 13.35	0.096 / 15.30	0.101 / 16.10	13.35	13.43
8	0.079 / 14.29	0.106 / 16.15	0.117 / 16.22	16.22	16.95
9	0.069 / 12.12	0.090 / 17.63	0.060 / 12.30	17.63	20.10
10	0.104 / 12.33	0.089 / 13.67	0.102 / 11.72	12.33	15.60
11	0.075 / 18.34	0.103 / 16.75	0.154 / 10.16	10.16	13.30
12	0.113 / 9.08	0.134 / 9.46	0.106 / 12.69	9.46	11.00
13	0.086 / 13.76	0.096 / 9.07	0.109 / 16.00	16.00	16.40
14	0.197 / 10.17	0.230 / 8.71	0.186 / 9.51	8.71	6.00
15	0.103 / 14.59	0.064 / 19.17	0.128 / 15.40	15.40	14.70

下载: 导出CSV

| 显示表格

图 7 多基线PolInSAR融合反演

Figure 7. Multi baseline PolInSAR fusion inversion

下载: 全尺寸图片幻灯片

4. 结束语

在多基线全极化数据可用条件下，弥补单基线InSAR观测信息不足以及几何结构单一的问题，对于反演结果整体精度提升具有重要作用。本文提出了一种星载重轨多基线PolInSAR反演森林高度的策略，对InSAR极化空间和观测几何空间进行扩展，主要结论如下：

(1) 该方法利用MCD相干优化算法获得对体散射最为敏感的极化方式，并基于时间去相干半经验模型进行森林高度反演，使每条单基线反演精度在一定程度上都有所提高。

(2) 利用由相干特性指标P确定的相干区域最优准则可以优选出同一分辨单元内最优的单基线森林高度反演结果。因此，相比仅利用单基线单一极化反演方法，多基线PolInSAR融合策略具有更好的稳定性，精度也更高。

图 1 机载多通道雷达前视成像三维几何结构图

Figure 1. 3D geometry for airborne multi-channel radar forward-looking imaging

下载: 全尺寸图片幻灯片

图 2 收发天线示意图

Figure 2. Schematic diagram of transmitting and receiving antennas

下载: 全尺寸图片幻灯片

图 3 迭代超分辨多通道雷达前视成像处理流程图

Figure 3. Flow chart of iterative super-resolution forward-looking imaging processing of multi-channel radar

下载: 全尺寸图片幻灯片

图 4 点目标前视成像结果

Figure 4. Forward-looking imaging results for point targets

下载: 全尺寸图片幻灯片

图 5 点扩散函数仿真

Figure 5. Simulation of Point Spread Function (PSF)

下载: 全尺寸图片幻灯片

图 6 第1组场景仿真成像结果对比

Figure 6. Forward-looking imaging results for the first scenario

下载: 全尺寸图片幻灯片

图 7 第2组场景仿真成像结果对比

Figure 7. Forward-looking imaging results for the second scenario

下载: 全尺寸图片幻灯片

图 8 区域1成像结果放大图

Figure 8. Enlarged image of zone 1

下载: 全尺寸图片幻灯片

图 9 区域2成像结果放大图

Figure 9. Enlarged image of zone 2

下载: 全尺寸图片幻灯片

图 10 实测数据成像结果

Figure 10. Forward-looking imaging results for the measured data

下载: 全尺寸图片幻灯片

图 11 实测数据成像结果细节图

Figure 11. Enlarged imaging results for the measured data

下载: 全尺寸图片幻灯片

图 12 海面区域实测数据成像结果

Figure 12. Forward-looking imaging results for the measured data of sea surface

下载: 全尺寸图片幻灯片

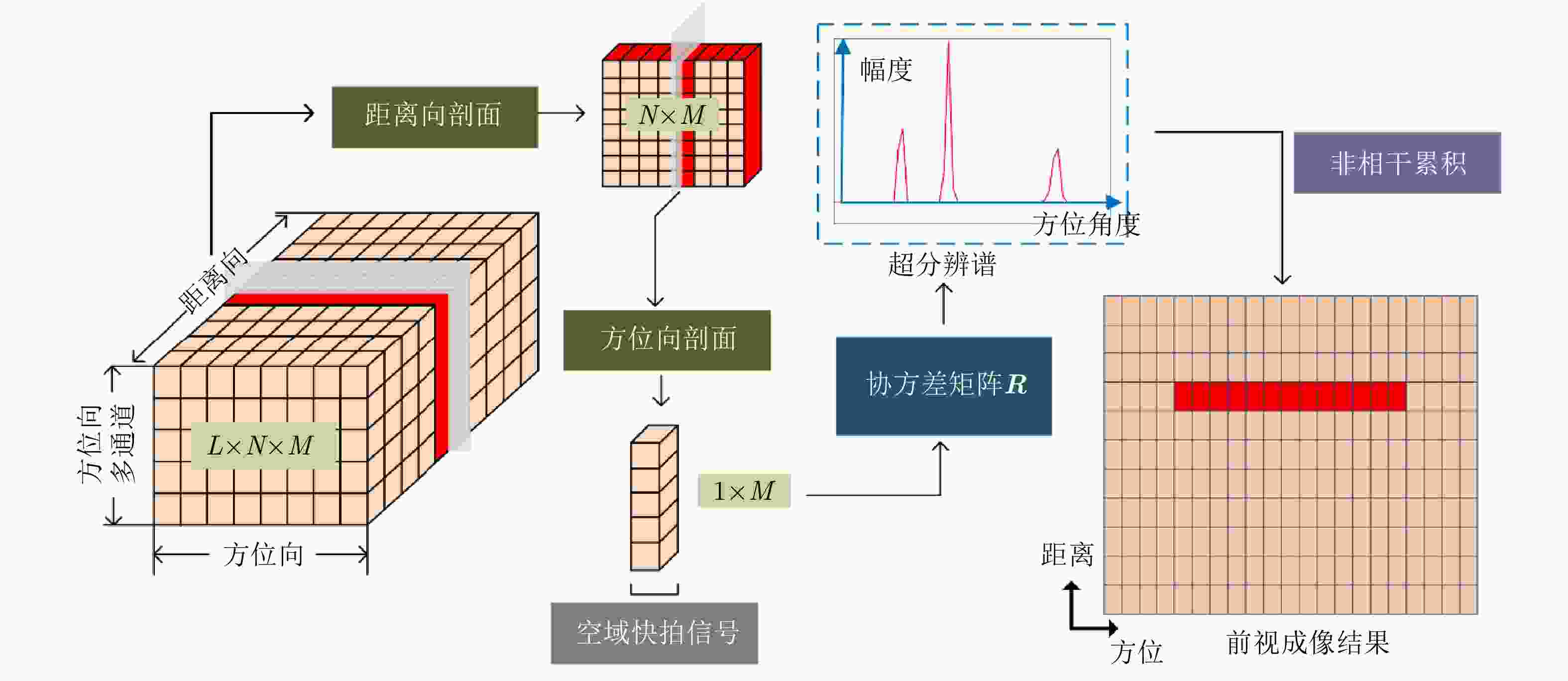
1 迭代超分辨算法流程

1. Flow chart of iterative super-resolution algorithm

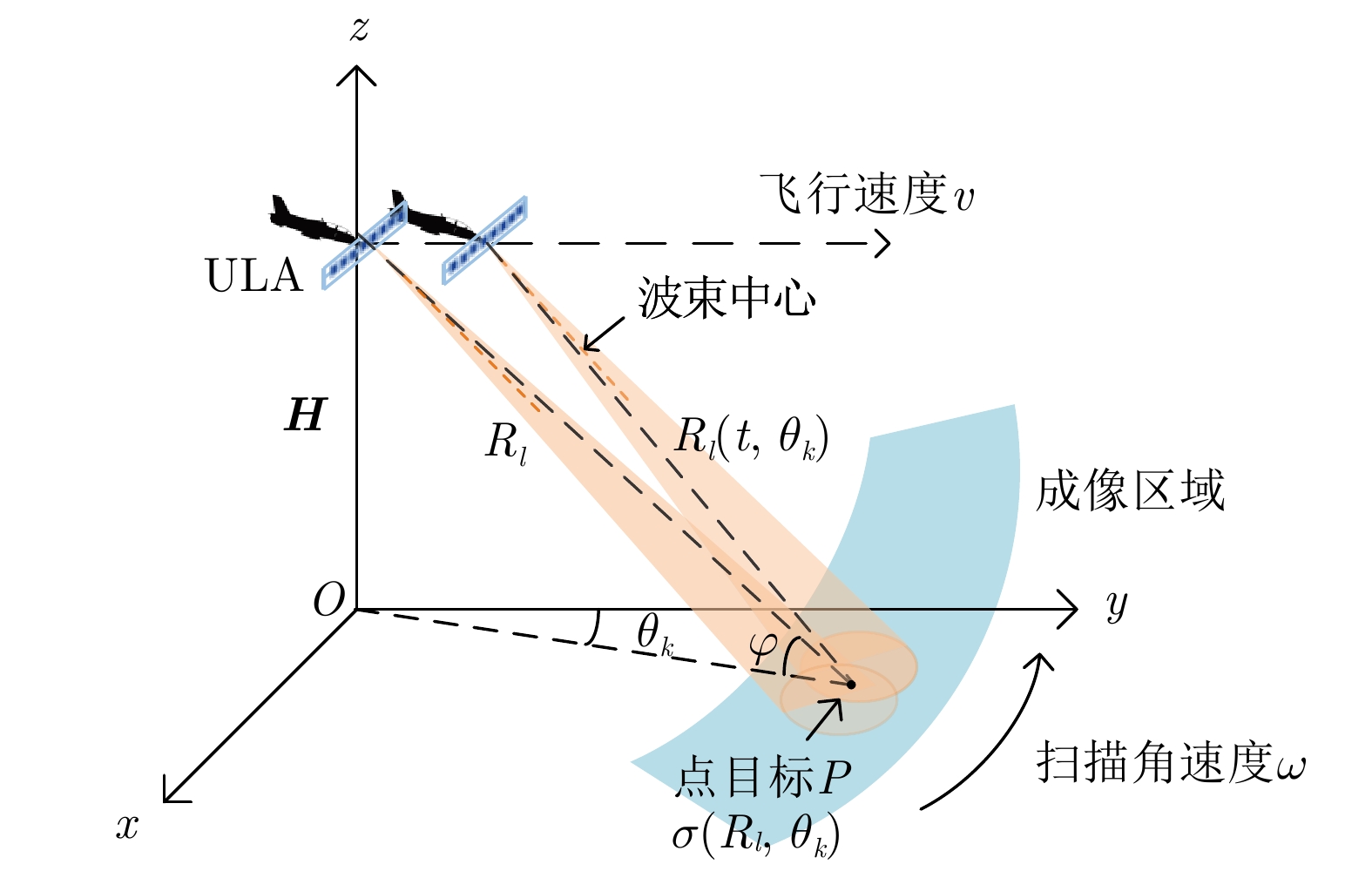
根据当前波束中心 $\bar \theta$ ，由式(12)计算得空域不混叠范围；在此范　围上构建空域导引矢量矩阵A
初始化：
${\hat {\boldsymbol{x}}_1} = {{\boldsymbol{A}}^{\text{H} } }{\boldsymbol{s}}$ ，基于此计算信号的空间功率分布矩阵 ${{\boldsymbol{P}}_1}$
重复以下操作：
${{\boldsymbol{w}}_{t + 1} } = {\left( {{\boldsymbol{A}}{{\boldsymbol{P}}_t}{{\boldsymbol{A}}^{\text{H} } } + {{\boldsymbol{R}}_{\rm{N}}} } \right)^{ - 1} }{\boldsymbol{A}}{{\boldsymbol{P}}_t}$
${\hat {\boldsymbol{x} }_{t + 1} } = {\boldsymbol{w} }_{t + 1} ^{\text{H} }{\boldsymbol{s} }$
${\hat {\boldsymbol{P}}_{t + 1} } = [{\hat {\boldsymbol{x}}_t}\hat {\boldsymbol{x}}_t^{\text{H} }] \odot {{\boldsymbol{I}}_{K \times K} }$
直到收敛

下载: 导出CSV

表 1 点目标仿真实验系统参数

Table 1. Point target simulation parameters

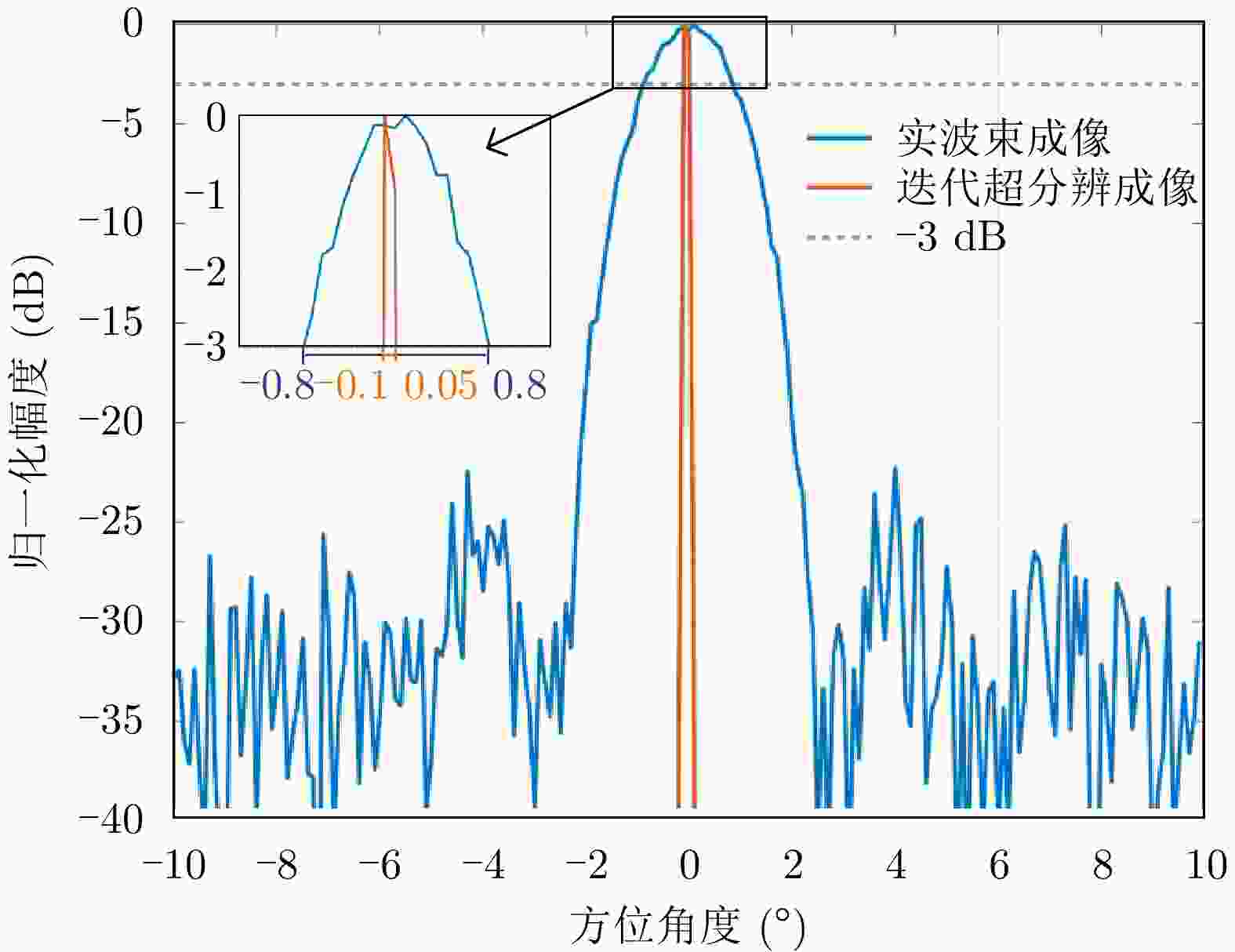
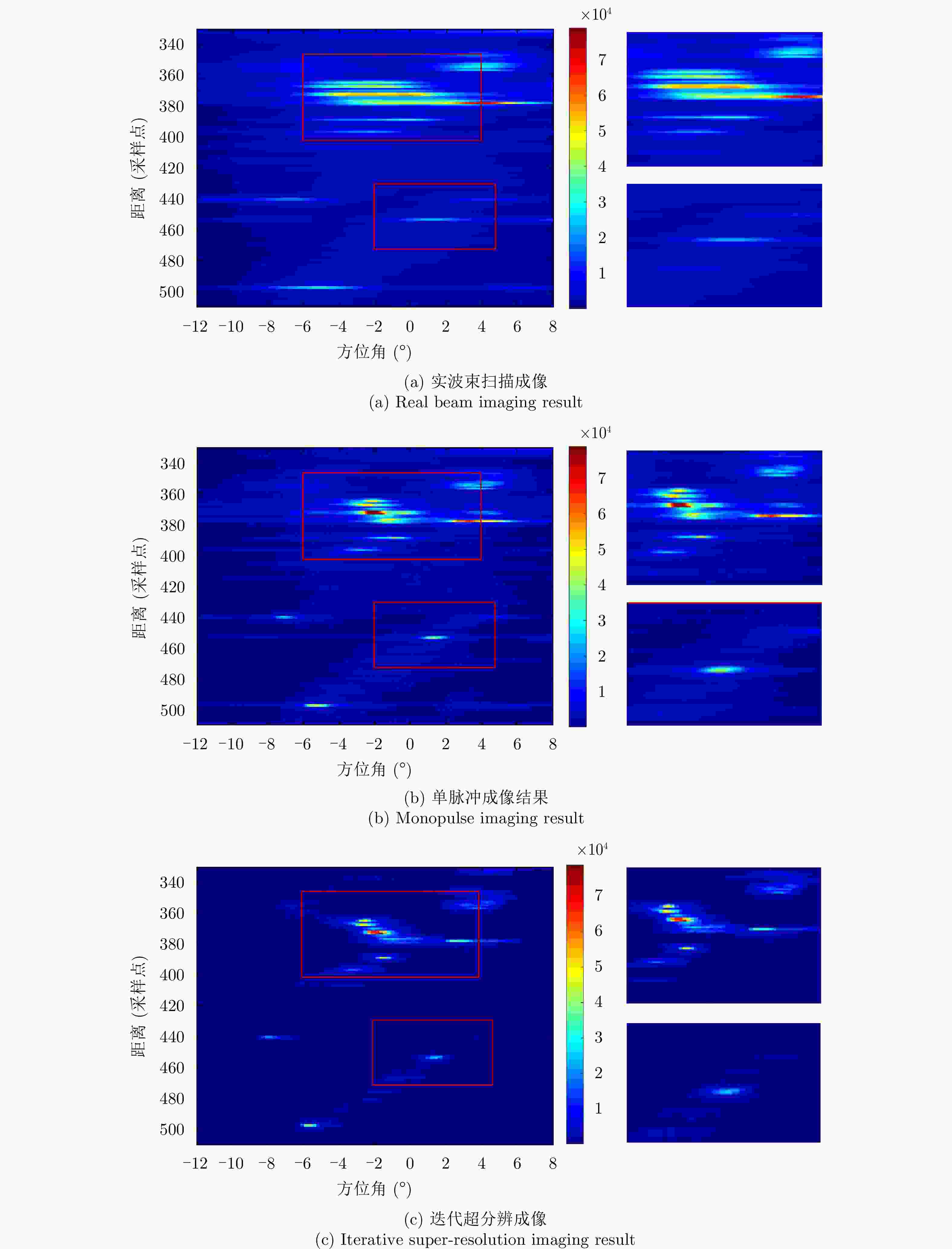
参数名称	参数值
雷达波长(m)	0.03
系统带宽(MHz)	20
采样频率(MHz)	30
脉冲重复频率(Hz)	1000
通道数	8
通道间隔(m)	0.09
波束方位向主瓣宽度(°)	2.11
机载平台运动速度(m/s)	100
波束扫描速度(°/s)	100
前视扫描范围(°)	–10～10
目标点距离(m)	1000, 1250, 1250, 1250, 1500
目标点方位角(°)	0, –3, –2, 3, 0
目标点SNR (dB)	20, 25, 25, 20, 20

下载: 导出CSV

表 2 场景仿真实验系统参数

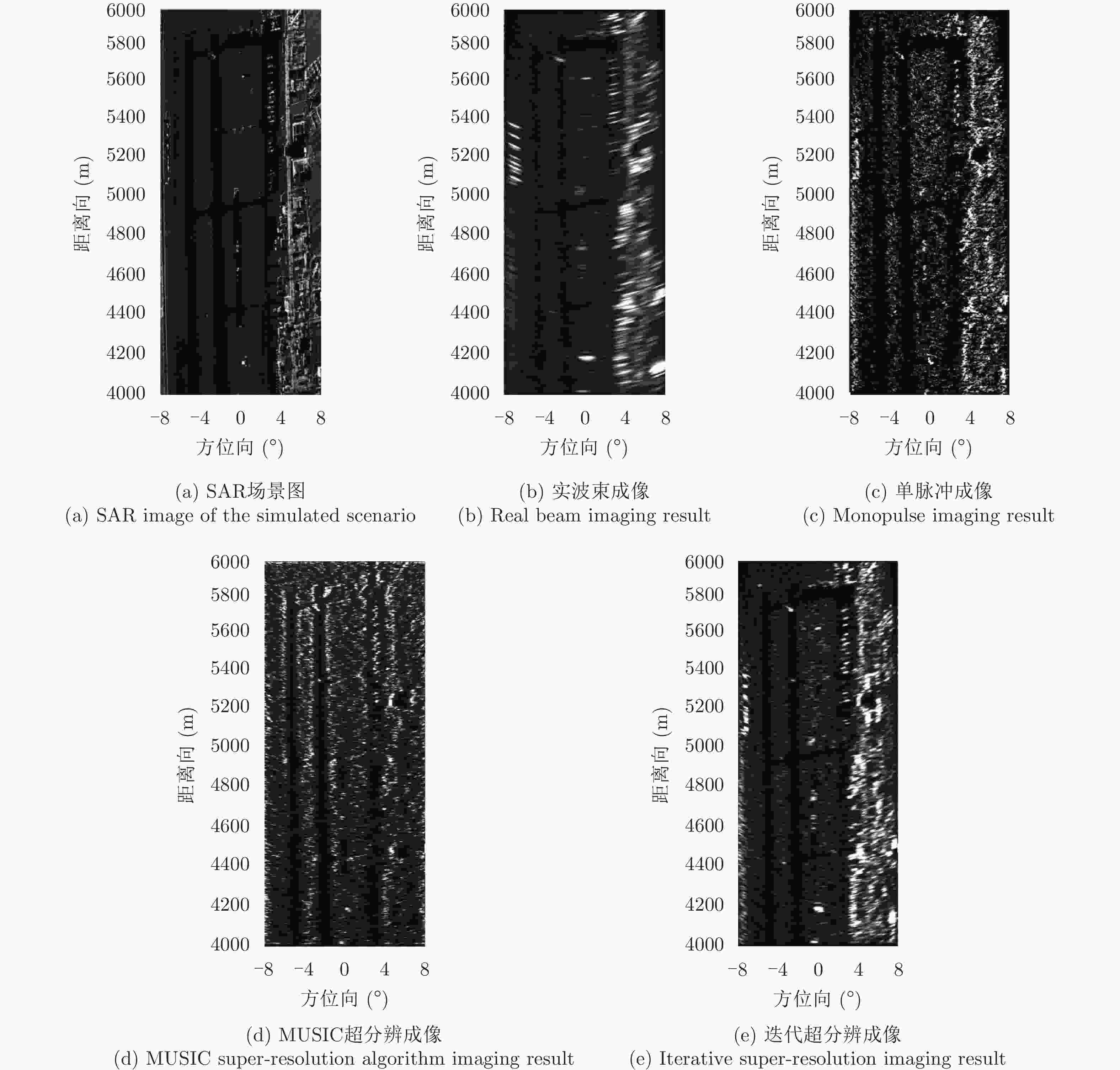
Table 2. Parameters of simulation for simulated scenario

参数名称	参数值 (场景1)	参数值 (场景2)
雷达波长(m)	0.03	0.03
系统带宽(MHz)	40	40
采样频率(MHz)	50	50
脉冲重复频率(Hz)	1000	1000
通道数	8	8
通道间隔(m)	0.045	0.120
波束方位向主瓣宽度(°)	4.23	1.59
波束扫描范围(°)	–20～20	–8～8
机载平台运动速度(m/s)	100	80

下载: 导出CSV

表 3 实测数据系统参数

Table 3. Parameters of measured data

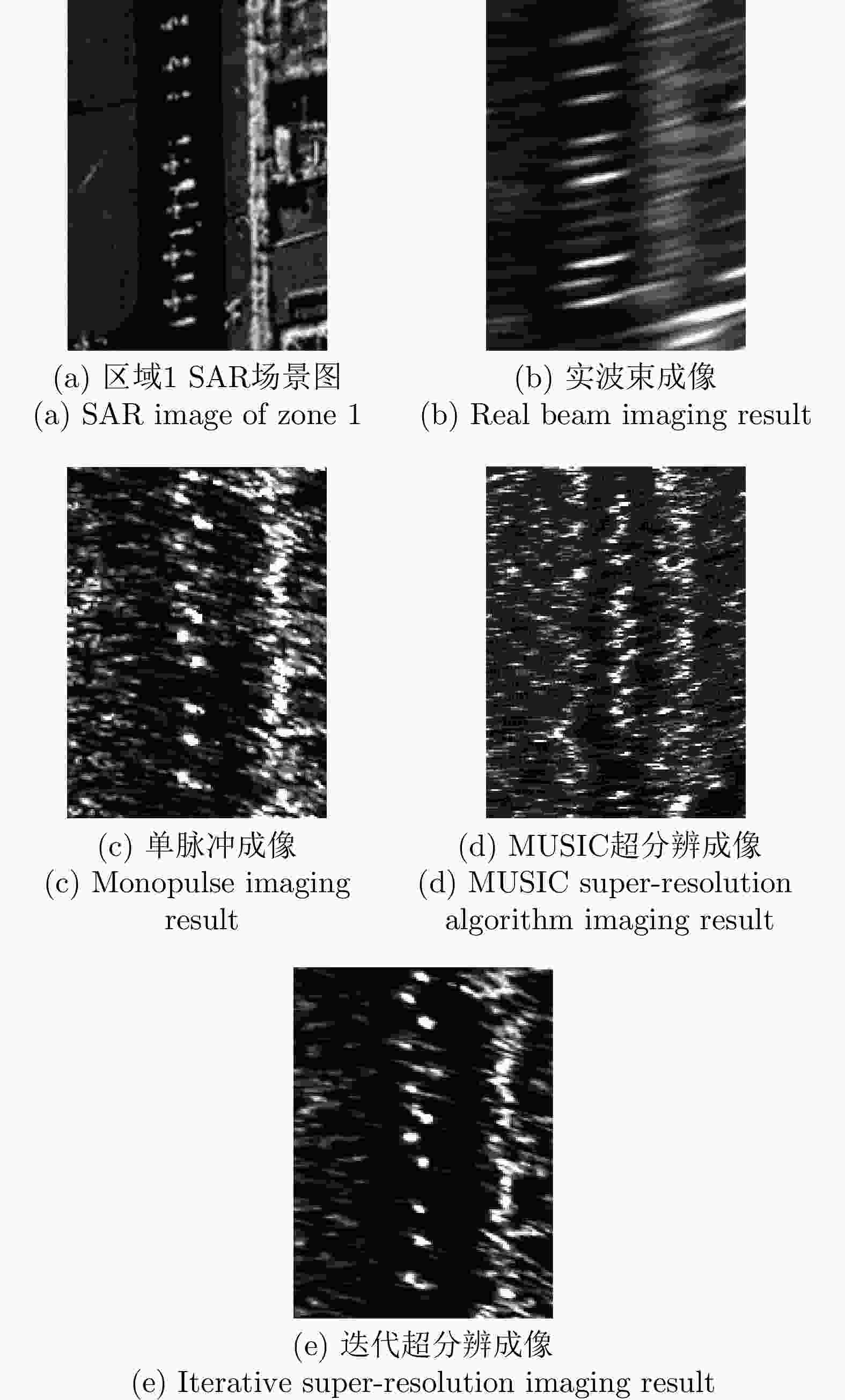
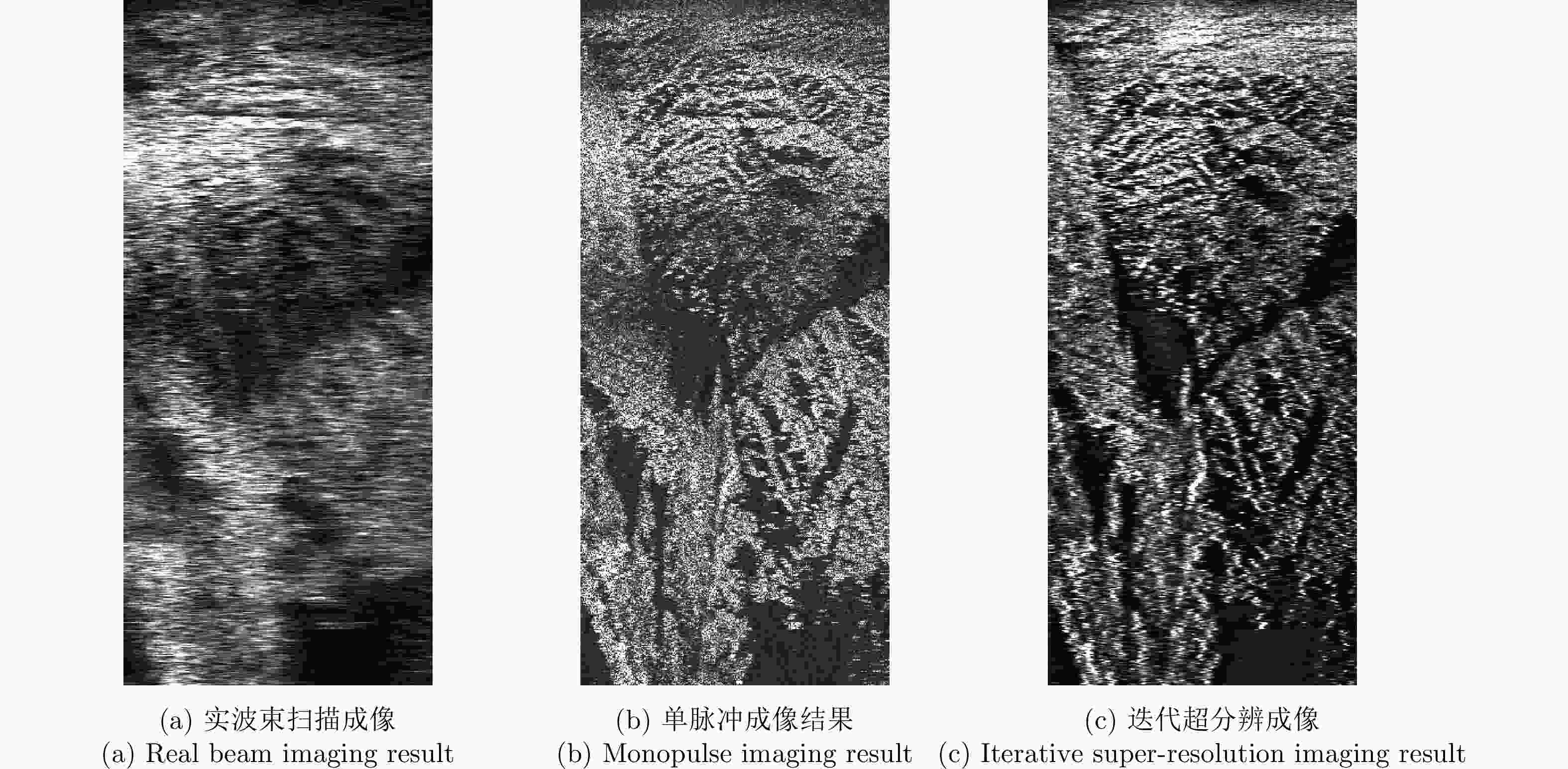
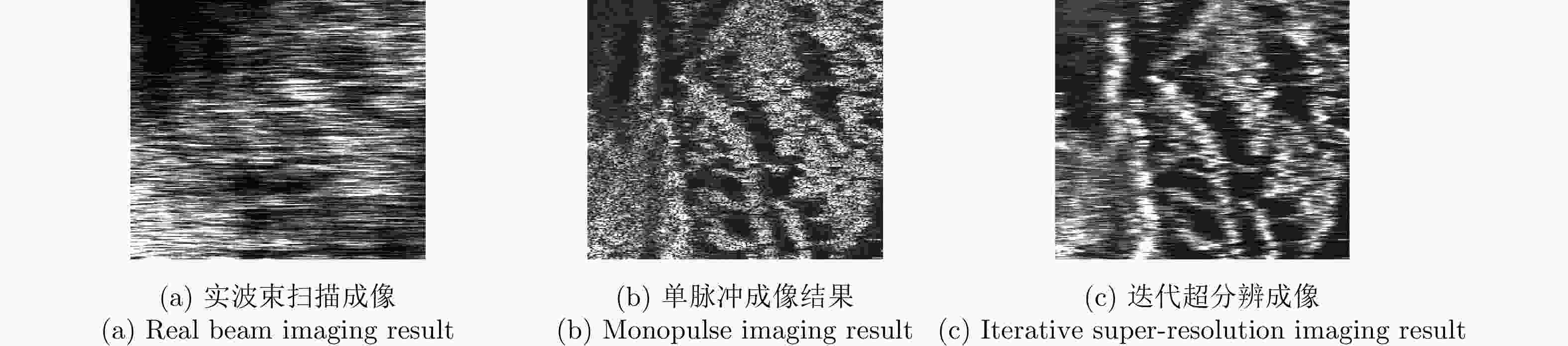
参数名称	第1组实测数据	第2组实测数据
雷达波段	X	Ku
脉冲重复频率(Hz)	417	3125
通道数	4	4
通道间隔(m)	0.150	0.037
波束主瓣宽度(°)	2.42	6.31
波束扫描范围(°)	–14～14	–20～20
扫描速度(°/s)	80	70
机载平台速度(m/s)	145	68

下载: 导出CSV

表 4 地面实测数据成像结果图像熵和对比度对比

Table 4. Entropy and contrast of the measured data results

成像方法	图像熵	对比度
实波束成像	3.8625	4.42
单脉冲成像结果	1.6530	8.12
迭代超分辨成像	1.2543	9.59

下载: 导出CSV

参考文献(32)

[1]	杨建宇. 雷达对地成像技术多向演化趋势与规律分析[J]. 雷达学报, 2019, 8(6): 669–692. doi: 10.12000/JR19099 YANG Jianyu. Multi-directional evolution trend and law analysis of radar ground imaging technology[J]. Journal of Radars, 2019, 8(6): 669–692. doi: 10.12000/JR19099
[2]	樊晨阳, 贺思三, 郭乾. 雷达前视成像技术的研究现状[J]. 电光与控制, 2021, 28(9): 59–64. doi: 10.3969/j.issn.1671-637X.2021.09.013 FAN Chenyang, HE Sisan, and GUO Qian. Research status of radar forward-looking imaging technology[J]. Electronics Optics&Control, 2021, 28(9): 59–64. doi: 10.3969/j.issn.1671-637X.2021.09.013
[3]	MOREIRA A, PRATS-IRAOLA P, YOUNIS M, et al. A tutorial on synthetic aperture radar[J]. IEEE Geoscience and Remote Sensing Magazine, 2013, 1(1): 6–43. doi: 10.1109/MGRS.2013.2248301
[4]	陈洪猛. 机载广域监视雷达高分辨成像方法研究[D]. [博士论文], 西安电子科技大学, 2016. CHEN Hongmeng. Study of high resolution imaging for airborne wide area surveillance radar[D]. [Ph. D. dissertation], Xidian University, 2016.
[5]	ONAT E and ÖZKAZANÇ Y. An analysis of Doppler beam sharpening technique used in fighter aircraft[C]. The 26th Signal Processing and Communications Applications Conference, Izmir, Turkey, 2018: 1–4.
[6]	MAO Deqing, ZHANG Yongchao, ZHANG Yin, et al. Doppler beam sharpening using estimated Doppler centroid based on edge detection and fitting[J]. IEEE Access, 2019, 7: 123604–123615. doi: 10.1109/ACCESS.2019.2937992
[7]	杨志伟, 贺顺, 廖桂生. 机载单通道雷达实波束扫描的前视探测[J]. 航空学报, 2012, 33(12): 2240–2245. YANG Zhiwei, HE Shun, and LIAO Guisheng. Forward-looking detection for airborne single-channel radar with beam scanning[J]. Acta Aeronautica et Astronautica Sinica, 2012, 33(12): 2240–2245.
[8]	温晓杨, 匡纲要, 胡杰民, 等. 基于实波束扫描的相控阵雷达前视成像[J]. 航空学报, 2014, 35(7): 1977–1991. doi: 10.7527/S1000-6893.2013.0545 WEN Xiaoyang, KUANG Gangyao, HU Jiemin, et al. Forward-looking imaging based on real beam scanning phased array radars[J]. Acta Aeronautica et Astronautica Sinica, 2014, 35(7): 1977–1991. doi: 10.7527/S1000-6893.2013.0545
[9]	SUTOR T, WITTE F, and MOREIRA A. New sector imaging radar for enhanced vision: SIREV[C]. SPIE 3691, Enhanced and Synthetic Vision, Orlando, USA, 1999.
[10]	吴迪, 朱岱寅, 田斌, 等. 单脉冲成像算法性能分析[J]. 航空学报, 2012, 33(10): 1905–1914. WU Di, ZHU Daiyin, TIAN Bin, et al. Performance evaluation for monopulse imaging algorithm[J]. Acta Aeronautica et Astronautica Sinica, 2012, 33(10): 1905–1914.
[11]	吴迪, 杨成杰, 朱岱寅, 等. 一种用于单脉冲成像的自聚焦算法[J]. 电子学报, 2016, 44(8): 1962–1968. doi: 10.3969/j.issn.0372-2112.2016.08.027 WU Di, YANG Chengjie, ZHU Daiyin, et al. An autofocusing algorithm for monopulse imaging[J]. Acta Electronica Sinica, 2016, 44(8): 1962–1968. doi: 10.3969/j.issn.0372-2112.2016.08.027
[12]	李悦丽, 马萌恩, 赵崇辉, 等. 基于单脉冲雷达和差通道多普勒估计的前视成像[J]. 雷达学报, 2021, 10(1): 131–142. doi: 10.12000/JR20111 LI Yueli, MA Meng’en, ZHAO Chonghui, et al. Forward-looking imaging via Doppler estimates of sum-difference measurements in scanning monopulse radar[J]. Journal of Radars, 2021, 10(1): 131–142. doi: 10.12000/JR20111
[13]	WALTERSCHEID I, ESPETER T, GIERULL C, et al. Results and analysis of hybrid bistatic SAR experiments with spaceborne, airborne and stationary sensors[C]. 2009 IEEE International Geoscience and Remote Sensing Symposium, Cape Town, South Africa, 2009: II-238–II-241.
[14]	YANG Jianyu, HUANG Yulin, YANG Haiguang, et al. A first experiment of airborne bistatic forward-looking SAR - Preliminary results[C]. 2013 IEEE International Geoscience and Remote Sensing Symposium, Melbourne, Australia, 2013: 4202–4204.
[15]	LIU Zhutian, YE Hongda, LI Zhongyu, et al. Optimally matched space-time filtering technique for BFSAR nonstationary clutter suppression[J]. IEEE Transactions on Geoscience and Remote Sensing, 2022, 60: 5210617. doi: 10.1109/TGRS.2021.3090462
[16]	武俊杰, 孙稚超, 杨建宇, 等. 基于GF-3照射的星机双基SAR成像及试验验证[J]. 雷达科学与技术, 2021, 19(3): 241–247. doi: 10.3969/j.issn.1672-2337.2021.03.002 WU Junjie, SUN Zhichao, YANG Jianyu, et al. Spaceborne-airborne bistatic SAR using GF-3 illumination—technology and experiment[J]. Radar Science and Technology, 2021, 19(3): 241–247. doi: 10.3969/j.issn.1672-2337.2021.03.002
[17]	ZHANG Yongchao, MAO Deqing, ZHANG Qian, et al. Airborne forward-looking radar super-resolution imaging using iterative adaptive approach[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2019, 12(7): 2044–2054. doi: 10.1109/JSTARS.2019.2920859
[18]	ZHANG Qiping, ZHANG Yin, HUANG Yulin, et al. TV-sparse super-resolution method for radar forward-looking imaging[J]. IEEE Transactions on Geoscience and Remote Sensing, 2020, 58(9): 6534–6549. doi: 10.1109/TGRS.2020.2977719
[19]	TUO Xingyu, ZHANG Yin, HUANG Yulin, et al. Fast sparse-TSVD super-resolution method of real aperture radar forward-looking imaging[J]. IEEE Transactions on Geoscience and Remote Sensing, 2021, 59(8): 6609–6620. doi: 10.1109/TGRS.2020.3027053
[20]	HUO Weibo, TUO Xingyu, ZHANG Yin, et al. Balanced tikhonov and total variation deconvolution approach for radar forward-looking super-resolution imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 3505805. doi: 10.1109/lgrs.2021.3072389
[21]	毛德庆. 机载雷达扫描波束超分辨成像方法研究[D]. [博士论文], 电子科技大学, 2022. MAO Deqing. Research on scanning beam super-resolution imaging methods for airborne radar[D]. [Ph. D. dissertation], University of Electronic Science and Technology of China, 2022.
[22]	ZHANG Yin, SHEN Jiahao, TUO Xingyu, et al. Scanning radar forward-looking superresolution imaging based on the Weibull distribution for a sea-surface target[J]. IEEE Transactions on Geoscience and Remote Sensing, 2022, 60: 5116111. doi: 10.1109/TGRS.2022.3194118
[23]	GUPTA I J, BEALS M J, and MOGHADDAR A. Data extrapolation for high resolution radar imaging[J]. IEEE Transactions on Antennas and Propagation, 1994, 42(11): 1540–1545. doi: 10.1109/8.362783
[24]	CHO B L and SUN S G. Cross-range resolution improvement in forward-looking imaging radar using autoregressive model-based data extrapolation[J]. IET Radar,Sonar&Navigation, 2015, 9(8): 933–941. doi: 10.1049/IET-RSN.2014.0495
[25]	张洁. 阵列雷达前视成像技术研究[D]. [硕士论文], 南京航空航天大学, 2018. ZHANG Jie. Study on array radar forward-looking imaging techniques[D]. [Master dissertation], Nanjing University of Aeronautics and Astronautics, 2018.
[26]	ZHANG Jie, WU Di, ZHU Daiyin, et al. An airborne/missile-borne array radar forward-looking imaging algorithm based on super-resolution method[C]. The 10th International Congress on Image and Signal Processing, BioMedical Engineering and Informatics, Shanghai, China, 2017: 1–5.
[27]	BLUNT S D and GERLACH K. Adaptive pulse compression via MMSE estimation[J]. IEEE Transactions on Aerospace and Electronic Systems, 2006, 42(2): 572–584. doi: 10.1109/TAES.2006.1642573
[28]	BLUNT S D, CHAN T, and GERLACH K. Robust DOA estimation: The reiterative superresolution (RISR) algorithm[J]. IEEE Transactions on Aerospace and Electronic Systems, 2011, 47(1): 332–346. doi: 10.1109/TAES.2011.5705679
[29]	YARDIBI T, LI Jian, STOICA P, et al. Source localization and sensing: A nonparametric iterative adaptive approach based on weighted least squares[J]. IEEE Transactions on Aerospace and Electronic Systems, 2010, 46(1): 425–443. doi: 10.1109/TAES.2010.5417172
[30]	ROBERTS W, STOICA P, LI Jian, et al. Iterative adaptive approaches to MIMO radar imaging[J]. IEEE Journal of Selected Topics in Signal Processing, 2010, 4(1): 5–20. doi: 10.1109/JSTSP.2009.2038964
[31]	张小飞, 张胜男, 徐大专. 自适应对角线加载的波束形成算法[J]. 中国空间科学技术, 2007, 27(2): 66–71. doi: 10.3321/j.issn:1000-758X.2007.02.011 ZHANG Xiaofei, ZHANG Shengnan, and XU Dazhuan. Adaptive diagonal loading beamforming algorithm[J]. Chinese Space Science and Technology, 2007, 27(2): 66–71. doi: 10.3321/j.issn:1000-758X.2007.02.011
[32]	CARLSON B D. Covariance matrix estimation errors and diagonal loading in adaptive arrays[J]. IEEE Transactions on Aerospace and Electronic Systems, 1988, 24(4): 397–401. doi: 10.1109/7.7181

施引文献

期刊类型引用(1)
1. 毛德庆，杨建宇，杨明杰，张永超，张寅，黄钰林. IAA-Net：一种实孔径扫描雷达迭代自适应角超分辨成像方法. 雷达学报. 2024(05): 1073-1091 . 本站查看
其他类型引用(0)

资源附件(0)

访问统计

图(12) / 表(5)

计量

文章访问数: 767
HTML全文浏览量: 253
PDF下载量: 266
被引次数: 1

1. 引言
2. 多基线PolInSAR森林高度反演策略
2.1 时间去相干半经验模型
2.2 MCD相干优化算法
2.3 森林高度反演方法
2.4 多基线融合策略
3. 实验与分析
3.1 实验区及数据分析
3.2 实验结果与分析
4. 结束语

基于机载多通道雷达迭代超分辨估计的前视成像

DOI: 10.12000/JR23085

通讯作者:
吴迪 wudi82@nuaa.edu.cn

朱岱寅 zhudy@nuaa.edu.cn

计量

Forward-looking Imaging via Iterative Super-resolution Estimation in Airborne Multi-channel Radar

Corresponding author: WU Di, wudi82@nuaa.edu.cn; ZHU Daiyin, zhudy@nuaa.edu.cn

1. 引言

2. 多基线PolInSAR森林高度反演策略

2.1 时间去相干半经验模型

2.2 MCD相干优化算法

2.3 森林高度反演方法

2.4 多基线融合策略

3. 实验与分析

3.1 实验区及数据分析

3.2 实验结果与分析

4. 结束语

期刊类型引用(1)

其他类型引用(0)

计量

目录

1. 引言

2. 多基线PolInSAR森林高度反演策略

2.1 时间去相干半经验模型

2.2 MCD相干优化算法

2.3 森林高度反演方法

2.4 多基线融合策略

3. 实验与分析

3.1 实验区及数据分析

3.2 实验结果与分析

4. 结束语

期刊介绍

联系我们

基于机载多通道雷达迭代超分辨估计的前视成像

DOI: 10.12000/JR23085

通讯作者: 吴迪 wudi82@nuaa.edu.cn 朱岱寅 zhudy@nuaa.edu.cn

计量

出版历程

Forward-looking Imaging via Iterative Super-resolution Estimation in Airborne Multi-channel Radar

Corresponding author: WU Di, wudi82@nuaa.edu.cn; ZHU Daiyin, zhudy@nuaa.edu.cn

1. 引言

2. 多基线PolInSAR森林高度反演策略

2.1 时间去相干半经验模型

2.2 MCD相干优化算法

2.3 森林高度反演方法

2.4 多基线融合策略

3. 实验与分析

3.1 实验区及数据分析

3.2 实验结果与分析

4. 结束语

期刊类型引用(1)

其他类型引用(0)

计量

出版历程

目录

1. 引言

2. 多基线PolInSAR森林高度反演策略

2.1 时间去相干半经验模型

2.2 MCD相干优化算法

2.3 森林高度反演方法

2.4 多基线融合策略

3. 实验与分析

3.1 实验区及数据分析

3.2 实验结果与分析

4. 结束语

期刊介绍

联系我们

通讯作者:
吴迪 wudi82@nuaa.edu.cn

朱岱寅 zhudy@nuaa.edu.cn