作者中心
专家审稿
责编办公
编辑办公
-
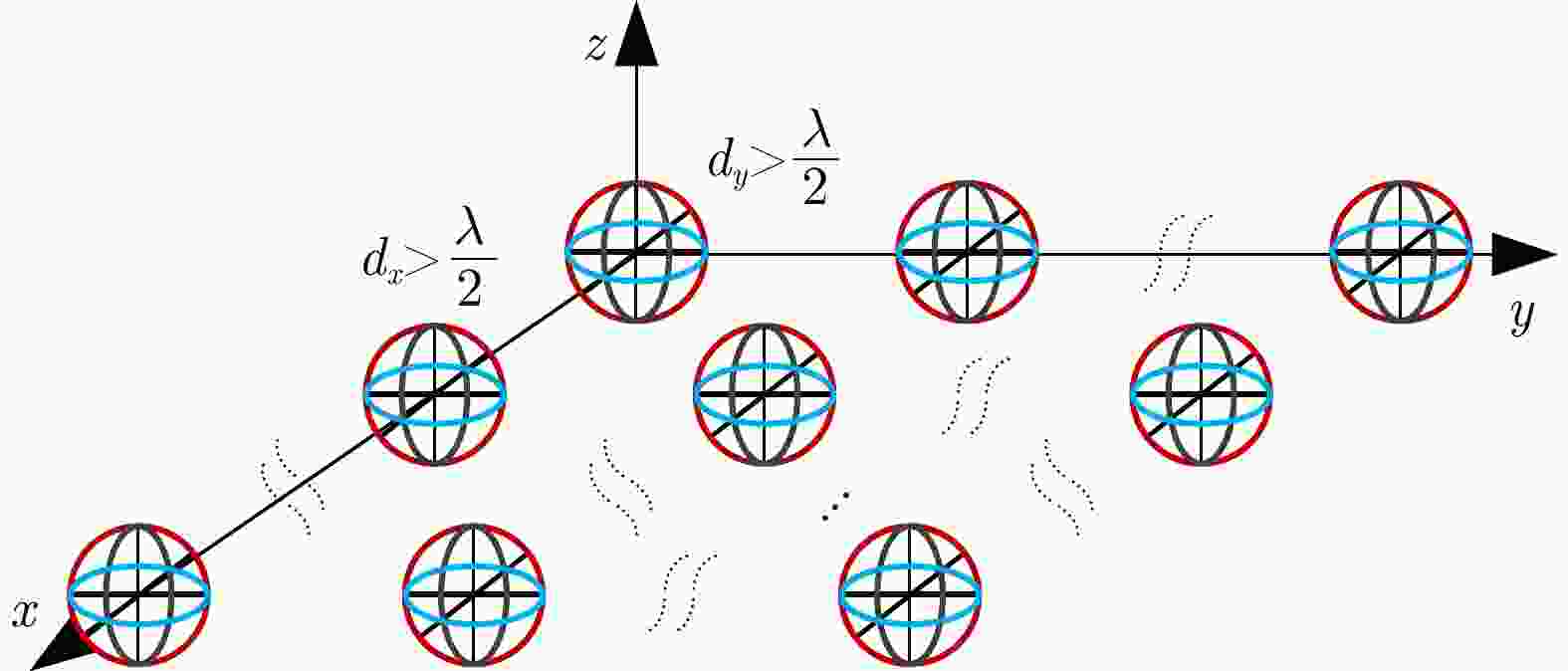
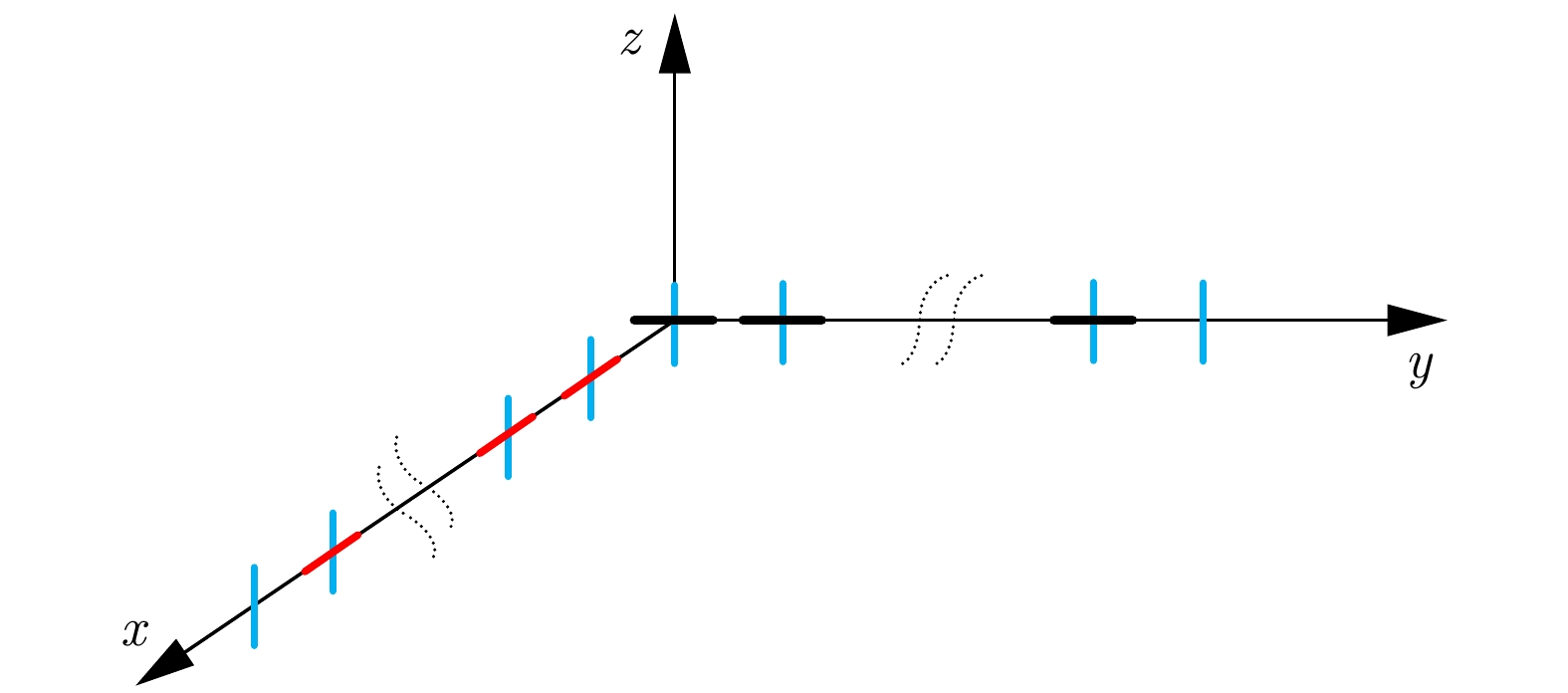
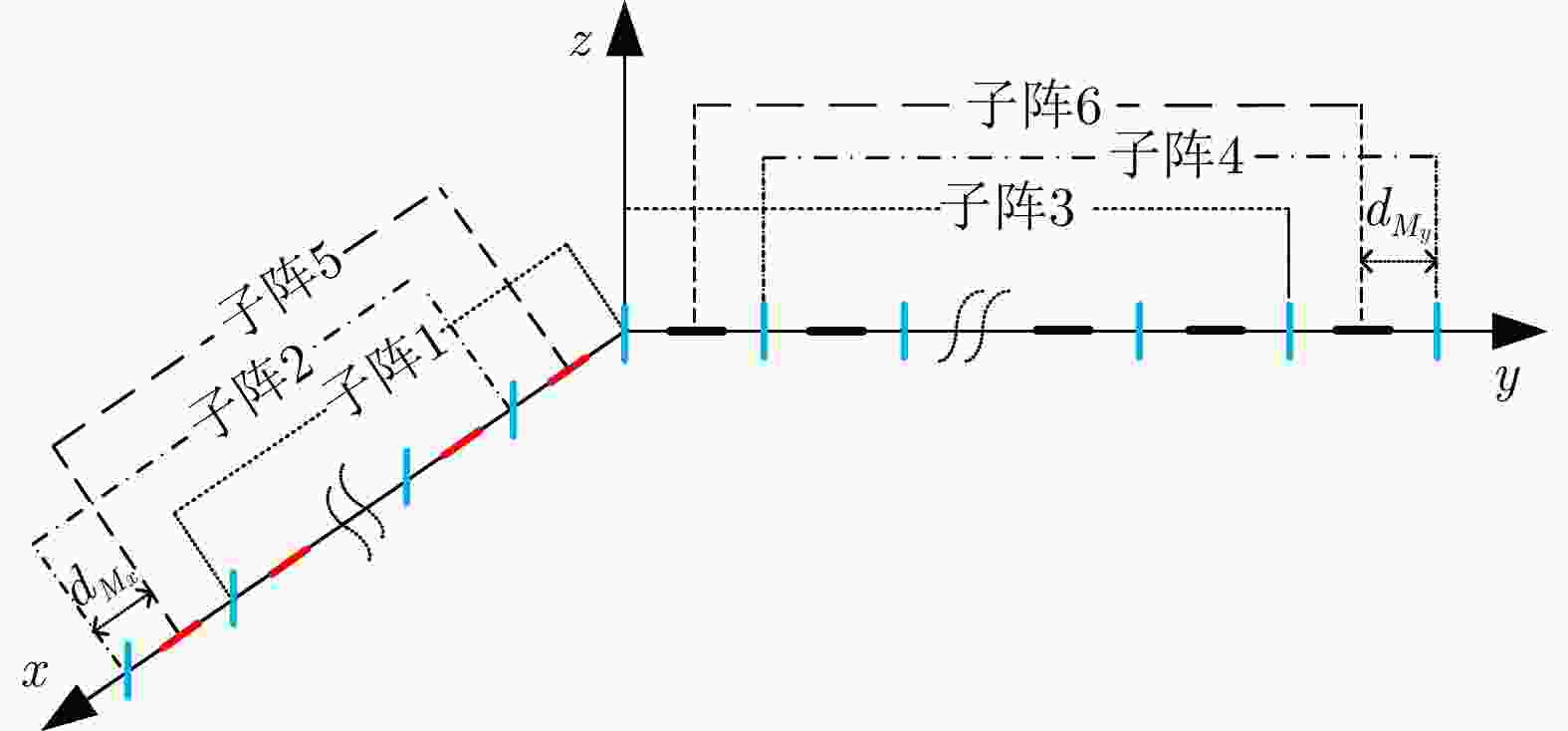
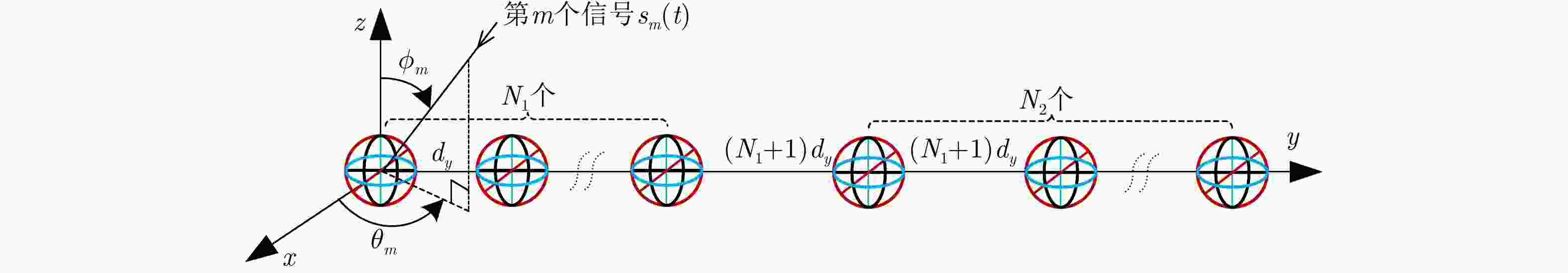摘要: 相较于稀疏标量阵列和均匀多极化阵列，稀疏多极化阵列由于其可感知信号的极化状态、避免极化失配以及增加阵列自由度、减小互耦效应与降低硬件成本等优点，对其进行系统性研究具有重要的应用价值和理论指导意义。稀疏多极化阵列的设计较之于稀疏标量阵列的设计更加多样化，因其不仅与天线阵元位置有关，还与天线阵元极化种类和阵元指向等因素有关。该文首先对近年来该领域内相关研究进行归纳总结，从非均匀稀疏、均匀稀疏、混合均匀与非均匀稀疏3种稀疏方式出发，介绍和探究了主流稀疏多极化阵列结构优化方式，然后从基于深度学习的稀疏多极化阵列优化设计、稀疏多极化多输入多输出(MIMO)雷达、稀疏极化频率分集阵(PFDA)雷达和稀疏PFDA-MIMO雷达、稀疏多极化智能超表面以及稀疏多极化阵列在家居智能通信和工业物联网等复杂室内场景下的应用等方面对未来的发展方向进行了展望。Abstract: As compared with sparse scalar arrays and uniform diversely polarized arrays, sparse diversely polarized arrays show respectively advantages of possessing the ability of sensing the polarization state of source signals, avoiding polarization mismatch and increasing the degrees of freedom, reducing mutual coupling, decreasing hardware cost, etc. Therefore, the comprehensive research on sparse diversely polarized array is of great importance in both theory and realistic applications. The design architecture of sparse diversely polarized arrays, which are related not only to the location of the array elements, but also to the dipole/loop type, orientation, and polarization states of the antenna elements, are more diverse than those of sparse scalar arrays. This paper summarizes the relevant researches in this field in recent years, introduces and explores the mainstream sparse diversely polarized array structure optimization approaches from three aspects: non-uniformly sparse, uniformly sparse and mixed uniformly and non-uniformly sparse. Then the future development of sparse diversely polarized arrays is discussed in terms of deep learning-based optimization approach, sparse Multiple-Input Multiple-Output (MIMO) diversely polarized array, sparse Polarimetric Frequency Diverse Array (PFDA) radar and sparse PFDA-MIMO radar, sparse polarimetric reconfigurable intelligent surface, and the application of sparse diversely polarized array in complex indoor scenes, such as smart communications in house and Industrial Internet of Things.
-
图 1 由6个NA偶极子阵元(轴向平行于y 轴)及10个偶极子ANA阵元(轴向平行于z 轴)组成的稀疏多极化阵列结构示意图
Figure 1. Sparse diversely polarized array composed of 6 NA dipoles (axial directions parallel to the y-axis) and 10 ANA dipoles (axial directions parallel to the z-axis)
图 2 由两层嵌套EMVS阵列组成的稀疏多极化阵列结构示意图
Figure 2. Sparse diversely polarized array composed of two-layer nested EMVS subarrays
图 3 由两层嵌套稀疏拉伸EMVS阵列组成的稀疏多极化阵列结构示意图
Figure 3. Sparse diversely polarized array composed of two-layer nested sparse stretched EMVS subarrays
图 5 由EMVS构成的矩形稀疏多极化阵列结构示意图
Figure 5. Rectangular sparse diversely polarized array constructed by EMVS
图 6 由3种指向的偶极子组成的稀疏L型多极化阵列结构示意图
Figure 6. Sparse L-shaped diversely polarized array composed of dipoles with three directions
图 8 空域分置交叉偶极子稀疏矩形多极化阵列结构示意图
Figure 8. Sparse rectangular diversely polarized array composed of spatially distributed cross-dipoles
图 9 轴向平移多线性稀疏多极化阵列结构示意图
Figure 9. Axial translation multilinear sparse diversely polarized array
图 10 以非均匀稀疏阵为子阵均匀稀疏摆放的多极化阵列结构示意图
Figure 10. Diversely polarized array composed of uniformly distributed non-uniform sparse subarrays
图 11 以均匀稀疏阵为子阵非均匀稀疏摆放的多极化阵列示意图
Figure 11. Diversely polarized array composed of non-uniformly distributed uniform sparse subarrays
图 12 图11所对应的均匀配置多极化阵列示意图
Figure 12. Uniformly distributed diversely polarized array corresponding to Fig. 11
图 13 从传统MIMO雷达到稀疏多极化MIMO雷达的发展脉络
Figure 13. Evolution from traditional MIMO radar to sparse diversely polarized MIMO radar
图 14 稀疏多极化RIS示意图(一种多极化配置方式)
Figure 14. Sparse polarimetric RIS (one diversely polarized configuration)
图 15 稀疏多极化RIS示意图(另一种多极化配置方式)
Figure 15. Sparse polarimetric RIS (another diversely polarized configuration)
表 1 稀疏多极化阵列设计研究的技术背景、理论基础、设计方法种类和设置及约束方式
Table 1. Technical background, theoretical basis, categorization of the configuration design, and setting/constraint approaches for sparse diversely polarized array
技术背景 理论基础 设计种类 设置及约束方式 1 均匀阵列阵元间互耦效应强2 均匀阵列自由度小3 均匀阵列硬件成本高4 标量稀疏阵列无法感知信号极化信息 1 互质阵和嵌套阵等稀疏阵列设计准则2 多极化阵列的矢量叉积性质 1 非均匀稀疏多极化阵列设计2 均匀稀疏多极化阵列设计3 混合均匀与非均匀稀疏多极化阵列设计 1 以互质阵和嵌套阵等稀疏阵为基本稀布约束方式，由不同极化方式的天线联合构建2 等距地稀布不同极化方式的天线3 融合上述两种设置方式分别约束阵列的均匀部分与非均匀部分 
下载: 导出CSV
表 2 标量与多极化MIMO雷达优缺点总结
Table 2. Summary of advantages and disadvantages of scalar and diversely polarized MIMO radars
MIMO雷达类型 主要优势 主要缺点 标量 均匀[54-62] 生成虚拟阵列，增加阵列孔径和自由度[76] 1 阵元间互耦效应降低估计精度；2 系统成本较高 1 极化失配
造成估计
精度损失；2 无法感知
信号极化
信息稀疏[63,69] 最小冗
余[63,64]1 进一步提升阵列孔径和自由度；2 减小阵元间互耦效应，提高角
度估计精度；3 降低系统成本1 阵元位置求解较复杂；2 缺少阵列孔径的一般表达式 嵌套[65,66] 存在间距较密阵元导致的互耦效应 互质[67,68] 差合阵存在孔洞 多极化 均匀[20,70-72] 减小极化失配，
提升角度估计
精度，增强极
化信息处理
能力生成虚拟阵列，增加阵列
孔径和自由度1 阵元间互耦效应降低估计精度；2 系统成本较高 稀疏[73-75] 1 进一步提升阵列孔径和自由度；2 减小阵元间互耦效应，提高角
度估计精度；3 降低系统成本阵列的多极化合理配置仍是一大难点
下载: 导出CSV
表 3 FDA雷达、FDA-MIMO雷达和PFDA-MIMO雷达优点总结
Table 3. Summary of potential advantages of FDA radar, FDA-MIMO radar and PFDA-MIMO radar
下载: 导出CSV
-
[1] HE Jin, WANG Yijing, SHU Ting, et al. Polarization, angle, and delay estimation for tri-polarized systems in multipath environments[J]. IEEE Transactions on Wireless Communications, 2022, 21(8): 5828–5841. doi: 10.1109/TWC.2022.3143834 [2] ZHU Dalin, CHOI J, and HEATH R W. Two-dimensional AoD and AoA acquisition for wideband millimeter-wave systems with dual-polarized MIMO[J]. IEEE Transactions on Wireless Communications, 2017, 16(12): 7890–7905. doi: 10.1109/TWC.2017.2754369 [3] YUE Yaxing, XU Yougen, LIU Zhiwen, et al. Parameter estimation of coexisted circular and strictly noncircular sources using diversely polarized antennas[J]. IEEE Communications Letters, 2018, 22(9): 1822–1825. doi: 10.1109/LCOMM.2018.2849402 [4] WANG Zhanling, YIN Jiapeng, PANG Chen, et al. An adaptive direction-dependent polarization state configuration method for high isolation in polarimetric phased array radar[J]. IEEE Transactions on Antennas and Propagation, 2021, 69(6): 3257–3272. doi: 10.1109/TAP.2020.3037704 [5] FRIEDLANDER B. Polarization sensitivity of antenna arrays[J]. IEEE Transactions on Signal Processing, 2019, 67(1): 234–244. doi: 10.1109/TSP.2018.2880708 [6] SHEN Shanpu, ZHANG Yujie, CHIU C Y, et al. A triple-band high-gain multibeam ambient RF energy harvesting system utilizing hybrid combining[J]. IEEE Transactions on Industrial Electronics, 2020, 67(11): 9215–9226. doi: 10.1109/tie.2019.2952819 [7] 庄钊文, 徐振海, 肖顺平, 等. 极化敏感阵列信号处理[M]. 北京: 国防工业出版社, 2005.ZHUANG Zhaowen, XU Zhenhai, XIAO Shunping, et al. Signal Processing of Polarization Sensitive Array[M]. Beijing: National Defense Industry Press, 2005. [8] ZHAO Kang, LIU Zhiwen, SHI Shuli, et al. Polarimetric clutter nulling space-time adaptive processing[C]. The 2020 4th International Conference on Digital Signal Processing, Chengdu, China, 2020: 331–335. [9] KHAN S and WONG K T. A six-component vector sensor comprising electrically long dipoles and large loops - To simultaneously estimate incident sources’ directions-of-arrival and polarizations[J]. IEEE Transactions on Antennas and Propagation, 2020, 68(8): 6355–6363. doi: 10.1109/TAP.2020.2988980 [10] PAL P and VAIDYANATHAN P P. Nested arrays: A novel approach to array processing with enhanced degrees of freedom[J]. IEEE Transactions on Signal Processing, 2010, 58(8): 4167–4181. doi: 10.1109/TSP.2010.2049264 [11] LIU Jianyan, ZHANG Yanmei, LU Yilong, et al. Augmented nested arrays with enhanced DOF and reduced mutual coupling[J]. IEEE Transactions on Signal Processing, 2017, 65(21): 5549–5563. doi: 10.1109/TSP.2017.2736493 [12] ZHOU Chengwei, GU Yujie, FAN Xing, et al. Direction-of-arrival estimation for coprime array via virtual array interpolation[J]. IEEE Transactions on Signal Processing, 2018, 66(22): 5956–5971. doi: 10.1109/TSP.2018.2872012 [13] ZHENG Wang, ZHANG Xiaofei, WANG Yunfei, et al. Padded coprime arrays for improved DOA estimation: Exploiting hole representation and filling strategies[J]. IEEE Transactions on Signal Processing, 2020, 68: 4597–4611. doi: 10.1109/TSP.2020.3013389 [14] ZHENG Zhi, WANG Wenqin, KONG Yangyang, et al. MISC array: A new sparse array design achieving increased degrees of freedom and reduced mutual coupling effect[J]. IEEE Transactions on Signal Processing, 2019, 67(7): 1728–1741. doi: 10.1109/TSP.2019.2897954 [15] SHEN Qing, LIU Wei, CUI Wei, et al. Simplified and enhanced multiple level nested arrays exploiting high-order difference co-arrays[J]. IEEE Transactions on Signal Processing, 2019, 67(13): 3502–3515. doi: 10.1109/TSP.2019.2914887 [16] FAN Xing, ZHOU Chengwei, GU Yujie, et al. Toeplitz matrix reconstruction of interpolated coprime virtual array for DOA estimation[C]. 2017 IEEE 85th Vehicular Technology Conference (VTC Spring), Sydney, NSW, Australia, 2017: 1–5. [17] ZHOU Chengwei, SHI Zhiguo, GU Yujie, et al. Coarray interpolation-based coprime array DOA estimation via covariance matrix reconstruction[C]. 2018 IEEE International Conference on Acoustics, Speech and Signal Processing, Calgary, AB, Canada, 2018: 3479–3483. [18] YUE Yaxing, ZHANG Zongyu, ZHOU Chengwei, et al. Closed-form two-dimensional DOA and polarization joint estimation using parallel non-collocated sparse COLD arrays[C]. 2022 IEEE 12th Sensor Array and Multichannel Signal Processing Workshop, Trondheim, Norway, 2022: 16–20. [19] TANG Mang, SHU Ting, HE Jin, et al. Direction-finding and polarization estimation with spread orthogonal loop and dipole arrays[J]. Circuits, System, and Signal Processing, 2021, 40(12): 6401–6415. doi: 10.1007/s00034-021-01776-9 [20] YUE Yaxing, XU Yougen, and LIU Zhiwen. Manifold separation and polarimetric element space based parameter estimation for polarimetric monostatic MIMO radar[C]. CIE Radar Conference, Haikou, China, 2021: 573–577. [21] SI Weijian, ZENG Fuhong, QU Zhiyu, et al. Two-dimensional DOA estimation via a novel sparse array consisting of coprime and nested subarrays[J]. IEEE Communications Letters, 2020, 24(6): 1266–1270. doi: 10.1109/LCOMM.2020.2979066 [22] YANG Yunlong, HOU Yuguan, MAO Xingpeng, et al. Stokes parameters and DOA estimation for nested polarization sensitive array in unknown nonuniform noise environment[J]. Signal Processing, 2020, 175: 107630. doi: 10.1016/j.sigpro.2020.107630 [23] HAN Keyong and NEHORAI A. Nested vector-sensor array processing via tensor modeling[J]. IEEE Transactions on Signal Processing, 2014, 62(10): 2542–2553. doi: 10.1109/TSP.2014.2314437 [24] SHI Zhiguo, ZHOU Chengwei, GU Yujie, et al. Source estimation using coprime array: A sparse reconstruction perspective[J]. IEEE Sensors Journal, 2017, 17(3): 755–765. doi: 10.1109/JSEN.2016.2637059 [25] 周成伟, 郑航, 顾宇杰, 等. 互质阵列信号处理研究进展: 波达方向估计与自适应波束成形[J]. 雷达学报, 2019, 8(5): 558–577. doi: 10.12000/JR19068ZHOU Chengwei, ZHENG Hang, GU Yujie, et al. Research progress on coprime array signal processing: Direction-of-Arrival estimation and adaptive beamforming[J]. Journal of Radars, 2019, 8(5): 558–577. doi: 10.12000/JR19068 [26] ZHENG Hang, SHI Zhiguo, ZHOU Chengwei, et al. Coupled coarray tensor CPD for DOA estimation with coprime L-shaped array[J]. IEEE Signal Processing Letters, 2021, 28: 1545–1549. doi: 10.1109/LSP.2021.3099074 [27] SHEN Yifan, ZHOU Chengwei, GU Yujie, et al. Vandermonde decomposition of coprime coarray covariance matrix for DOA estimation[C]. 2017 IEEE 18th International Workshop on Signal Processing Advances in Wireless Communications, Sapporo, Japan, 2017: 1–5. [28] YANG Minglei, DING Jin, CHEN Baixiao, et al. A multiscale sparse array of spatially spread electromagnetic-vector-sensors for direction finding and polarization estimation[J]. IEEE Access, 2018,, 6: 9807–9818. doi: 10.1109/ACCESS.2018.2799905 [29] LECUN Y, BENGIO Y, and HINTON G. Deep learning[J]. Nature, 2015, 521(7553): 436–444. doi: 10.1038/nature14539 [30] ZHOU Xingyue, YAN Yunde, and DUAN Rui. Deep learning based on striation images for underwater and surface target classification[J]. IEEE Signal Processing Letters, 2019, 26(9): 1378–1382. doi: 10.1109/LSP.2019.2919102 [31] ZHOU Xingyue, YAN Yonghong, and YANG Kunde. A multi-feature compression and fusion strategy of vertical self-contained hydrophone array[J]. IEEE Sensors Journal, 2021, 21(21): 24349–24358. doi: 10.1109/JSEN.2021.3112164 [32] 朱圣棋, 余昆, 许京伟, 等. 波形分集阵列新体制雷达研究进展与展望[J]. 雷达学报, 2021, 10(6): 795–810. doi: 10.12000/JR21188ZHU Shengqi, YU Kun, XU Jingwei, et al. Research progress and prospect for the noval waveform diverse array radar[J]. Journal of Radars, 2021, 10(6): 795–810. doi: 10.12000/JR21188 [33] TIAN Jianghao, CAO Xiangyu, GAO Jun, et al. A reconfigurable ultra-wideband polarization converter based on metasurface incorporated with PIN diodes[J]. Journal of Applied Physics, 2019, 125(13): 135105. doi: 10.1063/1.5067383 [34] 于惠存, 曹祥玉, 高军, 等. 一种宽带可重构反射型极化旋转表面[J]. 物理学报, 2018, 67(22): 224101. doi: 10.7498/aps.67.20181041YU Huicun, CAO Xiangyu, GAO Jun, et al. Broadband reconfigurable reflective polarization convertor[J]. Acta Physica Sinica, 2018, 67(22): 224101. doi: 10.7498/aps.67.20181041 [35] YU Huicun, CAO Xiangyu, GAO Jun, et al. Design of a wideband and reconfigurable polarization converter using a manipulable metasurface[J]. Optical Materials Express, 2018, 8(11): 3373–3381. doi: 10.1364/OME.8.003373 [36] MOLERO C, PALOMARES-CABALLERO Á, ALEX-AMOR A, et al. Metamaterial-based reconfigurable intelligent surface: 3D meta-atoms controlled by graphene structures[J]. IEEE Communications Magazine, 2021, 59(6): 42–48. doi: 10.1109/MCOM.001.2001161 [37] WONG K T. Direction finding/polarization estimation-dipole and/or loop triad(s)[J]. IEEE Transactions on Aerospace and Electronic Systems, 2001, 37(2): 679–684. doi: 10.1109/7.937478 [38] WONG K T and YUAN Xin. “Vector cross-product direction-finding” with an electromagnetic vector-sensor of six orthogonally oriented but spatially noncollocating dipoles/loops[J]. IEEE Transactions on Signal Processing, 2011, 59(1): 160–171. doi: 10.1109/TSP.2010.2084085 [39] YUE Yaxing, XU Yougen, ZHUANG Junpeng, et al. Mutual coupling self-calibration for parameter estimation with vector antennas[C]. 2019 IEEE International Conference on Signal, Information and Data Processing, Chongqing, China, 2019: 1–5. [40] YUE Yaxing, XU Yougen, and LIU Zhiwen. Closed-form two-dimensional DOA and polarization estimation of coexisted circular and noncircular signals[C]. CIE Radar Conference, Haikou, China, 2021: 1556–1560. [41] GONG Xiaofeng, JIANG Jiacheng, LI Hui, et al. Spatially spread dipole/loop quint for vector-cross-product-based direction finding and polarisation estimation[J]. IET Signal Processing, 2018, 12(5): 636–642. doi: 10.1049/iet-spr.2017.0232 [42] ZOLTOWSKI M D and WONG K T. ESPRIT-based 2-D direction finding with a sparse uniform array of electromagnetic vector sensors[J]. IEEE Transactions on Signal Processing, 2000, 48(8): 2195–2204. doi: 10.1109/78.852000 [43] 司伟建, 周炯赛, 曲志昱. 稀疏极化敏感阵列的波达方向和极化参数联合估计[J]. 电子与信息学报, 2016, 38(5): 1129–1134. doi: 10.11999/JEIT150840SI Jianwei, ZHOU Jiongsai, and QU Zhiyu. Joint DOA and polarization estimation with sparsely distributed polarization sensitive array[J]. Journal of Electronics &Information Technology, 2016, 38(5): 1129–1134. doi: 10.11999/JEIT150840 [44] 马慧慧, 陶海红. 稀疏拉伸式L型极化敏感阵列的二维波达方向和极化参数联合估计[J]. 电子与信息学报, 2020, 42(4): 902–909. doi: 10.11999/JEIT190208MA Huihui and TAO Haihong. Joint 2D-DOA and polarization parameter estimation with sparsely stretched l-shaped polarization sensitive array[J]. Journal of Electronics &Information Technology, 2020, 42(4): 902–909. doi: 10.11999/JEIT190208 [45] ZHENG Guimei. Two-dimensional DOA estimation for polarization sensitive array consisted of spatially spread crossed-dipole[J]. IEEE Sensors Journal, 2018, 18(12): 5014–5023. doi: 10.1109/JSEN.2018.2820168 [46] JOSHI S and BOYD S. Sensor selection via convex optimization[J]. IEEE Transactions on Signal Processing, 2009, 57(2): 451–462. doi: 10.1109/TSP.2008.2007095 [47] TOHIDI E, COUTINO M, CHEPURI S P, et al. Sparse antenna and pulse placement for colocated MIMO radar[J]. IEEE Transactions on Signal Processing, 2019, 67(3): 579–593. doi: 10.1109/TSP.2018.2881656 [48] WANG Xiangrong, ABOUTANIOS E, and AMIN M G. Adaptive array thinning for enhanced DOA estimation[J]. IEEE Signal Processing Letters, 2015, 22(7): 799–803. doi: 10.1109/LSP.2014.2370632 [49] ELBIR A M and MISHRA K V. Joint antenna selection and hybrid beamformer design using unquantized and quantized deep learning networks[J]. IEEE Transactions on Wireless Communications, 2020, 19(3): 1677–1688. doi: 10.1109/TWC.2019.2956146 [50] ELBIR A M and MISHRA K V. Deep learning design for joint antenna selection and hybrid beamforming in massive MIMO[C]. 2019 IEEE International Symposium on Antennas and Propagation and USNC-URSI Radio Science Meeting, Atlanta, USA, 2019: 1585–1586. [51] ZHANG Shunbo, ZHANG Shun, GAO Feifei, et al. Deep learning optimized sparse antenna activation for reconfigurable intelligent surface assisted communication[J]. IEEE Transactions on Communications, 2021, 69(10): 6691–6705. doi: 10.1109/TCOMM.2021.3097726 [52] WANDALE S and ICHIGE K. Design of sparse arrays via deep learning for enhanced DOA estimation[J]. EURASIP Journal on Advances in Signal Processing, 2021, 2021(1): 17. doi: 10.1186/S13634-021-00727-5 [53] BLISS D W and FORSYTHE K W. Multiple-input multiple-output (MIMO) radar and imaging: Degrees of freedom and resolution[C]. The Thrity-Seventh Asilomar Conference on Signals, Systems & Computers, Pacific Grove, USA, 2003: 54–59. [54] JIN Ming, LIAO Guisheng, and LI Jun. Joint DOD and DOA estimation for bistatic MIMO radar[J]. Signal Processing, 2009, 89(2): 244–251. doi: 10.1016/j.sigpro.2008.08.003 [55] ZHANG Xiaofei, XU Lingyun, XU Lei, et al. Direction of departure (DOD) and direction of arrival (DOA) estimation in MIMO radar with reduced-dimension MUSIC[J]. IEEE Communications Letters, 2010, 14(12): 1161–1163. doi: 10.1109/LCOMM.2010.102610.101581 [56] YUE Yaxing, XU Yougen, and LIU Zhiwen. Two-dimensional direction-of-arrival estimation in monostatic MIMO radar[C]. 2021 4th International Conference on Information Communication and Signal Processing, Shanghai, China, 2021: 60–64. [57] HASSANIEN A and VOROBYOV S A. Transmit energy focusing for DOA estimation in MIMO radar with colocated antennas[J]. IEEE Transactions on Signal Processing, 2011, 59(6): 2669–2682. doi: 10.1109/TSP.2011.2125960 [58] BENCHEIKH M L, WANG Yide, and HE Hongyang. Polynomial root finding technique for joint DOA DOD estimation in bistatic MIMO radar[J]. Signal Processing, 2010, 90(9): 2723–2730. doi: 10.1016/j.sigpro.2010.03.023 [59] LI Jianfeng, HE Yi, HE Lang, et al. DOD and DOA estimation for MIMO radar based on combined MUSIC and sparse Bayesian learning[C]. 2019 International Applied Computational Electromagnetics Society Symposium-China (ACES), Nanjing, China, 2019: 1–2. [60] BAIDOO E, HU Jurong, ZENG Bao, et al. Joint DOD and DOA estimation using tensor reconstruction based sparse representation approach for bistatic MIMO radar with unknown noise effect[J]. Signal Processing, 2021, 182: 107912. doi: 10.1016/j.sigpro.2020.107912 [61] LIU Yang, CHAI Jin, ZHANG Yinghui, et al. Low-complexity neural network based DOA estimation for wideband signals in massive MIMO systems[J]. AEU-International Journal of Electronics and Communications, 2021, 138: 153853. doi: 10.1016/J.AEUE.2021.153853 [62] MOLAEI A M, DEL HOUGNE P, FUSCO V, et al. Efficient joint estimation of DOA, range and reflectivity in near-field by using mixed-order statistics and a symmetric MIMO array[J]. IEEE Transactions on Vehicular Technology, 2022, 71(3): 2824–2842. doi: 10.1109/TVT.2021.3138251 [63] CHEN Chunyang and VAIDYANATHAN P P. Minimum redundancy MIMO radars[C]. 2008 IEEE International Symposium on Circuits and Systems, Seattle, USA, 2008: 45–48. [64] HUANG Yan, LIAO Guisheng, LI Jun, et al. Sum and difference coarray based MIMO radar array optimization with its application for DOA estimation[J]. Multidimensional Systems and Signal Processing, 2017, 28(4): 1183–1202. doi: 10.1007/s11045-016-0387-2 [65] ZHANG Yule, HU Guoping, ZHOU Hao, et al. DOA estimation of a novel generalized nested MIMO radar with high degrees of freedom and hole-free difference coarray[J]. Mathematical Problems in Engineering, 2021, 2021: 6622154. doi: 10.1155/2021/6622154 [66] YANG Minglei, SUN Lei, YUAN Xin, et al. A new nested MIMO array with increased degrees of freedom and hole-free difference coarray[J]. IEEE Signal Processing Letters, 2018, 25(1): 40–44. doi: 10.1109/lsp.2017.2766294 [67] LIU Donglei, ZHAO Yongbo, and DONG Shuxian. A novel co-prime MIMO radar model for DOA estimation[J]. Signal Processing, 2022, 199: 108606. doi: 10.1016/j.sigpro.2022.108606 [68] ZHANG Fei, JI Chuantang, ZHANG Zijing, et al. Non-circular signal DOA estimation based on coprime array MIMO radar[J]. EURASIP Journal on Advances in Signal Processing, 2021(1): 99. doi: 10.1186/S13634-021-00806-7 [69] SHI Junpeng, HU Guoping, ZHANG Xiaofei, et al. Sparsity-based DOA estimation of coherent and uncorrelated targets with flexible MIMO radar[J]. IEEE Transactions on Vehicular Technology, 2019, 68(6): 5835–5848. doi: 10.1109/TVT.2019.2913437 [70] WEN Fangqing, SHI Junpeng, and ZHANG Zijing. Joint 2D-DOD, 2D-DOA, and polarization angles estimation for bistatic EMVS-MIMO radar via PARAFAC analysis[J]. IEEE Transactions on Vehicular Technology, 2020, 69(2): 1626–1638. doi: 10.1109/TVT.2019.2957511 [71] DING Xueke, HU Ying, LIU Changming, et al. Coherent targets parameter estimation for EVS-MIMO radar[J]. Remote Sensing, 2022, 14(17): 4331. doi: 10.3390/rs14174331 [72] PONNUSAMY P, SUBRAMANIAM K, and CHINTAGUNTA S. Computationally efficient method for joint DOD and DOA estimation of coherent targets in MIMO radar[J]. Signal Processing, 2019, 165: 262–267. doi: 10.1016/j.sigpro.2019.07.015 [73] WANG Xianpeng, HUANG Mengxing, and WAN Liangtian. Joint 2D-DOD and 2D-DOA estimation for coprime EMVS–MIMO radar[J]. Circuits, Systems, and Signal Processing, 2021, 40(6): 2950–2966. doi: 10.1007/s00034-020-01605-5 [74] YANG Yongqiang, RUAN Ningjun, HUANG Guanjun, et al. A propagator method for bistatic coprime EMVS-MIMO radar[J]. Mathematical Problems in Engineering, 2021, 2021: 9954573. doi: 10.1155/2021/9954573 [75] 谢前朋, 潘小义, 陈吉源, 等. 基于新型阵列的双基地电磁矢量传感器MIMO雷达高分辨角度参数估计[J]. 电子与信息学报, 2021, 43(2): 270–276. doi: 10.11999/JEIT200130XIE Qianpeng, PAN Xiaoyi, CHEN Jiyuan, et al. High resolution angle parameter estimation for bistatic EMVS-MIMO radar based on a new designed array[J]. Journal of Electronics &Information Technology, 2021, 43(2): 270–276. doi: 10.11999/JEIT200130 [76] 赵永波, 刘宏伟. MIMO雷达技术综述[J]. 数据采集与处理, 2018, 33(3): 389–399. doi: 10.16337/j.1004-9037.2018.03.001ZHAO Yongbo and LIU Hongwei. Overview on MIMO radar[J]. Journal of Data Acquisition and Processing, 2018, 33(3): 389–399. doi: 10.16337/j.1004-9037.2018.03.001 [77] ANTONIK P, WICKS M C, GRIFFITHS H D, et al. Range-dependent beamforming using element level waveform diversity[C]. 2006 International Waveform Diversity & Design Conference, Lihue, USA, 2006: 1–6. [78] ANTONIK P, WICKS M C, GRIFFITHS H D, et al. Frequency diverse array radars[C]. 2006 IEEE Conference on Radar, Verona, USA, 2006: 3. [79] WICKS M C and ANTONIK P. Frequency diverse array with independent modulation of frequency, amplitude, and phase[P]. US 7319427, 2008. [80] WICKS M C and ANTONIK P. Method and apparatus for a frequency diverse array[P]. US 7511665, 2009. [81] ANTONIK P. An investigation of a frequency diverse array[D]. [Ph. D. dissertation], University College London, 2009. [82] BASIT A, WANG Wenqin, NUSENU S Y, et al. FDA based QSM for mmwave wireless communications: Frequency diverse transmitter and reduced complexity receiver[J]. IEEE Transactions on Wireless Communications, 2021, 20(7): 4571–4584. doi: 10.1109/TWC.2021.3060512 [83] NUSENU S Y, SHAO Huaizong, PAN Ye, et al. Directional modulation with precise legitimate location using time-modulation retrodirective frequency diversity array for secure IoT communications[J]. IEEE Systems Journal, 2021, 15(1): 1109–1119. doi: 10.1109/JSYST.2020.3010787 [84] SAMMARTINO P F, BAKER C J, and GRIFFITHS H D. Frequency diverse MIMO techniques for radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(1): 201–222. doi: 10.1109/TAES.2013.6404099 [85] 许京伟, 朱圣棋, 廖桂生, 等. 频率分集阵雷达技术探讨[J]. 雷达学报, 2018, 7(2): 167–182. doi: 10.12000/JR18023XU Jingwei, ZHU Shengqi, LIAO Guisheng, et al. An overview of frequency diverse array radar technology[J]. Journal of Radars, 2018, 7(2): 167–182. doi: 10.12000/JR18023 [86] SUN Yan, ZHENG Zhi, WANG Wenqin, et al. DOA estimation and tracking for FDA-MIMO radar signal[J]. Digital Signal Processing, 2020, 106: 102858. doi: 10.1016/j.dsp.2020.102858 [87] XU Tengxian, WANG Xianpeng, HUANG Mengxing, et al. Tensor-based reduced-dimension music method for parameter estimation in monostatic FDA-MIMO radar[J]. Remote Sensing, 2021, 13(18): 3772. doi: 10.3390/rs13183772 [88] CUI Can, XU Jian, GUI Ronghua, et al. Search-free DOD, DOA and range estimation for bistatic FDA-MIMO radar[J]. IEEE Access, 2018, 6: 15431–15445. doi: 10.1109/ACCESS.2018.2816780 [89] LIU Yibin, WANG Chunyang, ZHENG Guimei, et al. Joint range and angle estimation of low-elevation target with bistatic meter-wave FDA-MIMO radar[J]. Digital Signal Processing, 2022, 127: 103556. doi: 10.1016/j.dsp.2022.103556 [90] 王文钦, 陈慧, 郑植, 等. 频控阵雷达技术及其应用研究进展[J]. 雷达学报, 2018, 7(2): 153–166. doi: 10.12000/JR18029WANG Wenqin, CHEN Hui, ZHENG Zhi, et al. Advances on frequency diverse array radar and its applications[J]. Journal of Radars, 2018, 7(2): 153–166. doi: 10.12000/JR18029 [91] 陈阳, 田波, 王春阳, 等. FDA-MIMO抗干扰技术进展及前景展望[J]. 电光与控制, 2022, 29(8): 65–72. doi: 10.3969/j.issn.1671-637X.2022.08.012CHEN Yang, TIAN Bo, WANG Chunyang, et al. Progress and prospect of FDA-MIMO anti-jamming technology[J]. Electronics Optics Control, 2022, 29(8): 65–72. doi: 10.3969/j.issn.1671-637X.2022.08.012 [92] LAN Lan, XU Jingwei, LIAO Guisheng, et al. Suppression of mainbeam deceptive jammer with FDA-MIMO radar[J]. IEEE Transactions on Vehicular Technology, 2020, 69(10): 11584–11598. doi: 10.1109/TVT.2020.3014689 [93] 兰岚, 廖桂生, 许京伟, 等. FDA-MIMO雷达主瓣距离欺骗式干扰抑制方法[J]. 系统工程与电子技术, 2018, 40(5): 997–1003. doi: 10.3969/j.issn.1001-506X.2018.05.06LAN Lan, LIAO Guisheng, XU Jingwei, et al. Main-beam range deceptive jamming suppression approach with FDA-MIMO radar[J]. Systems Engineering and Electronics, 2018, 40(5): 997–1003. doi: 10.3969/j.issn.1001-506X.2018.05.06 [94] 高霞, 全英汇, 李亚超, 等. 基于BSS的FDA-MIMO雷达主瓣欺骗式干扰抑制方法[J]. 系统工程与电子技术, 2020, 42(9): 1927–1934. doi: 10.3969/j.issn.1001-506X.2020.09.07GAO Xia, QUAN Yinghui, LI Yachao, et al. Main-lobe deceptive jamming suppression with FDA-MIMO radar based on BSS[J]. Systems Engineering and Electronics, 2020, 42(9): 1927–1934. doi: 10.3969/j.issn.1001-506X.2020.09.07 [95] 许京伟, 廖桂生, 张玉洪, 等. 波形分集阵雷达抗欺骗式干扰技术[J]. 电子学报, 2019, 47(3): 545–551. doi: 10.3969/j.issn.0372-2112.2019.03.005XU Jingwei, LIAO Guisheng, ZHANG Yuhong, et al. On anti-jamming technique with waveform diverse array radar[J]. Acta Electronica Sinica, 2019, 47(3): 545–551. doi: 10.3969/j.issn.0372-2112.2019.03.005 [96] 陈浩, 李荣锋, 戴凌燕, 等. 基于 FVE 法的 FDA-MIMO 雷达主瓣密集假目标干扰抑制[J]. 空军预警学院学报, 2018, 32(6): 397–401. doi: 10.3969/j.issn.2095-5839.2018.06.002CHEN Hao, LI Rongfeng, DAI Lingyan, et al. FDA-MIMO radar mainlobe dense false target jamming suppression based on feature vector eliminating[J]. Journal of Air Force Early Warning Academy, 2018, 32(6): 397–401. doi: 10.3969/j.issn.2095-5839.2018.06.002 [97] QIN Si, ZHANG Y D, AMIN M G, et al. Frequency diverse coprime arrays with coprime frequency offsets for multitarget localization[J]. IEEE Journal of Selected Topics in Signal Processing, 2017, 11(2): 321–335. doi: 10.1109/JSTSP.2016.2627184 [98] CAO Ruisong, LIU Shengheng, MAO Zihuan, et al. Doubly-Toeplitz-based interpolation for joint DOA-range estimation using coprime FDA[C]. 2021 IEEE Radar Conference, Atlanta, USA, 2021: 1–6. [99] SEDIGHI S, SHANKAR B, MISHRA K V, et al. Optimum design for sparse FDA-MIMO automotive radar[C]. 2019 53rd Asilomar Conference on Signals, Systems, and Computers, Pacific Grove, USA, 2019: 913–918. [100] LI Binbin, BAI Weixiong, and ZHENG Guimei. Successive ESPRIT algorithm for joint DOA-range-polarization estimation with polarization sensitive FDA-MIMO radar[J]. IEEE Access, 2018, 6: 36376–36382. doi: 10.1109/ACCESS.2018.2844948 [101] LI Binbin, CHEN Hui, ZHENG Guimei, et al. Joint DOA-range-polarization estimation with polarization sensitive FDA-MIMO radar[C]. International Conference on Frontiers of Electronics, Information and Computation Technologies, Changsha, China, 2021: 22. [102] YU Nanfang, GENEVET P, KATS M A, et al. Light propagation with phase discontinuities: Generalized laws of reflection and refraction[J]. Science, 2011, 334(6054): 333–337. doi: 10.1126/science.1210713 [103] 杨欢欢, 曹祥玉, 高军, 等. 可重构电磁超表面及其应用研究进展[J]. 雷达学报, 2021, 10(2): 206–219. doi: 10.12000/JR20137YANG Huanhuan, CAO Xiangyu, GAO Jun, et al. Recent advances in reconfigurable metasurfaces and their applications[J]. Journal of Radars, 2021, 10(2): 206–219. doi: 10.12000/JR20137 [104] YANG Wanchen, CHEN Si, CHE Wenquan, et al. Compact high-gain metasurface antenna arrays based on higher-mode SIW cavities[J]. IEEE Transactions on Antennas and Propagation, 2018, 66(9): 4918–4923. doi: 10.1109/TAP.2018.2851659 [105] NIE Niansheng, YANG Xuesong, CHEN Zhining, et al. A low-profile wideband hybrid metasurface antenna array for 5G and WiFi systems[J]. IEEE Transactions on Antennas and Propagation, 2020, 68(2): 665–671. doi: 10.1109/TAP.2019.2940367 [106] YANG Wanchen, MENG Qian, CHE Wenquan, et al. Low-profile wideband dual-circularly polarized metasurface antenna array with large beamwidth[J]. IEEE Antennas and Wireless Propagation Letters, 2018, 17(9): 1613–1616. doi: 10.1109/LAWP.2018.2857625 [107] CUI Tiejun, QI Meiqing, WAN Xiang, et al. Coding metamaterials, digital metamaterials and programmable metamaterials[J]. Light:Science & Applications, 2014, 3(10): e218. doi: 10.1038/lsa.2014.99 [108] YANG Huanhuan, YANG Fan, CAO Xiangyu, et al. A 1600-element dual-frequency electronically reconfigurable reflectarray at X/Ku-band[J]. IEEE transactions on antennas and propagation, 2017, 65(6): 3024–3032. doi: 10.1109/TAP.2017.2694703 [109] BASAR E, DI RENZO M, DE ROSNY J, et al. Wireless communications through reconfigurable intelligent surfaces[J]. IEEE Access, 2019, 7: 116753–116773. doi: 10.1109/ACCESS.2019.2935192 [110] GUAN Xinrong, WU Qingqing, and ZHANG Rui. Intelligent reflecting surface assisted secrecy communication: Is artificial noise helpful or not?[J]. IEEE Wireless Communications Letters, 2020, 9(6): 778–782. doi: 10.1109/LWC.2020.2969629 [111] DI Boya, ZHANG Hongliang, SONG Lingyang, et al. Hybrid beamforming for reconfigurable intelligent surface based multi-user communications: Achievable rates with limited discrete phase shifts[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(8): 1809–1822. doi: 10.1109/JSAC.2020.3000813 [112] LI Wenting, GAO S, CAI Yuanming, et al. Polarization-reconfigurable circularly polarized planar antenna using switchable polarizer[J]. IEEE Transactions on Antennas and Propagation, 2017, 65(9): 4470–4477. doi: 10.1109/TAP.2017.2730240 [113] MA Xiaoliang, PAN Wenbo, HUANG Cheng, et al. An active metamaterial for polarization manipulating[J]. Advanced Optical Materials, 2014, 2(10): 945–949. doi: 10.1002/adom.201400212 [114] CUI Jianhua, HUANG Cheng, PAN Wenbo, et al. Dynamical manipulation of electromagnetic polarization using anisotropic meta-mirror[J]. Scientific Reports, 2016, 6(1): 30771. doi: 10.1038/srep30771 [115] TAO Zui, WAN Xiang, PAN Baicao, et al. Reconfigurable conversions of reflection, transmission, and polarization states using active metasurface[J]. Applied Physics Letters, 2017, 110(12): 121901. doi: 10.1063/1.4979033 [116] ZHANG Meng, ZHANG W, LIU A Q, et al. Tunable polarization conversion and rotation based on a reconfigurable metasurface[J]. Scientific Reports, 2017, 7(1): 12068. doi: 10.1038/s41598-017-11953-z [117] ELMOSSALLAMY M A, ZHANG Hongliang, SONG Lingyang, et al. Reconfigurable intelligent surfaces for wireless communications: Principles, challenges, and opportunities[J]. IEEE Transactions on Cognitive Communications and Networking, 2020, 6(3): 990–1002. doi: 10.1109/TCCN.2020.2992604 [118] Alexandropoulos G C, Shlezinger N, and Del Hougne P. Reconfigurable intelligent surfaces for rich scattering wireless communications: Recent experiments, challenges, and opportunities[J]. IEEE Communications Magazine, 2021, 59(6): 28–34. doi: 10.1109/MCOM.001.2001117 [119] HUANG Chongwen, ZAPPONE A, ALEXANDROPOULOS G C, et al. Reconfigurable intelligent surfaces for energy efficiency in wireless communication[J]. IEEE Transactions on Wireless Communications, 2019, 18(8): 4157–4170. doi: 10.1109/TWC.2019.2922609 [120] BJÖRNSON E, ÖZDOGAN Ö, and LARSSON E G. Reconfigurable intelligent surfaces: Three myths and two critical questions[J]. IEEE Communications Magazine, 2020, 58(12): 90–96. doi: 10.1109/MCOM.001.2000407 [121] DI RENZO M, ZAPPONE A, DEBBAH M, et al. Smart radio environments empowered by reconfigurable intelligent surfaces: How it works, state of research, and the road ahead[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(11): 2450–2525. doi: 10.1109/JSAC.2020.3007211 [122] HU Xiaoling, ZHONG Caijun, ZHANG Yu, et al. Location information aided multiple intelligent reflecting surface systems[J]. IEEE Transactions on Communications, 2020, 68(12): 7948–7962. doi: 10.1109/TCOMM.2020.3020577 [123] LIN Shaoe, ZHENG Beixiong, ALEXANDROPOULOS G C, et al. Adaptive transmission for reconfigurable intelligent surface-assisted OFDM wireless communications[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(11): 2653–2665. doi: 10.1109/JSAC.2020.3007038 [124] ZENG Ming, LI Xingwang, LI Gen, et al. Sum rate maximization for IRS-assisted uplink NOMA[J]. IEEE Communications Letters, 2021, 25(1): 234–238. doi: 10.1109/LCOMM.2020.3025978 [125] ZUO Jiakuo, LIU Yuanwei, BASAR E, et al. Intelligent reflecting surface enhanced millimeter-wave NOMA systems[J]. IEEE Communications Letters, 2020, 24(11): 2632–2636. doi: 10.1109/LCOMM.2020.3009158 [126] WU Qingqing and ZHANG Rui. Intelligent reflecting surface enhanced wireless network via joint active and passive beamforming[J]. IEEE Transactions on Wireless Communications, 2019, 18(11): 5394–5409. doi: 10.1109/TWC.2019.2936025 [127] TANG Wankai, DAI Junyan, CHEN Mingzheng, et al. MIMO transmission through reconfigurable intelligent surface: System design, analysis, and implementation[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(11): 2683–2699. doi: 10.1109/JSAC.2020.3007055 [128] WU Qingqing and ZHANG Rui. Towards smart and reconfigurable environment: Intelligent reflecting surface aided wireless network[J]. IEEE Communications Magazine, 2020, 58(1): 106–112. doi: 10.1109/MCOM.001.1900107 [129] CHEN Peng, CHEN Zhimin, ZHENG Beixiong, et al. Efficient DOA estimation method for reconfigurable intelligent surfaces aided UAV swarm[J]. IEEE Transactions on Signal Processing, 2022, 70: 743–755. doi: 10.1109/TSP.2022.3146791 [130] ZHANG Jiliang, GLAZUNOV A A, YANG Wenfei, et al. Fundamental wireless performance of a building[J]. IEEE Wireless Communications, 2022, 29(1): 186–193. doi: 10.1109/MWC.121.2100244 [131] ZHANG Jiliang, GLAZUNOV A A, and ZHANG Jie. Wireless performance evaluation of building layouts: Closed-form computation of figures of merit[J]. IEEE Transactions on Communications, 2021, 69(7): 4890–4906. doi: 10.1109/TCOMM.2021.3074546 [132] ANDREWS J G, ZHANG Xinchen, DURGIN G D, et al. Are we approaching the fundamental limits of wireless network densification?[J]. IEEE Communications Magazine, 2016, 54(10): 184–190. doi: 10.1109/MCOM.2016.7588290 [133] CALABUIG D, BARMPOUNAKIS S, GIMENEZ S, et al. Resource and mobility management in the network layer of 5G cellular ultra-dense networks[J]. IEEE Communications Magazine, 2017, 55(6): 162–169. doi: 10.1109/MCOM.2017.1600293 [134] BUSARI S A, HUQ K M S, MUMTAZ S, et al. Millimeter-wave massive MIMO communication for future wireless systems: A survey[J]. IEEE Communications Surveys & Tutorials, 2018, 20(2): 836–869. doi: 10.1109/COMST.2017.2787460 [135] ZENG Shuhao, ZHANG Hongliang, DI Boya, et al. Reconfigurable intelligent surface (RIS) assisted wireless coverage extension: RIS orientation and location optimization[J]. IEEE Communications Letters, 2021, 25(1): 269–273. doi: 10.1109/LCOMM.2020.3025345 [136] PENA D, FEICK R, HRISTOV H D, et al. Measurement and modeling of propagation losses in brick and concrete walls for the 900-MHz band[J]. IEEE Transactions on Antennas and Propagation, 2003, 51(1): 31–39. doi: 10.1109/TAP.2003.808539 [137] ZHANG Jiliang, LIAO Xi, GLAZUNOV A A, et al. Two-ray reflection resolution algorithm for planar material electromagnetic property measurement at the millimeter-wave bands[J]. Radio Science, 2020, 55(3): e2019RS006944. doi: 10.1029/2019RS006944 [138] ZHOU Yang, SHAO Yu, ZHANG Jiliang, et al. Wireless performance evaluation of building materials integrated with antenna arrays[J]. IEEE Communications Letters, 2022, 26(4): 942–946. doi: 10.1109/LCOMM.2022.3141390 -
图(15) / 表(3)
计量
- 文章访问数: 1794
- HTML全文浏览量: 686
- PDF下载量: 336
- 被引次数: 0
