作者中心
专家审稿
责编办公
编辑办公
Terrain Classification of Polarimetric SAR Images Using Semi-supervised Spatial-channel Selective Kernel Network
-
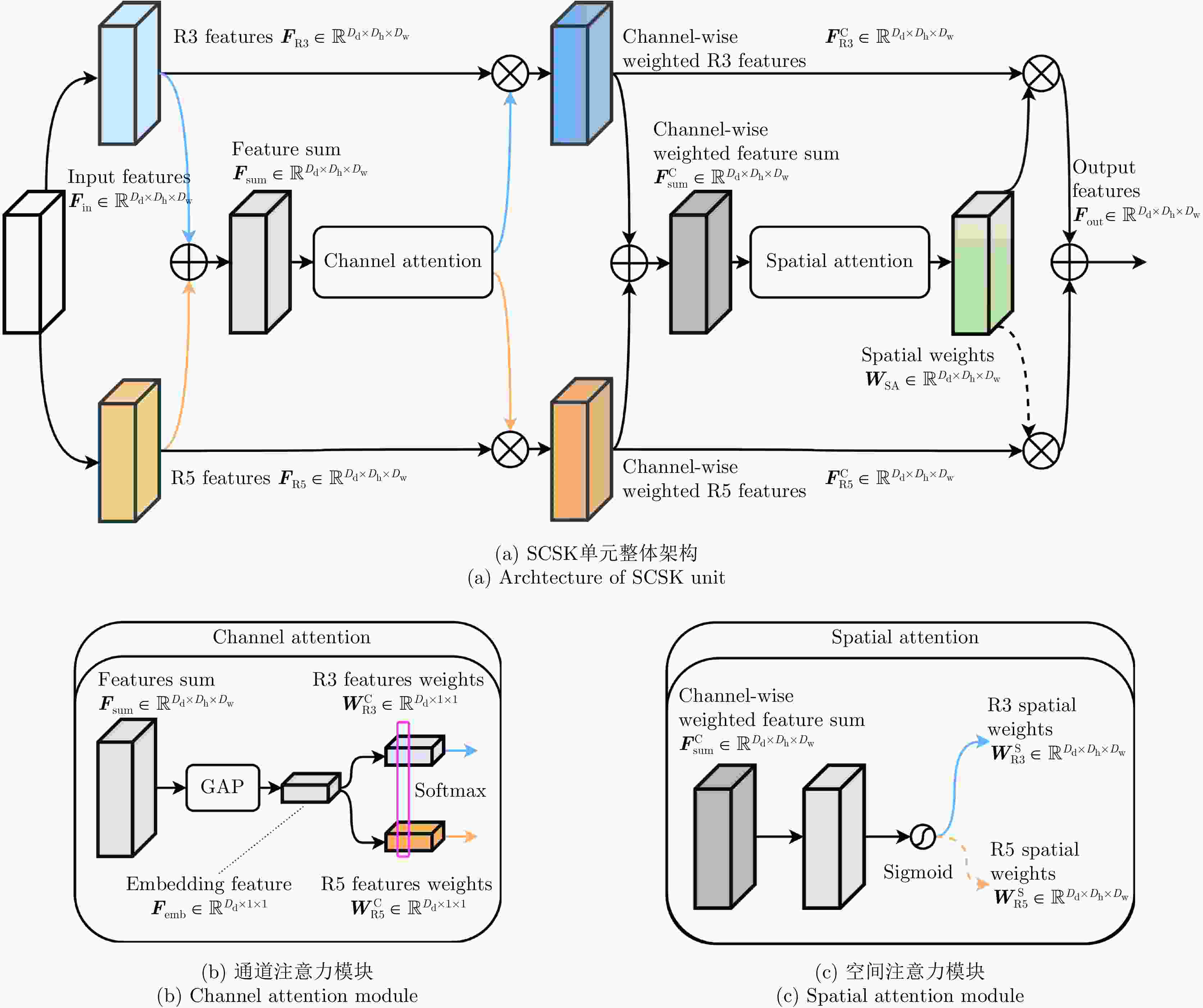
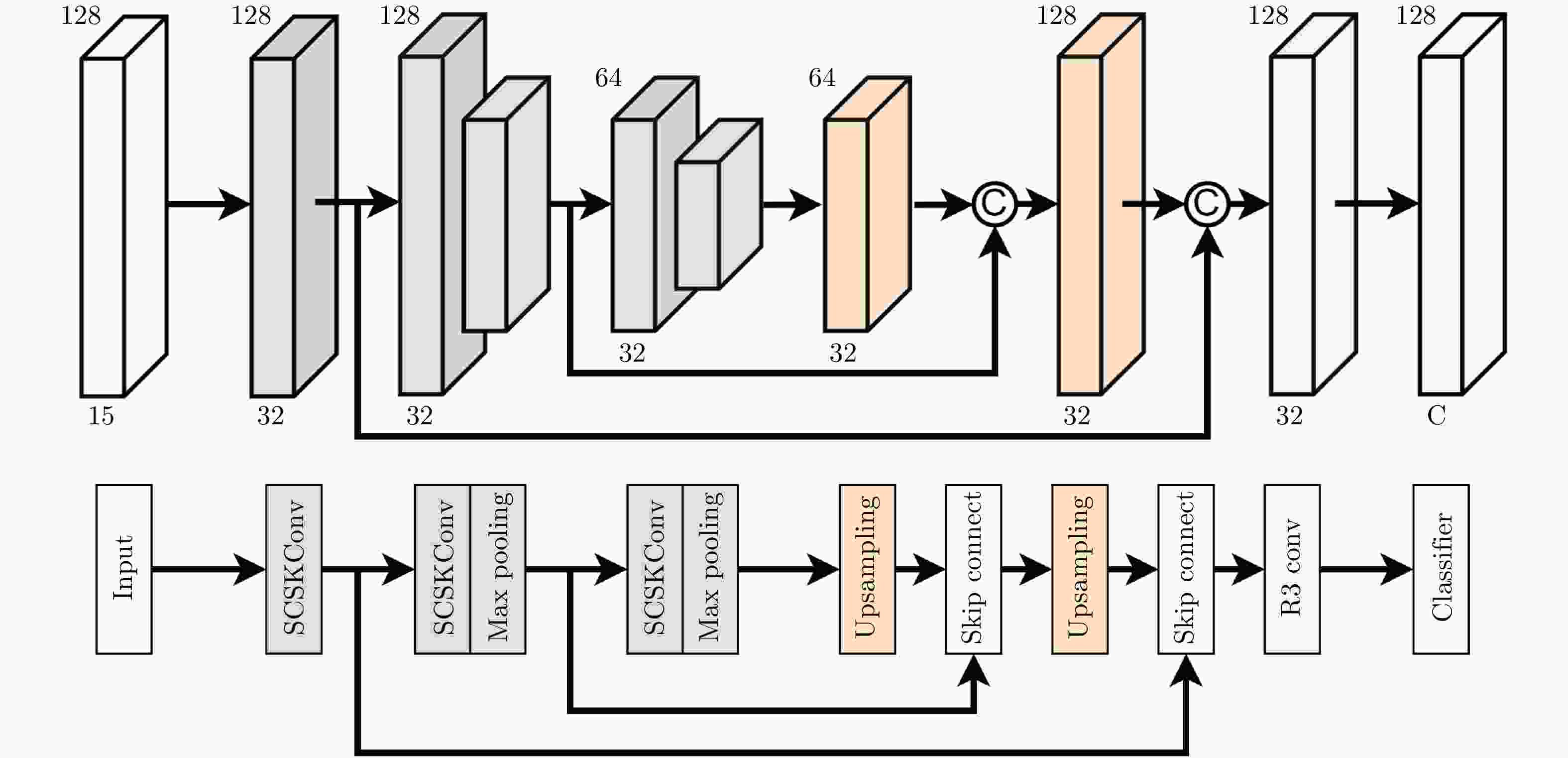
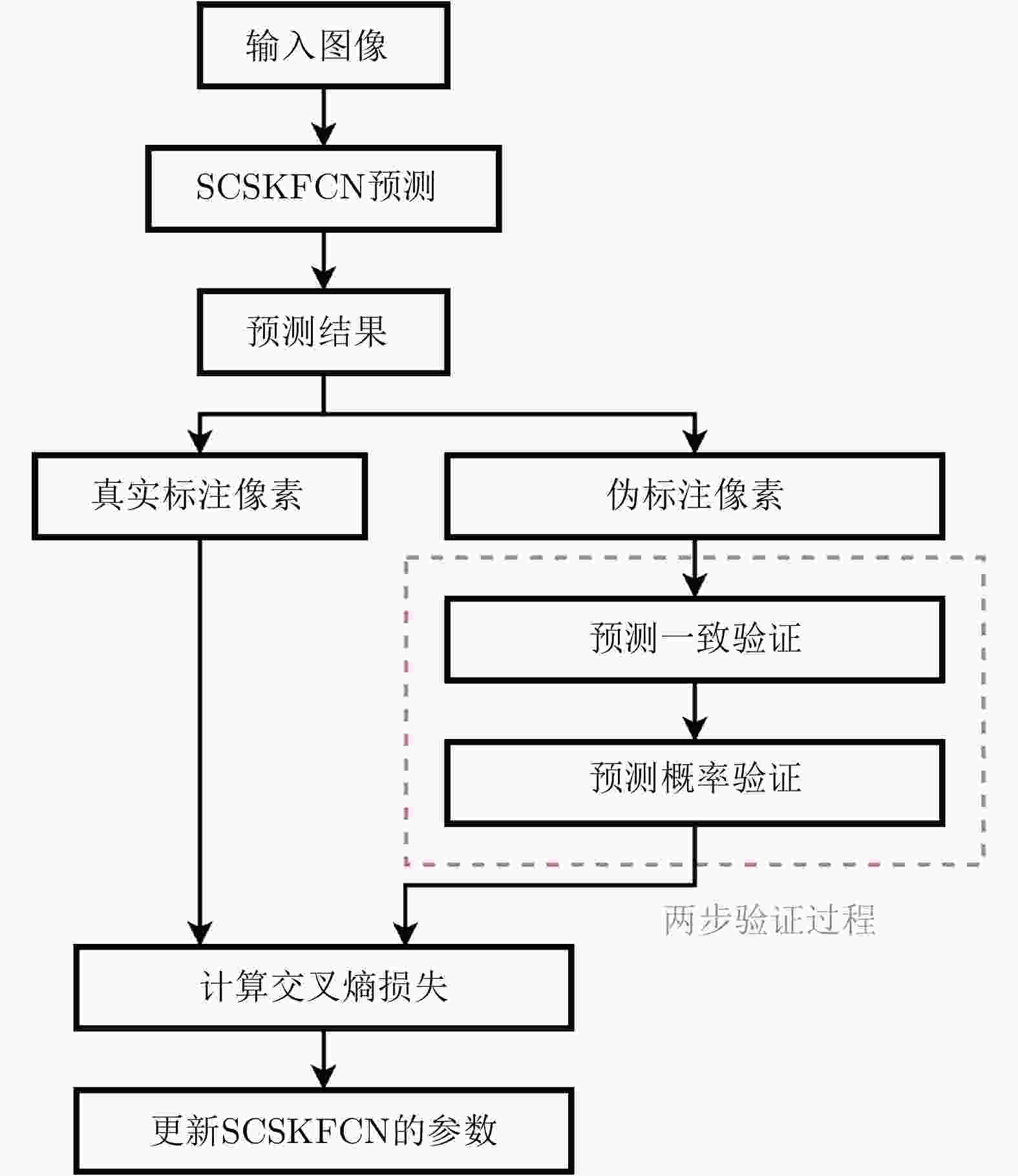
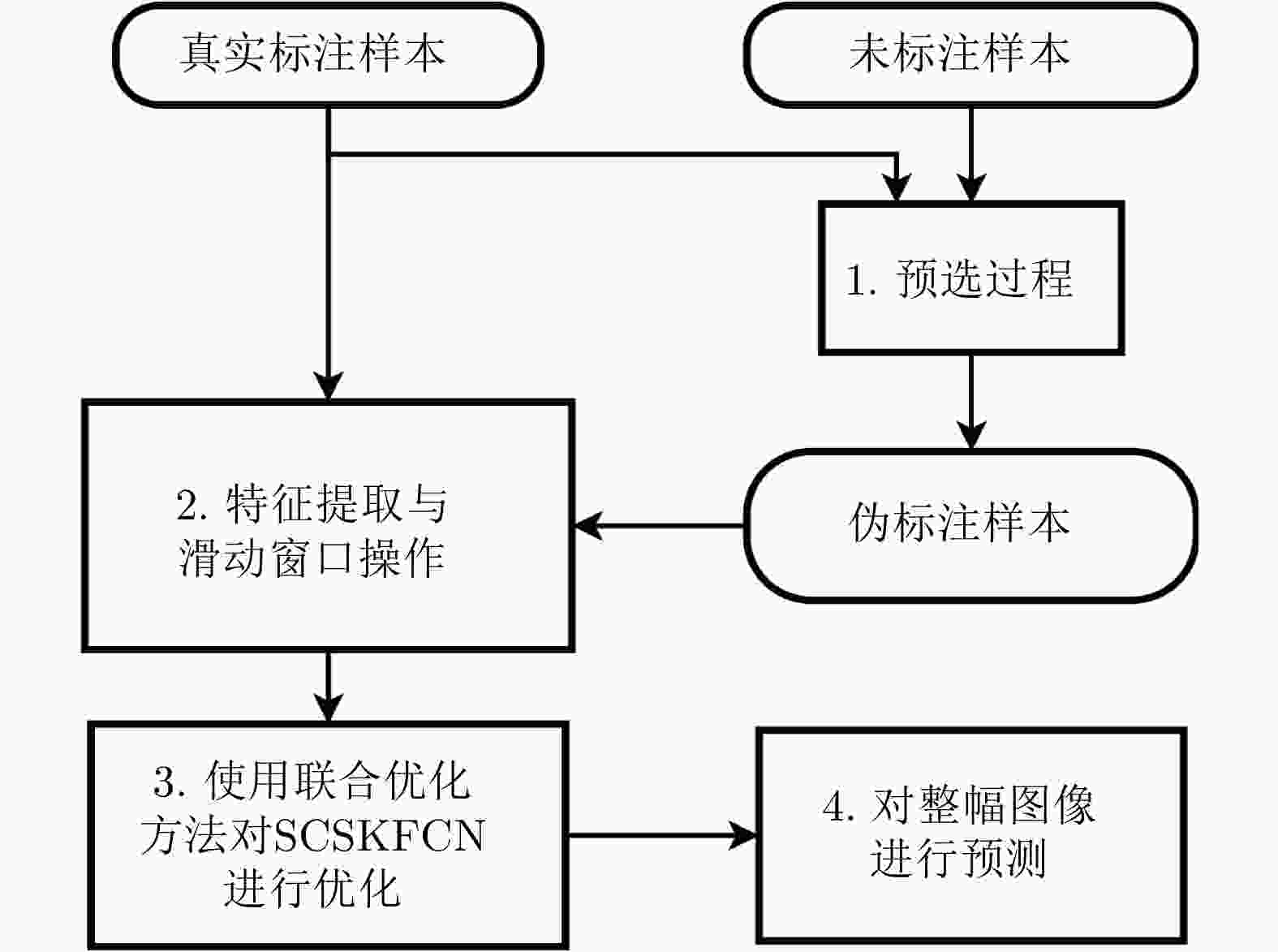
摘要: 针对极化合成孔径雷达(极化SAR)图像地物分类中标注样本数量少的问题，该文提出一种基于空间-通道选择性卷积核全卷积网络(SCSKFCN)和预选-联合优化半监督学习(SPUO)的极化SAR图像地物分类方法。SCSKFCN通过使用空间和通道注意力机制，对不同感受野的特征进行自适应加权融合，有效提升了模型的分类性能。SPUO能够高效地利用标注样本，挖掘无标注样本中蕴含的信息。它采用K-Wishart距离进行样本预选并生成伪标签，然后在联合优化阶段使用真实标注样本和伪标注样本同时对模型进行优化。在模型优化过程中，SPUO对伪标注样本进行两步验证并筛选可靠的伪标注样本参与优化。实验结果表明，该方法能够在只使用少量标注样本的条件下实现高精度、高效率的极化SAR图像地物分类。
-
关键词:
- 极化SAR图像地物分类 /
- 全卷积网络 /
- 注意力机制 /
- 半监督学习 /
- 空间-通道选择性卷积核网络
Abstract: In this paper, a Spatial-Channel Selective Kernel Fully Convolutional Network (SCSKFCN) and a Semi-supervised Preselection-United Optimization (SPUO) method are proposed for polarimetric Synthetic Aperture Radar (SAR) image classification. Integrated with spatial-channel attention mechanism, SCSKFCN adaptively fuses features that have different sizes of reception field, and achieves promising classification performance. SPUO can efficiently extract information contained in unlabeled samples according to annotated samples. It utilizes K-Wishart distance to preselect unlabeled samples for pseudo label generation, and then optimizes SCSKFCN with both labeled and pseudo labeled samples. During the training process of SCSKFCN, a two-step verification mechanism is applied on pseudo labeled samples to reserve reliable samples for united optimization. The experimental results show that the proposed SCSKFCN-SPUO can achieve promising performance and efficiency using limited number of annotated pixels. -
1. 引言
近年来关于太赫兹的研究日趋增加，相对于微波频段雷达，太赫兹雷达以其更高的空间分辨率和角分辨率具有更大的优势受到了越来越多的重视[1,2]。太赫兹辐射的光子能量低，对穿透物不会造成损伤，并且可以穿过大多数介电物质，实现无损检测。太赫兹波具有穿透性，能够实现对隐蔽物体的有效检测，可应用于安检相关的领域。太赫兹频段相比于微波频段频率更高，更容易发射大带宽信号，具有更高的分辨率，具有海量的频谱资源，可用于超宽带超高速无线通信。太赫兹波段目标表面的细微结构、粗糙度等细节会显著影响其后向散射特性，实现更小尺寸目标的探测、更精确目标的运动与物理参数反演[3]。太赫兹(terahertz, THz)波段位于微波与红外波之间，其频率范围为0.1～10 THz (1 THz=1012 Hz)，对应的波长为30 μm～3 mm。太赫兹频段目标散射特性是太赫兹雷达探测和成像应用的物理基础[4,5]，同时也是太赫兹雷达系统进行链路设计、特征提取以及成像算法的重要依据。国内首都师范大学太赫兹实验室研制了太赫兹数字全息成像系统，对太赫兹电磁波的振幅、相位、频率及偏振等全部光学信息的3维空间分布进行精确测量[6]。针对太赫兹波段目标的散射特性，美国麻省LOWELL大学毫米波实验室利用1.56 THz源在紧缩场中对粗糙表面圆柱体的目标散射特性进行了研究[4]。天津大学太赫兹研究中心搭建了以0.2 THz返波管振荡器源、热释电探测器、小型自动旋转光学平台等组成的太赫兹波目标散射特性实验测试系统，并对粗糙铜面的散射特性等进行了研究[7,8]。对于介质[9]和涂覆目标的太赫兹散射，北京航空航天大学江月松等人考虑粗糙度修正表面的散射系数研究了基于经验公式的涂覆目标的太赫兹散射特性[10]。
本文区别于以往采用经验公式[10]以粗糙度修正散射系数的研究方法，把随机粗糙面的建模理念应用到太赫兹波段表面粗糙目标的建模中。首先模拟生成了分形粗糙面近似代替实际复杂的粗糙面，对生成的分形粗糙面进行坐标变换导入计算机辅助设计(Computer Aided Design, CAD)建模软件建立具有粗糙表面的目标模型；然后对表面粗糙目标按照入射波的频率以满足物理光学近似的要求进行剖分。根据菲涅尔反射系数求得表面电流进而计算涂覆粗糙目标的雷达散射截面(Radar Cross Section, RCS)。并针对不同频率以及不同涂覆厚度的表面粗糙涂覆目标，分别进行了仿真分析。
2. 表面粗糙目标模型
2.1 分形粗糙面
自1982年Mandelbrot首次提出“分形”的概念[11]，指的是组成部分与整体以某种方式相形似，分形理论就在很多领域中得到应用。“分形”不同于通常意义上的长度、面积、体积等几何概念，分形内部的任何一个相对独立的部分，在一定程度上都应该是整体的再现和缩影，分形几何体内部存在无穷层次、具有见微知著、由点及面的自相似结构，即具有自相似性。由于粗糙面一般具有非线性的几何结构，因此采用非线性的方法模拟粗糙面更能反映其物理本质。自然界的许多物体，如地、海表面、植被和森林等都在一定尺度范围内存在统计意义上的自相似性，由此很多学者将分形理论应用于电磁散射领域中，用于粗糙面的模拟[12,13]。
1维带限Weierstrass-Mandelbrot分形函数的表达式为：
f(x)=√2δ[1−b(2D−4)]1/2[b(2D−4)N1−b(2D−4)(N2+1)]1/2⋅N2∑n=N1b(D−2)ncos(2πsbnx+φn) (1) 其中，
δ 为高度的均方根，b是空间基频，D为分形维数(1<D<2)，s为标度因子(s=K0/2π , K0为空间波数)，φn 为(0,2π) 上均匀分布的随机相位，该函数具有零均值。一般取b>1，b为有理数时，f(x)表现为周期函数；b为无理数时，f(x)为准周期函数。标度因子s决定频谱的位置，f(x)的无标度区间一般取(sbN1)−1 和(sbN2)−1 ，N=N2−N1+1 ，随着N的增加，越来越多的频率分量加到准周期。图1给出了1维分形粗糙面模型，当分维数D增加时，高频分量比重加大，低频分量作用减小，分形粗糙面的粗糙程度增大。根据瑞利判据，粗糙面相对于入射波的粗糙程度，除与粗糙面的高度函数有关还和入射波的频率有关。如普通的目标表面对于微波段来说是光滑的，但相对于太赫兹频段的波来说却是粗糙的。本文主要研究太赫兹波段下目标表面的微粗糙对其散射特性的影响。2.2 分形表面粗糙目标模型
目标表面粗糙度引起的表面起伏一般在其对应的光滑表面的法线方向[14]。因此，对于轴对称旋转目标而言，其表面的粗糙度可近似考虑为对应母线的起伏。将生成的1维分形粗糙面叠加到光滑目标模型对应的母线进行坐标变换，建立具有分形粗糙表面的目标模型。
对于如图2(a)所示的顶部为半球的粗糙钝锥模型，其母线可以表示为：
x={(r1+f(x))cosα,r1+Δhtanβ+f(x)cosβ,y>0y<0 (2) y={(r1+f(x))sinα,Δh+f(x)sinβ,y>0y<0 (3) 其中，r1为顶部半球半径，r2为底面半径，h为下部锥台高度，
β=atan((r2−r1)/h) 。将生成的圆锥母线导入CAD建模软件，对其绕Y轴旋转并进行坐标变换生成如图2(b)所示的具有分形粗糙表面的钝锥模型。2.3 算法理论
由Stratton-Chu积分公式，目标远区散射场利用物理光学可表示为[15]：
Es=−jk4πexp(−jkr)r∫sˆs×[ˆn×E−Z0ˆs×(ˆn×H)]exp(jkr⋅ˆs)ds (4) 其中，k和Z0分别为自由空间的波数和本征阻抗，
ˆs 为散射波的单位矢量，r为表面上一点的位置矢量，ˆn 为目标表面向外单位法矢量，E和H分别为边界上总的电场和总的磁场。涂覆介质表面的散射示意图如图3所示。其中
θi 为入射角，ˆi 和ˆs 分别为入射波和散射波的单位矢量，矢量ˆei∥ 和ˆer∥ 分别为入射电场、反射电场平行入射面的极化方向，矢量ˆe⊥ 为入射电场和反射电场垂直入射面的极化方向。
图 3 表面涂覆目标示意图Figure 3. Local coordinate systems for PO calculation with coating dielectricEi=E⊥ˆe⊥+E∥ˆe∥i,Es=R⊥E⊥ˆe⊥+R∥E∥ˆe∥r (5) 其中，
Ei 为边界上入射电场，Es 为边界上散射电场，E⊥ 和E∥ 分别为入射电场在ˆe⊥ 和ˆei∥ 方向的场分量，R⊥ 和R∥ 分别为涂覆介质表面在垂直极化和水平极化时的反射系数[16]。涂覆目标雷达散射截面的计算公式为：
σ=limR→∞4πR2|Es|2|Ei|2 (6) 3. 数值结果及讨论
3.1 验证算例
为了验证算法的正确性，先通过下面的模型算例加以说明。图4给出了3 GHz平面波TM极化入射下涂覆半球的双站雷达散射截面，其中半球的半径为0.5 m，涂覆厚度为d=2 cm，涂层介质相对介电常数为
εr=36.0 ，相对磁导率为μr=1.0 。RCS结果曲线可以看出物理光学法和多层快速多极子方法(MLFMA)吻合良好，验证了程序的正确性。图5给出了频率为3 THz的平面波入射下导体立方体的单站雷达散射截面，结果与文献[3]中采用多层快速多极子方法结果一致，可以看出物理光学方法用于计算THz频段目标散射的有效性。
3.2 数值结果
对于图2(b)所示的具有分形粗糙表面的钝锥模型，其顶部半球半径r1=1 mm，底面半径r2=3 mm，锥台高度h=12 mm，分形粗糙面的分维数D=1.5，b=1.5，均方根高度
δ=0.02mm 。涂覆材料相对介电常数εr=(4.0,−1.5) ，相对磁导率μr=(2.0,−1.0) ，涂覆层厚度d=0.03 mm。首先对钝锥导入CAD建模软件进行满足物理光学近似的网格剖分，根据菲涅尔反射系数得出钝锥表面电流分布进而计算其散射场。从图6中结果可以看出，对于模型尺寸相同的光滑钝锥与表面粗糙钝锥的单站雷达散射截面曲线走势基本一致，随着入射角的增大，RCS增大，垂直于锥面照射时达到最大峰值。图6(a)入射频率为30 GHz的情况下光滑钝锥与分形粗糙钝锥的RCS除了小角度基本上重合，可以看出在微波频段目标表面的微粗糙度对RCS的影响很小，可以忽略。图6(b)、图6(c)表明太赫兹波段下光滑钝锥和分形粗糙钝锥目标雷达散射截面出现差异，表面的分形粗糙度引起目标RCS曲线震荡起伏，且频率越高起伏越明显，曲线波动越大。因此在太赫兹波段，目标表面的粗糙度对其散射特性的影响需要考虑。
图 6 钝锥模型单站RCSFigure 6. Mono-static RCS of the coated blunt cone model with different incident frequency图7给出了入射波频率为3 THz的不同涂层厚度的粗糙表面目标的后向RCS。可以看出相对于表面为导体的情况，涂覆介质以后，钝锥目标的雷达散射截面几乎在所有角度都有明显减小，并且随着涂层厚度的增大，雷达散射截面持续减小。涂覆介质层对雷达散射截面的缩减有明显的作用，在一定范围内随着涂层厚度的增大，涂覆介质对电磁波的吸收增加表面粗糙钝锥的后向RCS减小。
图 7 不同涂覆厚度的钝锥单站RCSFigure 7. Mono-static RCS of the blunt cone models coated with different thicknesses图8给出了不同入射频率下钝锥单站RCS。随着频率的升高，表面粗糙钝锥的后向RCS多数角度下降，且频率越高RCS值下降得越多。随着频率的增大，入射波的波长变小，目标表面的粗糙度与入射波长的比值增大，粗糙度引起的漫散射效应增大，目标RCS受到表面粗糙度的影响，曲线峰值变得不明显。
图 8 不同入射频率钝锥模型单站RCSFigure 8. Mono-static RCS of the coating blunt cone models with different incident frequency图9给出了不同表面粗糙度的圆柱模型单站雷达散射截面，其半径为r=16.25 mm，高度为h=102 mm，入射波频率为0.3 THz。
图10给出了不同表面粗糙度的锥柱模型单站雷达散射截面，半径r=16.25 mm，顶部圆锥高度h1=48.5 mm，底部圆柱部分高度h2=102 mm，入射波频率为0.3 THz。从图9和图10给出的结果可以看出，随着均方根高度的增加，目标表面的粗糙度变大，相对于0.3 THz的入射波其波长仅有1 mm，目标更加粗糙，粗糙度对目标的散射结果影响增大。当粗糙度较小时，RCS曲线可以看作是在光滑模型散射结果叠加小起伏震荡；粗糙度增大以后由表面粗糙度引起的RCS起伏甚至在某些角度可以改变光滑模型的散射曲线。
图 10 不同粗糙度锥柱模型单站RCSFigure 10. Mono-static RCS of the cone-cylinder models with differentδ 4. 结论
本文参考分形粗糙面模拟随机环境的方法来建立具有分形粗糙表面目标，采用基于基尔霍夫近似的物理光学方法研究了涂覆目标的太赫兹散射特性。分析了不同的入射波频率以及不同涂层厚度的分形粗糙表面模型在太赫兹波段的散射特性。相对于微波频段波长远大于目标表面微米量级的粗糙度，粗糙度的影响可以不考虑，而在太赫兹波段，波长与粗糙度处于等量级，必须考虑到粗糙度对于目标散射结果的影响。目标表面有涂覆介质材料时，目标的雷达散射截面小于导体情况下的结果，且在一定的范围内涂覆层越厚，目标雷达散射截面吸收越明显。
-
图 3 空间-通道选择性卷积核全卷积网络(SCSKFCN)的架构
Figure 3. Architecture of Spatial-Channel Selective Kernel Fully Convolutional Network (SCSKFCN)
图 5 预选-联合优化半监督学习方法流程图
Figure 5. The framework of semi-supervised preselection and united optimization method
图 6 Flevoland图像分类结果图
Figure 6. Classification results of Flevoland image with different methods
图 7 Oberpfaffenhofen图像分类结果图
Figure 7. Classification results of Oberpfaffenhofen image with different methods
图 10 SCSK单元中卷积核尺寸组合的影响
Figure 10. Impact of different combinations of kernel sizes in SCSK unit
图 11 不同结构的SCSK单元的组成部分
Figure 11. Building blocks of different architectures of SCSK unit
图 14 SPUO中判断准则使用Wishart距离和K-Wishart距离的影响
Figure 14. Impact of using Wishart distance and K-Wishart distance as criterion in SPUO
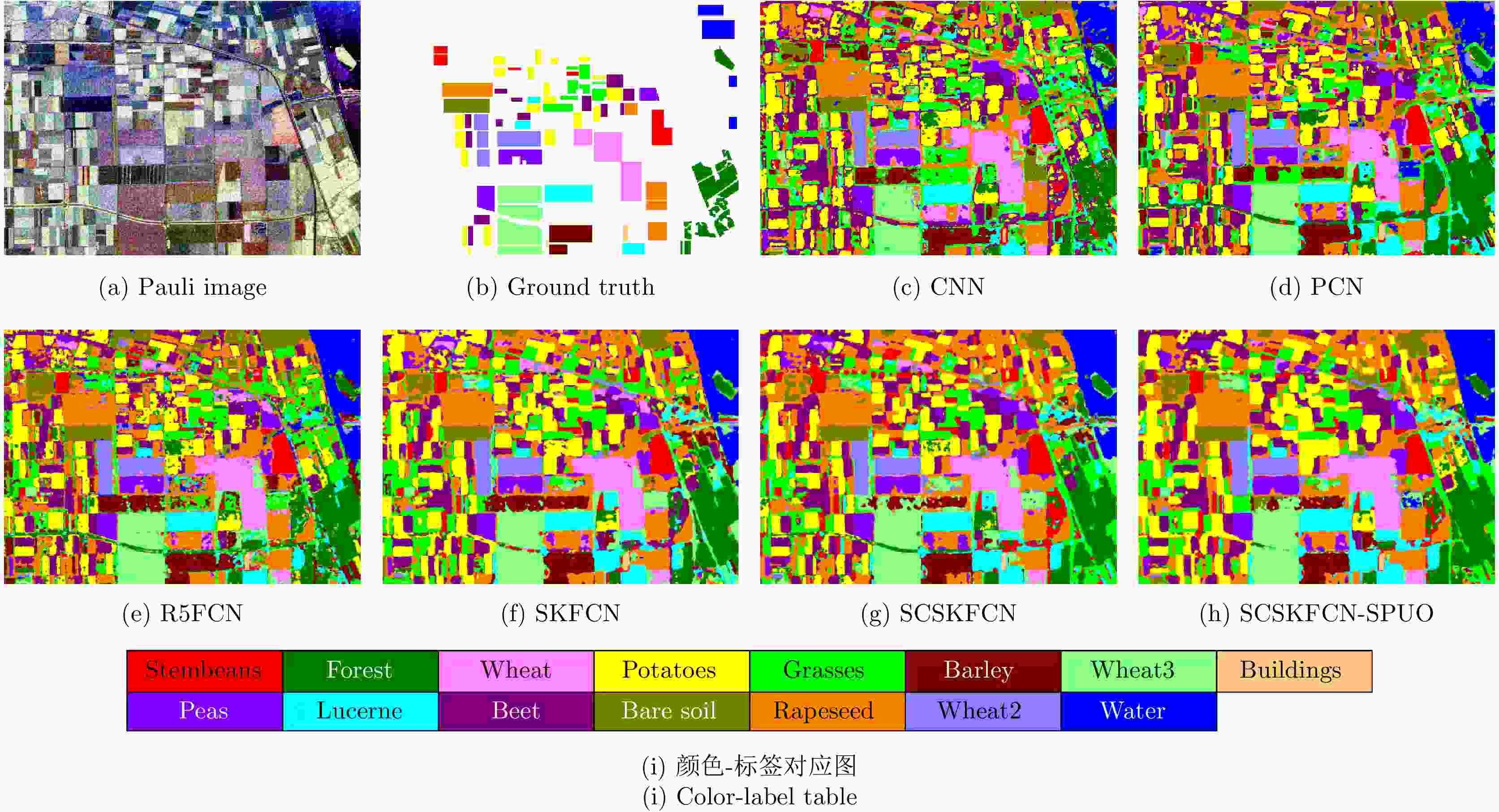
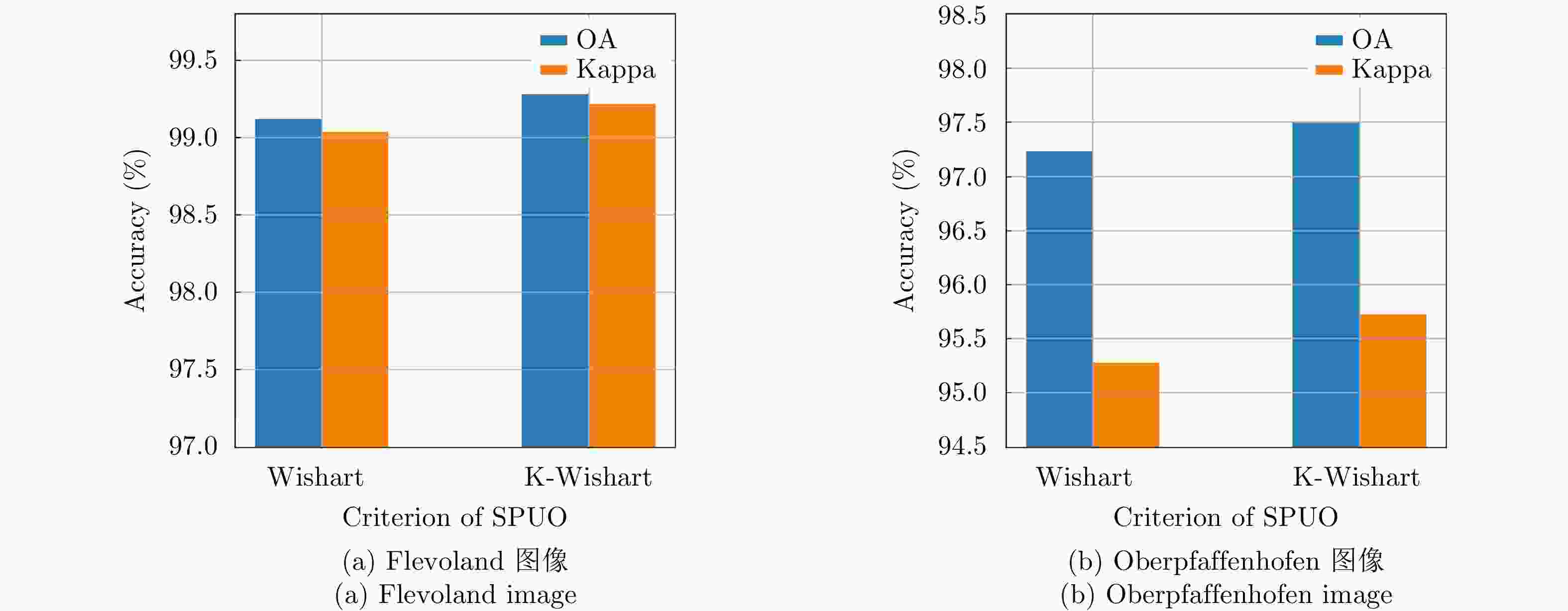
表 1 Flevoland图像分类结果表(%)
Table 1. Classification accuracy comparison on Flevoland image (%)
Method 1 2 3 4 5 6 7 8 9 10 CNN 99.30 93.36 92.22 91.59 93.56 92.79 95.73 97.93 97.54 98.59 PCN 92.11 95.76 98.71 96.49 91.07 96.27 97.52 96.87 96.13 92.23 R5FCN 99.86 98.32 99.32 96.27 93.03 92.12 96.00 97.45 98.15 98.39 SKFCN 99.89 97.39 99.60 98.55 95.42 97.28 96.81 98.80 97.28 98.98 SCSKFCN 99.96 98.60 99.75 98.74 96.47 97.60 97.73 98.99 97.89 98.79 Proposed 99.98 98.87 99.91 99.44 98.93 98.07 99.03 99.78 97.45 99.17 Method 11 12 13 14 15 OA Kappa Training (s) Test (s) CNN 92.25 96.69 98.84 96.78 65.42 95.19 94.76 247.7 3.1 PCN 96.62 96.32 99.05 98.06 81.26 96.36 96.04 216.8 1.2 R5FCN 97.12 97.68 99.61 99.08 86.12 97.41 97.18 89.3 0.9 SKFCN 99.15 99.08 99.61 99.46 83.77 98.46 98.32 105.6 1.0 SCSKFCN 99.47 99.43 99.64 99.73 82.65 98.80 98.70 112.4 1.1 Proposed 99.56 99.68 99.89 99.50 90.51 99.24 99.18 (19.1)+132.8 1.1 *注：Water(1); Stembeans(2); Forest(3); Potatoes(4); Grasses(5); Beet(6); Rapeseed(7); Peas(8); Lucerne(9); Bare soil(10); Wheat2(11); Wheat1(12); Wheat3(13); Barley(14); Building(15); Proposed表示SCSKFCN-SPUO方法 
下载: 导出CSV
表 2 Oberpfaffenhofen图像分类结果表(%)
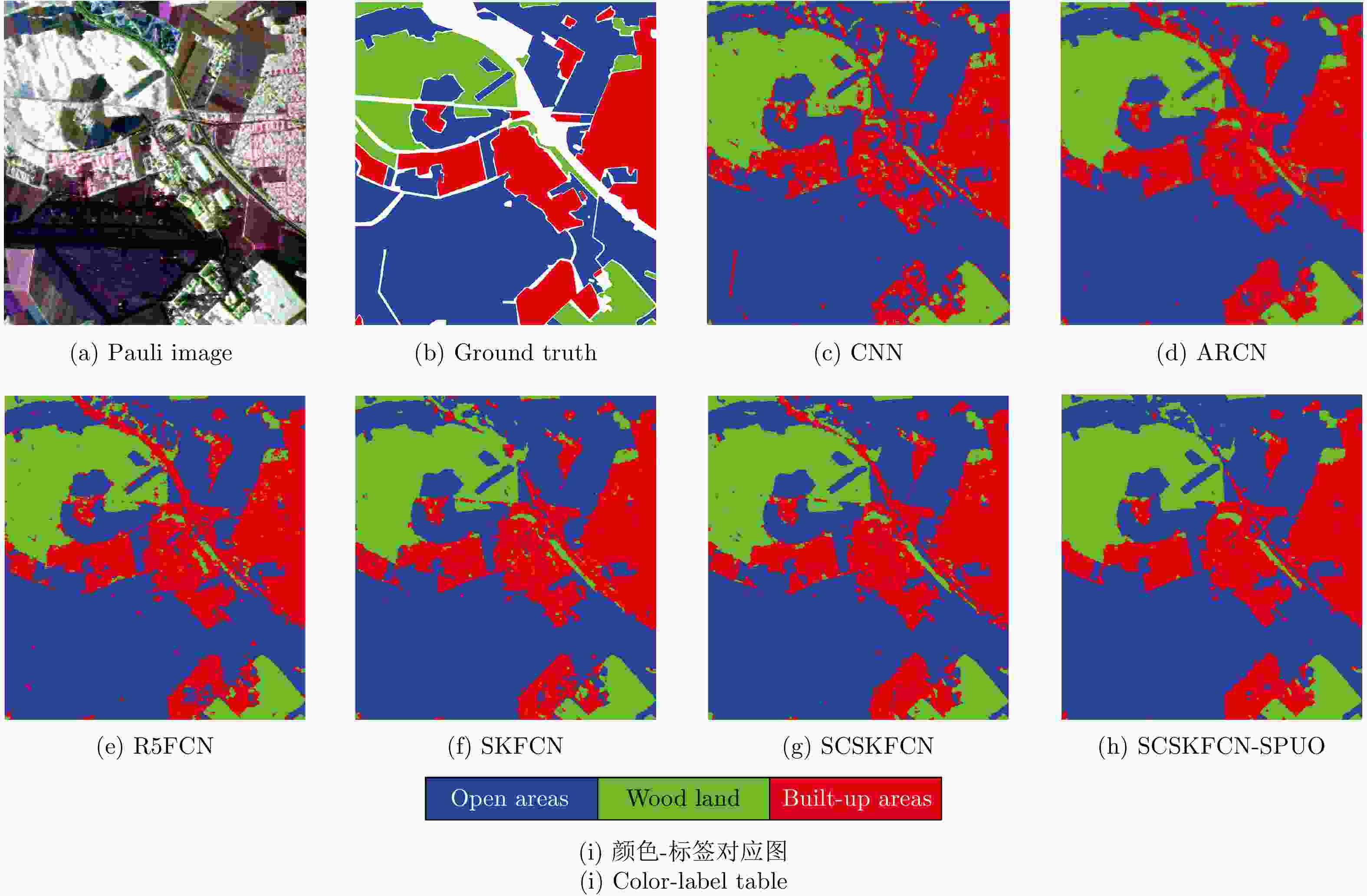
Table 2. Classification accuracy comparison on Oberpfaffenhofen image (%)
Class CNN ARCN R5FCN SKFCN SCSKFCN Proposed Wood land 95.02 96.13 94.97 96.25 96.68 97.18 Open areas 97.17 97.32 97.51 97.76 97.99 98.80 Built-up areas 86.42 93.92 90.47 94.27 94.64 94.86 OA 94.09 96.25 95.27 96.60 96.90 97.51 Kappa 89.88 93.64 91.95 94.23 94.74 95.76 Training (s) 225.9 189.6 120.1 137.2 159.2 (50.3)+173.6 Test (s) 4.6 1.6 1.5 1.7 1.9 1.9 *注：Proposed表示SCSKFCN-SPUO方法
下载: 导出CSV
-
[1] LEE J S and POTTIER E. Polarimetric Radar Imaging: From Basics to Applications[M]. Boca Raton, USA, CRC Press, 2017: 1–10. [2] LEE J S, GRUNES M R, AINSWORTH T L, et al. Unsupervised classification using polarimetric decomposition and the complex Wishart classifier[J]. IEEE Transactions on Geoscience and Remote Sensing, 1999, 37(5): 2249–2258. doi: 10.1109/36.789621 [3] WANG Haipeng, XU Feng and JIN Yaqiu. A review of polsar image classification: From polarimetry to deep learning[C]. IEEE International Geoscience and Remote Sensing Symposium, Yokohama, Japan, 2019: 3189–3192. doi: 10.1109/IGARSS.2019.8899902. [4] CLOUDE S R and POTTIER E. An entropy based classification scheme for land applications of polarimetric SAR[J]. IEEE Transactions on Geoscience and Remote Sensing, 1997, 35(1): 68–78. doi: 10.1109/36.551935 [5] FREEMAN A and DURDEN S L. A three-component scattering model for polarimetric SAR data[J]. IEEE Transactions on Geoscience and Remote Sensing, 1998, 36(3): 963–973. doi: 10.1109/36.673687 [6] DEMPSTER A P, LAIRD N M, and RUBIN D B. Maximum likelihood from incomplete data via the EM algorithm[J]. Journal of the Royal Statistical Society: Series B (Methodological) , 1977, 39(1): 1–38. doi: 10.1111/j.2517-6161.1977.tb01600.x [7] FUKUDA S and HIROSAWA H. Support vector machine classification of land cover: Application to polarimetric SAR data[C]. IGARSS 2001. Scanning the Present and Resolving the Future. Proceedings. IEEE 2001 International Geoscience and Remote Sensing Symposium, Sydney, Australia, 2001: 187–189. doi: 10.1109/IGARSS.2001.976097. [8] ZHANG Lamei, SUN Liangjie, and MOON W M. Polarimetric SAR image classification based on contextual sparse representation[C]. IEEE International Geoscience and Remote Sensing Symposium, Milan, Italy, 2015: 1837–1840. doi: 10.1109/IGARSS.2015.7326149. [9] ERSAHIN K, CUMMING I G, and WARD R K. Segmentation and classification of polarimetric SAR data using spectral graph partitioning[J]. IEEE Transactions on Geoscience and Remote Sensing, 2010, 48(1): 164–174. doi: 10.1109/TGRS.2009.2024303 [10] DU Peijun, SAMAT A, WASKE B, et al. Random forest and rotation forest for fully polarized SAR image classification using polarimetric and spatial features[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2015, 105: 38–53. doi: 10.1016/j.isprsjprs.2015.03.002 [11] LEE J S, GRUNES M R, POTTIER E, et al. Unsupervised terrain classification preserving polarimetric scattering characteristics[J]. IEEE Transactions on Geoscience and Remote Sensing, 2004, 42(4): 722–731. doi: 10.1109/TGRS.2003.819883 [12] LEE J S, SCHULER D L, LANG R H, et al. K-distribution for multi-look processed polarimetric SAR imagery[C]. IEEE International Geoscience and Remote Sensing Symposium, Pasadena, USA, 1994: 2179–2181. doi: 10.1109/IGARSS.1994.399685. [13] DOULGERIS A P, ANFINSEN S N, and ELTOFT T. Classification with a non-Gaussian model for PolSAR data[J]. IEEE Transactions on Geoscience and Remote Sensing, 2008, 46(10): 2999–3009. doi: 10.1109/TGRS.2008.923025 [14] DOULGERIS A P. An automatic U-distribution and Markov random field segmentation algorithm for PolSAR images[J]. IEEE Transactions on Geoscience and Remote Sensing, 2015, 53(4): 1819–1827. doi: 10.1109/TGRS.2014.2349575 [15] LECUN Y, BOTTOU L, BENGIO Y, et al. Gradient-based learning applied to document recognition[J]. Proceedings of the IEEE, 1998, 86(11): 2278–2324. doi: 10.1109/5.726791 [16] SHELHAMER E, LONG J, and DARRELL T. Fully convolutional networks for semantic segmentation[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2017, 39(4): 640–651. doi: 10.1109/TPAMI.2016.2572683 [17] ZHOU Yu, WANG Haipeng, XU Feng, et al. Polarimetric SAR image classification using deep convolutional neural networks[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(12): 1935–1939. doi: 10.1109/LGRS.2016.2618840 [18] CHEN Siwei and TAO Chensong. PolSAR image classification using polarimetric-feature-driven deep convolutional neural network[J]. IEEE Geoscience and Remote Sensing Letters, 2018, 15(4): 627–631. doi: 10.1109/LGRS.2018.2799877 [19] CHEN Siwei, LI Yongzhen, DAI Dahai, et al. Uniform polarimetric matrix rotation theory[C]. IEEE International Geoscience and Remote Sensing Symposium, Melbourne, Australia, 2013: 4166–4169. doi: 10.1109/IGARSS.2013.6723751. [20] MOHAMMADIMANESH F, SALEHI B, MAHDIANPARI M, et al. A new fully convolutional neural network for semantic segmentation of polarimetric SAR imagery in complex land cover ecosystem[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2019, 151: 223–236. doi: 10.1016/j.isprsjprs.2019.03.015 [21] LIU Xu, JIAO Licheng, TANG Xu, et al. Polarimetric convolutional network for PolSAR image classification[J]. IEEE Transactions on Geoscience and Remote Sensing, 2019, 57(5): 3040–3054. doi: 10.1109/TGRS.2018.2879984 [22] LI Yangyang, CHEN Yanqiao, LIU Guangyuan, et al. A novel deep fully convolutional network for PolSAR image classification[J]. Remote Sensing, 2018, 10(12): 1984. doi: 10.3390/rs10121984 [23] CHEN Yanqiao, LI Yangyang, JIAO Licheng, et al. Adversarial reconstruction-classification networks for PolSAR image classification[J]. Remote Sensing, 2019, 11(4): 415. doi: 10.3390/rs11040415 [24] GENG Jie, MA Xiaorui, FAN Jianchao, et al. Semisupervised classification of polarimetric SAR image via superpixel restrained deep neural network[J]. IEEE Geoscience and Remote Sensing Letters, 2018, 15(1): 122–126. doi: 10.1109/LGRS.2017.2777450 [25] BI Haixia, SUN Jian, and XU Zongben. A graph-based semisupervised deep learning model for PolSAR image classification[J]. IEEE Transactions on Geoscience and Remote Sensing, 2019, 57(4): 2116–2132. doi: 10.1109/TGRS.2018.2871504 [26] XIE Wen, MA Gaini, ZHAO Feng, et al. PolSAR image classification via a novel semi-supervised recurrent complex-valued convolution neural network[J]. Neurocomputing, 2020, 388: 255–268. doi: 10.1016/j.neucom.2020.01.020 [27] HUA Wenqiang, WANG Shuang, XIE Wen, et al. Dual-channel convolutional neural network for polarimetric SAR images classification[C]. IEEE International Geoscience and Remote Sensing Symposium, Yokohama, Japan, 2019: 3201–3204. doi: 10.1109/IGARSS.2019.8899103. [28] LI Xiang, WANG Wenhai, HU Xiaolin, et al. Selective kernel networks[C]. IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR), Long Beach, USA, 2019: 510–519. doi: 10.1109/CVPR.2019.00060. [29] WOO S, PARK J, LEE J Y, et al. CBAM: Convolutional block attention module[C]. 15th European Conference on Computer Vision-ECCV 2018, Munich, Germany, 2018: 3–19. doi: 10.1007/978-3-030-01234-2_1. [30] PARK J, WOO S, LEE J Y, et al. BAM: Bottleneck attention module[J]. arXiv preprint arXiv: 1807.06514, 2018. [31] XU Bing, WANG Naiyan, CHEN Tianqi, et al. Empirical evaluation of rectified activations in convolutional network[J]. arXiv preprint arXiv: 1505.00853, 2015. [32] MAAS A L, HANNUN A Y, and NG A Y. Rectifier nonlinearities improve neural network acoustic models[C]. The 30th International Conference on Machine Learning, Atlanta, USA, 2013. [33] YU F and KOLTUN V. Multi-scale context aggregation by dilated convolutions[J]. arXiv preprint arXiv: 1511.07122, 2015. [34] HU Jie, SHEN Li, ALBANIE S, et al. Squeeze-and-excitation networks[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2020, 42(8): 2011–2023. doi: 10.1109/TPAMI.2019.2913372 [35] LI Mu. Efficient mini-batch training for stochastic optimization[C]. The 20th ACM Sigkdd International Conference on Knowledge Discovery and Data Mining, Virtual Event, Singapore, 2014: 661–670. 期刊类型引用(4)
1. 杨帆，何嘉岳，杨瑶佳，金一飞，许慎恒，李懋坤. 界面电磁学的理论与应用. 微波学报. 2023(05): 52-61 . 
百度学术2. 王禄炀，兰峰，宋天阳，何贵举，潘一博，张雅鑫，陈智，杨梓强. 多功能动态波束调控的太赫兹编码超表面. 无线电通信技术. 2022(02): 247-252 . 百度学术3. 周嵩林，唐隽文，刘罗颢，吴优，刘长昊，金一飞，杨帆，许慎恒，李懋坤. 基于电磁表面的阵列天线及应用概述. 通信学报. 2022(12): 13-23 . 百度学术4. 李国英，嵇成高，于刚刚，关浩. 相控雷达成像测井仪器中收发天线系统设计. 测井技术. 2022(06): 696-700+706 . 百度学术其他类型引用(1)
-
下载:
百度学术
计量
- 文章访问数: 1568
- HTML全文浏览量: 684
- PDF下载量: 173
- 被引次数: 5
