作者中心
专家审稿
责编办公
编辑办公
-
摘要: 相位波前受轨道角动量调制的涡旋电磁波，在雷达前视成像领域得到广泛关注和研究。基于涡旋电磁波雷达成像原理和方法，该文重点对方位分辨力展开研究。首先，分析了贝塞尔幅度项对方位分辨性能的影响，结果表明，在贝塞尔幅度窗影响下，涡旋电磁波雷达方位分辨性能由有效OAM模态范围决定。其次，提出了一种有效OAM模态范围计算方法，并分别对方位角分辨率、空间分辨率以及超实孔径雷达分辨率进行了表征。最后，仿真分析了方位基本分辨性能随不同影响因素的变化规律，改变波长孔径比、成像俯仰角，能增大有效OAM模态范围，提升方位分辨性能。拟合得到了有效OAM模态范围、超实孔径雷达分辨率关于波长孔径比和成像俯仰角的近似表达式，为涡旋电磁波雷达参数设计与优化提供参考。Abstract: The vortex ElectroMagnetic (EM) wave, whose phase wavefront is modulated by the Orbital Angular Momentum (OAM), has received immense attention, especially in the field of forward-looking radar imaging. Based on the fundamental principle and imaging method of the vortex EM radar, the azimuth resolution was studied in this paper. First, the circumstance of considering the Bessel amplitude term was analyzed, indicating that the azimuth resolution was determined by the effective scope of OAM modes. Then, an effective method for calculating the scope of OAM modes was proposed, including the expressions of azimuth resolution, spatial resolution, and super-resolution were characterized. Finally, the fundamental resolution performance with different influencing factors was analyzed via simulations. The analysis showed that changing the wavelength aperture ratio and imaging elevation could increase the effective scope of OAM modes, which improved the azimuth resolution. Through data fitting, the approximate expressions of the effective scope of OAM modes and the resolution of the super-real aperture radar with respect to the wavelength aperture ratio and imaging elevation were obtained separately, providing a reference for the parameters design and optimization of vortex EM wave radar.
-
图 2 理想情况涡旋电磁波雷达方位空间分辨率以及超实孔径雷达分辨率曲线
Figure 2. Ideally spatial-resolution and the super resolution as a function of different influence factors
图 3 贝塞尔相位补偿及对应的点扩展函数图
Figure 3. Bessel term compensation and the corresponding point spread function of azimuth
图 4 不同参数下贝塞尔幅度窗及方位剖面图
Figure 4. Bessel windows and azimuth profiles with different parameters
图 5 有效OAM模态范围计算流程
Figure 5. The flowchart of the effective OAM modes scope calculation
-
[1] 保铮, 邢孟道, 王彤. 雷达成像技术[M]. 北京: 电子工业出版社, 2005: 89–181.BAO Zheng, XING Mengdao, and WANG Tong. Radar Imaging Technology[M]. Beijing: Electronic Industry Press, 2005: 89–181. [2] CHEN C C and ANDREWS H C. Target-motion-induced radar imaging[J]. IEEE Transactions on Aerospace and Electronic Systems, 1980, AES-16(1): 2–14. doi: 10.1109/TAES.1980.308873 [3] 王小谟, 张光义. 雷达与探测: 信息化战争的火眼金睛[M]. 第2版北京: 国防工业出版社, 2008: 185–230.WANG Xiaomo and ZHANG Guangyi. Radar and Detection: The Golden Eye of Information Warfare[M]. Second Edition Beijing: National Defense Industry Press, 2008: 185–230. [4] BAMLER R. A comparison of range-Doppler and wavenumber domain SAR focusing algorithms[J]. IEEE Transactions on Geoscience and Remote Sensing, 1992, 30(4): 706–713. doi: 10.1109/36.158864 [5] XING Mengdao, WU Renbiao, LAN Jinqiao, et al. Migration through resolution cell compensation in ISAR imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2004, 1(2): 141–144. doi: 10.1109/LGRS.2004.824766 [6] 张磊. 高分辨SAR/ISAR成像及误差补偿技术研究[D]. [博士论文], 西安电子科技大学, 2012.ZHANG Lei. Study on high resolution SAR/ISAR imaging and error correction[D]. [Ph. D. dissertation], Xidian University, 2012. [7] 吴迪, 朱岱寅, 朱兆达. 机载雷达单脉冲前视成像算法[J]. 中国图象图形学报, 2010, 15(3): 462–469. doi: 10.11834/jig.20100317WU Di, ZHU Daiyin, and ZHU Zhaoda. Research on nomopulse forward-looking imaging algorithm for airborne radar[J]. Journal of Image and Graphics, 2010, 15(3): 462–469. doi: 10.11834/jig.20100317 [8] 张慧. 微波毫米波阵列成像关键技术研究[D]. [博士论文], 东南大学, 2016.ZHANG Hui. Investigations on microwave and millimeter wave array imaging key technologies[D]. [Ph. D. dissertation], Southeast University, 2016. [9] 张寅. 运动平台前视雷达超分辨成像理论与方法[D]. [博士论文], 电子科技大学, 2016.ZHANG Yin. Theory and method of superresolution imaging for forward-looking radar of moving platform[D]. [Ph. D. dissertation], University of Electronic Science and Technology of China, 2016. [10] 何学智. 微波凝视关联成像的信息处理方法与仿真[D]. [博士论文], 中国科学技术大学, 2013.HE Xuezhi. The information processing methods and simulations in microwave staring correlated imaging[D]. [Ph. D. dissertation], University of Science and Technology of China, 2013. [11] 杨阳. 涡旋电磁波的产生及其在成像领域的应用[D]. [博士论文], 电子科技大学, 2020.YANG Yang. The generation of the vortex electromagnetic waves and its application in imaging region[D]. [Ph. D. dissertation], University of Electronic Science and Technology of China, 2020. [12] HUMBLET J. Sur le moment d’impulsion d’une onde électromagnétique[J]. Physica, 1943, 10(7): 585–603. doi: 10.1016/S0031-8914(43)90626-3 [13] POYNTING J H. The wave motion of a revolving shaft, and a suggestion as to the angular momentum in a beam of circularly polarised light[J]. Proceedings of the Royal Society A, 1909, 82(557): 560–567. doi: 10.1098/rspa.1909.0060 [14] BETH R A. Mechanical detection and measurement of the angular momentum of light[J]. Physical Review, 1936, 50(2): 115–125. doi: 10.1103/PhysRev.50.115 [15] MOHAMMADI S M, DALDORFF L K S, BERGMAN J E S, et al. Orbital angular momentum in radio—A system study[J]. IEEE Transactions on Antennas and Propagation, 2010, 58(2): 565–572. doi: 10.1109/TAP.2009.2037701 [16] LIU Kang, CHENG Yongqiang, YANG Zhaocheng, et al. Orbital-angular-momentum-based electromagnetic vortex imaging[J]. IEEE Antennas and Wireless Propagation Letters, 2015, 14: 711–714. doi: 10.1109/LAWP.2014.2376970 [17] LIU Kang, LIU Hongyan, QIN Yuliang, et al. Generation of OAM beams using phased array in the microwave band[J]. IEEE Transactions on Antennas and Propagation, 2016, 64(9): 3850–3857. doi: 10.1109/TAP.2016.2589960 [18] ZENG Yanzhi, WANG Yang, CHEN Zhihui, et al. Two-dimensional OAM radar imaging using uniform circular antenna arrays[C]. The 14th European Conference on Antennas and Propagation, Copenhagen, Denmark, 2020: 1–4. doi: 10.23919/EuCAP48036.2020.9135917. [19] CHEN Rui, LONG Wenxuan, GAO Yue, et al. Orbital angular momentum-based two-dimensional super-resolution targets imaging[C]. 2018 IEEE Global Conference on Signal and information Processing, Anaheim, USA, 2018: 1243–1246. doi: 10.1109/GlobalSIP.2018.8646368. [20] LIU Kang, LI Xiang, GAO Yue, et al. High-resolution electromagnetic vortex imaging based on sparse Bayesian learning[J]. IEEE Sensors Journal, 2017, 17(21): 6918–6927. doi: 10.1109/JSEN.2017.2754554 [21] LIU Hongyan, LIU Kang, CHENG Yongqiang, et al. Microwave vortex imaging based on dual coupled OAM beams[J]. IEEE Sensors Journal, 2020, 20(2): 806–815. doi: 10.1109/JSEN.2019.2943698 [22] LIU Kang, CHENG Yongqiang, GAO Yue, et al. Super-resolution radar imaging based on experimental OAM beams[J]. Applied Physics Letters, 2017, 110(16): 164102. 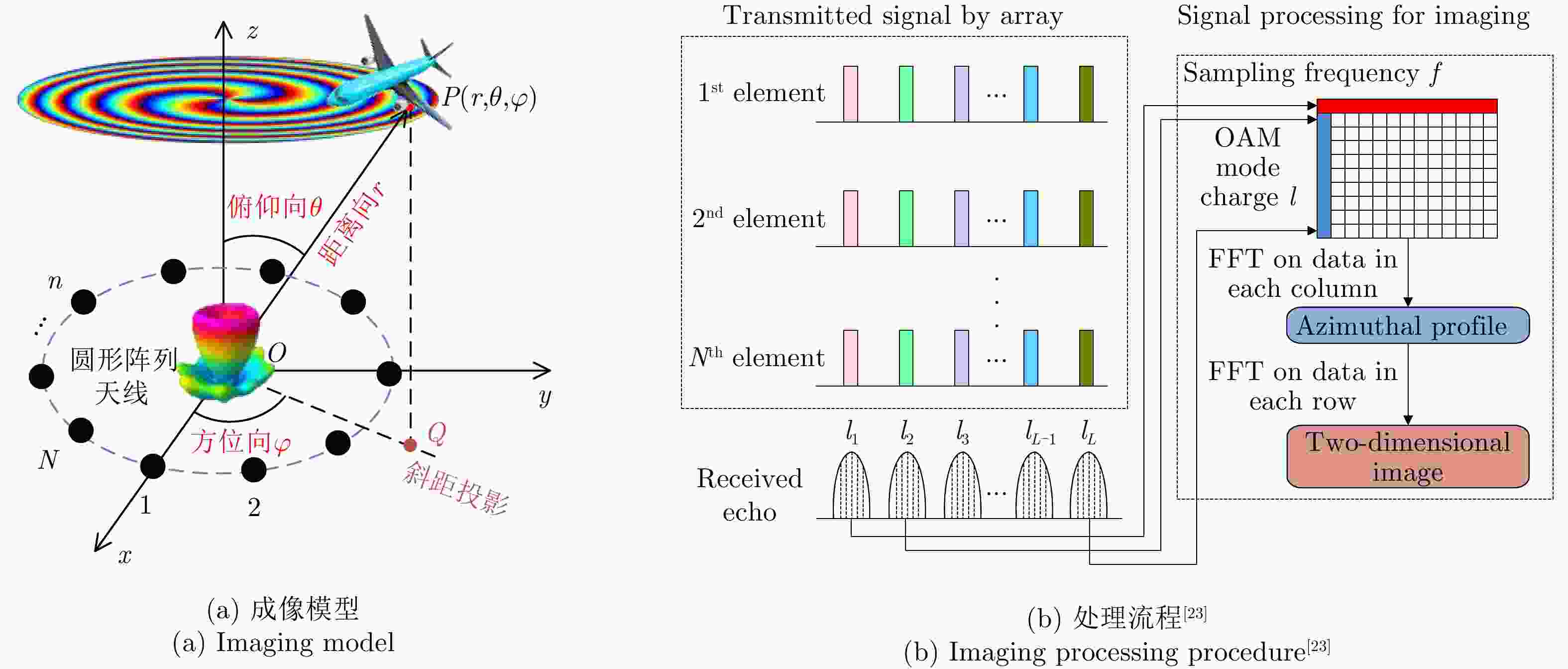doi: 10.1063/1.4981253 [23] LIU Kang, CHENG Yongqiang, LI Xiang, et al. Microwave-sensing technology using orbital angular momentum: Overview of its advantages[J]. IEEE Vehicular Technology Magazine, 2019, 14(2): 112–118. doi: 10.1109/MVT.2018.2890673 -
下载:
图(8)
计量
- 文章访问数: 3180
- HTML全文浏览量: 1543
- PDF下载量: 392
- 被引次数: 0
