任意多次散射机理的GTD散射中心模型频率依赖因子表达

闫华; 张磊; 陆金文; 邢笑宇; 李胜; 殷红成

doi:10.12000/JR21005

任意多次散射机理的GTD散射中心模型频率依赖因子表达

DOI: 10.12000/JR21005

电磁散射重点实验室北京 100854

基金项目: 国家重点研发计划(2018YFC0825804)

详细信息

作者简介:
闫　华(1981–)，男，博士，电磁散射重点实验室高级工程师，研究方向为雷达目标散射特性、计算电磁学、特征提取、参数化建模等

张　磊(1991–)，男，博士，电磁散射重点实验室工程师，主要研究方向为雷达目标散射特性、散射中心参数化建模、SAR目标识别等

陆金文(1994–)，男，电磁散射重点实验室博士研究生，主要研究方向为雷达目标散射特性、电磁散射参数化建模等

李　胜(1980–)，男，硕士，电磁散射重点实验室高级工程师，主要研究方向为雷达目标特征控制、特征提取等

殷红成(1967–)，男，博士，电磁散射重点实验室研究员，研究方向为雷达目标特性、计算电磁学、目标识别等

通讯作者:
闫华 yanhuabit@126.com

责任主编：朱国强 Corresponding Editor: ZHU Guoqiang
中图分类号: TN95
计量
- 文章访问数: 2561
- HTML全文浏览量: 1144
- PDF下载量: 186
- 被引次数: 0
出版历程
- 收稿日期: 2021-01-11
- 修回日期: 2021-03-01
- 网络出版日期: 2021-03-23
- 刊出日期: 2021-06-28

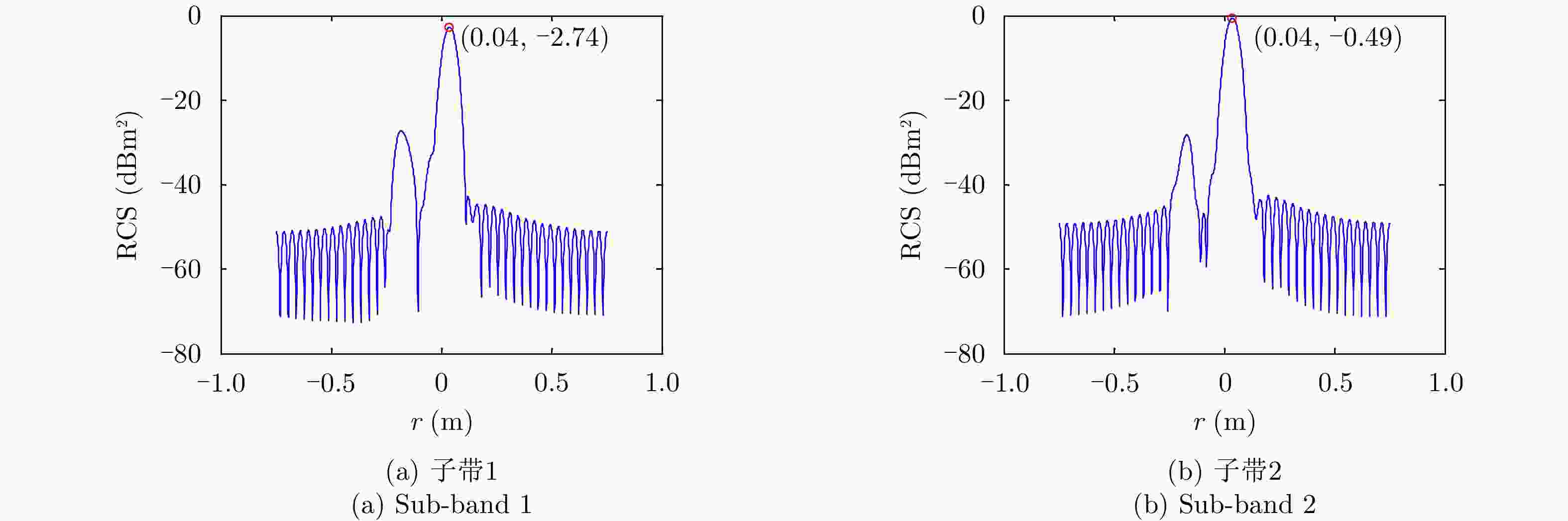
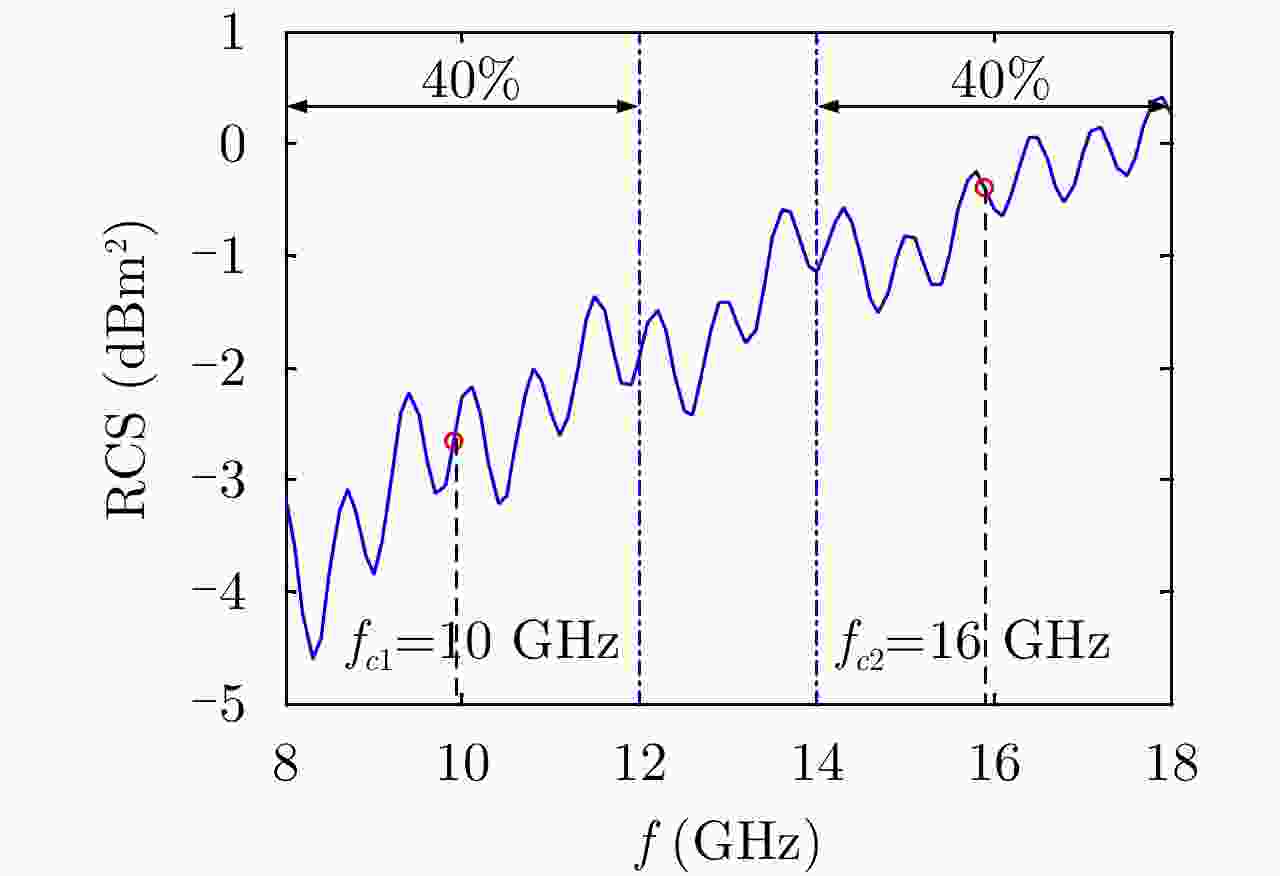
Frequency-dependent Factor Expression of the GTD Scattering Center Model for the Arbitrary Multiple Scattering Mechanism

Science and Technology on Electromagnetic Scattering Laboratory, Beijing 100854, China

Funds: The National Key Research and Development Program of China (2018YFC0825804)

More Information

Corresponding author: YAN Hua, yanhuabit@126.com

摘要

摘要: 几何绕射理论(GTD)模型是一种重要的散射中心模型，能准确描述雷达目标主要散射机理的频率依赖行为，但目前在频率依赖因子与散射机理类型之间尚未建立明确、一般的数学关系。该文从射线理论出发，结合几何光学(GO), GTD, 物理绕射理论(PTD)和驻相法(SPM)等方法，推导了理想电导体(PEC)目标任意多次散射机理的频率依赖因子数学表达式。该表达式具有简洁、统一的解析形式，指出散射中心频率依赖因子与形成散射中心的射线反射次数、射线经过的几何元素维数以及射线场焦散情况等因素有关。一系列典型组合体目标的电磁仿真与微波暗室测量数据验证了提出公式的有效性。该文提出的频率依赖因子表达可应用于正向参数化建模中频率依赖因子的正向推算。
- 散射中心模型 /
- 多次散射机理 /
- GTD模型 /
- 频率依赖因子
Abstract: This paper presents a derivation of a formula with a concise and uniform analytic form by the Stationary Phase Method (SPM) plus Geometrical Optics (GO), the Physical Theory of Diffraction (PTD), and Geometrical Theory of Diffraction (GTD) to calculate the frequency-dependent factor for the arbitrary multiple scattering mechanism, validated by the simulated and measured data of a series of canonical ensembles, validated by the simulated and measured data of a series of canonical ensembles. Although the GTD model, a scattering center model, can accurately describe the frequency-dependent characteristic of several main scattering mechanisms of the radar target, no explicit and general expression relates the frequency-dependent factor to the type of scattering mechanism. The derived formula relates the scattering center’s frequency-dependent factor with bounce times, dimensions of all the encountered geometrical elements, and a caustic type of ray contributing to the scattering center and can be applied to determine the parameter value of frequency-dependent factor of the GTD model and its derived versions in the forward parametric modeling.
- Scattering center model /
- Multiple scattering mechanism /
- GTD model /
- Frequency-dependent factor

HTML全文

图 1 圆柱-圆柱垂直组合体的RCS曲线与子带分割示意图

Figure 1. RCS curve for cylinder-cylinder orthogonal combination objects and sketch map of frequeny band splitting

下载: 全尺寸图片幻灯片

图 2 各子带散射中心参数提取结果

Figure 2. Results of scattering center extraction for each sub-band scattering data

下载: 全尺寸图片幻灯片

表 1 GTD模型频率依赖因子取值及其对应的散射机理类型

Table 1. The values of frequency-dependent factor of GTD model and corresponding mechanisms

频率依赖因子取值	散射机理类型
1	平板、二面角、三面角的反射
1/2	单弯曲曲面的反射
0	双弯曲曲面的反射、直边的绕射
–1/2	曲边的绕射
–1	尖顶、角的绕射

下载: 导出CSV

表 2 6种典型体尺寸参数列表

Table 2. Size parameters for 6 canonical objects

典型体名称	尺寸参数
方形平板	边长500 mm，厚度10 mm
圆柱体	(1)直径200 mm，长300 mm；(2)直径150 mm，长300 mm
球体	(1)直径100 mm；(2)直径300 mm
半圆锥体	(1)直径50 mm, 100 mm；(2)直径125 mm, 400 mm
直角四面体	(1)底边200 mm，棱边200 mm，劈角20°；(2)底边350 mm，棱边350 mm，劈角50°
圆盘	半径500 mm，厚度8 mm

下载: 导出CSV

表 3 20种组合体目标及其中产生的二次反射/绕射机理的几何结构示意与频率依赖因子取值

Table 3. Types of double reflection/diffraction mechanisms, 20 combination objects, corresponding geometric diagram and theoretical values of the frequency-dependent factor

散射机理类型	组合体名称	几何示意图	理论α值
镜面反射-镜面反射	平板-平板(垂直)		1
	圆柱-平板(垂直、平行)		1/2
	球-平板		0
	圆柱-圆柱(垂直、平行)		1/2
	球-圆柱		0
	球-球		0
边缘绕射-镜面反射	直劈-平板(垂直、平行)		0
	直劈-圆柱(垂直、平行)		0
	直劈-球		–1/2
	曲劈-平板		–1/2
	曲劈-圆柱		–1/2
	曲劈-球		–1/2
边缘绕射-边缘绕射	直劈-直劈(垂直、平行)		–1/2
	曲劈-直劈		–1
	曲劈-曲劈		–1

下载: 导出CSV

表 4 基于20种组合体仿真数据的二次散射机理形成散射中心的频率依赖因子估计与理论值对比

Table 4. Comparison of theoretical frequency-dependent factor values by proposed formula and estimated ones by simulation data for scattering centers induced by double scattering from 20 combination objects

组合体名称	估计α值		理论α值	组合体名称	估计α值		理论α值
组合体名称	VV	HH	理论α值	组合体名称	VV	HH	理论α值
平板-平板(垂直)	1.0000	0.9988	1	直劈-圆柱(垂直)	0.0383	0.0028	0
圆柱-平板(垂直)	0.5011	0.4949	1/2	直劈-圆柱(平行)	2.90e-4	–4.90e-5	0
圆柱-平板(平行)	0.5015	0.5753	1/2	直劈-球	–0.5040	–0.6427	–1/2
球-平板	0.0385	–0.1048	0	曲劈-平板	–0.4957	–0.4904	–1/2
圆柱-圆柱(垂直)	0.5499	0.5034	1/2	曲劈-圆柱	–0.3976	–0.4226	–1/2
圆柱-圆柱(平行)	0.5438	0.3807	1/2	曲劈-球	–0.5909	–0.6195	–1/2
球-圆柱	–0.0616	0.0831	0	直劈-直劈(垂直)	–0.5000	–0.5000	–1/2
球-球	–0.0591	–0.0591	0	直劈-直劈(平行)	–0.5000	–0.5000	–1/2
直劈-平板(垂直)	1.68e-4	–0.0053	0	曲劈-直劈	–0.9428	–1.1978	–1
直劈-平板(平行)	–4.05e-7	–2.34e-6	0	曲劈-曲劈	–0.9428	–1.0302	–1

下载: 导出CSV

表 5 基于2种组合体仿真数据的三次散射机理形成散射中心频率依赖因子估计与理论值对比

Table 5. Comparison of theoretical frequency-dependent factor values by proposed formula and estimated ones by simulation data for scattering centers induced by triple scattering from 2 combination objects

组合体名称	几何示意图	估计α值	理论α值
垂直三面角结构		0.9934	1
双顶帽结构		0.5097	1/2

下载: 导出CSV

表 6 基于7种组合体暗室测量数据的二次散射机理形成散射中心的频率依赖因子估计与理论值对比

Table 6. Comparison of theoretical frequency-dependent factor values by proposed formula and estimated ones by meas urementdata in microwave anechoic chamber for scattering centers induced by double scattering from 7 combination objects

机理类型	组合体名称	几何示意图	估计α值	理论α值
边缘绕射-镜面反射	四面体-圆盘(垂直)		0.1589	0
边缘绕射-边缘绕射	四面体-四面体(垂直)		–0.4829	–1/2
边缘绕射-镜面反射	四面体-圆柱(平行)		–0.0485	0
镜面反射-镜面反射	平板-圆柱(平行)		0.5345	1/2
镜面反射-镜面反射	直二面角(垂直)		1.0314	1
镜面反射-镜面反射	圆柱-圆盘(垂直)		0.5048	1/2
镜面反射-镜面反射	双圆柱(垂直)		0.5115	1/2

下载: 导出CSV

参考文献(37)

[1]	KELLER J B. Geometrical theory of diffraction[J]. Journal of the Optical Society of America, 1962, 52(2): 116–130. doi: 10.1364/JOSA.52.000116
[2]	黄培康, 殷红成, 许小剑. 雷达目标特性[M]. 北京: 电子工业出版社, 2005: 230–237. HUANG Peikang, YIN Hongcheng, and XU Xiaojian. Radar Target Signature[M]. Beijing: Publishing House of Electronics Industry, 2005: 230–237.
[3]	HURST M P and MITTRA R. Scattering center analysis via Prony’s method[J]. IEEE Transactions on Antennas and Propagation, 1987, 35(8): 986–988. doi: 10.1109/TAP.1987.1144210
[4]	CARRIÈRE R and MOSES R L. High-resolution parametric modeling of canonical radar scatterers with application to radar target identification[C]. The IEEE 1991 International Conference on Systems Engineering, Dayton, USA, 1991. doi: 10.1109/ICSYSE.1991.161070.
[5]	POTTER L C, CHIANG D M, CARRIÈRE R, et al. A GTD-based parametric model for radar scattering[J]. IEEE Transactions on Antennas and Propagation, 1995, 43(10): 1058–1067. doi: 10.1109/8.467641
[6]	代大海, 王雪松, 肖顺平. 基于相干极化GTD模型的散射中心提取新方法[J]. 系统工程与电子技术, 2007, 29(7): 1057–1061. doi: 10.3321/j.issn:1001-506X.2007.07.010 DAI Dahai, WANG Xuesong, and XIAO Shunping. Novel method for scattering center extraction based on coherent polarization GTD model[J]. Systems Engineering and Electronics, 2007, 29(7): 1057–1061. doi: 10.3321/j.issn:1001-506X.2007.07.010
[7]	FULLER D F. Phase history decomposition for efficient scatterer classification in SAR imagery[D]. [Ph. D. dissertation], Air Force Institute of Technology, 2011: 67–150.
[8]	DUAN Jia, ZHANG Lei, XING Mengdao, et al. Polarimetric target decomposition based on attributed scattering center model for synthetic aperture radar targets[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(12): 2095–2099. doi: 10.1109/LGRS.2014.2320053
[9]	HALMAN J and BURKHOLDER R J. Sparse expansions using physical and polynomial basis functions for compressed sensing of frequency domain EM scattering[J]. IEEE Antennas and Wireless Propagation Letters, 2015, 14: 1048–1051. doi: 10.1109/LAWP.2015.2394474
[10]	GERRY M J, POTTER L C, GUPTA I J, et al. A parametric model for synthetic aperture radar measurements[J]. IEEE Transactions on Antennas and Propagation, 1999, 47(7): 1179–1188. doi: 10.1109/8.785750
[11]	AI Fazhi, ZHOU Jianxiong, HU Lei, et al. The parametric model of non-uniformly distributed scattering centers[C]. The IET International Conference on Radar Systems (Radar 2012), Glasgow, UK, 2012. doi: 10.1049/cp.2012.1712.
[12]	冯艾茜, 郭琨毅, 盛新庆. 无翼平底弹头的属性散射中心模型改进与参数估计[J]. 北京理工大学学报, 2015, 35(9): 961–967. doi: 10.15918/j.tbit1001-0645.2015.09.016 FENG Aixi, GUO Kunyi, and SHENG Xinqing. Modification and parameter estimation of attributed scattering center model for flat-based warhead without wings[J]. Transactions of Beijing Institute of Technology, 2015, 35(9): 961–967. doi: 10.15918/j.tbit1001-0645.2015.09.016
[13]	LI Zenghui, JIN Kan, XU Bin, et al. An improved attributed scattering model optimized by incremental sparse Bayesian learning[J]. IEEE Transactions on Geoscience and Remote Sensing, 2016, 54(5): 2973–2987. doi: 10.1109/TGRS.2015.2509539
[14]	TSENG N Y and BURNSIDE W D. A very efficient RCS data compression and reconstruction technique[R]. NASA-CR-191378, 1992.
[15]	王菁. 光学区雷达目标散射中心提取及其应用研究[D]. [博士论文], 南京航空航天大学, 2010: 3–77. doi: 10.7666/d.d167227. WANG Jing. A study on radar optical region target scattering center extraction and its applications[D]. [Ph. D. dissertation], Nanjing University of Aeronautics and Astronautics, 2010: 3–77. doi: 10.7666/d.d167227.
[16]	BHALLA R and LING Hao. A fast algorithm for signature prediction and image formation using the shooting and bouncing ray technique[J]. IEEE Transactions on Antennas and Propagation, 1995, 43(7): 727–731. doi: 10.1109/8.391147
[17]	MENSA D L. High Resolution Radar Imaging[M]. Dedham, MA: Artech House, 1981.
[18]	RAYNAL A M. Feature-based exploitation of multidimensional radar signatures[D]. [PHD dissertation]. The University of Texas at Austin, 2008.
[19]	ZHOU Jianxiong, SHI Zhiguang, CHENG Xiao, et al. Automatic target recognition of SAR images based on global scattering center model[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(10): 3713–3729. doi: 10.1109/tgrs.2011.2162526
[20]	CHIANG H C and MOSES R L. ATR performance prediction using attributed scattering features[C]. SPIE 3721, Algorithms for Synthetic Aperture Radar Imagery VI, Orlando, United States, 1999: 785–796.
[21]	邢笑宇. 耦合散射中心模型频率依赖关系及其估计[D]. [硕士论文], 中国航天第二研究院, 2014: 18–28. XING Xiaoyu. EM scattering modeling and application research of complex targets in the typical environment[D]. [Master dissertation], The Second Academy of China Aerospace, 2014: 18–28.
[22]	YAN Hua, LI Sheng, LI Huanmin, et al. Monostatic GTD model for double scattering due to specular reflections or edge diffractions[C]. 2018 IEEE International Conference on Computational Electromagnetics, Chengdu, China, 2018. doi: 10.1109/COMPEM.2018.8496539.
[23]	HE Yang, HE Siyuan, ZHANG Yunhua, et al. A forward approach to establish parametric scattering center models for known complex radar targets applied to SAR ATR[J]. IEEE Transactions on Antennas and Propagation, 2014, 62(12): 6192–6205. doi: 10.1109/TAP.2014.2360700
[24]	LI Qifeng, GUO Kunyi, SHENG Xinqing, et al. High precise scattering centers models for cone-shaped targets based on induced currents[J]. International Journal of Antennas and Propagation, 2017, 2017: 7482895. doi: 10.1155/2017/7482895
[25]	张磊, 何思远, 朱国强, 等. 雷达目标三维散射中心位置正向推导和分析[J]. 电子与信息学报, 2018, 40(12): 2854–2860. doi: 10.11999/JEIT180115 ZHANG Lei, HE Siyuan, ZHU Guoqiang, et al. Forward derivation and analysis for 3-D scattering center position of radar target[J]. Journal of Electronics &Information Technology, 2018, 40(12): 2854–2860. doi: 10.11999/JEIT180115
[26]	LIU Jin, HE Siyuan, ZHANG Lei, et al. An automatic and forward method to establish 3-D parametric scattering center models of complex targets for target recognition[J]. IEEE Transactions on Geoscience and Remote Sensing, 2020, 58(12): 8701–8716. doi: 10.1109/TGRS.2020.2989856
[27]	LEE S W. Electromagnetic reflection from a conducting surface: Geometrical optics solution[J]. IEEE Transactions on Antennas and Propagation, 1975, 23(2): 184–191. doi: 10.1109/TAP.1975.1141040
[28]	汪茂光. 几何绕射理论[M]. 2版. 西安: 西安电子科技大学出版社, 1994. WANG Maoguang. Geometrical Diffraction Theory[M]. 2nd ed. Xi’an: Xidian University Press, 1994.
[29]	KOUYOUMJIAN R G and PATHAK P H. A uniform geometrical theory of diffraction for an edge in a perfectly conducting surface[J]. Proceedings of the IEEE, 1974, 62(11): 1448–1461. doi: 10.1109/PROC.1974.9651
[30]	PEREZ J and CATEDRA M F. Application of physical optics to the RCS computation of bodies modeled with NURBS surfaces[J]. IEEE Transactions on Antennas and Propagation, 1994, 42(10): 1404–1411. doi: 10.1109/8.320747
[31]	MICHAELI A. Equivalent edge currents for arbitrary aspects of observation[J]. IEEE Transactions on Antennas and Propagation, 1984, 32(3): 252–258. doi: 10.1109/TAP.1984.1143303
[32]	LING H, CHOU R C, and LEE S W. Shooting and bouncing rays: Calculating the RCS of an arbitrarily shaped cavity[J]. IEEE Transactions on Antennas and Propagation, 1989, 37(2): 194–205. doi: 10.1109/8.18706
[33]	CARLUCCIO G, ALBANI M, and PATHAK P H. Uniform asymptotic evaluation of surface integrals with polygonal integration domains in terms of UTD transition functions[J]. IEEE Transactions on Antennas and Propagation, 2010, 58(4): 1155–1163. doi: 10.1109/TAP.2010.2041171
[34]	殷红成, 朱国庆, 董纯柱, 等. 基于自适应射线管分裂的多次反射计算方法[J]. 系统工程与电子技术, 2013, 35(4): 700–706. doi: 10.3969/j.issn.1001-506X.2013.04.04 YIN Hongcheng, ZHU Guoqing, DONG Chunzhu, et al. Efficient multi-reflection computational method based on adaptive ray tube splitting[J]. Systems Engineering and Electronics, 2013, 35(4): 700–706. doi: 10.3969/j.issn.1001-506X.2013.04.04
[35]	侯兆国, 王超, 殷红成. 电大复杂目标电磁散射计算的特征基函数方法[J]. 制导与引信, 2009, 30(2): 24–29. doi: 10.3969/j.issn.1671-0576.2009.02.006 HOU Zhaoguo, WANG Chao, and YIN Hongcheng. Characteristic basis function method for electromagnetic scattering computation of electrically large complex target[J]. Guidance &Fuze, 2009, 30(2): 24–29. doi: 10.3969/j.issn.1671-0576.2009.02.006
[36]	FULLER D F and SAVILLE M A. The spectrum parted linked image test (SPLIT) algorithm for estimating the frequency dependence of scattering center amplitudes[C]. SPIE 7337, Algorithms for Synthetic Aperture Radar Imagery XVI, Orlando, United States, 2009. doi: 10.1117/12.819329.
[37]	QUINQUIS A, DEMETER S, and RADOI E. Enhancing the resolution of the radar target range profiles using a class of subspace eigenanalysis-based techniques[J]. Digital Signal Processing, 2001, 11(4): 288–303. doi: 10.1006/dspr.2001.0394.