基于稀疏孔径干涉ISAR技术的复杂运动舰船目标三维坐标恢复方法

王勇; 陈雪飞

doi:10.12000/JR18019

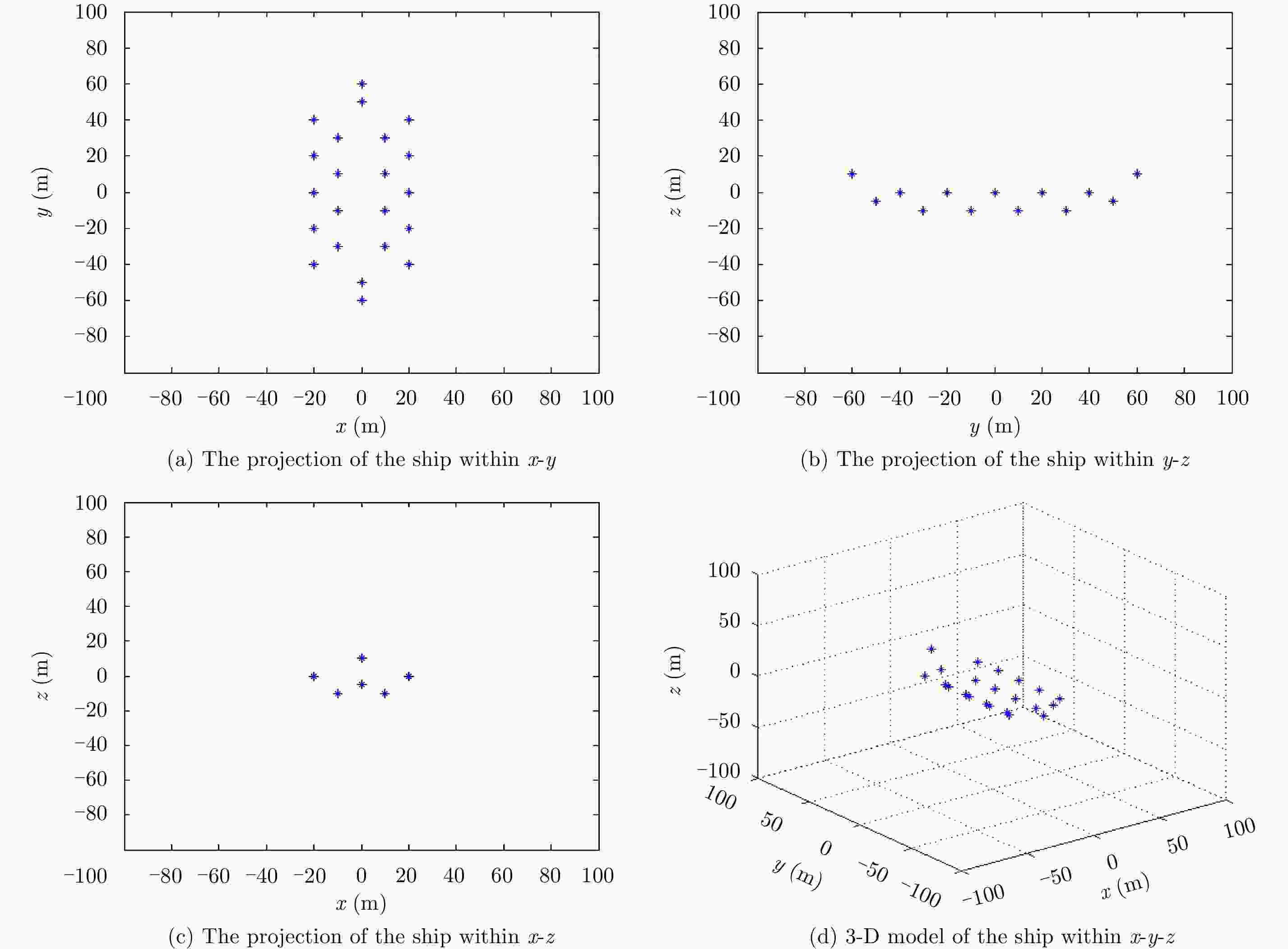
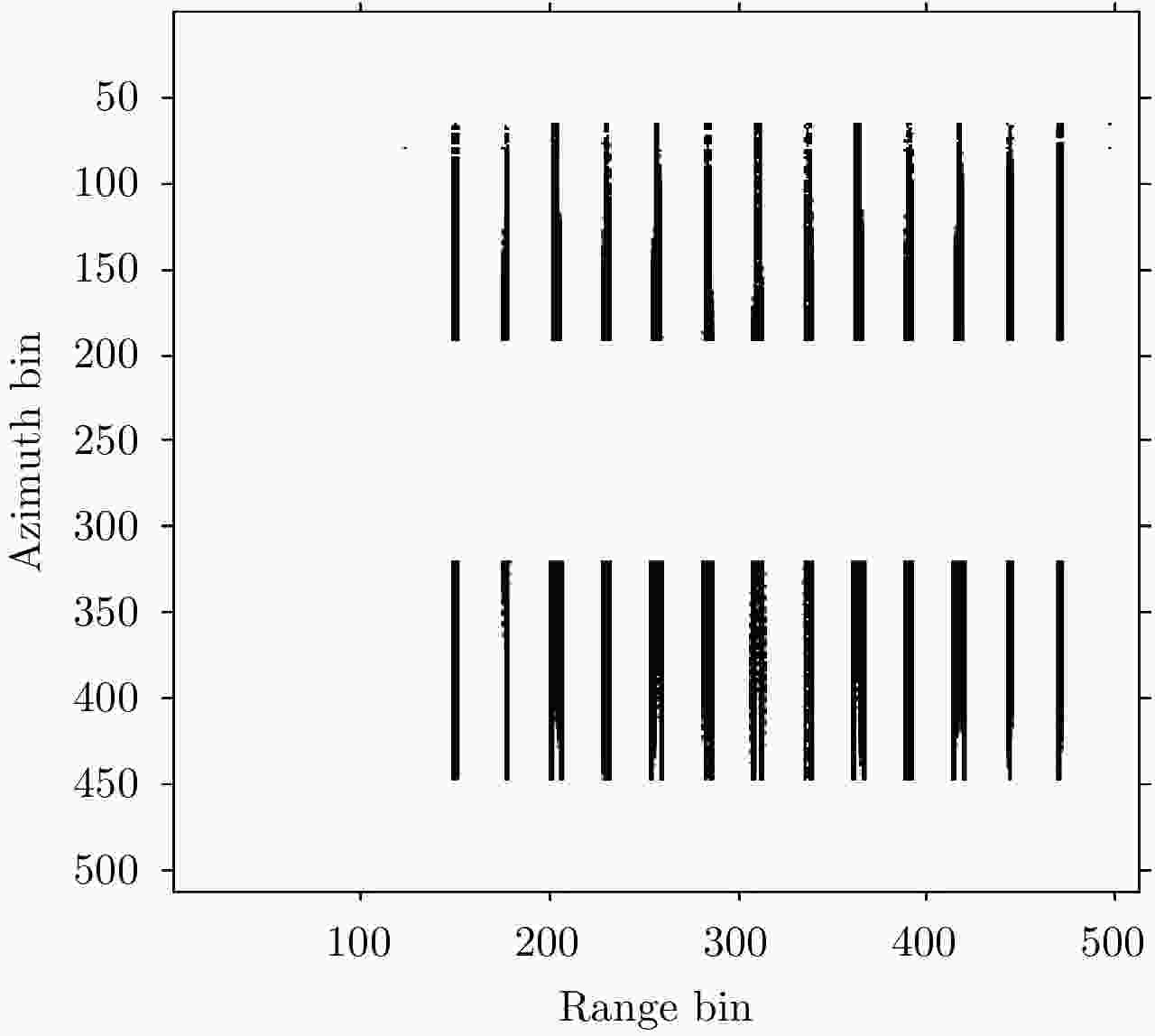
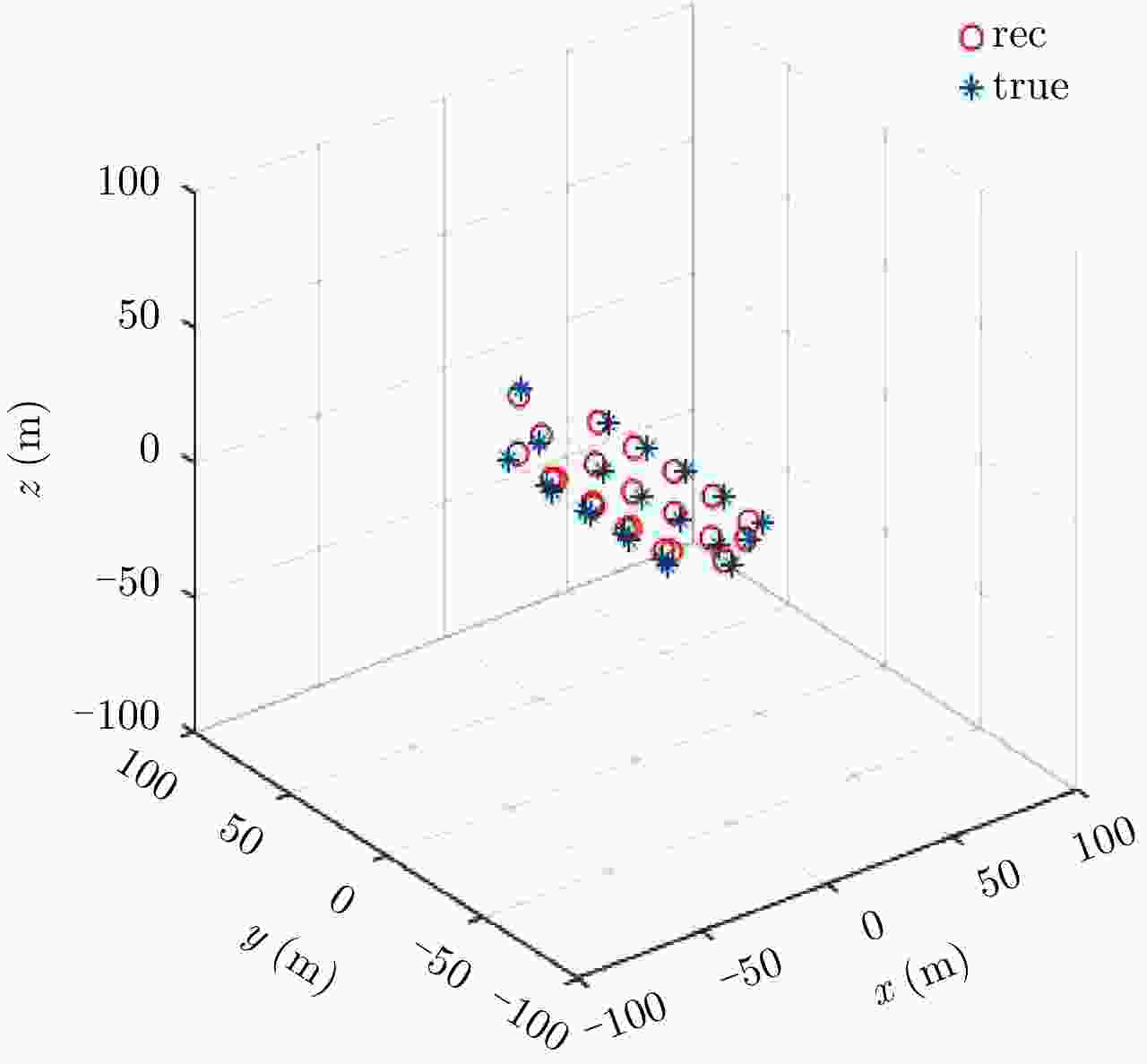
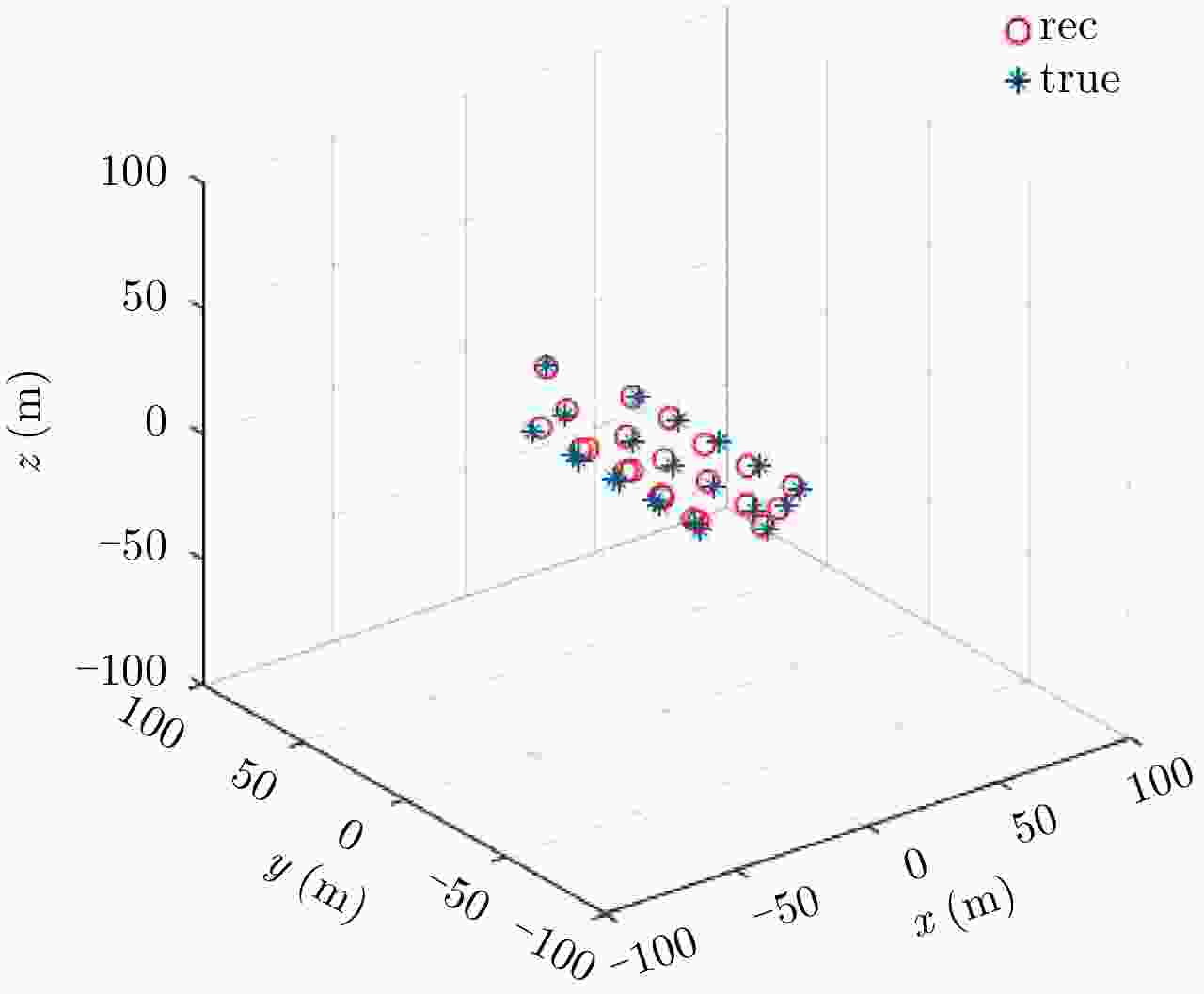
Three-dimensional Geometry Reconstruction of Ship Targets with Complex Motion for Interferometric ISAR with Sparse Aperture

doi: 10.12000/JR18019

Wang Yong^,,
Chen Xuefei

Research Institute of Electronic Engineering Technology, Harbin Institute of Technology, Harbin 150001, China

Funds: The National Natural Science Foundation of China (61622107, 61471149), The Fundamental Research Funds for the Central Universities.

More Information

Author Bio:
Wang Yong (SM’16) was born in 1979. He received the B. S. degree and M. S. degree from Harbin Institute of Technology (HIT), Harbin, China, in 2002 and 2004, respectively, both in electronic engineering. He received the Ph. D. degree in information and communication engineering from HIT in 2008. He is currently a professor with the institute of electronic engineering technology in HIT. His main research interests are in the fields of time frequency analysis of nonstationary signal, radar signal processing, and their application in synthetic aperture radar (SAR) imaging. Dr. Yong Wang has published more than 60 papers, and most of them appeared in the journals of IEEE Trans. On GRS, IET Signal Processing, Signal Processing, etc. He received the Program for New Century Excellent Talents in University of Ministry of Education of China in 2012, and the Excellent Doctor’s Degree nomination Award in China in 2010. E-mail: wangyong6012@hit.edu.cn

Chen Xuefei received the B. S. degree from Harbin Institute of Technology (HIT), Harbin, China, in 2017. She is now pursuing the M. E. degree in Harbin Institute of Technology. Her current research interests include the field of InISAR imaging, time-frequency signal analysis and ISAR imaging of the target with sparse aperture

Corresponding author: Wang Yong wangyong6012@hit.edu.cn

摘要

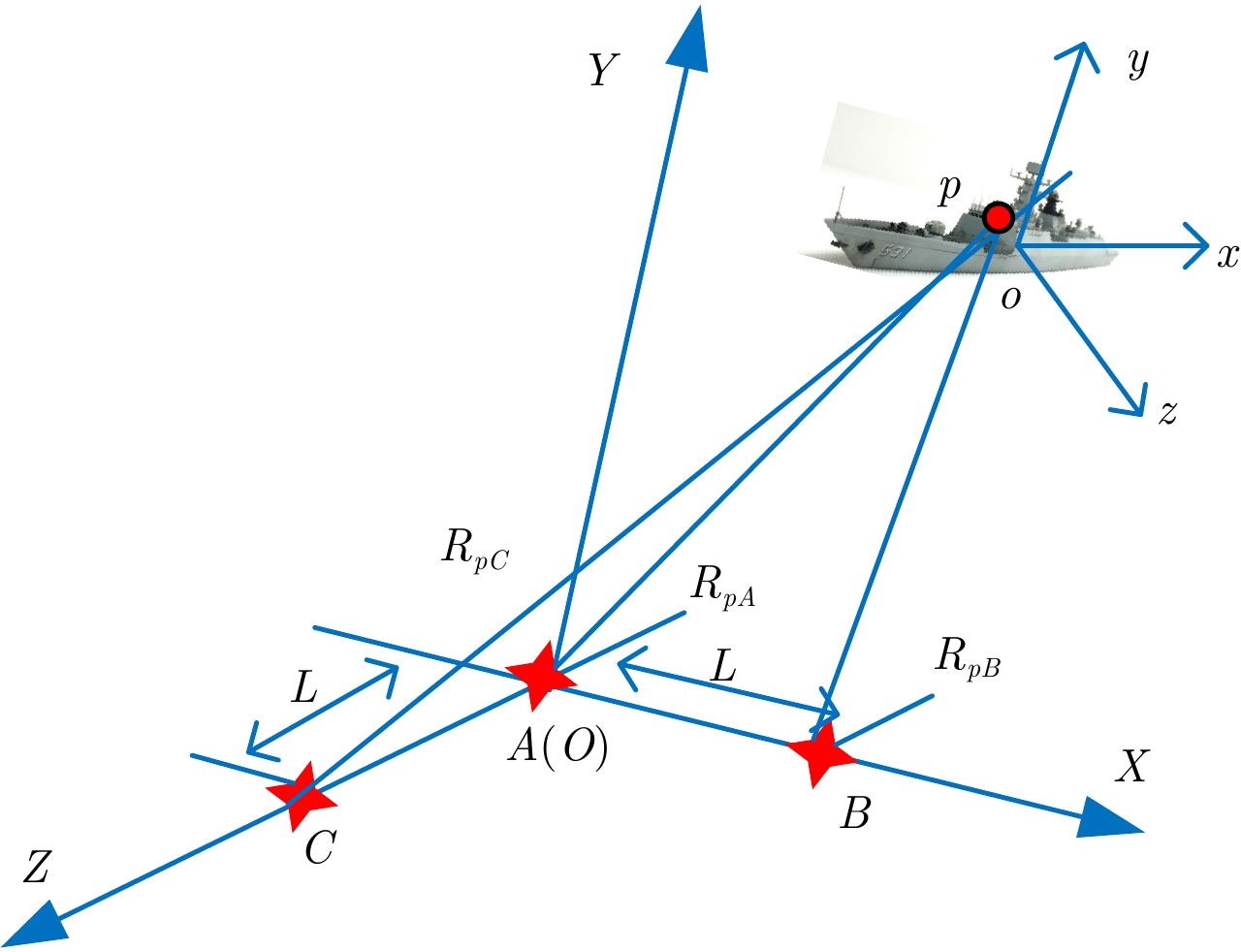
摘要: 基于正交双基线的3维干涉逆合成孔径雷达(ISAR)成像技术可获得目标的3维坐标信息，这对目标的分类与识别是非常有利的。然而，实际情况下回波数据一般都是稀疏的，这对传统的干涉成像技术带来一定的挑战。该文提出一种稀疏孔径情况下的舰船目标3维干涉成像算法，并采用最小熵方法实现回波数据的运动补偿与图像配准，同时基于梯度算子实现对稀疏数据的精确恢复。通过对方位向数据进行参数估计与压缩处理，可获得目标的2维ISAR成像结果，进而基于干涉技术实现对复杂运动舰船目标的3维成像。仿真数据验证了文中方法的有效性。
- 3维干涉ISAR成像 /
- 稀疏孔径 /
- 梯度算法 /
- 参数估计
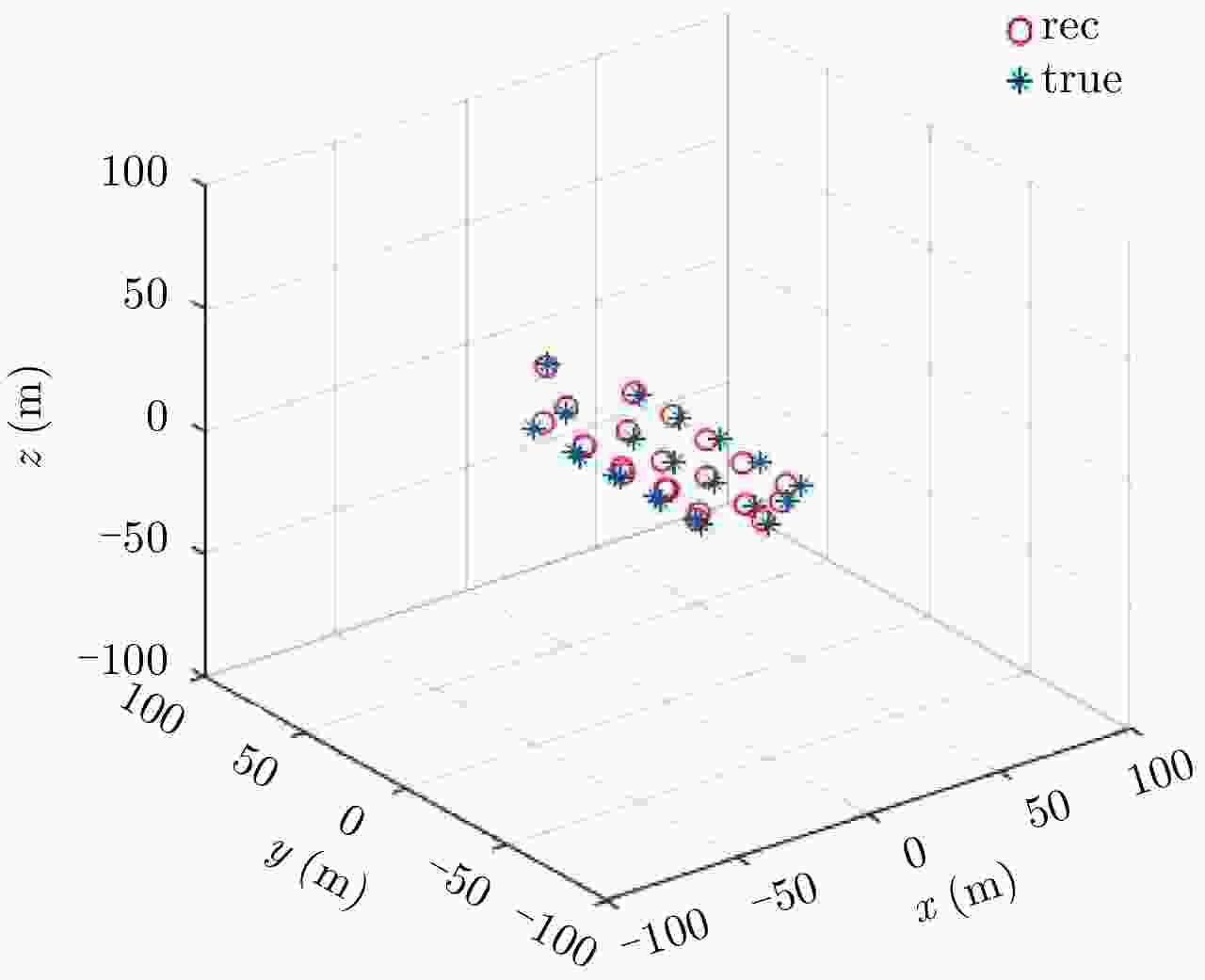
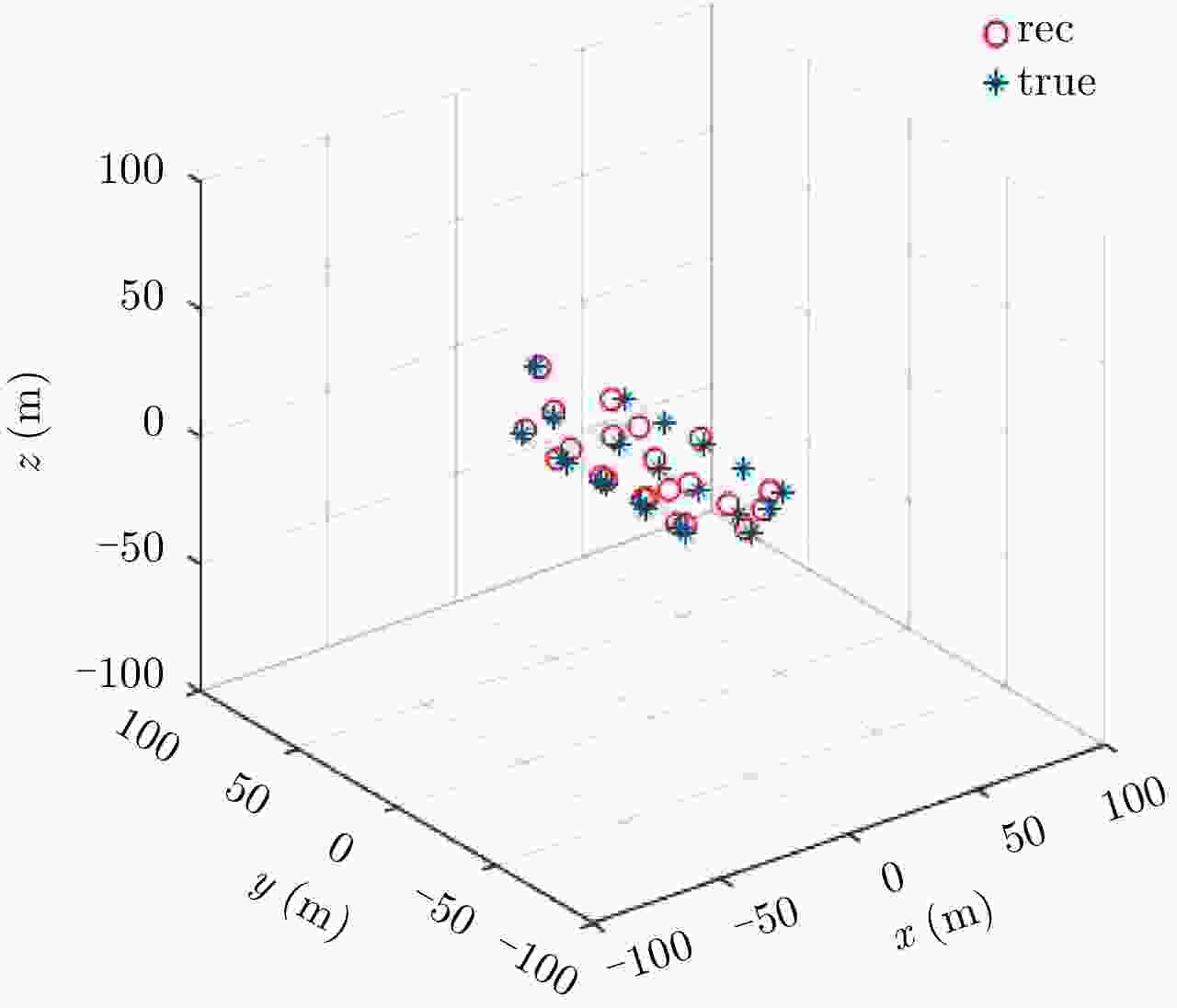
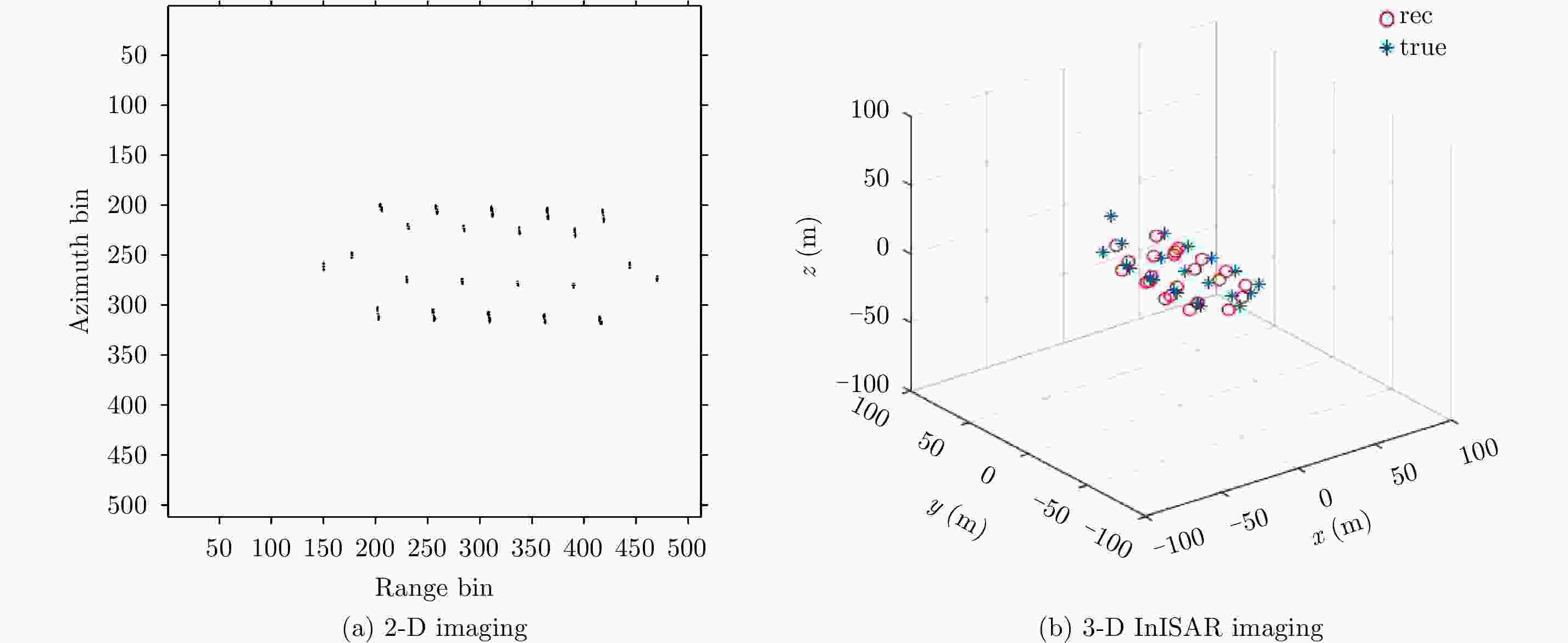
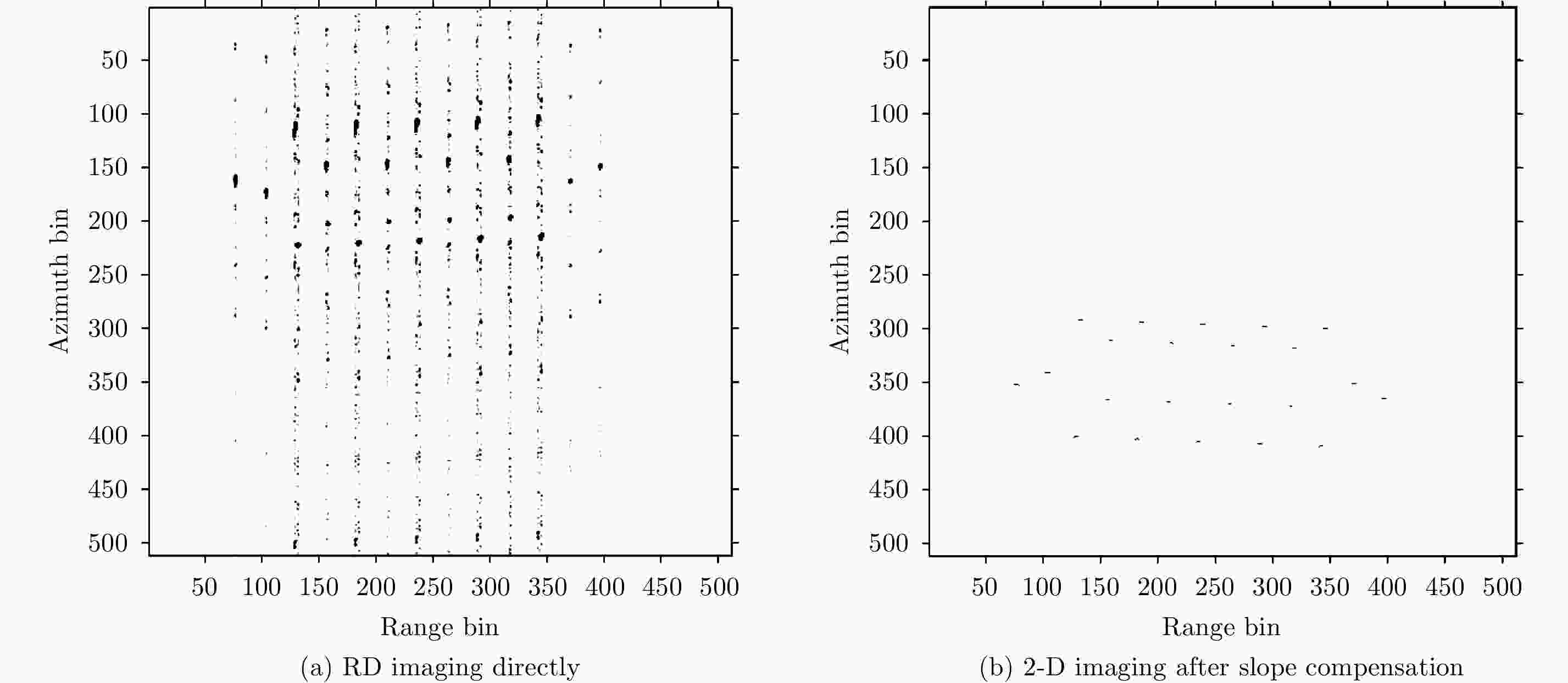
Abstract: Three-Dimensional (3-D) Interferometric Inverse Synthetic Aperture Radar (InISAR) imaging system based on the orthogonal double baseline can achieve the 3-D geometric reconstruction of a target effectively, which is extremely helpful in target classification and identification. However, only sparse aperture measurements are available in the actual imaging process, which might pose some challenges to the traditional InISAR imaging algorithms. In this study, a new method of 3-D InISAR imaging of a ship with sparse aperture is presented. Minimum entropy algorithms are adopted to realize motion compensation and image coregistration of the sparse echoes. A gradient-based technique is used to achieve highly accurate signal reconstruction for the sparse aperture. A two-Dimensional (2-D) ISAR image was achieved with azimuth compression via the parameters-estimation method, and the 3-D reconstruction of a ship was achieved via the interference approach. The obtained simulation results validate the feasibility of the presented approach.
- Three-Dimensional (3-D) Interferometric Inverse Synthetic Aperture Radar (InISAR) /
- Sparse aperture /
- Gradient-based method /
- Parameter estimation

HTML全文

Figure 1. 3-D InISAR imaging model

下载: 全尺寸图片幻灯片

Figure 2. The 3-D imaging model in XOY plane

下载: 全尺寸图片幻灯片

Figure 3. RMS

下载: 全尺寸图片幻灯片

Figure 4. GMS

下载: 全尺寸图片幻灯片

Figure 5. The ideal model for the ship

下载: 全尺寸图片幻灯片

Figure 6. RMS of the echoes from radar A with 2/4 sparse aperture

下载: 全尺寸图片幻灯片

Figure 7. GMS of the echoes from radar A with 2/4 sparse aperture

下载: 全尺寸图片幻灯片

Figure 9. 2-D ISAR images of radar A in 2/4 sparse aperture (GMS)

下载: 全尺寸图片幻灯片

Figure 10. 3-D reconstruction for the ship in 2/4 sparse aperture data (RMS)

下载: 全尺寸图片幻灯片

Figure 11. 3-D reconstruction for the ship in 1/4 sparse aperture data (RMS)

下载: 全尺寸图片幻灯片

Figure 12. 3-D reconstruction for the ship in 2/4 sparse aperture data (GMS)

下载: 全尺寸图片幻灯片

Figure 13. 3-D reconstruction for the ship in 1/4 sparse aperture data (GMS)

下载: 全尺寸图片幻灯片

Figure 15. The images of the target with 2/4 sparse aperture data (GMS) by using the OMP algorithm

下载: 全尺寸图片幻灯片

Figure 8. 2-D ISAR images of radar A in 2/4 sparse aperture (RMS)

下载: 全尺寸图片幻灯片

Figure 14. The images of the target with 2/4 sparse aperture data (RMS) by using the OMP algorithm

下载: 全尺寸图片幻灯片

Figure 16. Calculation and analysis of ${{\vec R}_{Ap}}\left( {{t_m}} \right)$

下载: 全尺寸图片幻灯片

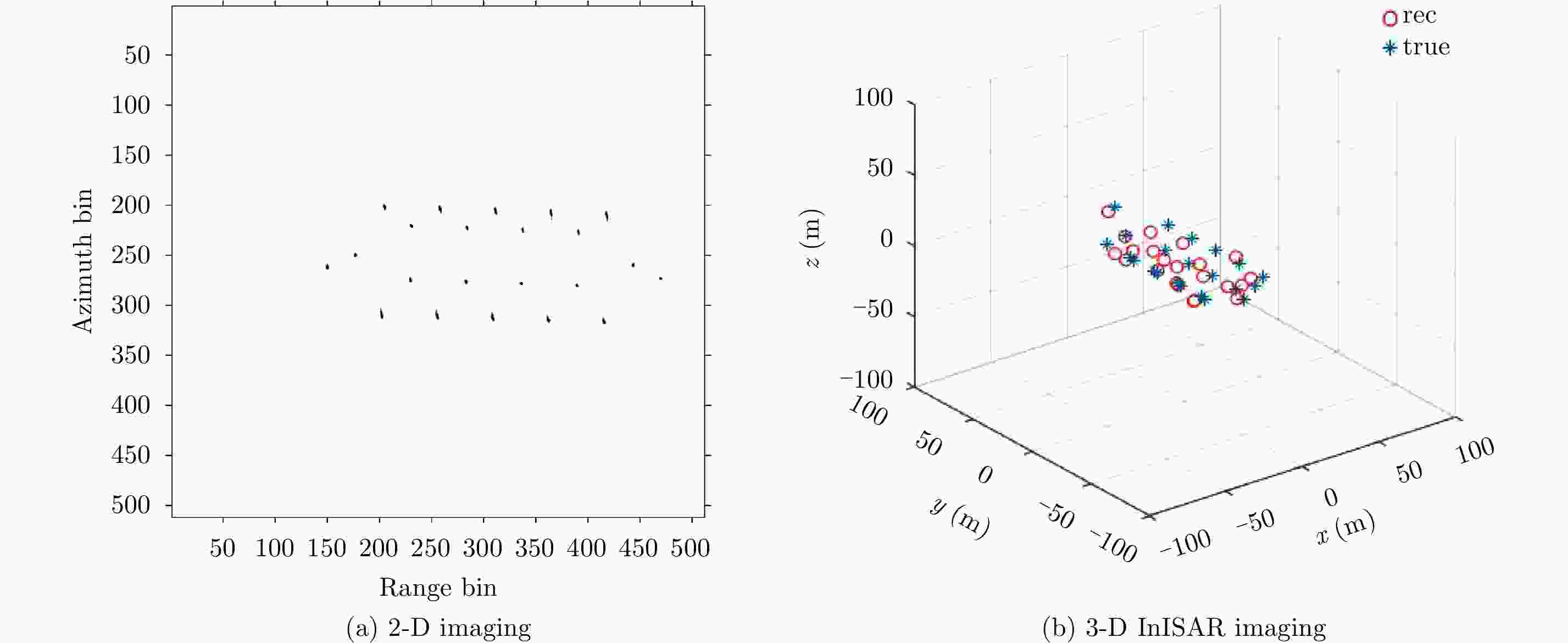
Table 1. Simulation parameters for the ship with complicated movement

Parameter	Value
Carrier frequency	10 GHz
Pulse width	20 us
Imaging time	2 s
Band width	400 MHz
Amplitude of roll	2.3 ${{π}} $/180
Amplitude of pitch	2.5 ${{π}} $/180
Amplitude of yaw	4.8 ${{π}} $/180
Length of the baseline	2 m
Sampling frequency	25.6 MHz
Number of the pulse	512
Pulse repetition frequency	256 Hz
Angular velocity of roll	2 ${{π}} $/12.2
Angular velocity of pitch	2 ${{π}} $/6.7
Angular velocity of yaw	2 ${{π}} $/14.2

下载: 导出CSV

参考文献(32)

[1]	Wang G Y, Xia X G, and Chen V C. Three-dimensional ISAR imaging of maneuvering targets using three receivers[J]. IEEE Transactions on Image Processing, 2001, 10(3): 436–447. DOI: 10.1109/83.908519
[2]	Ma C Z, Yeo T S, Zhang Q, et al. Three-dimensional ISAR imaging based on antenna array[J]. IEEE Transactions on Geoscience and Remote Sensing, 2008, 46(2): 504–515. DOI: 10.1109/TGRS.2007.909946
[3]	Liu L, Zhou F, Bai X R, et al. Joint cross-range scaling and 3D geometry reconstruction of ISAR targets based on factorization method[J]. IEEE Transactions on Image Processing, 2016, 25(4): 1740–1750. DOI: 10.1109/TIP.2016.2526905
[4]	Wang Y and Li X L. Three-dimensional interferometric ISAR imaging for the ship target under the bi-static configuration[J].IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2016, 9(4): 1505–1520. DOI: 10.1109/JSTARS.2015.2513774
[5]	Cao X H, Su F L, Sun H D, et al.. Three-dimensional In-ISAR imaging via the emulated Bistatic radar[C]. Proceedings of the 2nd IEEE Conference on Industrial Electronics and Applications, Harbin, China, 2007: 2826–2830.
[6]	Ma C Z, Yeo T S, Tan H S, et al. Three-dimensional ISAR imaging using a two-dimensional sparse antenna array[J]. IEEE Geoscience and Remote Sensing Letters, 2008, 5(3): 378–382. DOI: 10.1109/LGRS.2008.916071
[7]	Ng B, Tran H T, and An P. Total rotational velocity estimation using 3D interferometrie ISAR with squint geometry[C]. Proceedings of 2016 IEEE Radar Conference, Philadelphia, PA, USA, 2016: 1–6.
[8]	Tran H T, Giusti E, Martorella M, et al.. Estimation of the total rotational velocity of a non-cooperative target using a 3D InISAR system[C]. Proceedings of 2015 IEEE Radar Conference, Arlington, VA, USA, 2015: 937–941.
[9]	Martorella M, Stagliano D, Salvetti F, et al. 3D interferometric ISAR imaging of noncooperative targets[J]. IEEE Transactions on Aerospace and Electronic Systems, 2014, 50(4): 3102–3114. DOI: 10.1109/TAES.2014.130210
[10]	Lv Q, Su T, Zheng J B, et al. Three-dimensional interferometric inverse synthetic aperture radar imaging of maneuvering target based on the joint cross modified Wigner-Ville distribution[J]. Journal of Applied Remote Sensing, 2016, 10(1): 1–17.
[11]	Zhang L, Qiao Z J, Xing M D, et al. High-resolution ISAR imaging by exploiting sparse apertures[J]. IEEE Transactions on Antennas and Propagation, 2012, 60(2): 997–1008. DOI: 10.1109/TAP.2011.2173130
[12]	Zhong Li-hua, Hu Dong-hui, Ding Chi-biao, et al. ISAR sparse aperture imaging algorithm for large size target[J]. Journal of Radars, 2012, 1(3): 292–300. DOI: 10.3724/SP.J.1300.2012.20033
[13]	Pang L N, Zhang S S, Liu C, et al.. CS-based high-resolution ISAR imaging with adaptive sparse basis[C]. Proceedings of 2014 IEEE International Geoscience and Remote Sensing Symposium, Quebec City, QC, Canada, 2014: 362–365.
[14]	Bacci A, Staglianó D, Giusti E, et al.. 3D interferometric ISAR via compressive sensing[C]. Proceedings of 2014 11th European Radar Conference, Rome, Italy, 2014: 233–236.
[15]	Liu Y B, Li N, Wang R, et al. Achieving high-quality three-dimensional InISAR imageries of maneuvering target via super-resolution ISAR imaging by exploiting sparseness[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(4): 828–832. DOI: 10.1109/LGRS.2013.2279402
[16]	Chen Q Q, Xu G, Zhang L, et al. Three-dimensional interferometric inverse synthetic aperture radar imaging with limited pulses by exploiting joint sparsity[J]. IET Radar,Sonar&Navigation, 2015, 9(6): 692–701.
[17]	Xu G, Xing M D, Xia X G, et al. 3D geometry and motion estimations of maneuvering targets for interferometric ISAR with sparse aperture[J]. IEEE Transactions on Image Processing, 2016, 25(5): 2005–2020. DOI: 10.1109/TIP.2016.2535362
[18]	Qiu W, Martorella M, Zhou J X, et al. Three-dimensional inverse synthetic aperture radar imaging based on compressive sensing[J]. IET Radar,Sonar&Navigation, 2014, 9(4): 411–420.
[19]	Zhu D Y, Wang L, Yu Y S, et al. Robust ISAR range alignment via minimizing the entropy of the average range profile[J]. IEEE Geoscience and Remote Sensing Letters, 2009, 6(2): 204–208. DOI: 10.1109/LGRS.2008.2010562
[20]	Zhu D Y, Li Y, Yu X, et al.. Compressed ISAR autofocusing: Experimental results[C]. Proceedings of 2012 IEEE Radar Conference, Atlanta, GA, USA, 2012: 425–430.
[21]	Wang J, Liu X, and Zhou Z. Minimum-entropy phase adjustment for ISAR[J]. IEE Proceedings-Radar,Sonar and Navigation, 2004, 151(4): 203–209. DOI: 10.1049/ip-rsn:20040692
[22]	Wu W Z, Hu P J, Xu S Y, et al. Image registration for InISAR based on joint translational motion compensation[J]. IET Radar,Sonar&Navigation, 2017, 11(10): 1597–1603.
[23]	Zhu F, Zhang Q, Yan J B, et al.. Compressed sensing in ISAR imaging with sparse sub-aperture[C]. Proceedings of 2011 IEEE CIE International Conference on Radar, Chengdu, China, 2011, 2: 1463–1466.
[24]	Stanković L and Daković M. On a gradient-based algorithm for sparse signal reconstruction in the signal/measurements domain[J]. Mathematical Problems in Engineering, 2016, 2016: 6212674.
[25]	Wang Y. Radar imaging of non-uniformly rotating targets via a novel approach for multi-component AM-FM signal parameter estimation[J]. Sensors, 2015, 15(3): 6905–6923. DOI: 10.3390/s150306905
[26]	Fornaro G, Franceschetti G, and Lanari R. Interferometric SAR phase unwrapping using Green’s formulation[J].IEEE Transactions on Geoscience and Remote Sensing, 1996, 34(3): 720–727. DOI: 10.1109/36.499751
[27]	Li Fang-fang, Zhan Yi, Hu Dong-hui, et al. A fast method for InSAR phase unwrapping based on quality guide[J]. Journal of Radars, 2012, 1(2): 196–202. DOI: 10.3724/SP.J.1300.2012.20023
[28]	Li N, Wang L, and Zhu D Y. Optimal ISAR imaging time selection of ship targets using real data[C]. Proceedings of IET International Radar Conference 2013, Xi’an, China, 2013: 1–4.
[29]	Wang Y and Jiang Y C. ISAR imaging of a ship target using product high-order matched-phase transform[J]. IEEE Geoscience and Remote Sensing Letters, 2009, 6(4): 658–661. DOI: 10.1109/LGRS.2009.2013876
[30]	Wang Y and Jiang Y C. New approach for ISAR imaging of ship target with 3D rotation[J]. Multidimensional Systems and Signal Processing, 2010, 21(4): 301–318. DOI: 10.1007/s11045-010-0111-6
[31]	Li Y N, Fu Y W, Li X, et al.. An ISAR imaging method for multiple moving targets based on fractional Fourier transformation[C]. Proceedings of 2009 IEEE Radar Conference, Pasadena, CA, USA, 2009: 1–6.
[32]	Yamamoto K, Iwamoto M, and Kirimoto T. A new algorithm to calculate the reference image of ship targets for ATR using ISAR[C]. Proceedings of MTS/IEEE Conference and Exhibition OCEANS 2001, Honolulu, HI, USA, 2001, 4: 2601–2607.