Research on Resolution of Terahertz Coded-aperture Imaging
-
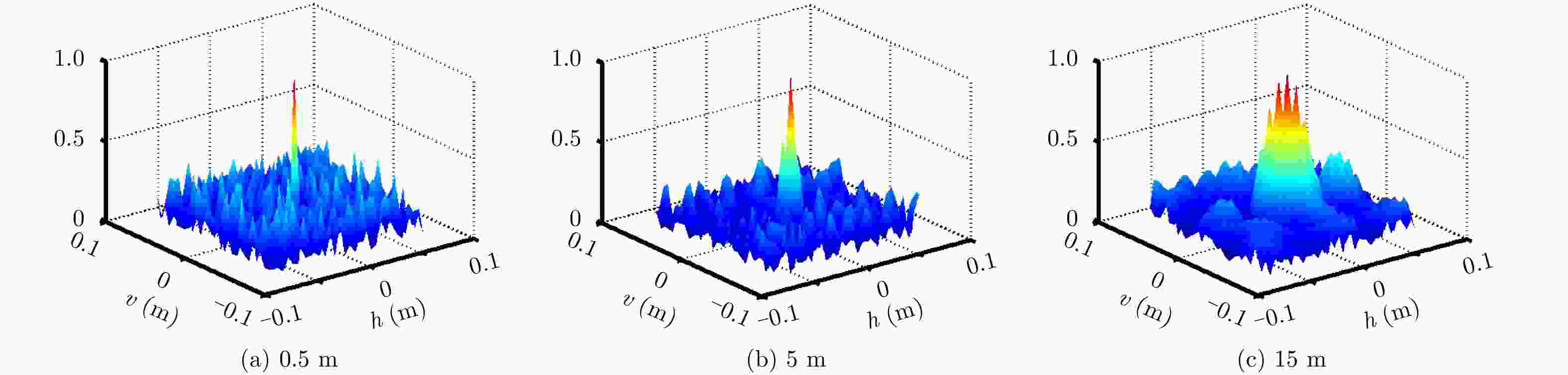
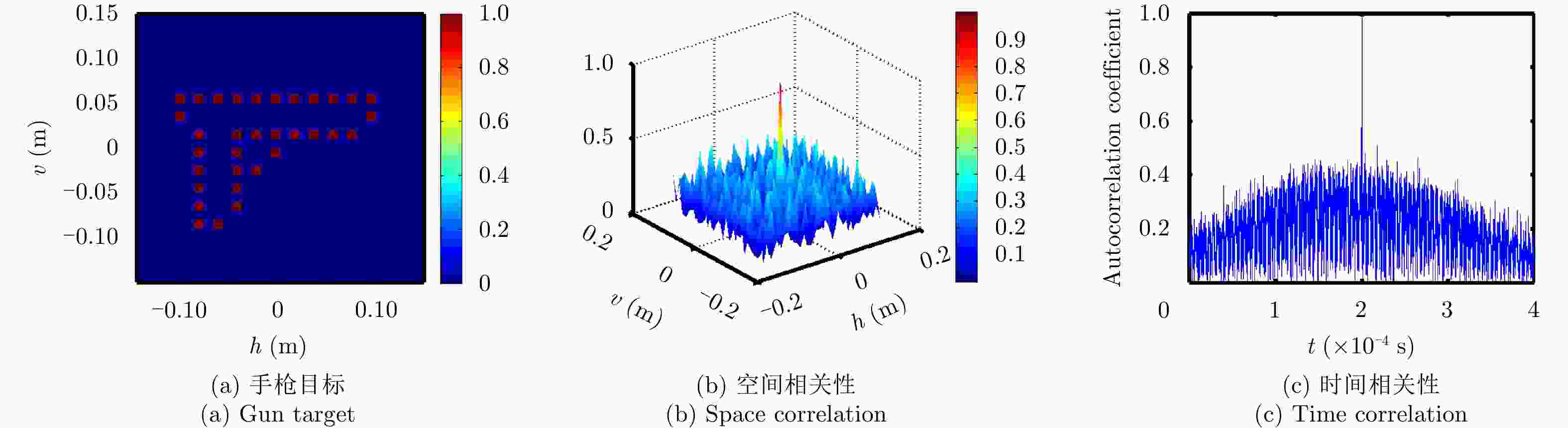
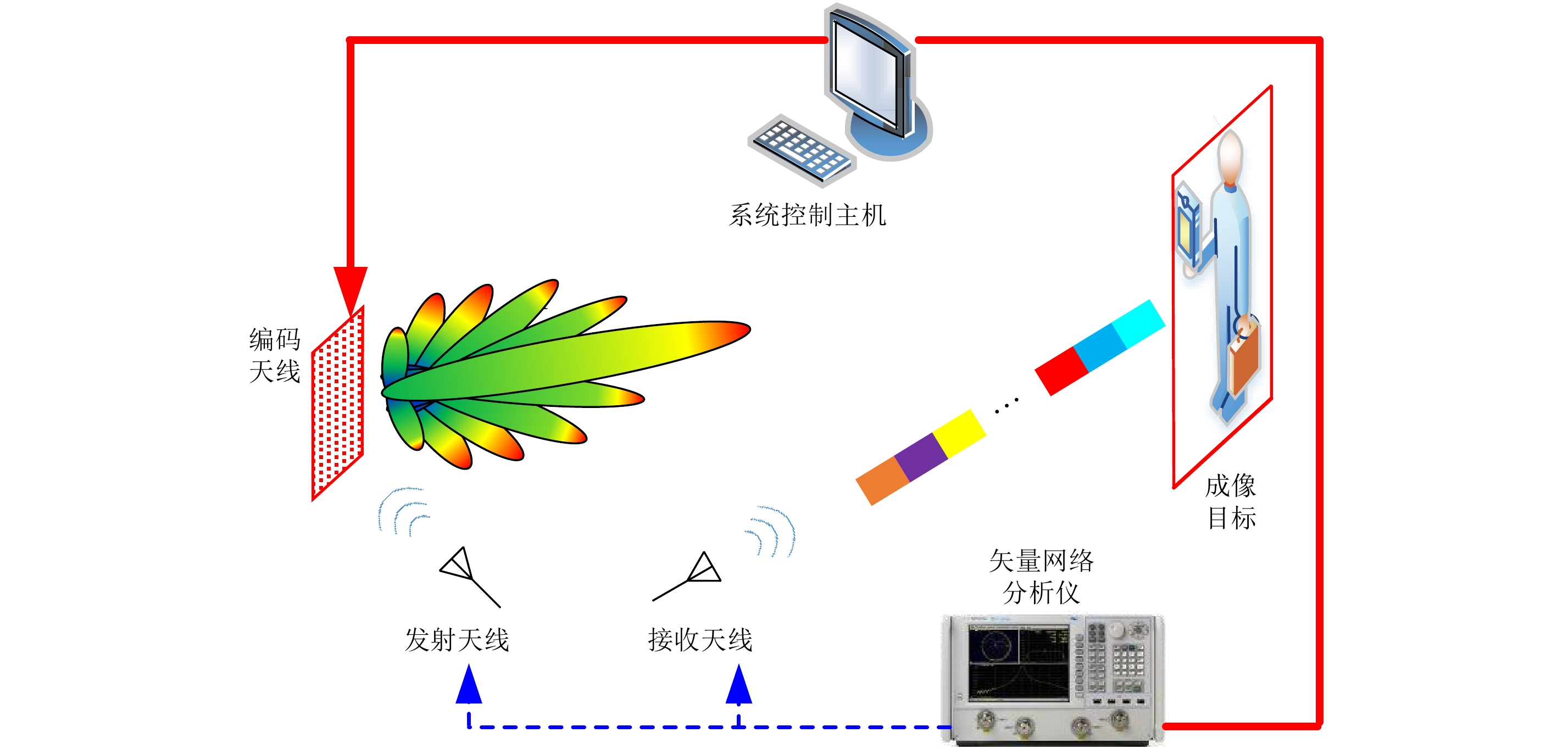
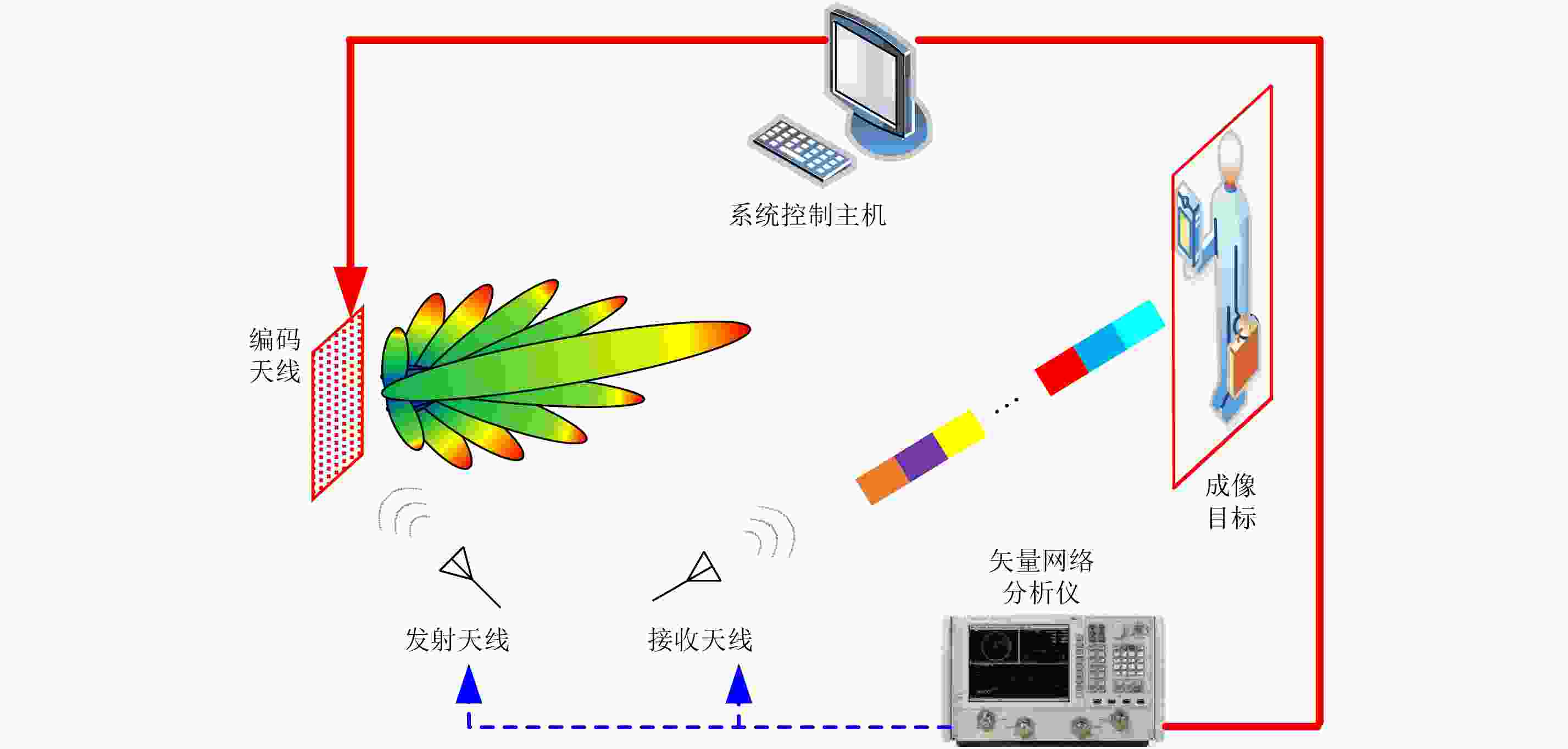
摘要: 太赫兹孔径编码成像综合了光学孔径编码成像和微波关联成像的基本原理,通过孔径编码天线改变目标区域太赫兹波空间幅相分布来实现高分辨、高帧率、前视凝视成像。基于孔径编码天线,该文设计了雷达成像系统和准光扫描光路,可同时实现系统孔径编码和波束扫描功能,理论推导并仿真分析了其成像质量影响因素,并在此基础上比较了不同算法对孔径编码成像分辨性能的影响,证明了稀疏重构类算法对孔径编码成像的优势,最后对比了孔径编码成像和同尺寸阵列实孔径成像的结果,论证出孔径编码成像系统具有高分辨,易于小型化,成本较低等优点。该成像方式可广泛应用于战场侦查、安检反恐和末制导等领域。Abstract: Terahertz coded-aperture imaging follows the basic principles of optical coded-aperture imaging and microwave coincidence imaging and is a novel imaging technique. Herein, the wave spatial distribution or illumination pattern is usually obtained by a sub-reflector antenna. Terahertz coded-aperture imaging has some significant advantages such as a high frame rate, high resolution, and ability of forward-looking and staring imaging. To achieve simultaneous functions of aperture coding and beam scanning, we designed a terahertz coded-aperture imaging system that utilizes digital sub-reflector antenna and quasi-optical techniques. Based on this system, we deduce and simulate the influencing factors on its resolution. Then, different algorithms are applied to the imaging model in order to verify the superiority of sparse reconstruction for coded-aperture imaging. Finally, we compare the imaging results of our imaging system and that of a traditional real aperture imaging structure for the same simulation parameters. The results prove that our imaging system performs better with high resolution, small volume, and low cost. This new imaging technique can be applied to areas such as battlefield reconnaissance, security checks, anti-terrorism, and terminal guidance.
-
Key words:
- Terahertz /
- Coded-aperture /
- Staring /
- Forward-looking
-
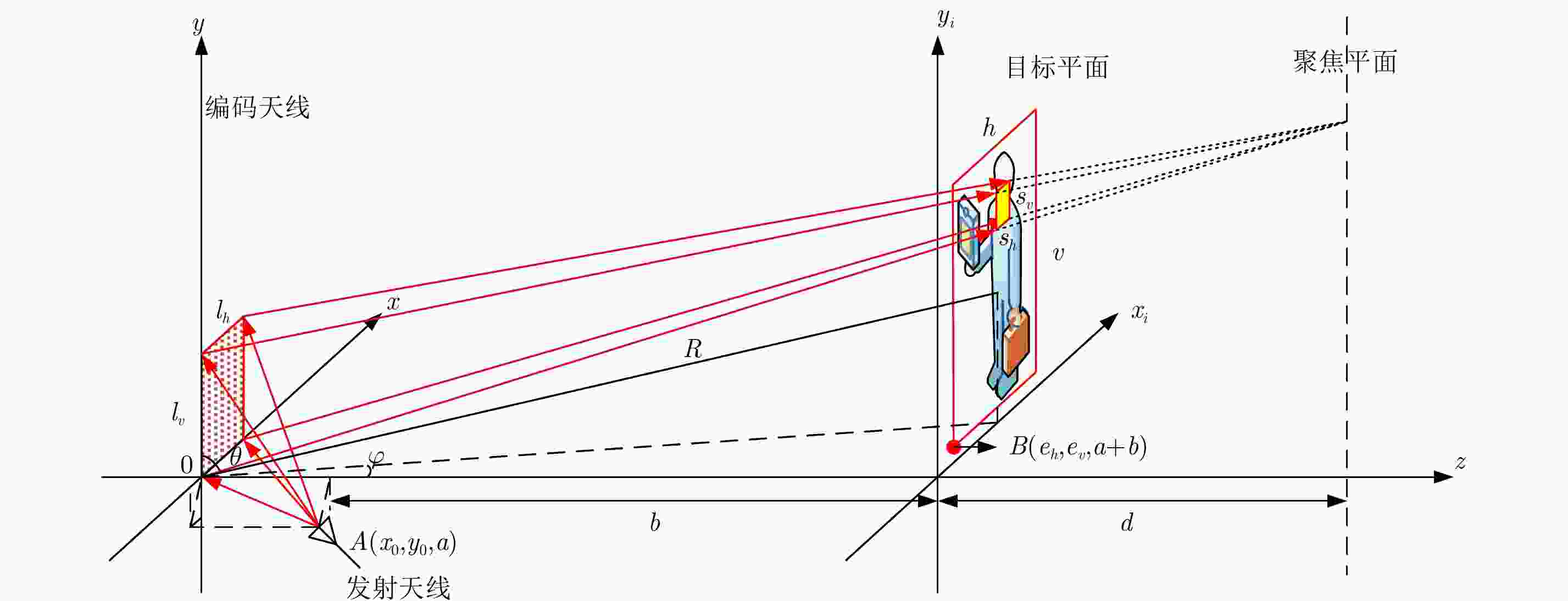
表 1 成像基本参数
Table 1. The basic imaging parameters
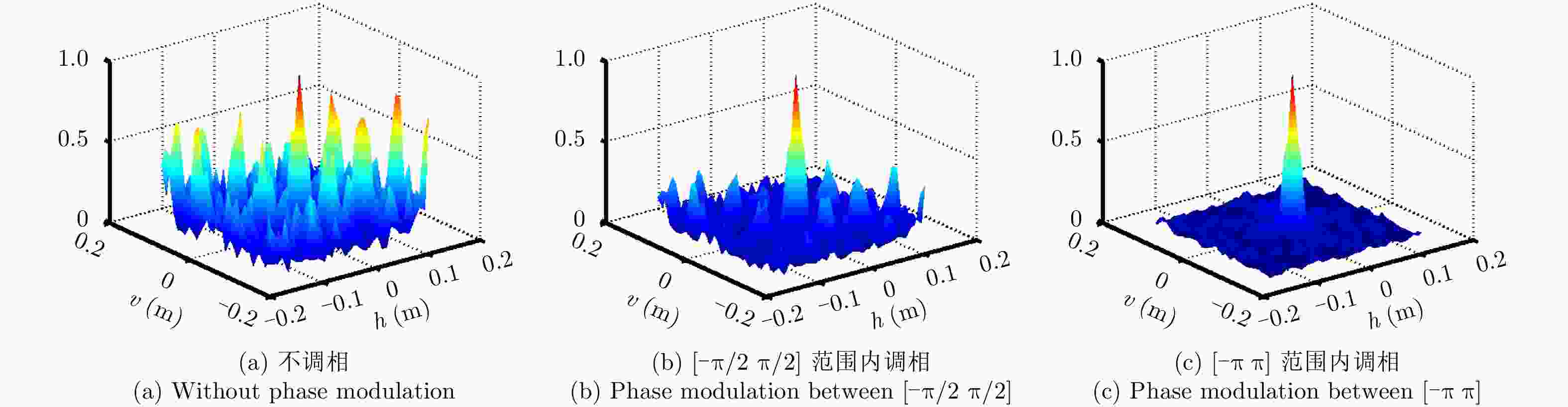
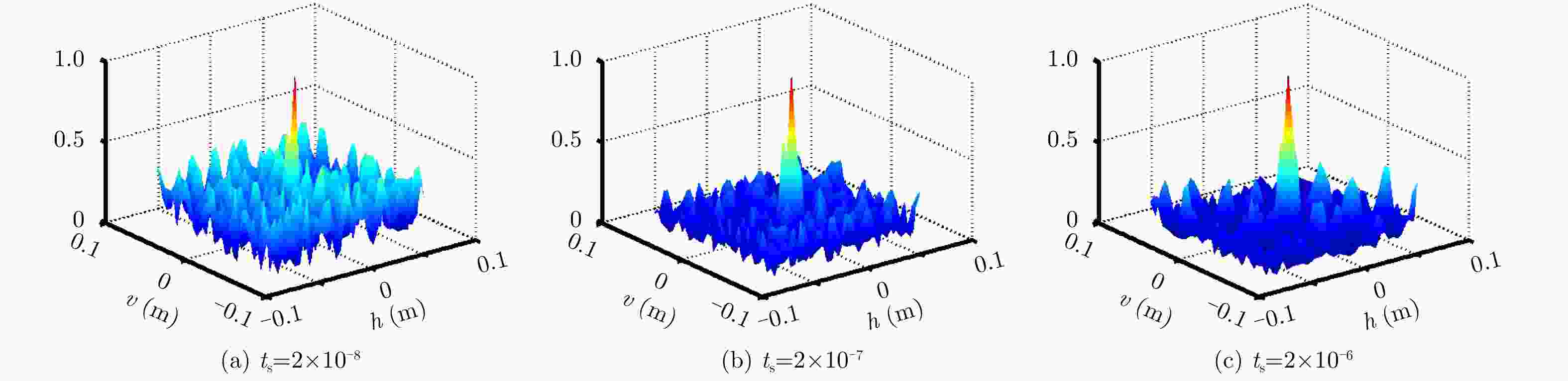
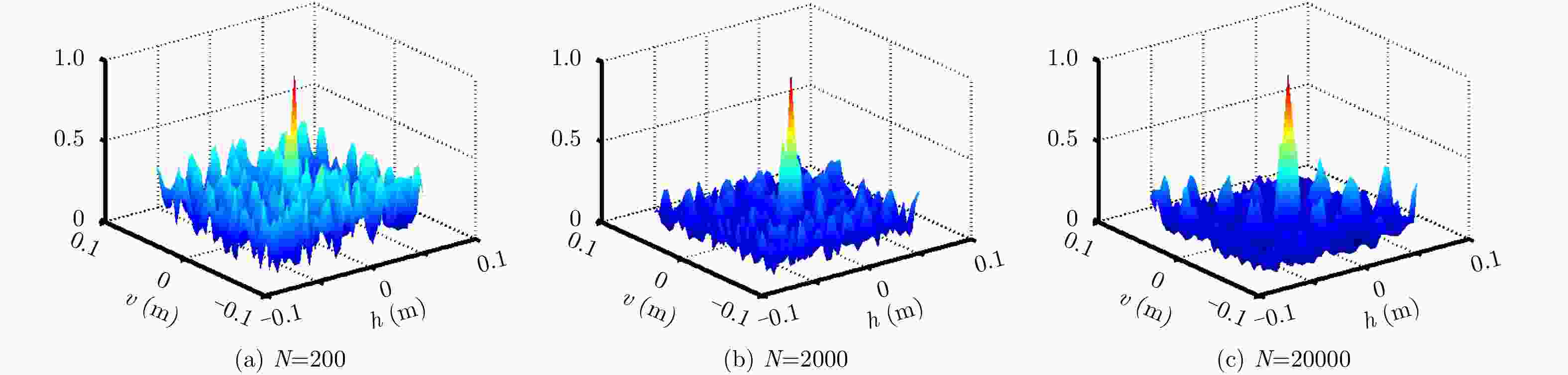
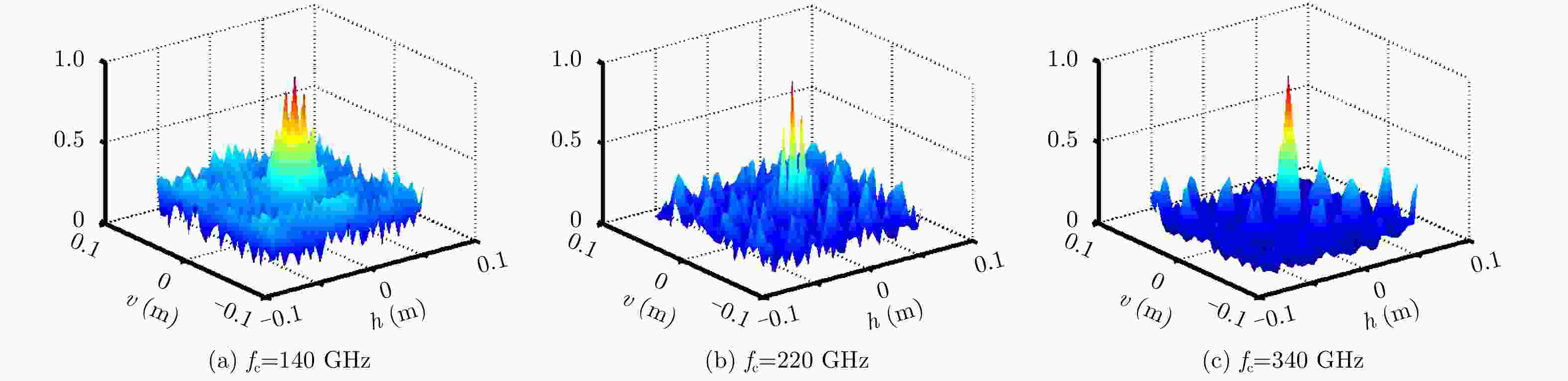
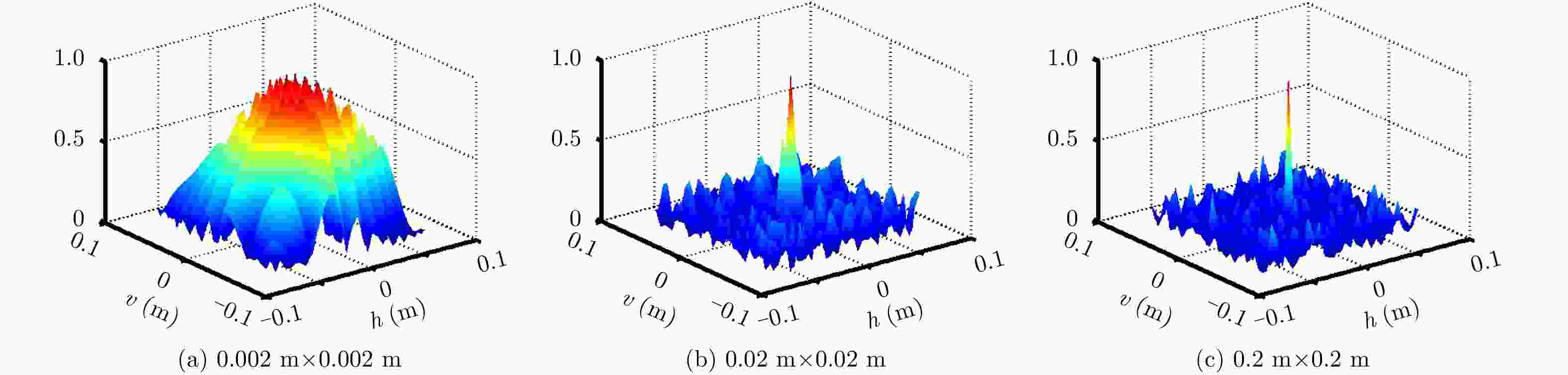
参数 取值 孔径编码天线阵元数量 20×20 孔径编码天线阵元边长lh×lv 0.02 m×0.02 m 孔径编码天线边长 0.3 m×0.3 m 信号振幅 A 1 信号中心频率 fc 340 GHz 信号线性调频系数 k 5×1012 随机调相范围 [– ${\rm{{{π}} }}$/2 ${\rm{{{π}} }}$/2] 采样间隔ts 2×10–6 s 采样次数N 2×103 目标中心斜距R 5 m 成像单元尺寸lc×lc 0.01 m×0.01 m 信噪比(SNR) 20 dB 表 2 成像时间比较
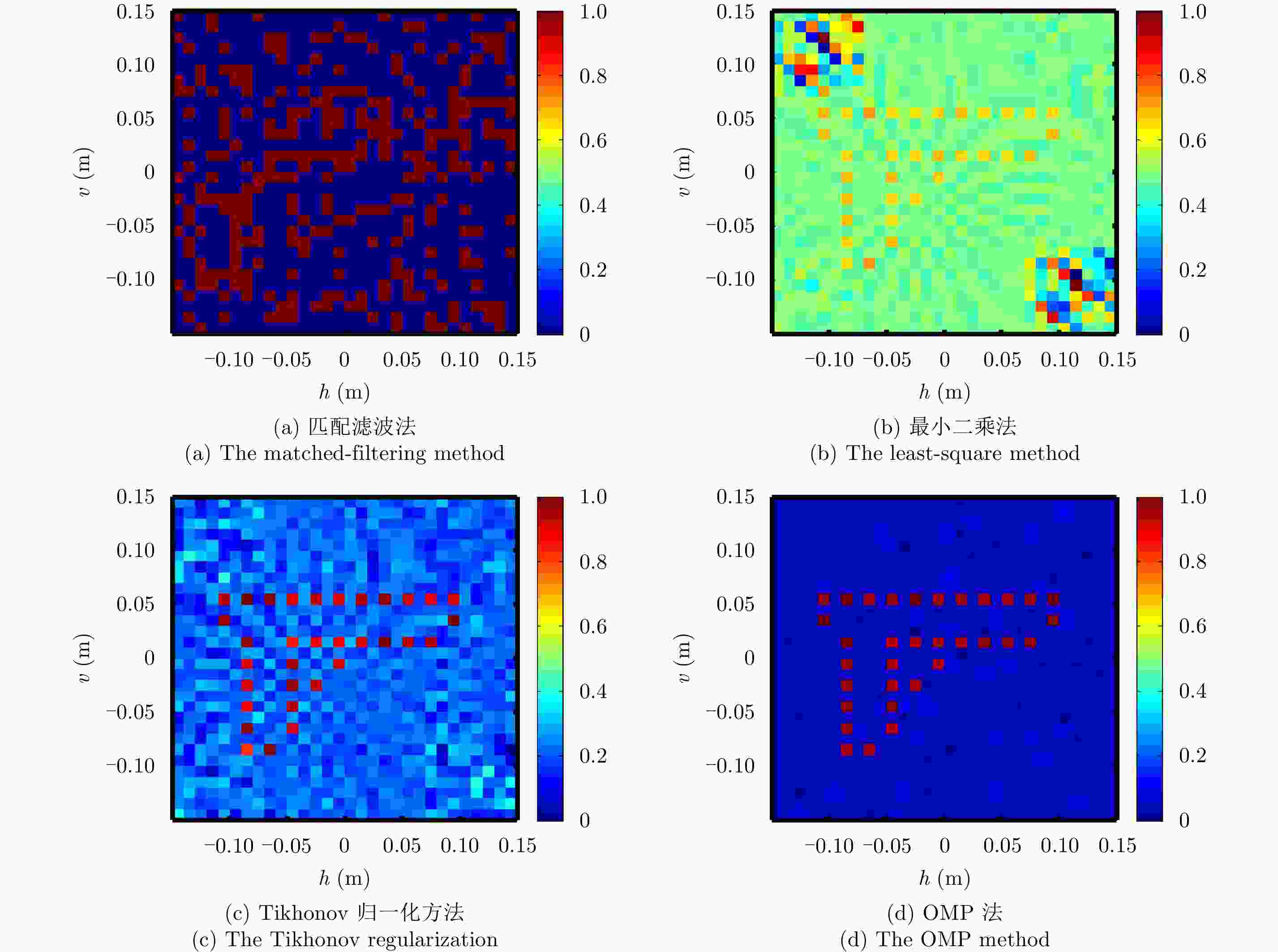
Table 2. The imaging time comparisons
成像算法 运行时间(s) 匹配滤波法 0.350714 最小二乘法 0.679656 Tikhonov法 1.239225 OMP法 0.850584 表 3 关键成像参数
Table 3. The key imaging parameters
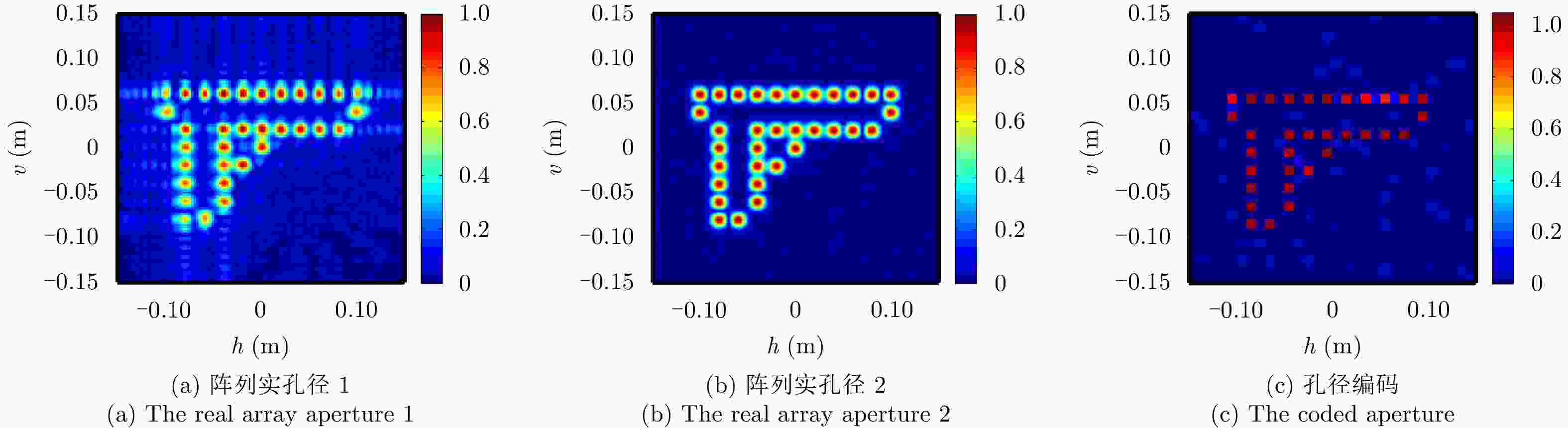
成像方式 编码孔径阵列
尺寸(m×m)编码孔径阵元数量 载频(GHz) 信噪比(dB) 成像距离(m) 阵列实孔径1 0.4×0.4 40×40 340 20 7 阵列实孔径2 0.4×0.4 400×400 340 20 7 孔径编码 0.4×0.4 40×40 340 20 7 -
[1] Liu H B, Zhong H, Karpowicz N, et al. Terahertz spectroscopy and imaging for defense and security applications[J]. Proceedings of the IEEE, 2007, 95(8): 1514–1527. DOI: 10.1109/JPROC.2007.898903 [2] Cooper K B, Dengler R J, Llombart N, et al. THz imaging radar for standoff personnel screening[J]. IEEE Transactions on Terahertz Science and Technology, 2011, 1(1): 169–182. DOI: 10.1109/TTHZ.2011.2159556 [3] Cui T J, Qi M Q, Wan X, et al. Coding metamaterials, digital metamaterials and programmable metamaterials[J]. Light:Science&Applications, 2014, 3(10): e218. [4] Levin A, Fergus R, Durand F, et al. Image and depth from a conventional camera with a coded aperture[J]. ACM Transactions on Graphics, 2007, 26(3): 70. DOI: 10.1145/1276377 [5] Chan W L, Charan K, Takhar D, et al. A single-pixel terahertz imaging system based on compressed sensing[J]. Applied Physics Letters, 2008, 93(12): 121105. DOI: 10.1063/1.2989126 [6] Li D Z, Li X, Cheng Y Q, et al. Radar coincidence imaging: An instantaneous imaging technique with stochastic signals[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(4): 2261–2277. DOI: 10.1109/TGRS.2013.2258929 [7] Li D Z, Li X, Cheng Y Q, et al. Radar coincidence imaging in the presence of target-motion-induced error[J]. Journal of Electronic Imaging, 2014, 23(2): 023014. DOI: 10.1117/1.JEI.23.2.023014 [8] Cooper K B, Dengler R J, Chattopadhyay G, et al. A high-resolution imaging radar at 580 GHz[J]. IEEE Microwave and Wireless Components Letters, 2008, 18(1): 64–66. DOI: 10.1109/LMWC.2007.912049 [9] Siegel P H. Terahertz technology[J]. IEEE Transactions on Microwave Theory and Techniques, 2002, 50(3): 910–928. DOI: 10.1109/22.989974 [10] Sheen D M, Hall T E, Severtsen R H, et al.. Active wideband 350 GHz imaging system for concealed-weapon detection[C]. Proceedings of SPIE Passive Millimeter-Wave Imaging Technology, Orlando, Florida, United States, 2009, 7309: 73090I. [11] Yu N F, Genevet P, Kats M A, et al. Light propagation with phase discontinuities: Generalized laws of reflection and refraction[J]. Science, 2011, 334(6054): 333–337. DOI: 10.1126/science.1210713 [12] Perez-Palomino G, Encinar J A, Dickie R, et al.. Preliminary design of a liquid crystal-based reflectarray antenna for beam-scanning in THz[C]. Proceedings of 2013 IEEE Antennas and Propagation Society International Symposium, Orlando, FL, USA, 2013: 2277–2278. [13] Perez-Palomino G, Barba M, Encinar J A, et al. Design and demonstration of an electronically scanned reflectarray antenna at 100 GHz using multiresonant cells based on liquid crystals[J]. IEEE Transactions on Antennas and Propagation, 2015, 63(8): 3722–3727. DOI: 10.1109/TAP.2015.2434421 [14] Llombart N, Cooper K B, Dengler R J, et al. Confocal ellipsoidal reflector system for a mechanically scanned active terahertz imager[J]. IEEE Transactions on Antennas and Propagation, 2010, 58(6): 1834–1841. DOI: 10.1109/TAP.2010.2046860 [15] Martinez-Lorenzo J A, Garcia-Pino A, Gonzalez-Valdes B, et al. Zooming and scanning Gregorian confocal dual reflector antennas[J]. IEEE Transactions on Antennas and Propagation, 2008, 56(9): 2910–2919. DOI: 10.1109/TAP.2008.928777 [16] Imaizumi Y, Suzuki Y, Kawakami Y, et al.. A study on an onboard Ka-band phased-array-fed imaging reflector antenna[C]. Proceedings of 2002 IEEE Antennas and Propagation Society International Symposium, San Antonio, TX, USA, 2002, 4: 144–147. [17] Hansen P C. Rank-Deficient and Discrete Ill-Posed Problems: Numerical Aspects of Linear Inversion[M]. Philadelphia, PA, USA: Society for Industrial and Applied Mathematics, 1998: 45–67. -



 作者中心
作者中心 专家审稿
专家审稿 责编办公
责编办公 编辑办公
编辑办公
 下载:
下载: