频域稀疏毫米波人体安检成像处理和快速成像稀疏阵列设计

田鹤; 李道京; 祁春超

doi:10.12000/JR17082

频域稀疏毫米波人体安检成像处理和快速成像稀疏阵列设计

DOI: 10.12000/JR17082

田鹤^1,2,,
李道京^1, ,,
祁春超^3, ,

①.
中国科学院电子学研究所微波成像技术重点实验室北京 100190
②.
中国科学院大学北京 100190
③.
华讯方舟科技有限公司深圳 518000

基金项目: 国家自然科学基金（61271422）

详细信息

作者简介:
田鹤：田鹤(1991–)，女，河北邢台人，中国科学院电子学研究所信号与信息处理专业博士研究生，研究方向为稀疏阵列天线雷达三维成像技术。E-mail: tianhe0407@126.com

李道京(1964–)，男，1986年和1991年在南京理工大学分别获通信与电子系统专业工学学士和硕士学位，2003年在西北工业大学电路与系统专业获工学博士学位，现为中国科学院电子学研究所研究员，博士生导师，研究方向为雷达系统和雷达信号处理。E-mail: lidj@mail.ie.ac.cn

祁春超(1977–)，男，高级工程师，深圳市高层次人才，2010年博士毕业于华中科技大学光学工程专业，博士毕业后在中国科学院光电研究院从事高重频、窄线宽、高功率、193 nm ArF光刻系统研制工作，2011年在深圳大学光学工程博士后流动站从事博士后研究工作，2013年–2015年在南方科技大学从事太赫兹领域的科研与教学工作，2015年至今在华讯方舟科技有限公司担任太赫兹首席科学家，主要从事毫米波人体安检仪的研制工作。E-mail: qichunchao@huaxunchina.cn

通讯作者:
李道京 lidj@mail.ie.ac.cn

祁春超 qichunchao@huaxunchina.cn

计量
- 文章访问数: 4699
- HTML全文浏览量: 1058
- PDF下载量: 643
- 被引次数: 0
出版历程
- 收稿日期: 2017-09-08
- 修回日期: 2017-11-17
- 网络出版日期: 2018-06-28

Millimeter-wave Human Security Imaging Based on Frequency-domain Sparsity and Rapid Imaging Sparse Array Architecture

Tian He^{1,2
,},
Li Daojing^{1
, ,},
Qi Chunchao^{3
, ,}

①.
Science and Technology on Microwave Imaging Laboratory, Institute of Electronics, Chinese Academy of Sciences, Beijing 100190, China
②.
University of Chinese Academy of Sciences, Beijing 100190, China
③.
China Communication Technology Co., Ltd., Shenzhen 518000, China

Funds: The National Natural Science Foundation of China (61271422)

摘要

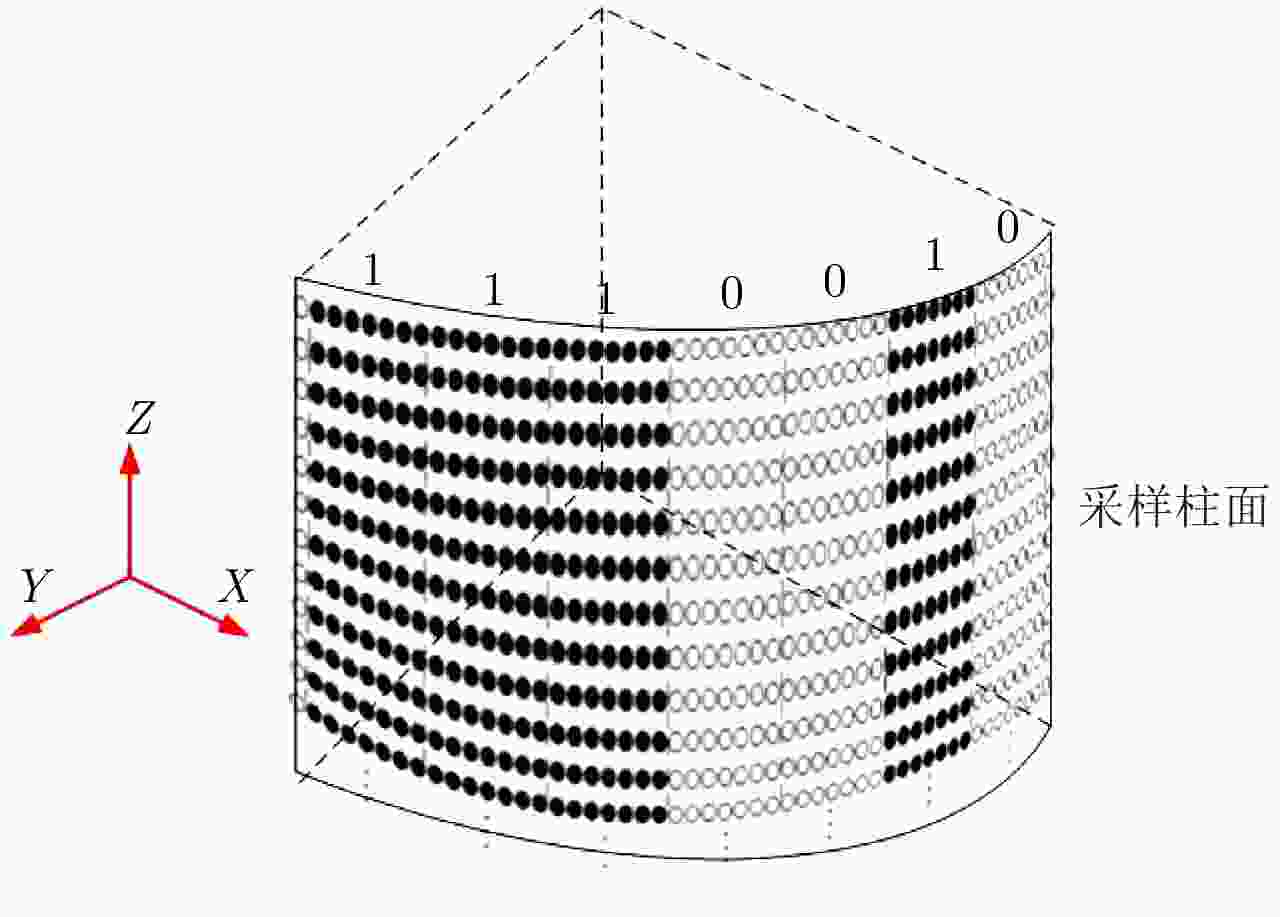
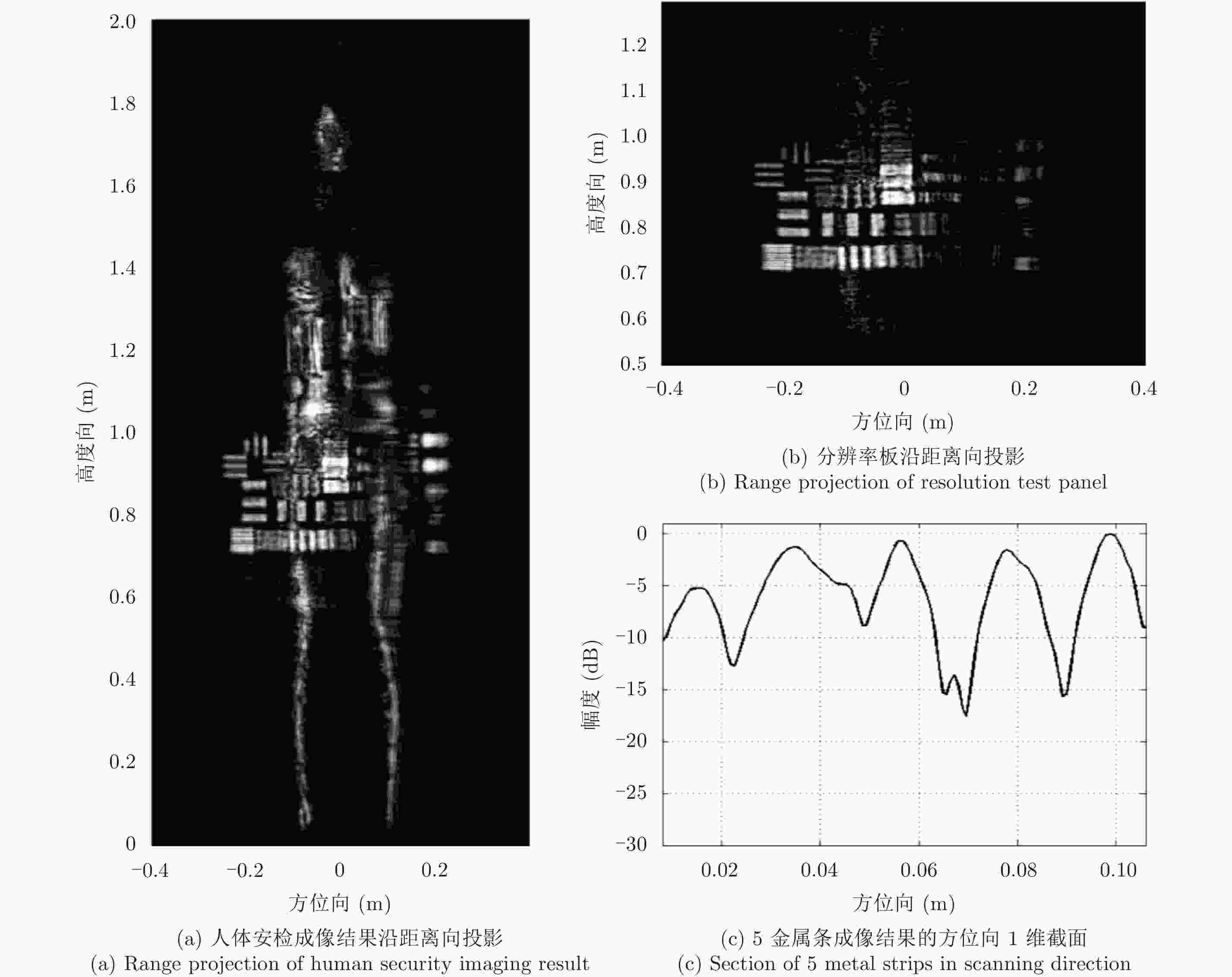
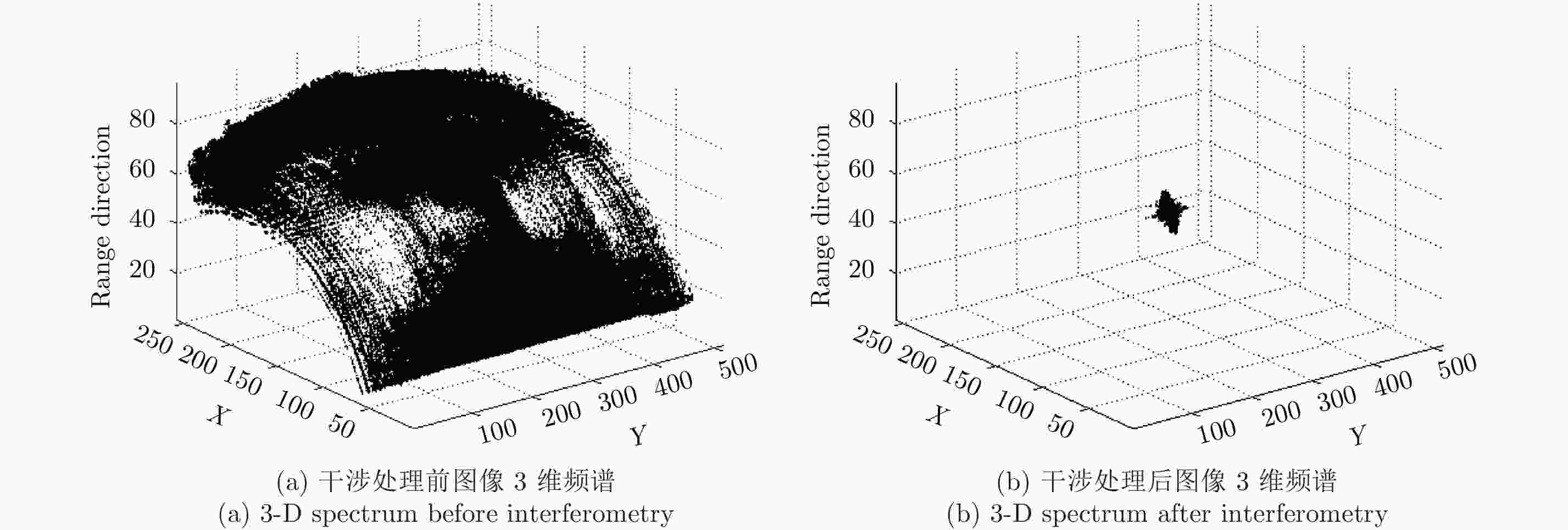
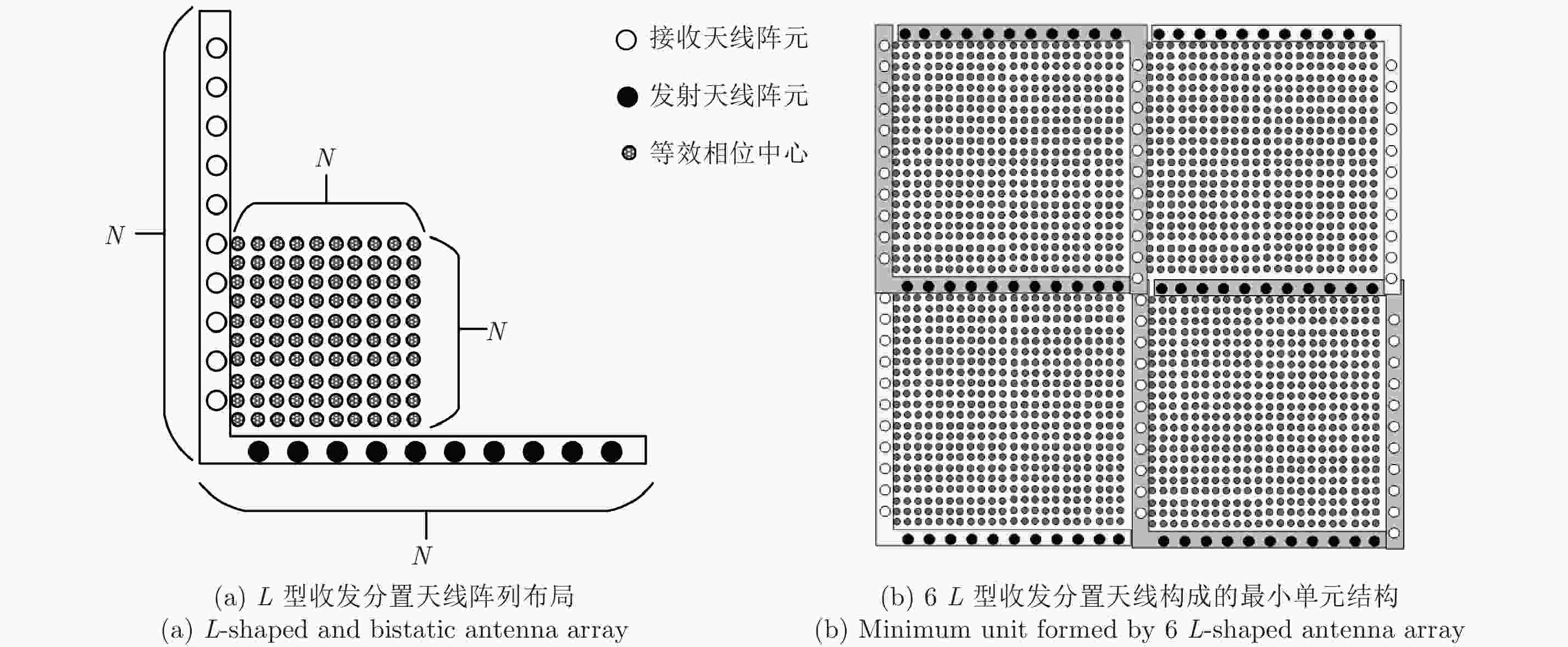
摘要: 该文研究工作包括频域稀疏毫米波人体安检成像数据处理和用于快速安检成像的稀疏阵列设计两部分。首先基于柱面扫描成像模型，采用巴克码随机稀疏采样方式减少成像所需数据量；提出一种基于干涉处理和频域压缩感知的3维成像算法，利用干涉处理使人体复图像在频域具备稀疏性，建立频域压缩感知测量模型并重建图像频谱，进而实现稀疏采样下人体安检图像3维重建。实际数据处理结果表明，该方法在数据采集量减少约50%条件下，可获得接近满采样对应的图像分辨率和成像效果，稀疏采样前后的图像相关系数优于0.9。其次基于频域稀疏成像方法、巴克码稀疏采样方式和收发分置工作模式，设计了用于快速安检成像的稀疏阵列布局，在保证人体成像质量前提下，稀疏率高达94.6%。该方法用于实际安检成像系统中可大幅增加安检通过速率、减少辐射单元数量和系统复杂度，在大人流量、高安检要求场所安全检测中具有重要应用价值和市场前景。
- 毫米波成像 /
- 3维成像 /
- 稀疏采样 /
- 稀疏阵列 /
- 干涉处理 /
- 人体安检
Abstract: This paper examines the processing of millimeter-wave imaging data based on sparse sampling and sparse array design for the rapid imaging of human security data. First, based on the cylindrical scanning imaging model, the Barker code-based randomly sparse sampling method is employed to reduce the scanning time. Then, a three-dimensional imaging algorithm based on interferometry and compressed sensing in the frequency domain is proposed, with sparse representation of the image in the frequency domain after interferometry and Compressed Sensing measurement model, to recover the image frequency spectrum, thereby implementing human security image reconstruction via sparse sampling. Real data processing results indicated that the proposed method could obtain image resolution and performance similar to those of complete samples and that the image correlation coefficients before and after sparse sampling were better than 0.9, with 50% time/data reduction. Furthermore, based on the Barker codes and multistatic work mode, a sparse array architecture for rapid imaging was designed with a sparse rate of 94.6% and the guarantee of imaging quality. The proposed method was found to considerably increase the passage rate and reduce the amount of radiation unit and system complexity, marking its application significance and market prospect in security clearance.
- Millimeter wave imaging /
- 3D imaging /
- Sparse sampling /
- Sparse array /
- Interferometry /
- Human security

HTML全文

图 1 毫米波人体安检柱面扫描3维成像模型

Figure 1. The cylindrical scanning 3-D imaging model of a millimeter wave human security system

下载: 全尺寸图片幻灯片

图 2 巴克码[1110010]的自相关函数图和方向图

Figure 2. The Autocorrelation and Beam pattern diagrams for Barker code of [1110010]

下载: 全尺寸图片幻灯片

图 3 柱面扫描型成像系统和测试目标

Figure 3. The cylindrical scanning imaging system and testing objectives

下载: 全尺寸图片幻灯片

图 4 柱面回波数据稀疏方式示意图

Figure 4. The sparse sampling method of cylindrical echo

下载: 全尺寸图片幻灯片

图 5 满采样条件下的人体安检直接成像结果

Figure 5. The direct imaging result under complete sampling

下载: 全尺寸图片幻灯片

图 6 等间隔稀疏采样条件下的人体安检直接成像结果

Figure 6. The direct imaging result under uniformly spaced sparse sampling

下载: 全尺寸图片幻灯片

图 7 基于巴克码稀疏采样条件下的人体安检直接成像结果

Figure 7. The direct imaging result under Barker code sparse sampling

下载: 全尺寸图片幻灯片

图 8 干涉处理前后图像3维频谱

Figure 8. The 3-D frequency spectrum before and after interferometry

下载: 全尺寸图片幻灯片

图 9 基于干涉处理和频域CS 3维成像算法的图像重建结果

Figure 9. The reconstruction of 3-D imaging algorithm based on interferometry and CS in frequency domain

下载: 全尺寸图片幻灯片

图 10 2维稀疏阵列中最小单元结构示意图

Figure 10. The structure of minimum unit in 2-D sparse array

下载: 全尺寸图片幻灯片

图 11 毫米波快速成像2维稀疏阵列布局示意图

Figure 11. The 2-D sparse array structure for millimeter wave fast imaging

下载: 全尺寸图片幻灯片

表 1 系统参数

Table 1. System parameters

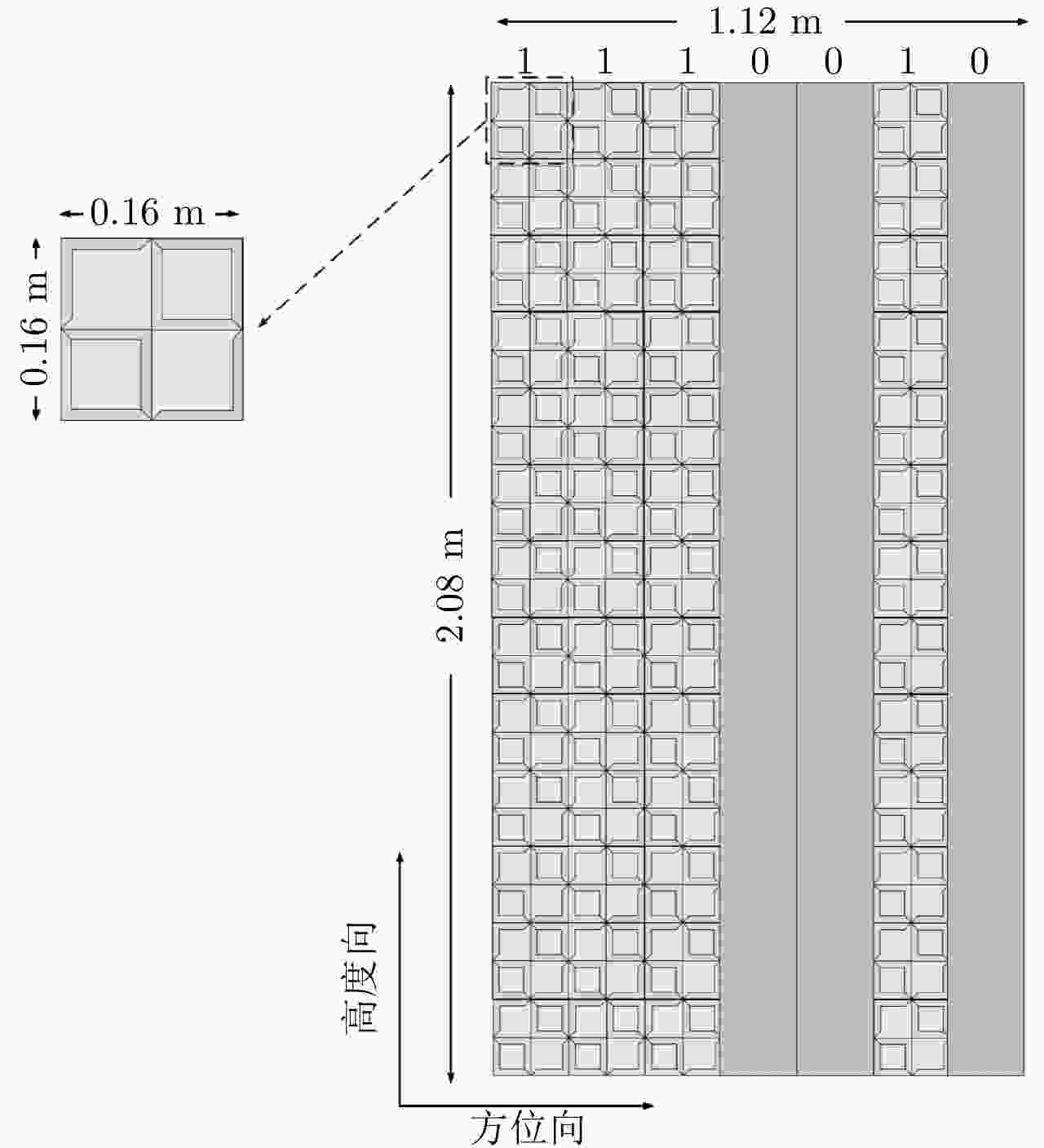
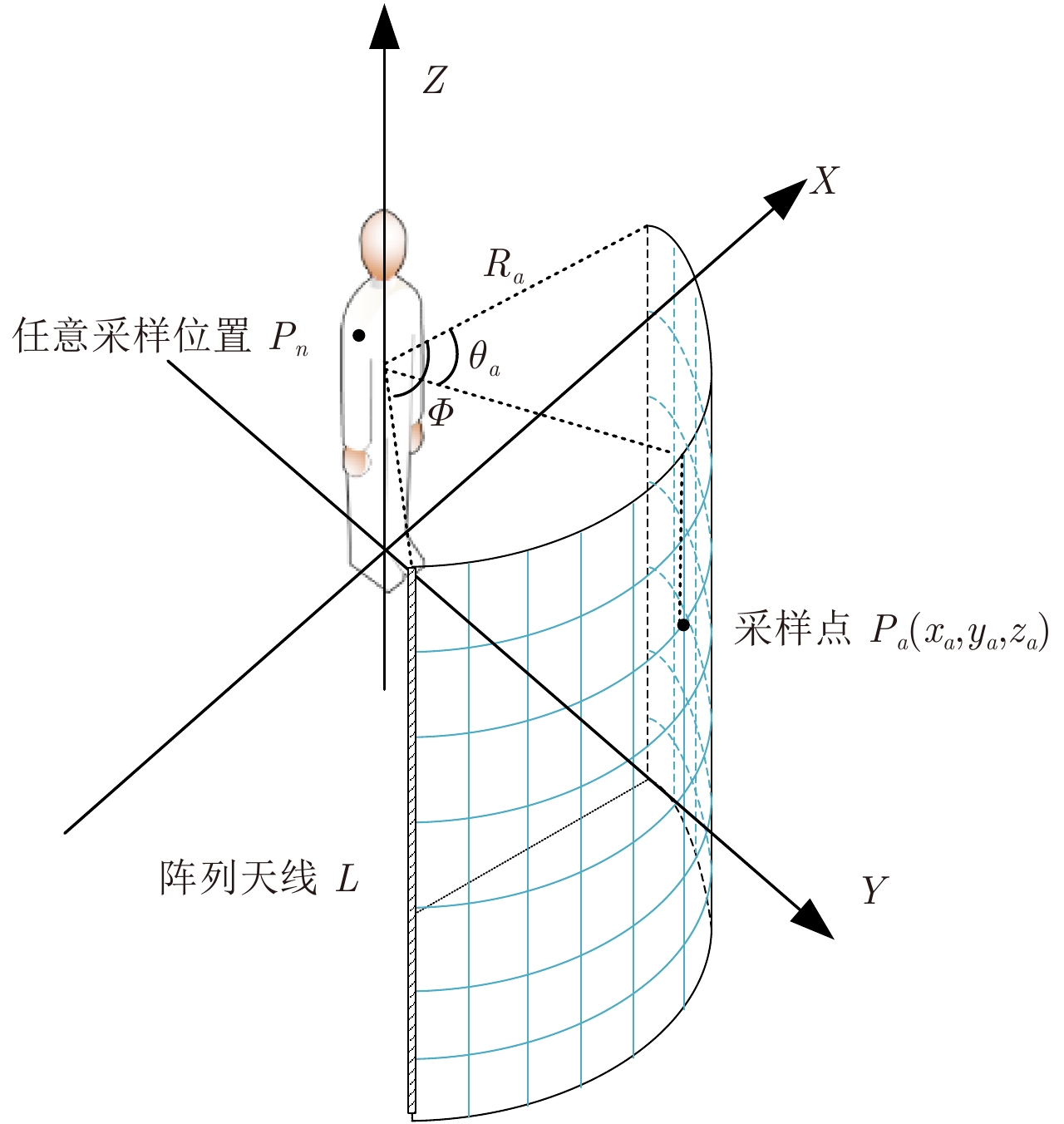
参数	数值
测试距离R_a (m)	0.675
发射信号带宽B_s (GHz)	9.50
雷达中心频率f₀ (GHz)	33.87
雷达工作波长 $\lambda $ (mm)	8.90
距离向采样率f_s (GHz)	9.50
距离向采样间隔d_r (cm)	1.58
方位向扫描角 ${{Φ}}$ (°)	66
方位向采样间隔d_a (°)	0.32
阵列长度L (m)	2
高度向采样间隔d_z (mm)	4.80
距离向分辨率 (cm)	1.58
方位向分辨率 (mm)	4.50
高度向分辨率 (mm)	4.50

下载: 导出CSV

表 2 3种巴克码序列

Table 2. The 3 kinds of Barker sequences

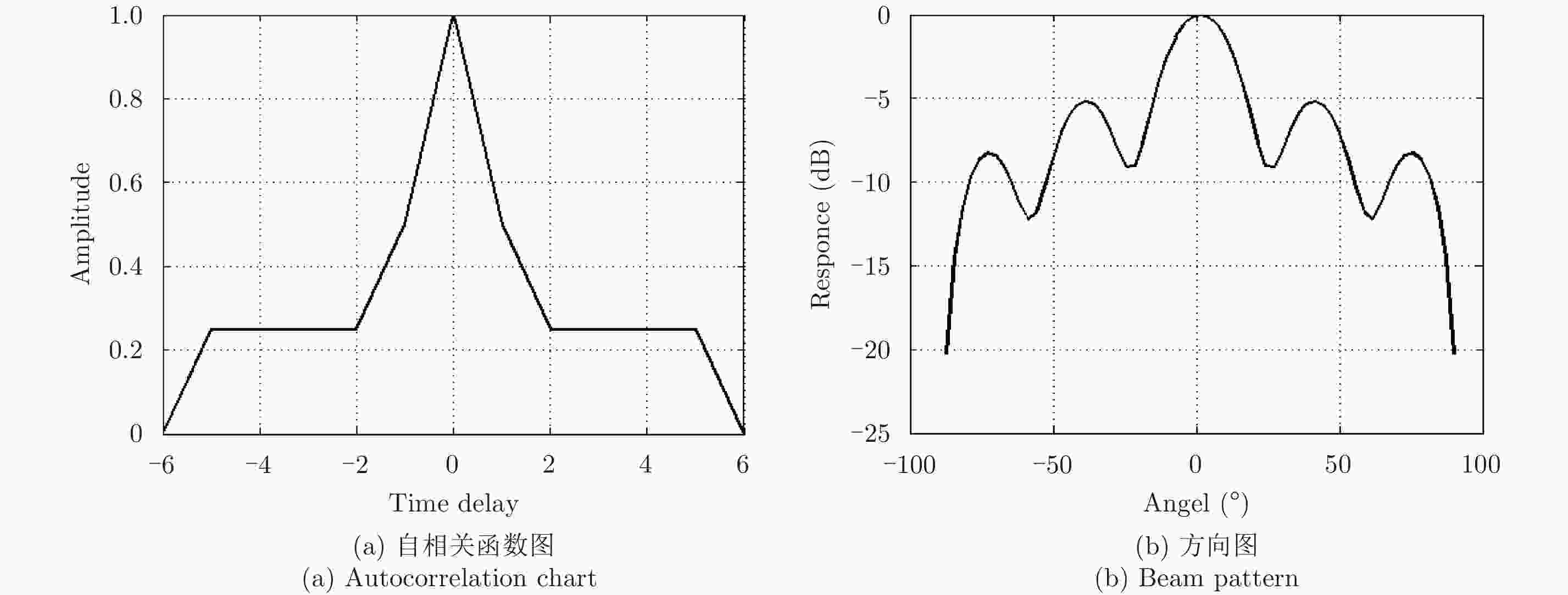
巴克码长度	码型	稀疏率(%)
7	1 1 1 0 0 1 0	42.86
11	1 1 1 0 0 0 1 0 0 1 0	54.55
13	1 1 1 1 1 0 0 1 1 0 1 0 1	30.77

下载: 导出CSV

表 3 7位巴克码稀疏采样方式对应的图像质量评价

Table 3. The image quality assessment corresponding to Barker code with length of 7

成像方法	相关系数	SSIM	MSE
等间隔稀疏采样直接成像	0.8698	0.8169	0.0431
7 位Barker码稀疏采样直接成像	0.8991	0.8973	0.0388
7 位Barker码稀疏采样干涉处理频域CS成像	0.9118	0.9036	0.0359

下载: 导出CSV

表 4 11位巴克码稀疏采样方式对应的图像质量评价

Table 4. The image quality assessment corresponding to Barker code with length of 11

成像方法	相关系数	SSIM	MSE
11位Barker码稀疏采样直接成像	0.8768	0.8552	0.0491
11位Barker码稀疏采样干涉处理频域CS成像	0.9028	0.8676	0.0418

下载: 导出CSV

表 5 13位巴克码稀疏采样方式对应的图像质量评价

Table 5. The image quality assessment corresponding to Barker code with length of 13

成像方法	相关系数	SSIM	MSE
13位Barker码稀疏采样直接成像	0.9159	0.8892	0.0343
13位Barker码稀疏采样干涉处理频域CS成像	0.9321	0.9052	0.0324

下载: 导出CSV

参考文献(22)

[1]	McMillan R W, Currie N C, Ferris D D, et al.. Concealed weapon detection using microwave and millimeter wave sensors[C]. Proceedings of 1998 International Conference on Microwave and Millimeter Wave Technology Proceedings, Beijing, 1998: 1–4. DOI: 10.1109/ICMMT.1998.768213.
[2]	温鑫, 黄培康, 年丰, 等. 主动式毫米波近距离圆柱扫描三维成像系统[J]. 系统工程与电子技术, 2014, 36(6): 1044–1049. DOI: 10.3969/j.issn.1001-506X.2014.06.05 Wen Xin, Huang Pei-kang, Nian Feng, et al. Active millimeter-wave near-field cylindrical scanning three-dimensional imaging system[J]. Systems Engineering and Electronics, 2014, 36(6): 1044–1049. DOI: 10.3969/j.issn.1001-506X.2014.06.05
[3]	Farhat N H and Guard W R. Millimeter wave holographic imaging of concealed weapons[J]. Proceedings of the IEEE, 1971, 59(9): 1383–1384. DOI: 10.1109/PROC.1971.8441
[4]	Gomez-Maqueda I, Almorox-Gonzalez P, Callejero-Andres C, et al. A millimeter-wave imager using an illuminating source[J]. IEEE Microwave Magazine, 2013, 14(4): 132–138. DOI: 10.1109/MMM.2013.2248652
[5]	Ahmed S S, Genghammer A, Schiessl A, et al. Fully electronic E-band personnel imager of 2 m² aperture based on a multistatic architecture[J]. IEEE Transactions on Microwave Theory and Techniques, 2013, 61(1): 651–657. DOI: 10.1109/TMTT.2012.2228221
[6]	Ahmed S S, Genghammer A, Schiessl A, et al.. Fully electronic active E-band personnel imager with 2 m² aperture[C]. Proceedings of 2012 IEEE MTT-S International Microwave Symposium Digest, Montreal, QC, Canada, 2012: 1–3. DOI: 10.1109/MWSYM.2012.6259549.
[7]	Li L C, Li D J, and Pan Z H. Compressed sensing application in interferometric synthetic aperture radar[J]. Science China Information Sciences, 2017, 60(10): 102305. DOI: 10.1007/s11432-016-9017-6
[8]	Tian H, Li D J, and Li L C. Simulation of signal reconstruction based sparse flight downward-looking 3D imaging SAR[C]. Proceedings of 2015 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Milan, 2015: 3762–3765. DOI: 10.1109/IGARSS.2015.7326642.
[9]	Holubnychyi A. Generalized binary barker sequences and their application to radar technology[C]. Proceedings of 2013 Signal Processing Symposium (SPS), Serock, 2013: 1–9. DOI: 10.1109/SPS.2013.6623610.
[10]	Detlefsen J, Dallinger A, and Schelkshorn S. Approaches to millimeter-wave imaging of humans[C]. Proceedings of the First European Radar Conference, Amsterdam, the Netherlands, 2004: 279–282.
[11]	Rosen P A, Hensley S, Joughin I R, et al. Synthetic aperture radar interferometry[J]. Proceedings of the IEEE, 2000, 88(3): 333–382. DOI: 10.1109/5.838084
[12]	Tian H and Li D J. Sparse flight array SAR downward-looking 3-D imaging based on compressed sensing[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(10): 1395–1399. DOI: 10.1109/LGRS.2016.2560238
[13]	张清娟, 李道京, 李烈辰. 连续场景的稀疏阵列SAR侧视三维成像研究[J]. 电子与信息学报, 2013, 35(5): 1097–1102. DOI: 10.3724/SP.J.1146.2012.01136 Zhang Qing-juan, Li Dao-jing, and Li Lie-chen. Research on continuous scene side-looking 3D imaging based on sparse array[J]. Journal of Electronics&Information Technology, 2013, 35(5): 1097–1102. DOI: 10.3724/SP.J.1146.2012.01136
[14]	田鹤, 李道京, 潘洁, 等. 基于修正均匀冗余阵列正反编码的稀疏阵列SAR下视三维成像处理[J]. 电子与信息学报, 2017, 39(9): 2203–2211. DOI: 10.11999/JEIT161209 Tian He, Li Dao-jing, Pan Jie, et al. Downward-looking 3D imaging processing of sparse array SAR based on modified uniformly redundant arrays positive and negative coding[J]. Journal of Electronics&Information Technology, 2017, 39(9): 2203–2211. DOI: 10.11999/JEIT161209
[15]	李烈辰, 李道京. 基于压缩感知的连续场景稀疏阵列SAR三维成像[J]. 电子与信息学报, 2014, 36(9): 2166–2172. DOI: 10.3724/SP.J.1146.2013.01645 Li Lie-chen and Li Dao-jing. Sparse array SAR 3D imaging for continuous scene based on compressed sensing[J]. Journal of Electronics&Information Technology, 2014, 36(9): 2166–2172. DOI: 10.3724/SP.J.1146.2013.01645
[16]	Candès E and Romberg J. Sparsity and incoherence in compressive sampling[J]. Inverse Problems, 2007, 23(3): 969–985. DOI: 10.1088/0266-5611/23/3/008
[17]	Donoho D L. Compressed sensing[J]. IEEE Transactions on Information Theory, 2006, 52(4): 1289–1306. DOI: 10.1109/TIT.2006.871582
[18]	Baraniuk R and Steeghs P. Compressive radar imaging[C]. Proceedings of 2007 IEEE Radar Conference, Boston, Mass, USA, 2007: 128–133. DOI: 10.1109/RADAR.2007.374203.
[19]	Patel V M, Easley G R, Healy jr D M, et al. Compressed synthetic aperture radar[J]. IEEE Journal of Selected Topics in Signal Processing, 2010, 4(2): 244–254. DOI: 10.1109/JSTSP.2009.2039181
[20]	Tian H and Li D J. Sparse sampling-based microwave 3D imaging using interferometry and frequency-domain principal component analysis[J]. IET Radar,Sonar&Navigation, 2017, 11(12): 1886–1891. DOI: 10.1049/iet-rsn.2017.0087
[21]	Schiessl A, Ahmed S S, Genghammer A, et al.. A technology demonstrator for a 0.5 m x 0.5 m fully electronic digital beamforming mm-Wave imaging system[C]. Proceedings of the 5th European Conference on Antennas and Propagation (EUCAP), Rome, 2011: 2606–2609.
[22]	Tian H, Li D J, and Hu X. Microwave three-dimensional imaging under sparse sampling based on MURA code[C]. Proceedings of 2016 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Beijing, 2016: 7411–7414. DOI: 10.1109/IGARSS.2016.7730933.