作者中心
专家审稿
责编办公
编辑办公
Polarimetric SAR Target Scattering Interpretation in Rotation Domain: Theory and Application
-
摘要: 雷达目标的后向散射敏感于目标姿态与雷达视线的相对几何关系。雷达目标的这种散射多样性给以极化合成孔径雷达(SAR)为代表的成像雷达信息处理与应用造成诸多不便，是当前目标散射机理精细解译和定量应用面临的主要技术瓶颈之一。该文回顾并介绍一种在绕雷达视线旋转域解译目标散射机理的新思路，主要包括新近提出的统一的极化矩阵旋转理论和极化相干特征旋转域可视化解译理论。目标散射旋转域解译方法的核心思想是将特定几何关系下获得的目标信息拓展到绕雷达视线的旋转域，为目标散射信息深度挖掘和利用奠定基础。该文详细分析上述方法导出的一系列新的极化特征参数集，并开展应用验证。对比研究证实了旋转域解译方法在地物辨识与分类等领域的应用潜力。Abstract: Backscattering of radar targets is sensitive to the relative geometry between target orientations and the radar line of sight. This scattering diversity makes imaging radar represented by polarimetric Synthetic Aperture Radar (SAR) information processing and applications very difficult. This situation has become one of the main bottlenecks in the interpretation of the target scattering mechanism and quantitative applications. In this work, we review and introduce a new interpretation of the target scattering mechanism in the rotation domain along the radar line of sight. This concept includes the recently established uniform polarimetric matrix rotation theory and polarimetric coherence pattern visualization and interpretation in the rotation domain. The core idea of target scattering interpretation in the rotation domain is to extend the amount of target information acquired at a given geometry to the rotation domain, which then provides fundamentals for the deep mining and utilization of target scattering information. This work mainly focuses on the investigation of derived new polarimetric feature sets and application demonstrations. Comparison study results validate the promising potential for the application of the established interpretation framework in the rotation domain with respect to target discrimination and classification.
-
图 4 极化相干特征旋转域优化前后对比图
Figure 4. Comparison of polarimetric coherence without and with optimization in rotation domain
图 5 极化相干特征旋转域优化前后的统计分布图
Figure 5. Histograms of polarimetric coherence for full scene AIRSAR data
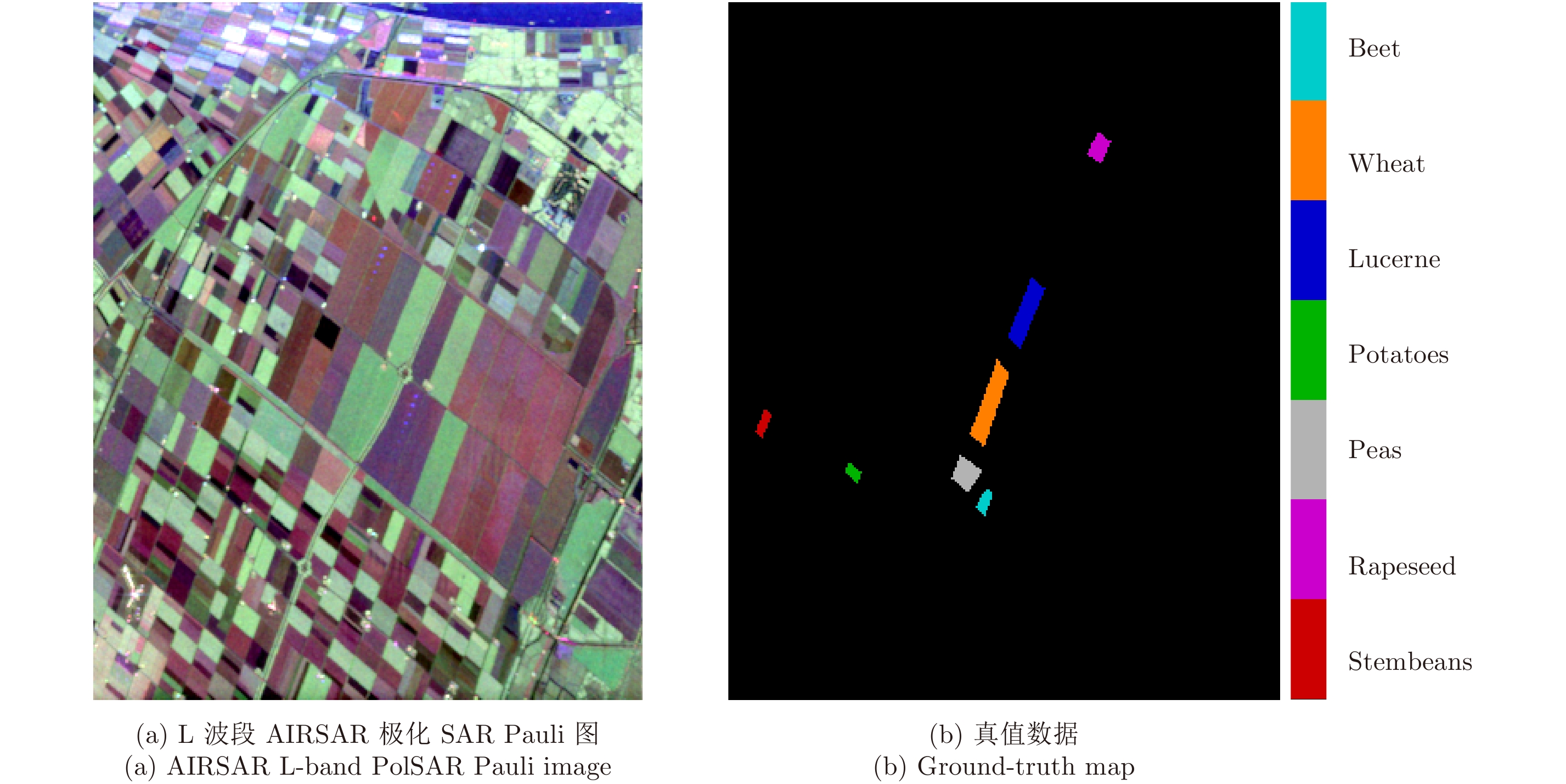
图 6 AIRSAR数据中7种已知地物的旋转域极化相干特征的可视化图。(a1)–(a4) 茎豆，(b1)–(b4) 油菜，(c1)–(c4)豌豆，(d1)–(d4)土豆，(e1)–(e4)紫苜蓿，(f1)–(f4)小麦，(g1)–(g4)甜菜。其中，1–4分别代表 $\left| {{\gamma _{\left( {{\rm{HH}} + {\rm{VV}}} \right) - \left( {{\rm{HH}} - {\rm{VV}}} \right)}}\left( \theta \right)} \right|$, $\left| {{\gamma _{\left( {{\rm{HH}} - {\rm{VV}}} \right) - \left( {{\rm{HV}}} \right)}}\left( \theta \right)} \right|$, $\left| {{\gamma _{{\rm{HH}} - {\rm{VV}}}}\left( \theta \right)} \right|$和 $\left| {{\gamma _{{\rm{HH}} - {\rm{HV}}}}\left( \theta \right)} \right|$
Figure 6. Polarimetric coherence patterns of the seven crop types from AIRSAR data. (a1)–(a4)stembeans, (b1)–(b4)rapeseed, (c1)–(c4)peas, (d1)–(d4)potatoes, (e1)–(e4)lucerne, (f1)–(f4)wheat and (g1)–(g4)beet. The numbers 1–4 indicate $\left| {{\gamma _{\left( {{\rm{HH}} + {\rm{VV}}} \right) - \left( {{\rm{HH}} - {\rm{VV}}} \right)}}\left( \theta \right)} \right|$, $\left| {{\gamma _{\left( {{\rm{HH}} - {\rm{VV}}} \right) - \left( {{\rm{HV}}} \right)}}\left( \theta \right)} \right|$, $\left| {{\gamma _{{\rm{HH}} - {\rm{VV}}}}\left( \theta \right)} \right|$ and $\left| {{\gamma _{{\rm{HH}} - {\rm{HV}}}}\left( \theta \right)} \right|$, respectively
图 7 旋转域极化相干特征典型值对比图
Figure 7. Errorbar plots of typical polarimetric coherence parameters
图 8 旋转域极化相干度对比图
Figure 8. Errorbar plots for polarimetric coherence degree in rotation domain
图 9 旋转域极化相干起伏度对比图
Figure 9. Errorbar plots of polarimetric coherence fluctuation in rotation domain
图 10 旋转域极化相干对比度对比图
Figure 10. Errorbar plots of polarimetric coherence contrast in rotation domain
图 11 旋转域极化相干宽度对比图
Figure 11. Errorbar plots of polarimetric coherence beamwidth ${\rm{B}}{{\rm{W}}_{0.95}}$ in rotation domain
图 12 旋转域极化相干特征最大化角对比图
Figure 12. Errorbar plots of maximum rotation angles ${\theta _{\gamma {\rm{ - }}\max }}$ (in deg) which produce maximized coherence in rotation domain
图 13 旋转域极化相干特征最小化角对比图
Figure 13. Errorbar plots of minimum rotation angles ${\theta _{\gamma {\rm{ - }}\min }}$ (in deg) which produce minimized coherence in rotation domain
图 14 基于极化特征组合的农作物辨识结果
Figure 14. Crops discrimination results based on combinations of derived polarimetric parameters
表 1 旋转域极化相干矩阵的振荡参数集[40]
Table 1. Oscillation parameter set of polarimetric coherence matrix in rotation domain
元素项 $A = \sqrt \cdot $ B $\omega $ ${\theta _0} = \displaystyle \frac{1}{\omega }{\rm{Angle}}\left\{ \cdot \right\}$ ${\mathop{\rm Re}\nolimits} \left[ {{T_{12}}\left( \theta \right)} \right]$ ${{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{12}}} \right] + {{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{13}}} \right]$ 0 2 ${\mathop{\rm Re}\nolimits} \left[ {{T_{13}}} \right] + j{\mathop{\rm Re}\nolimits} \left[ {{T_{12}}} \right]$ ${\mathop{\rm Re}\nolimits} \left[ {{T_{13}}\left( \theta \right)} \right]$ ${{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{12}}} \right] + {{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{13}}} \right]$ 0 2 $ - {\mathop{\rm Re}\nolimits} \left[ {{T_{12}}} \right] + j{\mathop{\rm Re}\nolimits} \left[ {{T_{13}}} \right]$ ${\mathop{\rm Im}\nolimits} \left[ {{T_{12}}\left( \theta \right)} \right]$ ${{\mathop{\rm Im}\nolimits} ^2}\left[ {{T_{12}}} \right] + {{\mathop{\rm Im}\nolimits} ^2}\left[ {{T_{13}}} \right]$ 0 2 ${\mathop{\rm Im}\nolimits} \left[ {{T_{13}}} \right] + j{\mathop{\rm Im}\nolimits} \left[ {{T_{12}}} \right]$ ${\mathop{\rm Im}\nolimits} \left[ {{T_{13}}\left( \theta \right)} \right]$ ${{\mathop{\rm Im}\nolimits} ^2}\left[ {{T_{12}}} \right] + {{\mathop{\rm Im}\nolimits} ^2}\left[ {{T_{13}}} \right]$ 0 2 $ - {\mathop{\rm Im}\nolimits} \left[ {{T_{12}}} \right] + j{\mathop{\rm Im}\nolimits} \left[ {{T_{13}}} \right]$ ${\mathop{\rm Re}\nolimits} \left[ {{T_{23}}\left( \theta \right)} \right]$ $\frac{1}{4}{\left( {{T_{33}} - {T_{22}}} \right)^2} + {{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{23}}} \right]$ 0 4 $\frac{1}{2}\left( {{T_{33}} - {T_{22}}} \right) + j{\mathop{\rm Re}\nolimits} \left[ {{T_{23}}} \right]$ ${T_{22}}\left( \theta \right)$ $\frac{1}{4}{\left( {{T_{33}} - {T_{22}}} \right)^2} + {{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{23}}} \right]$ $\frac{1}{2}\left( {{T_{22}} + {T_{33}}} \right)$ 4 ${\mathop{\rm Re}\nolimits} \left[ {{T_{23}}} \right] + j\frac{1}{2}\left( {{T_{22}} - {T_{33}}} \right)$ ${T_{33}}\left( \theta \right)$ $\frac{1}{4}{\left( {{T_{33}} - {T_{22}}} \right)^2} + {{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{23}}} \right]$ $\frac{1}{2}\left( {{T_{22}} + {T_{33}}} \right)$ 4 $ - {\mathop{\rm Re}\nolimits} \left[ {{T_{23}}} \right] + j\frac{1}{2}\left( {{T_{33}} - {T_{22}}} \right)$ ${\left| {{T_{12}}\left( \theta \right)} \right|^2}$ ${{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{12}}T_{13}^ * } \right] + \frac{1}{4}{\left( {{{\left| {{T_{13}}} \right|}^2} - {{\left| {{T_{12}}} \right|}^2}} \right)^2}$ $\frac{1}{2}\left( {{{\left| {{T_{12}}} \right|}^2} + {{\left| {{T_{13}}} \right|}^2}} \right)$ 4 ${\mathop{\rm Re}\nolimits} \left[ {{T_{12}}T_{13}^ * } \right] + j\frac{1}{2}\left( {{{\left| {{T_{12}}} \right|}^2} - {{\left| {{T_{13}}} \right|}^2}} \right)$ ${\left| {{T_{13}}\left( \theta \right)} \right|^2}$ ${{\mathop{\rm Re}\nolimits} ^2}\left[ {{T_{12}}T_{13}^ * } \right] + \frac{1}{4}{\left( {{{\left| {{T_{13}}} \right|}^2} - {{\left| {{T_{12}}} \right|}^2}} \right)^2}$ $\frac{1}{2}\left( {{{\left| {{T_{12}}} \right|}^2} + {{\left| {{T_{13}}} \right|}^2}} \right)$ 4 $ - {\mathop{\rm Re}\nolimits} \left[ {{T_{12}}T_{13}^ * } \right] + j\frac{1}{2}\left( {{{\left| {{T_{13}}} \right|}^2} - {{\left| {{T_{12}}} \right|}^2}} \right)$ ${\left| {{T_{23}}\left( \theta \right)} \right|^2}$ $\frac{1}{4}{\left\{ {\frac{1}{4}{{\left( {{T_{33}} - {T_{22}}} \right)}^2} + {{{\mathop{\rm Re}\nolimits} }^2}\left[ {{T_{23}}} \right]} \right\}^2}$ $\begin{array}{l}\frac{1}{2}\left\{ {\frac{1}{4}{{\left( {{T_{33}} - {T_{22}}} \right)}^2} + {{{\mathop{\rm Re}\nolimits} }^2}\left[ {{T_{23}}} \right]} \right\}\\\quad + {{\mathop{\rm Im}\nolimits} ^2}\left[ {{T_{23}}} \right]\end{array}$ 8 $\begin{array}{l}\frac{1}{2}\left( {{T_{33}} - {T_{22}}} \right){\mathop{\rm Re}\nolimits} \left[ {{T_{23}}} \right] \\\quad+ j\frac{1}{2}\left[ {{{{\mathop{\rm Re}\nolimits} }^2}\left[ {{T_{23}}} \right] - \frac{1}{4}{{\left( {{T_{33}} - {T_{22}}} \right)}^2}} \right]\end{array}$ 
下载: 导出CSV
表 2 AIRSAR数据极化相干特征旋转域优化前后对比结果
Table 2. Comparison of polarimetric coherence without and with optimization in rotation domain for AIRSAR data
极化相干特征 原始值均值 最优值均值 增强百分比(%) $\left| {{\gamma _{\left( {{\rm{HH}} + {\rm{VV}}} \right) - \left( {{\rm{HH}} - {\rm{VV}}} \right)}}\left( \theta \right)} \right|$ 0.30 0.33 10.00 $\left| {{\gamma _{\left( {{\rm{HH}} - {\rm{VV}}} \right) - {\rm{HV}}}}\left( \theta \right)} \right|$ 0.11 0.48 336.36 $\left| {{\gamma _{{\rm{HH}} - {\rm{VV}}}}\left( \theta \right)} \right|$ 0.35 0.64 82.86 $\left| {{\gamma _{{\rm{HH}} - {\rm{HV}}}}\left( \theta \right)} \right|$ 0.13 0.45 246.15
下载: 导出CSV
-
[1] Lee J S and Pottier E. Polarimetric Radar Imaging: From Basics to Applications[M]. Boca Raton: CRC Press, 2009. [2] Cloude S R. Polarisation Application in Remote Sensing[M]. Oxford: Oxford University Press, 2009. [3] 金亚秋, 徐丰. 极化散射与SAR遥感信息理论与方法[M]. 北京: 科学出版社, 2008.Jin Ya-qiu and Xu Feng. Theory and Approach for Polarimetric Scattering and Information Retrieval of SAR Remote Sensing[M]. Beijing: Science Press, 2008. [4] 张红, 王超, 刘萌, 等. 极化SAR理论、方法与应用[M]. 北京: 科学出版社, 2015.Zhang Hong, Wang Chao, Liu Meng, et al.. Theory, Approach and Application of Polarimetric SAR[M]. Beijing: Science Press, 2015. [5] Dell’Acqua F and Gamba P. Remote sensing and earthquake damage assessment: Experiences, limits, and perspectives[J]. Proceedings of the IEEE, 2012, 100(10): 2876–2890. doi: 10.1109/JPROC.2012.2196404 [6] Sato M, Chen S W, and Satake M. Polarimetric SAR analysis of Tsunami damage following the March 11, 2011 East Japan Earthquake[J]. Proceedings of the IEEE, 2012, 100(10): 2861–2875. doi: 10.1109/JPROC.2012.2200649 [7] Chen S W and Sato M. Tsunami damage investigation of built-up areas using multitemporal spaceborne full polarimetric SAR images[J]. IEEE Transactions on Geoscience and Remote Sensing, 2013, 51(4): 1985–1997. doi: 10.1109/TGRS.2012.2210050 [8] Chen S W, Wang X S, and Sato M. Urban damage level mapping based on scattering mechanism investigation using fully polarimetric SAR data for the 3.11 East Japan earthquake[J]. IEEE Transactions on Geoscience and Remote Sensing, 2016, 54(12): 6919–6929. doi: 10.1109/TGRS.2016.2588325 [9] 吴一戎. 多维度合成孔径雷达成像概念[J]. 雷达学报, 2013, 2(2): 135–142. http://radars.ie.ac.cn/CN/abstract/abstract93.shtmlWu Yi-rong. Concept of multidimensional space joint-observation SAR[J]. Journal of Radars, 2013, 2(2): 135–142. http://radars.ie.ac.cn/CN/abstract/abstract93.shtml [10] 洪文. 圆迹SAR成像技术研究进展[J]. 雷达学报, 2012, 1(2): 124–135. http://radars.ie.ac.cn/CN/abstract/abstract29.shtmlHong Wen. Progress in circular SAR imaging technique[J]. Journal of Radars, 2012, 1(2): 124–135. http://radars.ie.ac.cn/CN/abstract/abstract29.shtml [11] 张杰, 张晰, 范陈清, 等. 极化SAR在海洋探测中的应用与探讨[J]. 雷达学报, 2016, 5(6): 596–606. http://radars.ie.ac.cn/CN/abstract/abstract390.shtmlZhang Jie, Zhang Xi, Fan Chen-qing, et al.. Discussion on application of polarimetric synthetic aperture radar in marine surveillance[J]. Journal of Radars, 2016, 5(6): 596–606. http://radars.ie.ac.cn/CN/abstract/abstract390.shtml [12] 许成斌, 周伟, 丛瑜, 等. 基于峰值区域的高分辨率极化SAR舰船目标特征分析与鉴别[J]. 雷达学报, 2015, 4(3): 367–373. http://radars.ie.ac.cn/CN/abstract/abstract213.shtmlXu Cheng-bin, Zhou Wei, Cong Yu, et al.. Ship analysis and detection in high-resolution Pol-SAR imagery based on peak zone[J]. Journal of Radars, 2015, 4(3): 367–373. http://radars.ie.ac.cn/CN/abstract/abstract213.shtml [13] 王雪松. 雷达极化技术研究现状与展望[J]. 雷达学报, 2016, 5(2): 119–131. http://radars.ie.ac.cn/CN/abstract/abstract346.shtmlWang Xue-song. Status and prospects of radar polarimetry techniques[J]. Journal of Radars, 2016, 5(2): 119–131. http://radars.ie.ac.cn/CN/abstract/abstract346.shtml [14] 杨汝良, 戴博伟, 李海英. 极化合成孔径雷达极化层次和系统工作方式[J]. 雷达学报, 2016, 5(2): 132–142. http://radars.ie.ac.cn/CN/abstract/abstract337.shtmlYang Ru-liang, Dai Bo-wei, and Li Hai-ying. Polarization hierarchy and system operating architecture for polarimetric synthetic aperture radar[J]. Journal of Radars, 2016, 5(2): 132–142. http://radars.ie.ac.cn/CN/abstract/abstract337.shtml [15] 代大海, 廖斌, 肖顺平, 等. 雷达极化信息获取与处理的研究进展[J]. 雷达学报, 2016, 5(2): 143–155. http://radars.ie.ac.cn/CN/abstract/abstract316.shtmlDai Da-hai, Liao Bin, Xiao Shun-ping, et al.. Advancements on radar polarization information acquisition and processing[J]. Journal of Radars, 2016, 5(2): 143–155. http://radars.ie.ac.cn/CN/abstract/abstract316.shtml [16] 赵春雷, 王亚梁, 阳云龙, 等. 雷达极化信息获取及极化信号处理技术研究综述[J]. 雷达学报, 2016, 5(6): 620–638. http://radars.ie.ac.cn/CN/abstract/abstract389.shtmlZhao Chun-lei, Wang Ya-liang, Yang Yun-long, et al.. Review of radar polarization information acquisition and polarimetric signal processing techniques[J]. Journal of Radars, 2016, 5(6): 620–638. http://radars.ie.ac.cn/CN/abstract/abstract389.shtml [17] Huynen J R. Phenomenological Theory of Radar Targets[D]. [Ph.D. dissertation], Delft University of Technology, 1970. [18] Cloude S R and Pottier E. An entropy based classification scheme for land applications of polarimetric SAR[J]. IEEE Transactions on Geoscience and Remote Sensing, 1997, 35(1): 68–78. doi: 10.1109/36.551935 [19] Touzi R. Target scattering decomposition in terms of roll-invariant target parameters[J]. IEEE Transactions on Geoscience and Remote Sensing, 2007, 45(1): 73–84. doi: 10.1109/TGRS.2006.886176 [20] Paladini R, Martorella M, and Berizzi F. Classification of man-made targets via invariant coherency-matrix eigenvector decomposition of polarimetric SAR/ISAR images[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(8): 3022–3034. doi: 10.1109/TGRS.2011.2116121 [21] Chen S W, Li Y Z, Wang X S, et al.. Modeling and interpretation of scattering mechanisms in polarimetric synthetic aperture radar: Advances and perspectives[J]. IEEE Signal Processing Magazine, 2014, 31(7): 79–89. [22] Xu F and Jin Y Q. Deorientation theory of polarimetric scattering targets and application to terrain surface classification[J]. IEEE Transactions on Geoscience and Remote Sensing, 2005, 43(10): 2351–2364. doi: 10.1109/TGRS.2005.855064 [23] An W T, Cui Y, and Yang J. Three-component model-based decomposition for polarimetric SAR data[J]. IEEE Transactions on Geoscience and Remote Sensing, 2010, 48(6): 2732–2739. doi: 10.1109/TGRS.2010.2041242 [24] Yamaguchi Y, Sato A, Boerner W M, et al.. Four-component scattering power decomposition with rotation of coherency matrix[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(6): 2251–2258. doi: 10.1109/TGRS.2010.2099124 [25] Van Zyl J J, Arii M, and Kim Y. Model-based decomposition of polarimetric SAR covariance matrices constrained for nonnegative eigenvalues[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(9): 3452–3459. doi: 10.1109/TGRS.2011.2128325 [26] Arii M, Van Zyl J J, and Kim Y. Adaptive model-based decomposition of polarimetric SAR covariance matrices[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(3): 1104–1113. doi: 10.1109/TGRS.2010.2076285 [27] Neumann M, Ferro-Famil L, and Reigber A. Estimation of forest structure, ground, and canopy layer characteristics from multibaseline polarimetric interferometric SAR data[J]. IEEE Transactions on Geoscience and Remote Sensing, 2010, 48(3): 1086–1104. doi: 10.1109/TGRS.2009.2031101 [28] Chen S W, Wang X S, Xiao S P, et al.. General polarimetric model-based decomposition for coherency matrix[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(3): 1843–1855. doi: 10.1109/TGRS.2013.2255615 [29] Ballester-Berman J D and Lopez-Sanchez J M. Applying the Freeman-Durden decomposition concept to polarimetric SAR interferometry[J]. IEEE Transactions on Geoscience and Remote Sensing, 2010, 48(1): 466–479. doi: 10.1109/TGRS.2009.2024304 [30] Chen S W, Wang X S, Li Y Z, et al.. Adaptive model-based polarimetric decomposition using PolInSAR coherence[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(3): 1705–1718. doi: 10.1109/TGRS.2013.2253780 [31] Wang C L, Yu W D, Wang R, et al.. Comparison of nonnegative eigenvalue decompositions with and without reflection symmetry assumptions[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(4): 2278–2287. doi: 10.1109/TGRS.2013.2259177 [32] Li H Z, Wang C, Zhang H, et al.. A unified three-component scattering model for polarimetric coherent target decomposition[J]. International Journal of Remote Sensing, 2012, 33(9): 2868–2891. doi: 10.1080/01431161.2011.622728 [33] Zhu F Y, Zhang Y H, and Li D. An extension of a complete model-based decomposition of polarimetric SAR data[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(2): 287–291. doi: 10.1109/LGRS.2015.2511076 [34] Deng L and Yan Y N. Improving the Yamaguchi4 decomposition method using selective polarization orientation compensation[J]. Canadian Journal of Remote Sensing, 2016, 42(2): 125–135. doi: 10.1080/07038992.2016.1160774 [35] Zou B, Zhang Y, Cao N, et al.. A four-component decomposition model for PolSAR data using asymmetric scattering component[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2015, 8(3): 1051–1061. doi: 10.1109/JSTARS.2014.2380151 [36] Wang Y H, Liu H W, and Jiu B. PolSAR coherency matrix decomposition based on constrained sparse representation[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(9): 5906–5922. doi: 10.1109/TGRS.2013.2293663 [37] Cloude S R and Pottier E. A review of target decomposition theorems in radar polarimetry[J]. IEEE Transactions on Geoscience and Remote Sensing, 1996, 34(3): 498–518. [38] 张腊梅, 段宝龙, 邹斌. 极化SAR图像目标分解方法的研究进展[J]. 电子与信息学报, 2016, 38(12): 3289–3297. http://youxian.cnki.com.cn/yxdetail.aspx?filename=LDAX20170807001&dbname=CAPJ2015Zhang Lamei, Duan Baolong, and Zou Bin. Research development on target decomposition method of polarimetric SAR image[J]. Journal of Electronics & Information Technology, 2016, 38(12): 3289–3297. http://youxian.cnki.com.cn/yxdetail.aspx?filename=LDAX20170807001&dbname=CAPJ2015 [39] Chen S W, Ohki M, Shimada M, et al.. Deorientation effect investigation for model-based decomposition over oriented built-up areas[J]. IEEE Geoscience and Remote Sensing Letters, 2013, 10(3): 273–277. [40] Chen S W, Wang X S, and Sato M. Uniform polarimetric matrix rotation theory and its applications[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(8): 4756–4770. doi: 10.1109/TGRS.2013.2284359 [41] Chen S W, Li Y Z, and Wang X S. A visualization tool for polarimetric SAR data investigation[C]. The 11th European Conference on Synthetic Aperture Radar, Hamburg, Germany, 2016: 579–582. [42] Chen S W and Wang X S. Polarimetric coherence pattern: A visualization tool for PolSAR data investigation[C]. The IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Beijing, China, 2016: 7509–7512. [43] Xiao S P, Chen S W, Chang Y L, et al.. Polarimetric coherence optimization and its application for manmade target extraction in PolSAR data[J]. IEICE Transactions on Electronics, 2014, 97(6): 566–574. [44] Chen S W, Li Y Z, and Wang X S. Crop discrimination based on polarimetric correlation coefficients optimization for PolSAR data[J]. International Journal of Remote Sensing, 2015, 36(16): 4233–4249. doi: 10.1080/01431161.2015.1079345 [45] Lee J S, Schuler D L, Ainsworth T L, et al.. On the estimation of radar polarization orientation shifts induced by terrain slopes[J]. IEEE Transactions on Geoscience and Remote Sensing, 2002, 40(1): 30–41. doi: 10.1109/36.981347 [46] Chen S W, Wang X S, and Sato M. PolInSAR complex coherence estimation based on covariance matrix similarity test[J]. IEEE Transactions on Geoscience and Remote Sensing, 2012, 50(11): 4699–4710. doi: 10.1109/TGRS.2012.2192937 [47] 陶臣嵩, 陈思伟, 李永祯, 等. 结合旋转域极化特征的极化SAR地物分类[J]. 雷达学报, 录用待刊. doi: 10.12000/JR16131Tao Chensong, Chen Siwei, Li Yongzhen, et al. Polarimetric SAR terrain classification using polarimetric feature derived from rotation domain[J]. Journal of Radars. doi: 10.12000/JR16131 [48] Ainsworth T L, Schuler D L, and Lee J S. Polarimetric SAR characterization of man-made structures in urban areas using normalized circular-pol correlation coefficients[J]. Remote Sensing of Environment, 2008, 112(6): 2876–2885. doi: 10.1016/j.rse.2008.02.005 [49] Yamaguchi Y, Yamamoto Y, Yamada H, et al.. Classification of terrain by implementing the correlation coefficient in the circular polarization basis using X-band POLSAR data[J]. IEICE Transactions on Communications, 2008, E91B(1): 297–301. -
计量
- 文章访问数: 5072
- HTML全文浏览量: 1035
- PDF下载量: 732
- 被引次数: 0
