一种同时全极化雷达正交多相编码波形设计方法

王福来; 庞晨; 李永祯; 王雪松

doi:10.12000/JR16150

一种同时全极化雷达正交多相编码波形设计方法

DOI: 10.12000/JR16150

王福来^1, ,,
庞晨^1,,
李永祯¹,
王雪松¹²

①.
国防科学技术大学电子信息系统复杂电磁环境效应国家重点实验室长沙 410073
②.
国防科学技术大学理学院长沙 410073

基金项目: 国家自然科学基金（61490690，61490694，61501478）

详细信息

作者简介:
王福来(1993–)，男，辽宁人，国防科技大学电子科学与工程学院在读硕士生，主要研究方向为极化信息处理、雷达目标分辨与识别技术。E-mail: wangfulai12@nudt.edu.cn

庞晨：庞晨(1986–)，男，湖北人，博士，国防科技大学电子科学与工程学院讲师，主要研究方向为极化信息处理、雷达目标分辨与识别技术。E-mail: pangchen@nudt.edu.cn

李永祯(1977–)，男，内蒙古人，博士，国防科技大学电子科学与工程学院研究员，主要研究方向为雷达极化信息处理、空间电子对抗、目标检测与识别

王雪松(1972–)，男，内蒙古人，博士，教授，国防科技大学理学院院长，主要研究方向为极化信息处理、雷达目标识别、新体制雷达技术

通讯作者:
王福来 wangfulai12@nudt.edu.cn

计量
- 文章访问数: 4094
- HTML全文浏览量: 1109
- PDF下载量: 667
- 被引次数: 0
出版历程
- 收稿日期: 2016-12-22
- 修回日期: 2017-02-20
- 网络出版日期: 2017-08-28

Orthogonal Polyphase Coded Waveform Design Method for Simultaneous Fully Polarimetric Radar

①.
State Key Laboratory of Complex Electromagnetic Environment Effects on Electronics and Information System, National University of Defense Technology, Changsha 410073, China
②.
School of Science, National University of Defense Technology, Changsha 410073, China

Funds: The National Natural Science Foundation of China (61490690, 61490694, 61501478)

摘要

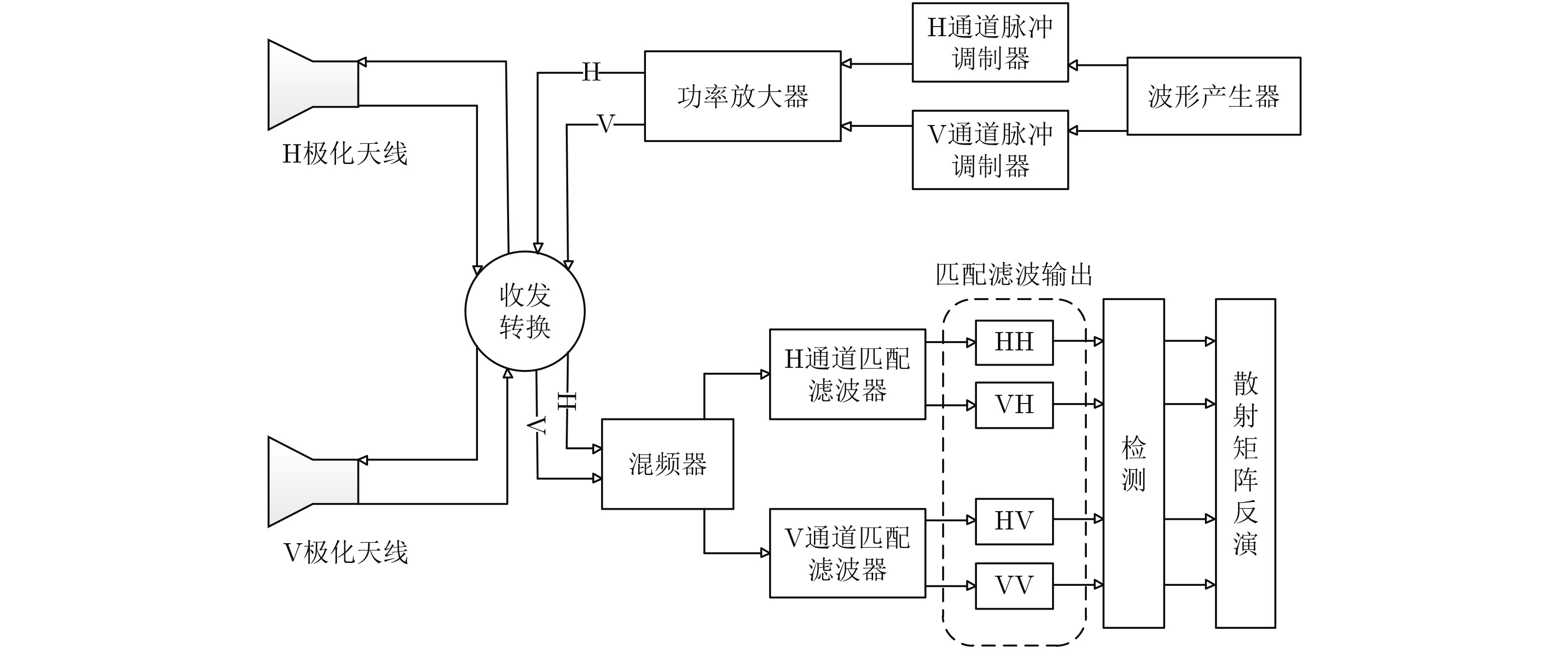
摘要: 为了获得精确的极化散射矩阵测量结果，同时全极化测量体制雷达对发射信号的正交性能提出了很高要求。传统的设计方法得到的正交多相编码波形正交性能受码长的限制，同时对多普勒频移比较敏感。该文提出一种具有较好多普勒容限的正交多相编码波形优化设计方法，针对目标匀速和匀加速运动状态，考虑波形的峰值旁瓣比和波形隔离度等指标，将波形设计问题转化为非线性优化问题，利用遗传算法进行求解。仿真结果表明，相比于Deng, Khan等人提出的编码，该文设计的正交多相编码波形具有更好的多普勒容限，同时峰值旁瓣比和正交性能提升约为1.5～2 dB，能够提高同时全极化测量体制雷达的测量精度。
- 雷达 /
- 同时全极化测量 /
- 正交多相编码 /
- 多普勒容限
Abstract: To obtain an accurate polarization scattering matrix, simultaneous full polarization radar systems must transmit two signals. The performance of orthogonal polyphase codes designed by the traditional method is limited by the code length and is sensitive to Doppler frequency. In this paper, we propose a design method for orthogonal polyphase codes that have good Doppler tolerance. We consider the peak sidelobe level and isolation and transform the signal design problem into a nonlinear optimization problem, which we solve using a genetic algorithm. Our simulation results show that our proposed orthogonal polyphase codes have better Doppler tolerance and their peak sidelobe levels and orthogonal performances are 1.5～2 dB better than the codes designed by Deng or Khan. As such, the new design can improve the measurement accuracy of simultaneous full polarization radar systems.
- Radar /
- Simultaneous full polarization /
- Orthogonal poly-phase codes /
- Doppler tolerance

HTML全文

图 1 同时全极化测量雷达信号处理流程图

Figure 1. Signal processing flow chart of simultaneous full-polarization radar

下载: 全尺寸图片幻灯片

图 2 GA算法流程图

Figure 2. Flow chart of GA algorithm

下载: 全尺寸图片幻灯片

图 3 静止目标仿真结果图

Figure 3. Simulation results of stationary target

下载: 全尺寸图片幻灯片

图 4 匀速运动(v₀T=0.38)目标仿真结果图

Figure 4. Simulation results of uniform motional (v₀T=0.38) target

下载: 全尺寸图片幻灯片

图 5 匀加速运动(aT ²=0.55)目标仿真结果图

Figure 5. Simulation results of uniformly accelerated motional (aT ²=0.55) target

下载: 全尺寸图片幻灯片

图 6 相关函数随目标运动速度增加变化仿真结果图

Figure 6. Simulation results of variation chart of correlation function with increase of speed

下载: 全尺寸图片幻灯片

表 2 N=40时算法仿真结果(dB)

Table 2. Simulation results of N=40 (dB)

算法	${v_0} = 0$, α = 0		${v_0}T = 0.2$, α = 0		${v_0} = 0$, αT ² = 0.25
算法	PSL	I	PSL	I	PSL	I
本文编码(G=10)	–16.1	–14.2	–15.9	–13.9	–15.9	–14.0
Deng编码^[5]	–16.3	–14.0	–15.7	–13.6	–15.6	–13.5
Khan编码^[12]	–16.0	–7.3	–16.0	–7.1	–15.9	–7.2

下载: 导出CSV

表 3 N=512时算法仿真结果(dB)

Table 3. Simulation results of N=512 (dB)

算法	${v_0} = 0$, α = 0		${v_0}T = 0.8$, α = 0		${v_0} = 0$, αT ² = 0.9
算法	PSL	I	PSL	I	PSL	I
本文编码(G=64)	–23.8	–22.9	–17.4	–17.1	–15.8	–15.7
Deng编码^[5]	–22.5	–21.7	–15.9	–15.5	–14.3	–14.0

下载: 导出CSV

表 1 编码波形优化设计主要参数

Table 1. Main parameters for optimization of poly-phase codes

参数	数值
载波频率f₀ (MHz)	100
信号时宽T (ms)	0.128
信号带宽B (MHz)	1
相位编码数M	4
子序列数G	32
交叉概率	0.7
变异概率	0.1
种群数量	100
最大遗传代数	300

下载: 导出CSV

参考文献(16)

[1]	Melvin L S and Banner G P. Radars for the detection and tracking of ballistic missiles, satellites, and planets[J]. Lincoln laboratory Journal, 2000, 12(2): 217–244.
[2]	Giuli D, Fossi M, and Facheris L. Radar target scattering matrix measurement through orthogonal signals[J]. Radar and Signal Processing,IEE Proceeding F, 1993, 140(4): 233–242.
[3]	李永祯, 王雪松, 曾永虎, 等. 宽带雷达目标的瞬态极化时频分布表征[J]. 雷达科学与技术, 2004, 2(6): 321–326 Li Yong-zhen, Wang Xue-song, Zeng Yong-hu, et al. Time-Frequency Distribution Characterization of Radar Targets’ Instantaneous Polarization Scattering[J]. Radar Science and Technology, 2004, 2(6): 321–326
[4]	Babur G, Krasnov O, Yarovoy A, et al. Nearly Orthogonal Waveforms for MIMO FMCW Radar[J]. IEEE Transactions onAerospace and Electronic Systems, 2013, 49(3): 1426–1437. DOI: 10.1109/TAES.2013.6557996
[5]	Deng H. Polyphase code design for orthogonal netted radar systems[J]. IEEE Trans on Signal Processing, 2004, 52(11): 3126–3135. DOI: 10.1109/TSP.2004.836530
[6]	Liu B. Orthogonal Discrete Frequency-Coding Waveform Set Design with Minimized Autocorrelation Sidelobes[J]. IEEE Transactions on Aerospace and Electronic Systems, 2009, 45(4): 1650–1657. DOI: 10.1109/TAES.2009.5310326
[7]	Gao C, Teh K C, and Liu A. Orthogonal Frequency Diversity Waveform with Range-Doppler Optimization for MIMO Radar[J]. IEEE Signal Processing Letters, 2014, 21(10): 1201–1205. DOI: 10.1109/LSP.2014.2329944
[8]	Wang Z, Tigrek F, Krasnov O, et al. Interleaved OFDM Radar for Simultaneous Polarimetric Measurement[J]. IEEE Transactions on Aerospace and Electronic Systems, 2012, 48(3): 2085–2099. DOI: 10.1109/TAES.2012.6237580
[9]	Brunkow D A, George J, Bring V N, et al.. Recent data system and antenna upgrades to the CSU-CHILL radar[C]. AMS 32nd Conference on Radar Meteorology, Albuquerque, New Mexico, USA, 2005.
[10]	Xiao Jin-jun and Arye Nehorai. Joint Transmitter and Receiver Polarization Optimization for Scattering Estimation in Clutter[J]. IEEE Transaction on Signal Processing, 2009, 57(10): 4142–4147. DOI: 10.1109/TSP.2009.2022887
[11]	Nadav Levanon and Eli Mozeson. Radar Signals[M]. Hoboken, New Jersey: John Wiley & Sons, 2004: 101–103.
[12]	Khan H A and Edwards D J. Doppler problems in orthogonal MIMO radar[C]. IEEE Conference on Radar, Verona, NY, USA, April 24–27, 2006, Vol. 1: 24–27.
[13]	Frank R L. Polyphase Complementary-Codes[J]. IEEE Transactions on Information Theory, 1980, 26: 641–647. DOI: 10.1109/TIT.1980.1056272
[14]	Lei Y J, Zhang S W, Li X W, et al.. MATLAB GA Algorithm Toolbox and It’s Applications[M]. Xi’an: Xidian Press, 2005: 66–70.
[15]	刘波. MIMO雷达正交波形设计及信号处理研究[D]. [博士论文], 电子科技大学, 2008. Liu Bo. Study on orthogonal waveform design and signal processing for MIMO radar[D]. [Ph.D. dissertation], University of Electronic Science and Technology of China, 2008.
[16]	王璐璐, 王宏强, 王满喜, 等. 雷达目标检测的最优波形设计综述[J]. 雷达学报, 2016, 5(5): 487–498 Wang Lulu, Wang Hongqiang, Wang Manxi, et al. An Overview of Radar Waveform Optimization for Target Detection[J]. Journal of Radars, 2016, 5(5): 487–498