Submit Manuscript
Peer Review
Editor Work
| Citation: | WANG Xinhai, WANG Chaoyu, ZHANG Ning, et al. Phase-only method for designing a unimodular radar waveform with low ISL[J]. Journal of Radars, 2022, 11(2): 255–263. doi: 10.12000/JR21137
|
Phase-only Method for Designing a Unimodular Radar Waveform with Low ISL
DOI: 10.12000/JR21137
More Information-
Abstract
Radar waveform optimization has recently drawn much attention. The radar waveform possesses not only constant amplitude but also a low autocorrelation sidelobe level. However, because of the presence of the constant modular constraint, the problem of optimizing the waveform is non-convex, which is difficult to address. The feasible domain used by the current methods usually contains the vector space with two dimensions: amplitude and phase. The optimization procedures accompanied by the constant constraint enlarge the difficulty and amount of calculation. Herein, the problem of designing unimodular sequences with low autocorrelation sidelobes is addressed, and a novel approach based on phase optimization is presented. The feasible domain is compressed into the vector space with only the phase dimension. The proposed method conducts a deep analysis of the relationship between the phases of the elements in the unimodular sequence and successively updates the vector with a closed-form solution in an element-by-element manner at each iteration using the coordinate descent method, which comprises low computational complexity. By compressing the feasible domain and updating the vector variable using the closed solution, the integrated sidelobe level and computation efficiency are improved. Representative numerical simulations are provided to verify the effectiveness of the proposed method. -
-
References
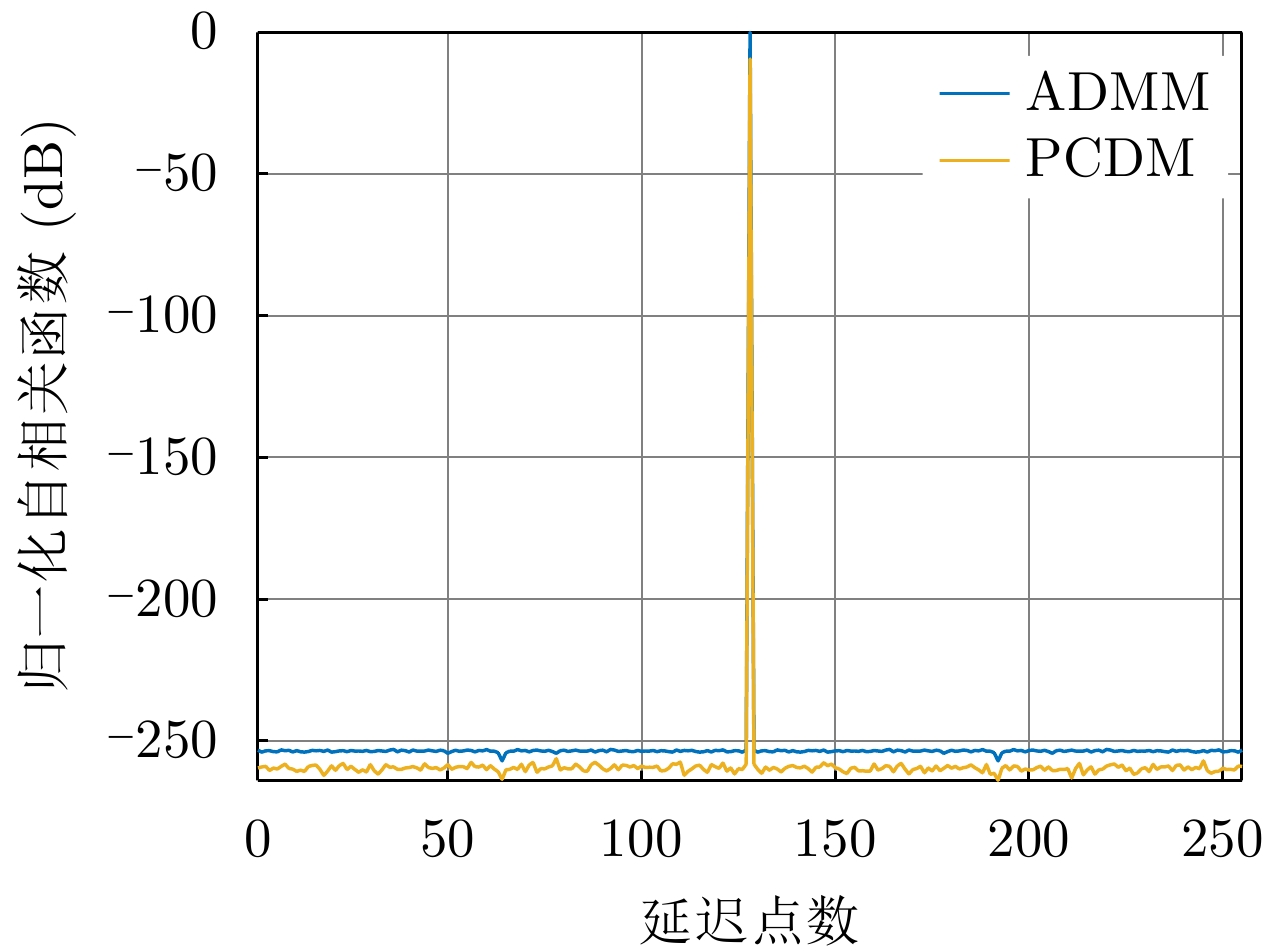
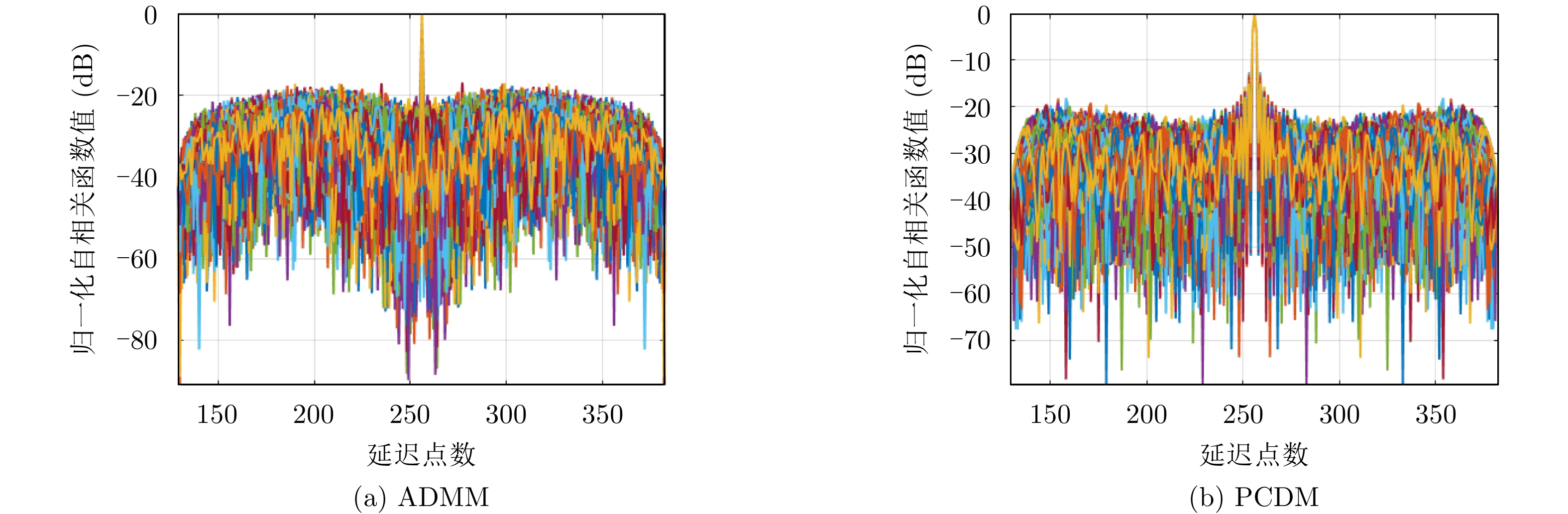
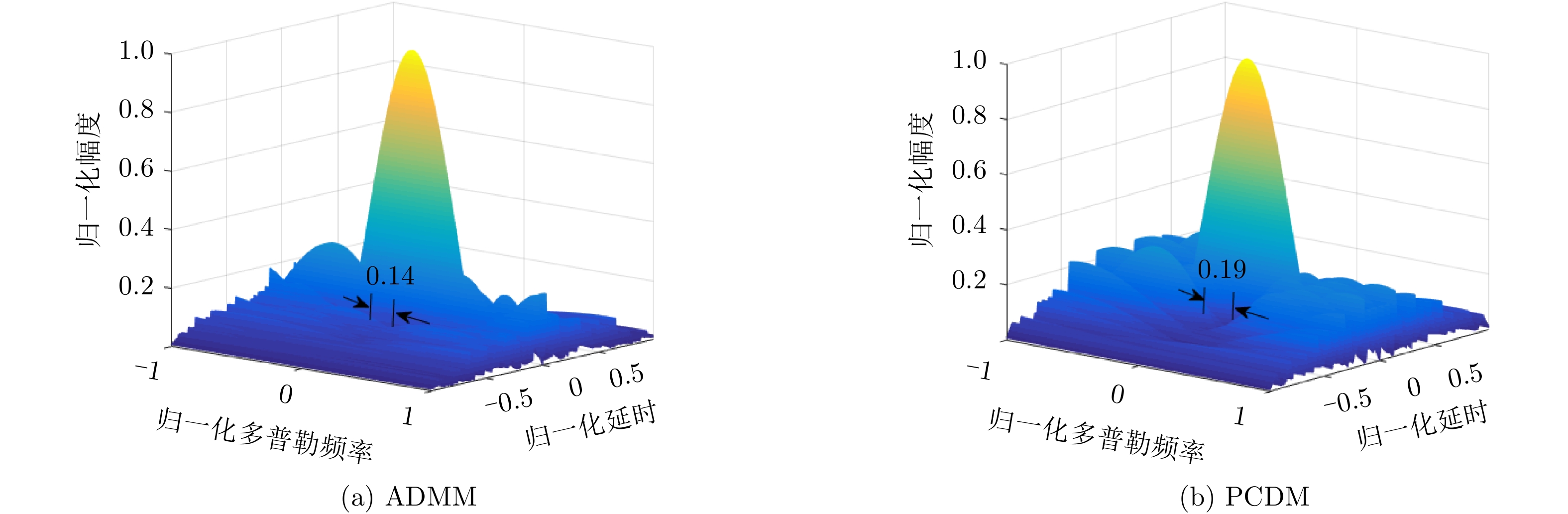
[1] GINI F, DE MAIO A, and PATTON L. Waveform Design and Diversity for Advanced Radar Systems[M]. London, UK: Institution of Engineering and Technology, 2012: 5.[2] SKOLNIK M I. Introduction to Radar Systems[M]. 3rd ed. New York, USA: McGraw-Hill Education (Asia) Co. , 2007. 10–56.[3] WANG Xinhai, ZHANG Gong, ZHANG Yu, et al. Design of spectrally compatible waveform with constant modulus for colocated multiple-input multiple-output radar[J]. IET Radar, Sonar & Navigation, 2019, 13(8): 1373–1388. doi: 10.1049/iet-rsn.2019.0009[4] DAVIS M E and PILLAI S U. Waveform diversity for ultra-wide band surveillance radars[J]. IET Radar, Sonar & Navigation, 2014, 8(9): 1226–1233. doi: 10.1049/iet-rsn.2014.0366[5] GOLAY M. A class of finite binary sequences with alternate auto-correlation values equal to zero (Corresp. )[J]. IEEE Transactions on Information Theory, 1972, 18(3): 449–450. doi: 10.1109/TIT.1972.1054797[6] BARKER R H. Group Synchronizing of Binary Digital Systems[M]. JACKSON W. Communication Theory. New York: Academic Press, 1953: 273–287.[7] GOLAY M. Sieves for low autocorrelation binary sequences[J]. IEEE Transactions on Information Theory, 1977, 23(1): 43–51. doi: 10.1109/TIT.1977.1055653[8] MERTENS S. Exhaustive search for low-autocorrelation binary sequences[J]. Journal of Physics A:Mathematical and General, 1996, 29(18): L473–L481. doi: 10.1088/0305-4470/29/18/005[9] KOCABAS S E and ATALAR A. Binary sequences with low aperiodic autocorrelation for synchronization purposes[J]. IEEE Communications Letters, 2003, 7(1): 36–38. doi: 10.1109/LCOMM.2002.807438[10] JEDWAB J. A survey of the merit factor problem for binary sequences[C]. Third International Conference on Sequences and Their Applications, Seoul, Korea, 2005: 30–55. doi: 10.1007/11423461_2.[11] WANG S. Efficient heuristic method of search for binary sequences with good aperiodic autocorrelations[J]. Electronics Letters, 2008, 44(12): 731–732. doi: 10.1049/el:20081058[12] FRANK R. Polyphase codes with good nonperiodic correlation properties[J]. IEEE Transactions on Information Theory, 1963, 9(1): 43–45. doi: 10.1109/TIT.1963.1057798[13] ZHANG N and GOLOMB S W. Polyphase sequence with low autocorrelations[J]. IEEE Transactions on Information Theory, 1993, 39(3): 1085–1089. doi: 10.1109/18.256535[14] NUNN C J and COXSON G E. Polyphase pulse compression codes with optimal peak and integrated sidelobes[J]. IEEE Transactions on Aerospace and Electronic Systems, 2009, 45(2): 775–781. doi: 10.1109/TAES.2009.5089560[15] DE MAIO A, DE NICOLA S, HUANG Yongwei, et al. Design of phase codes for radar performance optimization with a similarity constraint[J]. IEEE Transactions on Signal Processing, 2009, 57(2): 610–621. doi: 10.1109/TSP.2008.2008247[16] CUI Guolong, JING Ying, LU Shuping, et al. Dual-Use Unimodular Sequence Design via Frequency Nulling Modulation[J]. IEEE Access, 2018(6): 62470–62481. doi: 10.1109/ACCESS.2018.2876644[17] SOLTANALIAN M and STOICA P. Computational design of sequences with good correlation properties[J]. IEEE Transactions on Signal Processing, 2012, 60(5): 2180–2193. doi: 10.1109/TSP.2012.2186134[18] STOICA P, HE Hao, and LI Jian. New algorithms for designing unimodular sequences with good correlation properties[J]. IEEE Transactions on Signal Processing, 2009, 57(4): 1415–1425. doi: 10.1109/TSP.2009.2012562[19] STOICA P, HE Hao, and LI Jian. On designing sequences with impulse-like periodic correlation[J]. IEEE Signal Processing Letters, 2009, 16(8): 703–706. doi: 10.1109/LSP.2009.2021378[20] SONG Junxiao, BABU P, and PALOMAR D P. Optimization methods for designing sequences with low autocorrelation sidelobes[J]. IEEE Transactions on Signal Processing, 2015, 63(15): 3998–4009. doi: 10.1109/TSP.2015.2425808[21] BORWEIN P and FERGUSON R. Polyphase sequences with low autocorrelation[J]. IEEE Transactions on Information Theory, 2005, 51(4): 1564–1567. doi: 10.1109/TIT.2004.842778[22] LIANG Junli, SO H C, LEUNG C S, et al. Waveform design with unit modulus and spectral shape constraints via Lagrange programming neural network[J]. IEEE Journal of Selected Topics in Signal Processing, 2015, 9(8): 1377–1386. doi: 10.1109/JSTSP.2015.2464178[23] LIANG Junli, SO H C, LI Jian, et al. Unimodular sequence design based on alternating direction method of multipliers[J]. IEEE Transactions on Signal Processing, 2016, 64(20): 5367–5381. doi: 10.1109/TSP.2016.2597123[24] BOYD S, PARIKH N, CHU E, et al. Distributed optimization and statistical learning via the alternating direction method of multipliers[J]. Foundations and Trends ® in Machine Learning, 2011, 3(1): 1–122. doi: 10.1561/2200000016[25] ZHANG Jindong, ZHU Daiyin, and ZHANG Gong. New antivelocity deception jamming technique using pulses with adaptive initial phases[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(2): 1290–1300. doi: 10.1109/TAES.2013.6494414[26] BONNANS J F, GILBERT J C, LEMARÉCHAL C, et al. Numerical Optimization: Theoretical and Practical Aspects[M]. Berlin, Heidelberg: Springer, 2006, 67–76. doi: 10.1007/978-3-540-35447-5.[27] TANG Liang, ZHU Yongfeng, and FU Qiang. Fast algorithm for designing periodic/aperiodic sequences with good correlation and stopband properties[J]. EURASIP Journal on Advances in Signal Processing, 2018, 2018(1): 57. doi: 10.1186/s13634-018-0579-z[28] ESMAEILI-NAJAFABADI H, LEUNG H, and MOO P W. Unimodular waveform design with desired ambiguity function for cognitive radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2020, 56(3): 2489–2496. doi: 10.1109/TAES.2019.2942411[29] GRANT M. CVX: MATLAB software for disciplined convex programming[EB/OL]. http://cvxr.com/cvx, 2020.[30] PEAUCELLE D, HENRION D, LABIT Y, et al. User’s guide for SEDUMI interface 1.04[J]. Siam Journal on Optimization - SIAMJO, 2002. -
Proportional views
- Publishing Ethics
- Journal Insights
- Abstracting & Indexing
- Peer Review Policies
- Guide for Authors
- Conference
- ISSN 2095-283X (Print)ISSN 2097-339X (Online)
- CN 10-1030/TN
- CODEN LXEUAO
About Journal
- Sponsor: China Radio Detection and Ranging Industry Association (CRIA)
- Phone: 010-58887062
- Email：radars@aircas.ac.cn
- Publisher: Leida Xuebao Bianjibu (Editorial office of the Journal of Radars)
Contacts Us
京ICP备20021838号-8
Supported by: Beijing Renhe Information Technology Co. Ltd
Export File
Citation
Format
Content
DownLoad:
- Figure 1. Comparison of the autocorrelations of the periodic waveforms obtained by different methods: ADMM and PCDM
- Figure 2. Comparison of the average autocorrelations of the periodic waveforms obtained by ADMM and PCDM
- Figure 3. The convergence performance of optimizing the period waveform using the different methods: ADMM and PCDM
- Figure 4. Comparison of the autocorrelations of the aperiod waveforms obtained by different methods: ADMM and PCDM
- Figure 5. Comparison of the average autocorrelations of the aperiod waveforms obtained by ADMM and PCDM
- Figure 6. The convergence performance of optimizing the aperiod waveform using the different methods: ADMM and PCDM
- Figure 7. The ambiguity function of the periodic waveform obtained by the different methods: ADMM and PCDM
- Figure 8. The ambiguity function of the aperiodic waveform obtained by the different methods: ADMM and PCDM